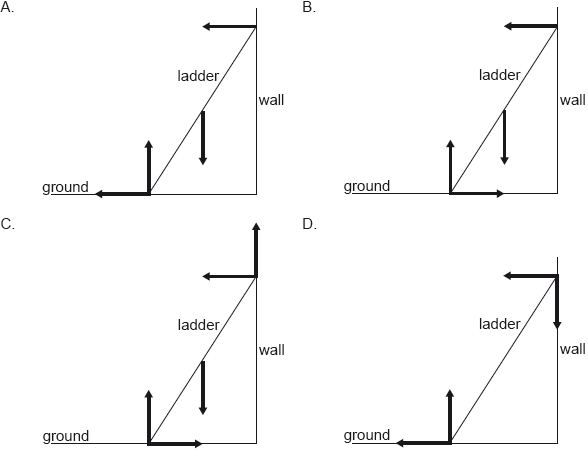
A uniform ladder resting in equilibrium on rough ground leans against a smooth wall. Which diagram correctly shows the forces acting on the ladder?
Answer/Explanation
Markscheme
B
Question
A heat engine does 300 J of work during one cycle. In this cycle 900 J of energy is wasted. What is the efficiency of the engine?
A. 0.25
B. 0.33
C. 0.50
D. 0.75
Answer/Explanation
Markscheme
A
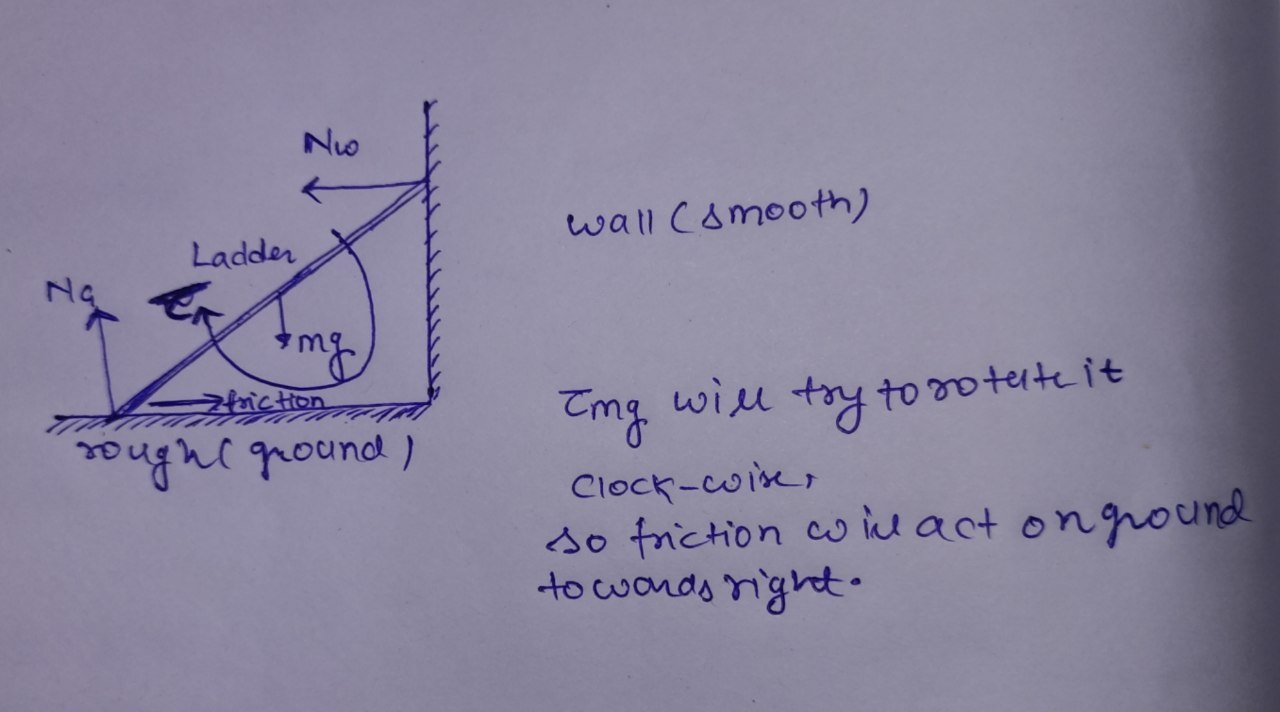
Heat Engine :
A Heat engine is a device which helps us to get an amount of work when connected to two thermal reservoirs, one at a higher temperature and another one at a lower temperature.

In the above diagram, we can see
- Two thermal reservoirs one at a higher temperature \(T_1 \) and at a lower temperature \(T_2 \)
- Heat \(Q_1 \) flows from the higher temperature reservoir to the engine E
- \(W \) is the work obtained in the Engine
- Heat \(Q_2 \) flows from the engine to the cold reservoir.
- \(Q_1=W+Q_2 \)
The efficiency of a thermal reservoir is given as
\(\eta = \frac {W}{Q_1}=\frac {Q_1-Q_2}{Q_1}= \frac {T_1-T_2}{T_1} \)
Given that a heat engine does W = 300 J of work while exhausting \(Q_2=900 J \).
Heat supplied to the heat engine is given by \(Q_1 =W+Q_2 =300J +900J = 1200J \)
The efficiency of the engine is given by
\(\begin{align*} \eta &= \frac {W}{Q_1}\\ &= \frac {300}{1200}\\ &=0.25 \Rightarrow (Answer) \end{align*} \)
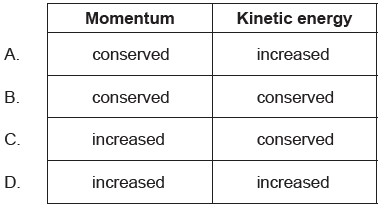
A moving system undergoes an explosion. What is correct for the momentum of the system and the kinetic energy of the system when they are compared immediately before and after the explosion?
Answer/Explanation
Markscheme
A
Explosions occur when energy is transformed from one kind e.g. chemical potential energy to another e.g. heat energy or kinetic energy extremely quickly. So, like in inelastic collisions, total kinetic energy is not conserved in explosions. But total momentum is always conserved.
The chemical energy of the shell is converted to mechanical energy. The external potential energy gets converted to its kinetic energy due to explosion. So kinetic energy increases
The following can be determined for a solid substance.
I. The average kinetic energy \({E_{{{\rm{K}}_{{\rm{ave}}}}}}\) of the molecules
II. The total kinetic energy \({E_{{{\rm{K}}_{{\rm{tot}}}}}}\) of the molecules
III. The total potential energy \({E_{{{\rm{P}}_{{\rm{tot}}}}}}\) of the molecules
Which is/are equal to the internal energy of this solid substance?
A. I only
B. I and III only
C. II only
D. II and III only
Answer/Explanation
Markscheme
D
It’s the sum of total Kinetic energy ( translational + rotational ) of its molecules and the total potential energy of its molecules with respect to each other
for example an ideal mono-atomic gas each molecule has 3 degrees of freedom (all transitional) and no potential energy between the molecules ( no attraction nor repulsion between the molecules) so its internal energy is
\(U=\frac{3}{2}NKT\)
Where N is the number of the molecules and K is the Boltzmann’s constant and finally T is the Temperature in absolute scale ( kelvin if you use SI, Rankine if you use imperial units)