Question
A solid cylinder of height h and density ρ rests on a flat surface.
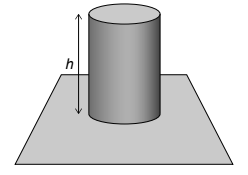
(a) Show that the pressure pC exerted by the cylinder on the surface is given by pC = ρgh. [2]
A tube of constant circular cross-section, sealed at one end, contains an ideal gas trapped by a cylinder of mercury of length 0.035 m. The whole arrangement is in the Earth’s atmosphere. The density of mercury is 1.36 × 104 kg m–3.

When the mercury is above the gas column the length of the gas column is 0.190 m.
(b) (i) Show that (Po + Pm) × 0.190 =\(\frac{nRT}{A}\) where
po = atmospheric pressure
pm = pressure due to the mercury column
T = temperature of the trapped gas
n = number of moles of the trapped gas
A = cross-sectional area of the tube. [2]
The tube is slowly rotated until the gas column is above the mercury

The length of the gas column is now 0.208 m. The temperature of the trapped gas does not change during the process.
(b) (ii) Determine the atmospheric pressure. Give a suitable unit for your answer. [4]
(b) (iii) Outline why the gas particles in the tube hit the mercury surface less often after the tube has been rotated. [1]
Answer/Explanation
Ans:
a weight of cylinder = AhgΡ pressure = \(\frac{F}{A}=\frac{Ahgp}{A}\)
b i use of PV = nRT and V = Area ×(0.190) seen substitution of P = po + pm «re-arrangement to give answer»
b ii recognition that \(\frac{nRT}{A}\) is constant
OR
190po +190pm = 208po – 208pm OR po \(\frac{398}{18}\) pm pressure due to mercury pm = 0.035 × 1.36 × 104 × 9.81 ( =4.67 103 Pa) 1.03 ×105 Pa OR Nm-2 OR kgm-1s -2
b iii same number of particles to collide with a larger surface area OR greater volume with constant rms speed decreases collision frequency