2023-May-Physics_paper_1__TZ1_HL – All Questions with detailed solution
2023-May-Physics_paper_1__TZ1_HL
Topic: Uncertainties and errors
Calculate:\(\frac{\text{Diameter of atom}}{\text{Diameter of nucleus}} ?\)
A. \(10^1\)
B. \(10^3\)
C. \(10^5\)
D. \(10^7\)
▶️Answer/Explanation
Ans:C
The diameter of an atom is much larger than the diameter of its nucleus. The nucleus of an atom is extremely small compared to the size of the entire atom.
The approximate diameter of an atom is on the order of 0.1 nanometers (1 Ångström), while the diameter of the nucleus is on the order of 1 femtometer (1 fm). Therefore, the ratio of the diameter of an atom to the diameter of its nucleus is:
The ratio of the diameter of an atom to the diameter of its nucleus is:
\(\frac{\text{Diameter of atom}}{\text{Diameter of nucleus}} = \frac{0.1\, \text{nm}}{1\, \text{fm}} = 10^5\)
2023-May-Physics_paper_1__TZ1_HL
Topic: Uncertainties and errors
Given:The percentage uncertainties in the measurements are:
 (momentum) p and (mass) m.
(momentum) p and (mass) m.
Calculate: percentage uncertainty in the kinetic energy?
A. 7%
B. 10%
C. 13%
D. 14%
▶️Answer/Explanation
Ans:B
\(\begin{aligned} p^2 & =2 m k \\ k & =\frac{p^2}{2 m} \\ \pm \frac{\Delta k}{k} & = \pm\left(2 \times \frac{\Delta p}{p}+1 \times \frac{\Delta m}{m}\right)\end{aligned}\)
\(\left(\frac{\Delta k}{k} \times 100\right)=2 \times 3+4=10 \%\)
2023-May-Physics_paper_1__TZ1_HL
Topic: Vectors and scalars
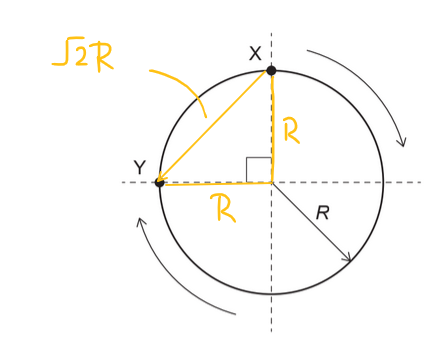
Calculate: magnitude of displacement from X to Y?
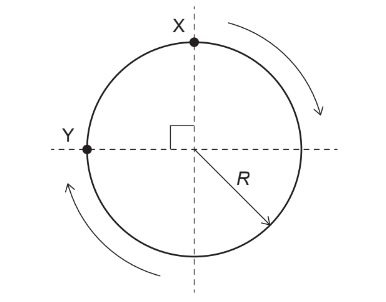
A. \( R \frac{3 \pi}{2}\)
B. \(R \frac{\pi}{2}\)
C. \(R \sqrt{2}\)
D. \(R\)
▶️Answer/Explanation
Ans:C
The displacement from point X to point Y is a chord of the circle and can be represented by the line connecting points X and Y.
2023-May-Physics_paper_1__TZ1_HL
Topic: Motion
Given: A stone with a mass of m is launched vertically upward with an initial speed u from the summit of a cliff. The velocity of the stone at the moment just before it reaches the ground is denoted as v.

Calculate: magnitude of the change in momentum of the stone?
A. \(m\left(\frac{v+u}{2}\right)\)
B. \(m\left(\frac{v-u}{2}\right)\)
C. \(m(v+u)\)
D. \(m(v-u)\)
▶️Answer/Explanation
Ans:C
The initial momentum of the stone, when it is projected vertically upwards with speed \(u\), is \(+mu\) (upwards is considered positive).
The final momentum of the stone, just before it hits the ground, is \(-mv\) (downwards is considered negative).
Now, the change in momentum is:
Change in momentum = Final momentum – Initial momentum
Change in momentum = \(-mv – mu\)
Change in momentum = \(m(-v – u)\)
Change in momentum = \(m(-(v + u))\)
So, the magnitude of the change in momentum is:
\(|m(v + u)|\)
2023-May-Physics_paper_1__TZ1_HL
Topic: Motion
Given:A car accelerates uniformly. The car passes point \(X\) at time \(t_1\) with velocity \(v_1\) and point \(Y\) at time \(t_2\) with velocity \(v_2\). The distance \(X Y\) is \(s\).
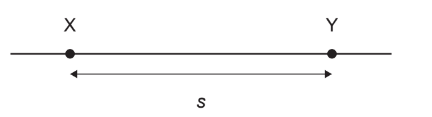
Discuss:Which is correct?
The following expressions are proposed for the magnitude of its acceleration a:
I. \(a=\frac{2 s}{\left(t_2-t_1\right)^2}\)
II. \(a=\frac{v_2^2-v_1^2}{2 s}\)
III. \(a=\frac{v_2-v_1}{t_2-t_1}\)
▶️Answer/Explanation
Ans:C
To determine which expressions for the magnitude of acceleration (\(a\)) are correct, we can analyze each of the proposed expressions one by one:
I. \(a = \frac{2s}{(t_2 – t_1)^2}\)
Expression I is incorrect because it assumes that the initial velocity \(\left(v_1\right)\) is zero, which may not be the case for the uniformly accelerating car.
II. \(a = \frac{v_2^2 – v_1^2}{2s}\)
This expression is also correct. It’s derived from the kinematic equation for uniformly accelerated motion: \(v^2 = u^2 + 2as\), where \(v_2\) is the final velocity, \(v_1\) is the initial velocity, \(s\) is the displacement, and \(a\) is the acceleration. Solving for \(a\) results in this expression.
III. \(a = \frac{v_2 – v_1}{t_2 – t_1}\)
This expression is also correct and is derived from the definition of acceleration as the rate of change of velocity over time.
So, expressions (II, and III) are correct.
