Question
Consider the following reaction.
\[{\text{5B}}{{\text{r}}^ – }{\text{(aq)}} + {\text{BrO}}_3^ – {\text{(aq)}} + {\text{6}}{{\text{H}}^ + }{\text{(aq)}} \to {\text{3B}}{{\text{r}}_2}{\text{(aq)}} + {\text{3}}{{\text{H}}_2}{\text{O(l)}}\]
The rate expression for the reaction is found to be:
\[{\text{rate}} = k{\text{[B}}{{\text{r}}^ – }{\text{][BrO}}_3^ – {\text{][}}{{\text{H}}^ + }{{\text{]}}^2}\]
Which statement is correct?
A. The overall order is 12.
B. Doubling the concentration of all of the reactants at the same time would increase the rate of the reaction by a factor of 16.
C. The units of the rate constant, \(k\), are \({\text{mol}}\,{\text{d}}{{\text{m}}^{ – 3}}{{\text{s}}^{ – 1}}\).
D. A change in concentration of \({\text{B}}{{\text{r}}^ – }\) or \({\text{BrO}}_3^ – \) does not affect the rate of the reaction.
▶️Answer/Explanation
B
As, \[{\text{rate}} = k{\text{[B}}{{\text{r}}^ – }{\text{][BrO}}_3^ – {\text{][}}{{\text{H}}^ + }{{\text{]}}^2}\]
overall order of reaction = 1+1+2 = 4 and a change in concentration of \({\text{B}}{{\text{r}}^ – }\) or \({\text{BrO}}_3^ – \) affects the rate of the reaction.
If we double the concentration of each reactant, rate increases 2*2*22 = 16 times.
Rate = Concentration/time
k = Rate / (Concentration)4
k = Concentration/time/(Concentration)4 = (Concentration)-3 (time)-1= (mol dm-3)-3 (s)-1
Question
The rate expression for a reaction is:
\[{\text{rate}} = k{\text{[X][Y]}}\]
Which statement is correct?
A. As the temperature increases the rate constant decreases.
B. The rate constant increases with increased temperature but eventually reaches a constant value.
C. As the temperature increases the rate constant increases.
D. The rate constant is not affected by a change in temperature.
▶️Answer/Explanation
C
Arrhenius equation is k=Ae−Ea/RT
With increase in temperature, the rate of the reaction and the rate constant increases. As a generalization, the rate of the reaction (and the rate constant) becomes almost double for every ten degree rise in temperature.
Question
Consider the following reaction mechanism.
\[\begin{array}{*{20}{l}} {{\text{Step 1}}}&{{{\text{H}}_2}{{\text{O}}_2} + {{\text{I}}^ – } \to {{\text{H}}_2}{\text{O}} + {\text{I}}{{\text{O}}^ – }}&{{\text{slow}}} \\ {{\text{Step 2}}}&{{{\text{H}}_2}{{\text{O}}_2} + {\text{I}}{{\text{O}}^ – } \to {{\text{H}}_2}{\text{O}} + {{\text{O}}_2} + {{\text{I}}^ – }}&{{\text{fast}}} \end{array}\]
Which statement correctly identifies the rate-determining step and the explanation?
A. Step 2 because it is the faster step
B. Step 1 because it is the slower step
C. Step 1 because it is the first step
D. Step 2 because it is the last step
▶️Answer/Explanation
B
The rate determining step is the slowest step of a chemical reaction that determines the speed (rate) at which the overall reaction proceeds.
Question
The following data were obtained for the reaction between gases A and B.
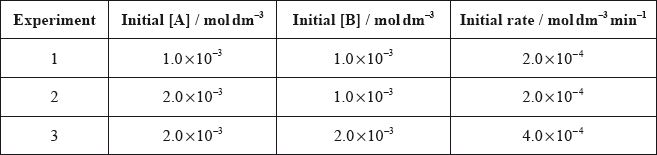
Which relationship represents the rate expression for the reaction?
A. \({\text{rate}} = k{{\text{[B]}}^{\text{2}}}\)
B. \({\text{rate}} = k{{\text{[A]}}^{\text{2}}}\)
C. \({\text{rate}} = k{\text{[A]}}\)
D. \({\text{rate}} = k{\text{[B]}}\)
▶️Answer/Explanation
D
From experiment 1 and 2, As initial concen of A changes, rate does not change. It means rate is independent of concentration of A.
From experiment 2 and 3, As initial concen of B doubles, rate is doubled. It means rate increases linearly with concen of B. Hence, \({\text{rate}} = k{\text{[B]}}\).
Question
Consider the following reaction.
\[{\text{2P}} + {\text{Q}} \to {\text{R}} + {\text{S}}\]
This reaction occurs according to the following mechanism.
\[\begin{array}{*{20}{l}} {{\text{P}} + {\text{Q}} \to {\text{X}}}&{slow} \\ {{\text{P}} + {\text{X}} \to {\text{R}} + {\text{S}}}&{fast} \end{array}\]
What is the rate expression?
A. rate \( = k{\text{[P]}}\)
B. rate \( = k{\text{[P][X]}}\)
C. rate \( = k{\text{[P][Q]}}\)
D. rate \( = k{{\text{[P]}}^{\text{2}}}{\text{[Q]}}\)
▶️Answer/Explanation
C
The rate determining step is the slowest step of a chemical reaction that determines the speed (rate) at which the overall reaction proceeds.
The reaction rate depends on the number of collisions between reactants per unit time, which is proportional to the product of the concentrations of the reactants.
Hence, rate \( = k{\text{[P][Q]}}\)
