Question
An electrochemical cell is made from an iron half-cell connected to a cobalt half-cell:
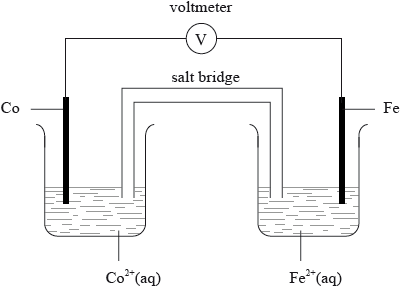
The standard electrode potential for \({\text{F}}{{\text{e}}^{2 + }}{\text{(aq)}} + {\text{2}}{{\text{e}}^ – } \rightleftharpoons {\text{Fe(s)}}\) is –0.45 V. The total cell potential obtained when the cell is operating under standard conditions is 0.17 V. Cobalt is produced during the spontaneous reaction.
An electrolytic cell is made using a very dilute solution of sodium chloride.
Predict the products by giving the relevant half-equation for the reaction occurring at each electrode if the electrolyte of the cell described in part (c) was changed to:
Define the term standard electrode potential and state the meaning of the minus sign in the value of –0.45 V.
Calculate the value for the standard electrode potential for the cobalt half-cell.
Deduce which species acts as the oxidizing agent when the cell is operating.
Deduce the equation for the spontaneous reaction taking place when the iron half-cell is connected instead to an aluminium half-cell.
Explain the function of the salt bridge in an electrochemical cell.
\({{\text{[Co(}}{{\text{H}}_{\text{2}}}{\text{O}}{{\text{)}}_{\text{6}}}{\text{]}}^{2 + }}\)
\({\text{C}}{{\text{o}}_{\text{2}}}{{\text{(S}}{{\text{O}}_{\text{4}}}{\text{)}}_{\text{3}}}\)
\({{\text{[CoC}}{{\text{l}}_{\text{4}}}{\text{]}}^{2 – }}\)
Draw a labelled diagram of the cell. Use an arrow to show the direction of the electron flow and identify the positive and negative electrodes.
Give the formulas of all the ions present in the solution.
Predict the products obtained at each electrode and state the half-equation for the formation of each product.
Deduce the molar ratios of the products obtained at the two electrodes.
concentrated sodium chloride
molten sodium bromide
Answer/Explanation
Markscheme
the voltage obtained when the half-cell is connected to the standard hydrogen electrode;
under standard conditions of 298 K and \({\text{1 mol}}\,{\text{d}}{{\text{m}}^{ – 3}}\) solutions;
electrons flow (in the external circuit) from the half-cell to the hydrogen electrode / the metal in the half-cell is above hydrogen in the ECS / Fe is a better reducing agent than \({{\text{H}}_2}\) / Fe is oxidised more readily than \({{\text{H}}_2}\);
–0.28 V;
\({\text{C}}{{\text{o}}^{2 + }}\)/cobalt(II) ion;
\({\text{2Al}} + {\text{3F}}{{\text{e}}^{2 + }} \to {\text{3Fe}} + {\text{2A}}{{\text{l}}^{3 + }}\);
Award [1] for correct reactants and products and [1] for correctly balanced, ignore states.
Do not accept \( \rightleftharpoons \)
to complete the electrical circuit / OWTTE;
by allowing the movement of ions;
+2;
+3;
+2;
Only penalize once if roman numerals are used or if written as 2+ or 3+.
diagram to show:
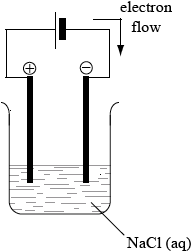
battery/source of electricity connected to two electrodes in the solution with positive and negative electrodes correctly labelled;
electrons/current flowing from the cell to the negative electrode;
labelled solution of sodium chloride;
If the connecting wires to electrodes are immersed in the solution [1 max].
\({\text{N}}{{\text{a}}^ + }\), \({{\text{H}}^ + }{\text{/}}{{\text{H}}_{\text{3}}}{{\text{O}}^ + }\), \({\text{C}}{{\text{l}}^ – }\), \({\text{O}}{{\text{H}}^ – }\)
All four correct [2], any three correct [1].
hydrogen at (–)/cathode and oxygen at (+)/anode;
\({\text{2}}{{\text{H}}^ + } + {\text{2}}{{\text{e}}^ – } \to {{\text{H}}_2}{\text{ / 2}}{{\text{H}}_2}{\text{O}} + {\text{2}}{{\text{e}}^ – } \to {{\text{H}}_{\text{2}}} + 2{\text{O}}{{\text{H}}^ – }\);
\({\text{4O}}{{\text{H}}^ – } \to {{\text{O}}_{\text{2}}} + 2{{\text{H}}_2}{\text{O}} + {\text{4}}{{\text{e}}^ – }{\text{ / 2}}{{\text{H}}_2}{\text{O}} \to {{\text{O}}_2} + {\text{4}}{{\text{H}}^ + } + {\text{4}}{{\text{e}}^ – }\);
Accept e instead of e–
If electrodes omitted or wrong way round [2 max].
Ratio of \({{\text{H}}_{\text{2}}}:{{\text{O}}_{\text{2}}}\) is \(2:1\);
\({\text{(}} – {\text{)/(cathode) 2}}{{\text{H}}^ + } + {\text{2}}{{\text{e}}^ – } \to {{\text{H}}_2}/{\text{2}}{{\text{H}}_2}{\text{O}} + {\text{2}}{{\text{e}}^ – } \to {{\text{H}}_2} + {\text{2O}}{{\text{H}}^ – }\);
\({\text{( + )/(anode) 2C}}{{\text{l}}^ – } \to {\text{C}}{{\text{l}}_2} + {\text{2}}{{\text{e}}^ – }\);
Accept e instead of e–.
If electrodes omitted or wrong way round [1 max]
\({\text{(}} – {\text{)/(cathode) N}}{{\text{a}}^ + } + {{\text{e}}^ – } \to {\text{Na}}\);
\({\text{( + )/(anode) 2B}}{{\text{r}}^ – } \to {\text{B}}{{\text{r}}_2} + {\text{2}}{{\text{e}}^ – }\);
Accept e instead of e–.
If electrodes omitted or wrong way round [1 max].
Examiners report
This question was poorly answered. In part (a), the definition of standard electrode potential was poorly stated, with the standard hydrogen electrode rarely mentioned.
Many candidates had difficulty determining the value of the standard electrode potential for the cobalt half-cell.
Few gave \({\text{C}}{{\text{o}}^{2 + }}\) as the oxidizing agent.
If a penalty had already been incurred in Question 4, no further penalty was applied; otherwise the use of the equilibrium arrow in this question was penalized once only.
[N/A]
In part (b), most candidates correctly determined the oxidation states, although they were frequently written incorrectly as 2+ or 3+.
In part (b), most candidates correctly determined the oxidation states, although they were frequently written incorrectly as 2+ or 3+.
In part (b), most candidates correctly determined the oxidation states, although they were frequently written incorrectly as 2+ or 3+.
In part (c) many candidates drew a voltaic cell instead of an electrolytic cell.
[N/A]
[N/A]
[N/A]
Half-equations were frequently the wrong way round, and electrodes were not identified. Candidates who included states of matter in their equations frequently wrote the wrong state and were penalized.
Half-equations were frequently the wrong way round, and electrodes were not identified. Candidates who included states of matter in their equations frequently wrote the wrong state and were penalized.
Question
Magnesium has three stable isotopes, \(^{{\text{24}}}{\text{Mg}}\), \(^{{\text{25}}}{\text{Mg}}\) and \(^{{\text{26}}}{\text{Mg}}\). The relative abundance of each isotope is 78.99%, 10.00% and 11.01%, respectively, and can be determined using a mass spectrometer.
Calculate, showing your working, the relative atomic mass, \({A_{\text{r}}}\), of magnesium, giving your answer to two decimal places.
Answer/Explanation
Markscheme
\(({A_{\text{r}}} = ){\text{ }}0.7899 \times 24 + 0.1000 \times 25 + 0.1101 \times 26\);
24.32;
Award [2] for correct final answer.
Award [1 max] for 24.31 with correct working.
Award [0] for 24.31 (Data Booklet value) if working is incorrect or no working is shown.
Final answer must be to 2 decimal places to score [2].
Examiners report
This question generally scored well. The processes involved in a mass spectrometer were well understood; except for the importance of forming a cation. The calculation of \({A_{\text{r}}}\) and the requirement to give an answer to two decimal places was performed very well.
