Question
The aircraft for a particular flight has 72 seats. The airline’s records show that historically
for this flight only 90% of the people who purchase a ticket arrive to board the flight.
They assume this trend will continue and decide to sell extra tickets and hope that no
more than 72 passengers will arrive.
The number of passengers that arrive to board this flight is assumed to follow a binomial
distribution with a probability of 0.9.
(a) The airline sells 74 tickets for this flight. Find the probability that more than 72
passengers arrive to board the flight. [3]
(b) (i) Write down the expected number of passengers who will arrive to board the
flight if 72 tickets are sold. [2]
(ii) Find the maximum number of tickets that could be sold if the expected number
of passengers who arrive to board the flight must be less than or equal to 72. [2]
Each passenger pays $150 for a ticket. If too many passengers arrive, then the airline will
give $300 in compensation to each passenger that cannot board.
(c) Find, to the nearest integer, the expected increase or decrease in the money made
by the airline if they decide to sell 74 tickets rather than 72. [8]
Answer/Explanation
Ans
5. (a) (let T be the number of passengers who arrive)
(P = ( T > 72) =) P = ( T ≥ 73 ) OR 1 – P ( T ≤ 72) (A1)
T ~ B (74, 0.9) OR n = 74 (M1)
= 0.00379 (0.00379124…) A1
Note: Using the distribution B(74, 0.1) , to work with the 10% that do not arrive
for the flight, here and throughout this question, is a valid approach.
[3 marks]
(b) (i) 72 × 0.9 (M1)
64.8 A1
(ii) n × 0.9 = 72 (M1)
80 A1
[4 marks]
(c) METHOD 1
EITHER
when selling 74 tickets
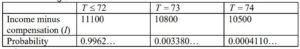
top row A1A1
bottom row A1A1
Note: Award A1A1 for each row correct. Award A1 for one correct
entry and A1 for the remaining entries correct.
E(I) = 11100 × 0.9962… + 10800 × 0.00338… + 10500 × 0.000411 ≈ 11099 (M1)A1
OR
income is 74 × 150 = 11100 (A1)
expected compensation is
0.003380…× 300 + 0.0004110… × 600 ( = 1.26070…) (M1)A1A1
expected income when selling 74 tickets is 11100 − 1.26070… (M1)
=11098.73.. (= $11099) A1
THEN
income for 72 tickets = 72 × 150 = 10800 (A1)
so expected gain ≈11099 − 10800 = $299 A1
Question 5 continued
METHOD 2
for 74 tickets sold, let C be the compensation paid out
P (T = 73) = 0.00338014 …, P= (T=74 ) = 0.000411098 A1A1
E(C) = 0.003380… × 300 + 0.0004110… × 600 (= 1.26070) (M1)A1A1
extra expected revenue = 300 –1.01404… – 0.246658… (300 −1.26070…)
(A1)(M1)
Note: Award A1 for the 300 and M1 for the subtraction.
= $299 (to the nearest dollar) A1
METHOD 3
let D be the change in income when selling 74 tickets

(A1)(A1)
Note: Award A1 for one error, however award A1A1 if there is no explicit mention that
T = 73 would result in D = 0 and the other two are correct.
P (T ≤ 73) 0.9962…, P (T = 74) = 0.000411098 A1A1
E(D) = 300 × 0.9962…+ 0 × 0.003380… − 300 × 0.0004110 (M1)A1A1
= $299 A1
[8 marks]
[Total 15 marks]
Question
A biased coin is weighted such that the probability of obtaining a head is \(\frac{4}{7}\). The coin is tossed 6 times and X denotes the number of heads observed. Find the value of the ratio \(\frac{{{\text{P}}(X = 3)}}{{{\text{P}}(X = 2)}}\).
Answer/Explanation
Markscheme
recognition of \(X \sim {\text{B}}\left( {6,\frac{4}{7}} \right)\) (M1)
\({\text{P}}(X = 3) = \left( {\begin{array}{*{20}{c}}
6 \\
3
\end{array}} \right){\left( {\frac{4}{7}} \right)^3}{\left( {\frac{3}{7}} \right)^3}\left( { = 20 \times \frac{{{4^3} \times {3^3}}}{{{7^6}}}} \right)\) A1
\({\text{P}}(X = 2) = \left( {\begin{array}{*{20}{c}}
6 \\
3
\end{array}} \right){\left( {\frac{4}{7}} \right)^2}{\left( {\frac{3}{7}} \right)^4}\left( { = 15 \times \frac{{{4^2} \times 34}}{{{7^6}}}} \right)\) A1
\(\frac{{{\text{P}}(X = 3)}}{{{\text{P}}(X = 2)}} = \frac{{80}}{{45}}\left( { = \frac{{16}}{9}} \right)\) A1
[4 marks]
Examiners report
Many correct answers were seen to this and the majority of candidates recognised the need to use a Binomial distribution. A number of candidates, although finding the correct expressions for \({\text{P}}(X = 3)\) and \({\text{P}}(X = 4)\), were unable to perform the required simplification.
Question
On Saturday, Alfred and Beatrice play 6 different games against each other. In each game, one of the two wins. The probability that Alfred wins any one of these games is \(\frac{2}{3}\).
Show that the probability that Alfred wins exactly 4 of the games is \(\frac{{80}}{{243}}\).
(i) Explain why the total number of possible outcomes for the results of the 6 games is 64.
(ii) By expanding \({(1 + x)^6}\) and choosing a suitable value for x, prove
\[64 = \left( {\begin{array}{*{20}{c}}
6 \\
0
\end{array}} \right) + \left( {\begin{array}{*{20}{c}}
6 \\
1
\end{array}} \right) + \left( {\begin{array}{*{20}{c}}
6 \\
2
\end{array}} \right) + \left( {\begin{array}{*{20}{c}}
6 \\
3
\end{array}} \right) + \left( {\begin{array}{*{20}{c}}
6 \\
4
\end{array}} \right) + \left( {\begin{array}{*{20}{c}}
6 \\
5
\end{array}} \right) + \left( {\begin{array}{*{20}{c}}
6 \\
6
\end{array}} \right)\]
(iii) State the meaning of this equality in the context of the 6 games played.
The following day Alfred and Beatrice play the 6 games again. Assume that the probability that Alfred wins any one of these games is still \(\frac{2}{3}\).
(i) Find an expression for the probability Alfred wins 4 games on the first day and 2 on the second day. Give your answer in the form \({\left( {\begin{array}{*{20}{c}}
6 \\
r
\end{array}} \right)^2}{\left( {\frac{2}{3}} \right)^s}{\left( {\frac{1}{3}} \right)^t}\) where the values of r, s and t are to be found.
(ii) Using your answer to (c) (i) and 6 similar expressions write down the probability that Alfred wins a total of 6 games over the two days as the sum of 7 probabilities.
(iii) Hence prove that \(\left( {\begin{array}{*{20}{c}}
{12} \\
6
\end{array}} \right) = {\left( {\begin{array}{*{20}{c}}
6 \\
0
\end{array}} \right)^2} + {\left( {\begin{array}{*{20}{c}}
6 \\
1
\end{array}} \right)^2} + {\left( {\begin{array}{*{20}{c}}
6 \\
2
\end{array}} \right)^2} + {\left( {\begin{array}{*{20}{c}}
6 \\
3
\end{array}} \right)^2} + {\left( {\begin{array}{*{20}{c}}
6 \\
4
\end{array}} \right)^2} + {\left( {\begin{array}{*{20}{c}}
6 \\
5
\end{array}} \right)^2} + {\left( {\begin{array}{*{20}{c}}
6 \\
6
\end{array}} \right)^2}\).
Alfred and Beatrice play n games. Let A denote the number of games Alfred wins. The expected value of A can be written as \({\text{E}}(A) = \sum\limits_{r = 0}^n {r\left( {\begin{array}{*{20}{c}}
n \\
r
\end{array}} \right)} \frac{{{a^r}}}{{{b^n}}}\).
(i) Find the values of a and b.
(ii) By differentiating the expansion of \({(1 + x)^n}\), prove that the expected number of games Alfred wins is \(\frac{{2n}}{3}\).
Answer/Explanation
Markscheme
\(B\left( {6,\frac{2}{3}} \right)\) (M1)
\(p(4) = \left( {\begin{array}{*{20}{c}}
6 \\
4
\end{array}} \right){\left( {\frac{2}{3}} \right)^4}{\left( {\frac{1}{3}} \right)^2}\) A1
\(\left( {\begin{array}{*{20}{c}}
6 \\
4
\end{array}} \right) = 15\) A1
\( = 15 \times \frac{{{2^4}}}{{{3^6}}} = \frac{{80}}{{243}}\) AG
[3 marks]
(i) 2 outcomes for each of the 6 games or \({2^6} = 64\) R1
(ii) \({(1 + x)^6} = \left( {\begin{array}{*{20}{c}}
6 \\
0
\end{array}} \right) + \left( {\begin{array}{*{20}{c}}
6 \\
1
\end{array}} \right)x + \left( {\begin{array}{*{20}{c}}
6 \\
2
\end{array}} \right){x^2} + \left( {\begin{array}{*{20}{c}}
6 \\
3
\end{array}} \right){x^3} + \left( {\begin{array}{*{20}{c}}
6 \\
4
\end{array}} \right){x^4} + \left( {\begin{array}{*{20}{c}}
6 \\
5
\end{array}} \right){x^5} + \left( {\begin{array}{*{20}{c}}
6 \\
6
\end{array}} \right){x^6}\) A1
Note: Accept \(^n{C_r}\) notation or \(1 + 6x + 15{x^2} + 20{x^3} + 15{x^4} + 6{x^5} + {x^6}\)
setting x = 1 in both sides of the expression R1
Note: Do not award R1 if the right hand side is not in the correct form.
\(64 = \left( {\begin{array}{*{20}{c}}
6 \\
0
\end{array}} \right) + \left( {\begin{array}{*{20}{c}}
6 \\
1
\end{array}} \right) + \left( {\begin{array}{*{20}{c}}
6 \\
2
\end{array}} \right) + \left( {\begin{array}{*{20}{c}}
6 \\
3
\end{array}} \right) + \left( {\begin{array}{*{20}{c}}
6 \\
4
\end{array}} \right) + \left( {\begin{array}{*{20}{c}}
6 \\
5
\end{array}} \right) + \left( {\begin{array}{*{20}{c}}
6 \\
6
\end{array}} \right)\) AG
(iii) the total number of outcomes = number of ways Alfred can win no games, plus the number of ways he can win one game etc. R1
[4 marks]
(i) Let \({\text{P}}(x,{\text{ }}y)\) be the probability that Alfred wins x games on the first day and y on the second.
\({\text{P(4, 2)}} = \left( {\begin{array}{*{20}{c}}
6 \\
4
\end{array}} \right) \times {\left( {\frac{2}{3}} \right)^4} \times {\left( {\frac{1}{3}} \right)^2} \times \left( {\begin{array}{*{20}{c}}
6 \\
2
\end{array}} \right) \times {\left( {\frac{2}{3}} \right)^2} \times {\left( {\frac{1}{3}} \right)^4}\) M1A1
\({\left( {\begin{array}{*{20}{c}}
6 \\
2
\end{array}} \right)^2}{\left( {\frac{2}{3}} \right)^6}{\left( {\frac{1}{3}} \right)^6}\) or \({\left( {\begin{array}{*{20}{c}}
6 \\
4
\end{array}} \right)^2}{\left( {\frac{2}{3}} \right)^6}{\left( {\frac{1}{3}} \right)^6}\) A1
r = 2 or 4, s = t = 6
(ii) P(Total = 6) =
P(0, 6) + P(1, 5) + P(2, 4) + P(3, 3) + P(4, 2) + P(5, 1) + P(6, 0) (M1)
\( = {\left( {\begin{array}{*{20}{c}}
6 \\
0
\end{array}} \right)^2}{\left( {\frac{2}{3}} \right)^6}{\left( {\frac{1}{3}} \right)^6} + {\left( {\begin{array}{*{20}{c}}
6 \\
1
\end{array}} \right)^2}{\left( {\frac{2}{3}} \right)^6}{\left( {\frac{1}{3}} \right)^6} + … + {\left( {\begin{array}{*{20}{c}}
6 \\
6
\end{array}} \right)^2}{\left( {\frac{2}{3}} \right)^6}{\left( {\frac{1}{3}} \right)^6}\) A2
\( = \frac{{{2^6}}}{{{3^{12}}}}\left( {{{\left( {\begin{array}{*{20}{c}}
6 \\
0
\end{array}} \right)}^2} + {{\left( {\begin{array}{*{20}{c}}
6 \\
1
\end{array}} \right)}^2} + {{\left( {\begin{array}{*{20}{c}}
6 \\
2
\end{array}} \right)}^2} + {{\left( {\begin{array}{*{20}{c}}
6 \\
3
\end{array}} \right)}^2} + {{\left( {\begin{array}{*{20}{c}}
6 \\
4
\end{array}} \right)}^2} + {{\left( {\begin{array}{*{20}{c}}
6 \\
5
\end{array}} \right)}^2} + {{\left( {\begin{array}{*{20}{c}}
6 \\
6
\end{array}} \right)}^2}} \right)\)
Note: Accept any valid sum of 7 probabilities.
(iii) use of \(\left( {\begin{array}{*{20}{c}}
6 \\
i
\end{array}} \right) = \left( {\begin{array}{*{20}{l}}
6 \\
{6 – i}
\end{array}} \right)\) (M1)
(can be used either here or in (c)(ii))
P(wins 6 out of 12) \( = \left( {\begin{array}{*{20}{c}}
{12} \\
6
\end{array}} \right) \times {\left( {\frac{2}{3}} \right)^6} \times {\left( {\frac{1}{3}} \right)^6} = \frac{{{2^6}}}{{{3^{12}}}}\left( {\begin{array}{*{20}{c}}
{12} \\
6
\end{array}} \right)\) A1
\( = \frac{{{2^6}}}{{{3^{12}}}}\left( {{{\left( {\begin{array}{*{20}{c}}
6 \\
0
\end{array}} \right)}^2} + {{\left( {\begin{array}{*{20}{c}}
6 \\
1
\end{array}} \right)}^2} + {{\left( {\begin{array}{*{20}{c}}
6 \\
2
\end{array}} \right)}^2} + {{\left( {\begin{array}{*{20}{c}}
6 \\
3
\end{array}} \right)}^2} + {{\left( {\begin{array}{*{20}{c}}
6 \\
4
\end{array}} \right)}^2} + {{\left( {\begin{array}{*{20}{c}}
6 \\
5
\end{array}} \right)}^2} + {{\left( {\begin{array}{*{20}{c}}
6 \\
6
\end{array}} \right)}^2}} \right) = \frac{{{2^6}}}{{{3^{12}}}}\left( {\begin{array}{*{20}{c}}
{12} \\
6
\end{array}} \right)\) A1
therefore \({\left( {\begin{array}{*{20}{c}}
6 \\
0
\end{array}} \right)^2} + {\left( {\begin{array}{*{20}{c}}
6 \\
1
\end{array}} \right)^2} + {\left( {\begin{array}{*{20}{c}}
6 \\
2
\end{array}} \right)^2} + {\left( {\begin{array}{*{20}{c}}
6 \\
3
\end{array}} \right)^2} + {\left( {\begin{array}{*{20}{c}}
6 \\
4
\end{array}} \right)^2} + {\left( {\begin{array}{*{20}{c}}
6 \\
5
\end{array}} \right)^2} + {\left( {\begin{array}{*{20}{c}}
6 \\
6
\end{array}} \right)^2} = \left( {\begin{array}{*{20}{c}}
{12} \\
6
\end{array}} \right)\) AG
[9 marks]
(i) \({\text{E}}(A) = \sum\limits_{r = 0}^n {r\left( {\begin{array}{*{20}{c}}
n \\
r
\end{array}} \right)} {\left( {\frac{2}{3}} \right)^r}{\left( {\frac{1}{3}} \right)^{n – r}} = \sum\limits_{r = 0}^n {r\left( {\begin{array}{*{20}{c}}
n \\
r
\end{array}} \right)} \frac{{{2^r}}}{{{3^n}}}\)
(a = 2, b = 3) M1A1
Note: M0A0 for a = 2, b = 3 without any method.
(ii) \(n{(1 + x)^{n – 1}} = \sum\limits_{r = 1}^n {\left( {\begin{array}{*{20}{c}}
n \\
r
\end{array}} \right)} r{x^{r – 1}}\) A1A1
(sigma notation not necessary)
(if sigma notation used also allow lower limit to be r = 0)
let x = 2 M1
\(n{3^{n – 1}} = \sum\limits_{r = 1}^n {\left( {\begin{array}{*{20}{c}}
n \\
r
\end{array}} \right)} r{2^{r – 1}}\)
multiply by 2 and divide by \({3^n}\) (M1)
\(\frac{{2n}}{3} = \sum\limits_{r = 1}^n {\left( {\begin{array}{*{20}{c}}
n \\
r
\end{array}} \right)} r\frac{{{2^r}}}{{{3^n}}}\left( { = \sum\limits_{r = 0}^n {\left( {\begin{array}{*{20}{c}}
n \\
r
\end{array}} \right)} \frac{{{2^r}}}{{{3^n}}}} \right)\) AG
[6 marks]
Examiners report
This question linked the binomial distribution with binomial expansion and coefficients and was generally well done.
(a) Candidates need to be aware how to work out binomial coefficients without a calculator
This question linked the binomial distribution with binomial expansion and coefficients and was generally well done.
(b) (ii) A surprising number of candidates chose to work out the values of all the binomial coefficients (or use Pascal’s triangle) to make a total of 64 rather than simply putting 1 into the left hand side of the expression.
This question linked the binomial distribution with binomial expansion and coefficients and was generally well done.
This question linked the binomial distribution with binomial expansion and coefficients and was generally well done.
(d) This was poorly done. Candidates were not able to manipulate expressions given using sigma notation.
Question
A biased coin is tossed five times. The probability of obtaining a head in any one throw is \(p\).
Let \(X\) be the number of heads obtained.
Find, in terms of \(p\), an expression for \({\text{P}}(X = 4)\).
(i) Determine the value of \(p\) for which \({\text{P}}(X = 4)\) is a maximum.
(ii) For this value of \(p\), determine the expected number of heads.
Answer/Explanation
Markscheme
\(X \sim {\text{B}}(5,{\text{ }}p)\) (M1)
\({\text{P}}(X = 4) = \left( {\begin{array}{*{20}{c}} 5 \\ 4 \end{array}} \right){p^4}(1 – p)\) (or equivalent) A1
[2 marks]
(i) \(\frac{{\text{d}}}{{{\text{d}}p}}(5{p^4} – 5{p^5}) = 20{p^3} – 25{p^4}\) M1A1
\(5{p^3}(4 – 5p) = 0 \Rightarrow p = \frac{4}{5}\) M1A1
Note: Do not award the final A1 if \(p = 0\) is included in the answer.
(ii) \({\text{E}}(X) = np = 5\left( {\frac{4}{5}} \right)\) (M1)
\( = 4\) A1
[6 marks]
Examiners report
This question was generally very well done and posed few problems except for the weakest candidates.
This question was generally very well done and posed few problems except for the weakest candidates.
Question
[Maximum mark: 4]
The probability of obtaining heads on a biased coin is 0.18. The coin is tossed seven times.
(a) Find the probability of obtaining exactly two heads. [2]
(b) Find the probability of obtaining at least two heads. [2]
Answer/Explanation
Answer:
B(n, p) with n = 7 and p = 0.18
(a) P(X = 2) = 0.252
(b) P(X ≥ 2) = 0.368
Question
[Maximum mark: 5]
A factory makes switches. The probability that a switch is defective is 0.04.
The factory tests a random sample of 100 switches.
(a) Find the mean number of defective switches in the sample. [1]
(b) Find the probability that there are exactly six defective switches in the sample. [2]
(c) Find the probability that there is at least one defective switch in the sample. [2]
Answer/Explanation
Answer:
B(n, p) with n = 100 and p = 0.04
(a) mean = np = 100 x 0.04 = 4
(b) P(X = 6) = 0.105
(c) P(X ≥ 1) = 0.983
