Question
A function is defined as \(f(x) = k\sqrt x \), with \(k > 0\) and \(x \geqslant 0\) .
(a) Sketch the graph of \(y = f(x)\) .
(b) Show that f is a one-to-one function.
(c) Find the inverse function, \({f^{ – 1}}(x)\) and state its domain.
(d) If the graphs of \(y = f(x)\) and \(y = {f^{ – 1}}(x)\) intersect at the point (4, 4) find the value of k .
(e) Consider the graphs of \(y = f(x)\) and \(y = {f^{ – 1}}(x)\) using the value of k found in part (d).
(i) Find the area enclosed by the two graphs.
(ii) The line x = c cuts the graphs of \(y = f(x)\) and \(y = {f^{ – 1}}(x)\) at the points P and Q respectively. Given that the tangent to \(y = f(x)\) at point P is parallel to the tangent to \(y = {f^{ – 1}}(x)\) at point Q find the value of c .
Answer/Explanation
Markscheme
(a)
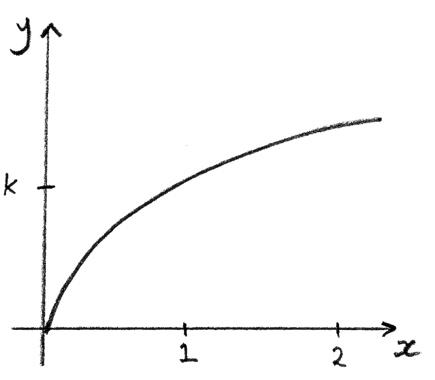 A1
A1
Note: Award A1 for correct concavity, passing through (0, 0) and increasing.
Scales need not be there.
[1 mark]
(b) a statement involving the application of the Horizontal Line Test or equivalent A1
[1 mark]
(c) \(y = k\sqrt x \)
for either \(x = k\sqrt y \) or \(x = \frac{{{y^2}}}{{{k^2}}}\) A1
\({f^{ – 1}}(x) = \frac{{{x^2}}}{{{k^2}}}\) A1
\({\text{dom}}\left( {{f^{ – 1}}(x)} \right) = \left[ {0,\infty } \right[\) A1
[3 marks]
(d) \(\frac{{{x^2}}}{{{k^2}}} = k\sqrt x \,\,\,\,\,\)or equivalent method M1
\(k = \sqrt x \)
\(k = 2\) A1
[2 marks]
(e) (i) \(A = \int_a^b {({y_1} – {y_2}){\text{d}}x} \) (M1)
\(A = \int_0^4 {\left( {2{x^{\frac{1}{2}}} – \frac{1}{4}{x^2}} \right){\text{d}}x} \) A1
\( = \left[ {\frac{4}{3}{x^{\frac{3}{2}}} – \frac{1}{{12}}{x^3}} \right]_0^4\) A1
\( = \frac{{16}}{3}\) A1
(ii) attempt to find either \(f'(x)\) or \(({f^{ – 1}})'(x)\) M1
\(f'(x) = \frac{1}{{\sqrt x }},{\text{ }}\left( {({f^{ – 1}})'(x) = \frac{x}{2}} \right)\) A1A1
\(\frac{1}{{\sqrt c }} = \frac{c}{2}\) M1
\(c = {2^{\frac{2}{3}}}\) A1
[9 marks]
Total [16 marks]
Question
The function \(f\) is defined by \(f\left( x \right) = \frac{{ax + b}}{{cx + d}}\), for \(x \in \mathbb{R},\,\,x \ne – \frac{d}{c}\).
The function \(g\) is defined by \(g\left( x \right) = \frac{{2x – 3}}{{x – 2}},\,\,x \in \mathbb{R},\,\,x \ne 2\)
Find the inverse function \({f^{ – 1}}\), stating its domain.[5]
Express \(g\left( x \right)\) in the form \(A + \frac{B}{{x – 2}}\) where A, B are constants.[2]
Sketch the graph of \(y = g\left( x \right)\). State the equations of any asymptotes and the coordinates of any intercepts with the axes.[3]
The function \(h\) is defined by \(h\left( x \right) = \sqrt x \), for \(x\) ≥ 0.
State the domain and range of \(h \circ g\).[4]
Answer/Explanation
Markscheme
attempt to make \(x\) the subject of \(y = \frac{{ax + b}}{{cx + d}}\) M1
\(y\left( {cx + d} \right) = ax + b\) A1
\(x = \frac{{dy – b}}{{a – cy}}\) A1
\({f^{ – 1}}\left( x \right) = \frac{{dx – b}}{{a – cx}}\) A1
Note: Do not allow \(y = \) in place of \({f^{ – 1}}\left( x \right)\).
\(x \ne \frac{a}{c},\,\,\,\left( {x \in \mathbb{R}} \right)\) A1
Note: The final A mark is independent.
[5 marks]
\(g\left( x \right) = 2 + \frac{1}{{x – 2}}\) A1A1
[2 marks]
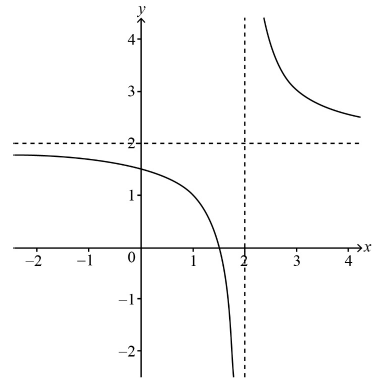
hyperbola shape, with single curves in second and fourth quadrants and third quadrant blank, including vertical asymptote \(x = 2\) A1
horizontal asymptote \(y = 2\) A1
intercepts \(\left( {\frac{3}{2},\,0} \right),\,\left( {0,\,\frac{3}{2}} \right)\) A1
[3 marks]
the domain of \(h \circ g\) is \(x \leqslant \frac{3}{2},\,\,x > 2\) A1A1
the range of \(h \circ g\) is \(y \geqslant 0,\,\,y \ne \sqrt 2 \) A1A1
[4 marks]