Question
The ten numbers \({x_1},{\text{ }}{x_2},{\text{ }} \ldots ,{\text{ }}{x_{10}}\) have a mean of 10 and a standard deviation of 3.
Find the value of \(\sum\limits_{i = 1}^{10} {{{({x_i} – 12)}^2}} \).
▶️Answer/Explanation
Markscheme
EITHER
let \({y_i} = {x_i} – 12\)
\(\bar x = 10 \Rightarrow \bar y = – 2\) M1A1
\({\sigma _x} = {\sigma _y} = 3\) A1
\(\frac{{\sum\limits_{i = 1}^{10} {y_i^2} }}{{10}} – {{\bar y}^2} = 9\) M1A1
\(\sum\limits_{i = 1}^{10} {y_i^2} = 10(9 + 4) = 130\) A1
OR
\(\sum\limits_{i = 1}^{10} {{{({x_i} – 12)}^2} = \sum\limits_{i = 1}^{10} {x_i^2 – 24\sum\limits_{i = 1}^{10} {{x_i} + 144\sum\limits_{i = 1}^{10} 1 } } } \) M1A1
\(\bar x = 10 \Rightarrow \sum\limits_{i = 1}^{10} {{x_i} = 100} \) A1
\({\sigma _x} = 3,{\text{ }}\frac{{\sum\limits_{i = 1}^{10} {x_i^2} }}{{10}} – {{\bar x}^2} = 9\) (M1)
\( \Rightarrow \sum\limits_{i = 1}^{10} {x_i^2} = 10(9 + 100)\) A1
\(\sum\limits_{i = 1}^{10} {{{({x_i} – 12)}^2} = 1090 – 2400 + 1440 = 130} \) A1
[6 marks]
Examiners report
Very few candidates answered this question well, but among those a variety of nice approaches were seen. Most candidates though revealed an inability to deal with sigma expressions, especially \(\sum\limits_{i = 1}^{i = 10} {144} \). Some tried to use expectation algebra but could not then relate those results to sigma expressions (often the factor 10 was forgotten). In a few cases candidates attempted to show the result using particular examples.
Question
On Saturday, Alfred and Beatrice play 6 different games against each other. In each game, one of the two wins. The probability that Alfred wins any one of these games is \(\frac{2}{3}\).
Show that the probability that Alfred wins exactly 4 of the games is \(\frac{{80}}{{243}}\).
(i) Explain why the total number of possible outcomes for the results of the 6 games is 64.
(ii) By expanding \({(1 + x)^6}\) and choosing a suitable value for x, prove
\[64 = \left( {\begin{array}{*{20}{c}}
6 \\
0
\end{array}} \right) + \left( {\begin{array}{*{20}{c}}
6 \\
1
\end{array}} \right) + \left( {\begin{array}{*{20}{c}}
6 \\
2
\end{array}} \right) + \left( {\begin{array}{*{20}{c}}
6 \\
3
\end{array}} \right) + \left( {\begin{array}{*{20}{c}}
6 \\
4
\end{array}} \right) + \left( {\begin{array}{*{20}{c}}
6 \\
5
\end{array}} \right) + \left( {\begin{array}{*{20}{c}}
6 \\
6
\end{array}} \right)\]
(iii) State the meaning of this equality in the context of the 6 games played.
The following day Alfred and Beatrice play the 6 games again. Assume that the probability that Alfred wins any one of these games is still \(\frac{2}{3}\).
(i) Find an expression for the probability Alfred wins 4 games on the first day and 2 on the second day. Give your answer in the form \({\left( {\begin{array}{*{20}{c}}
6 \\
r
\end{array}} \right)^2}{\left( {\frac{2}{3}} \right)^s}{\left( {\frac{1}{3}} \right)^t}\) where the values of r, s and t are to be found.
(ii) Using your answer to (c) (i) and 6 similar expressions write down the probability that Alfred wins a total of 6 games over the two days as the sum of 7 probabilities.
(iii) Hence prove that \(\left( {\begin{array}{*{20}{c}}
{12} \\
6
\end{array}} \right) = {\left( {\begin{array}{*{20}{c}}
6 \\
0
\end{array}} \right)^2} + {\left( {\begin{array}{*{20}{c}}
6 \\
1
\end{array}} \right)^2} + {\left( {\begin{array}{*{20}{c}}
6 \\
2
\end{array}} \right)^2} + {\left( {\begin{array}{*{20}{c}}
6 \\
3
\end{array}} \right)^2} + {\left( {\begin{array}{*{20}{c}}
6 \\
4
\end{array}} \right)^2} + {\left( {\begin{array}{*{20}{c}}
6 \\
5
\end{array}} \right)^2} + {\left( {\begin{array}{*{20}{c}}
6 \\
6
\end{array}} \right)^2}\).
Alfred and Beatrice play n games. Let A denote the number of games Alfred wins. The expected value of A can be written as \({\text{E}}(A) = \sum\limits_{r = 0}^n {r\left( {\begin{array}{*{20}{c}}
n \\
r
\end{array}} \right)} \frac{{{a^r}}}{{{b^n}}}\).
(i) Find the values of a and b.
(ii) By differentiating the expansion of \({(1 + x)^n}\), prove that the expected number of games Alfred wins is \(\frac{{2n}}{3}\).
▶️Answer/Explanation
Markscheme
\(B\left( {6,\frac{2}{3}} \right)\) (M1)
\(p(4) = \left( {\begin{array}{*{20}{c}}
6 \\
4
\end{array}} \right){\left( {\frac{2}{3}} \right)^4}{\left( {\frac{1}{3}} \right)^2}\) A1
\(\left( {\begin{array}{*{20}{c}}
6 \\
4
\end{array}} \right) = 15\) A1
\( = 15 \times \frac{{{2^4}}}{{{3^6}}} = \frac{{80}}{{243}}\) AG
[3 marks]
(i) 2 outcomes for each of the 6 games or \({2^6} = 64\) R1
(ii) \({(1 + x)^6} = \left( {\begin{array}{*{20}{c}}
6 \\
0
\end{array}} \right) + \left( {\begin{array}{*{20}{c}}
6 \\
1
\end{array}} \right)x + \left( {\begin{array}{*{20}{c}}
6 \\
2
\end{array}} \right){x^2} + \left( {\begin{array}{*{20}{c}}
6 \\
3
\end{array}} \right){x^3} + \left( {\begin{array}{*{20}{c}}
6 \\
4
\end{array}} \right){x^4} + \left( {\begin{array}{*{20}{c}}
6 \\
5
\end{array}} \right){x^5} + \left( {\begin{array}{*{20}{c}}
6 \\
6
\end{array}} \right){x^6}\) A1
Note: Accept \(^n{C_r}\) notation or \(1 + 6x + 15{x^2} + 20{x^3} + 15{x^4} + 6{x^5} + {x^6}\)
setting x = 1 in both sides of the expression R1
Note: Do not award R1 if the right hand side is not in the correct form.
\(64 = \left( {\begin{array}{*{20}{c}}
6 \\
0
\end{array}} \right) + \left( {\begin{array}{*{20}{c}}
6 \\
1
\end{array}} \right) + \left( {\begin{array}{*{20}{c}}
6 \\
2
\end{array}} \right) + \left( {\begin{array}{*{20}{c}}
6 \\
3
\end{array}} \right) + \left( {\begin{array}{*{20}{c}}
6 \\
4
\end{array}} \right) + \left( {\begin{array}{*{20}{c}}
6 \\
5
\end{array}} \right) + \left( {\begin{array}{*{20}{c}}
6 \\
6
\end{array}} \right)\) AG
(iii) the total number of outcomes = number of ways Alfred can win no games, plus the number of ways he can win one game etc. R1
[4 marks]
(i) Let \({\text{P}}(x,{\text{ }}y)\) be the probability that Alfred wins x games on the first day and y on the second.
\({\text{P(4, 2)}} = \left( {\begin{array}{*{20}{c}}
6 \\
4
\end{array}} \right) \times {\left( {\frac{2}{3}} \right)^4} \times {\left( {\frac{1}{3}} \right)^2} \times \left( {\begin{array}{*{20}{c}}
6 \\
2
\end{array}} \right) \times {\left( {\frac{2}{3}} \right)^2} \times {\left( {\frac{1}{3}} \right)^4}\) M1A1
\({\left( {\begin{array}{*{20}{c}}
6 \\
2
\end{array}} \right)^2}{\left( {\frac{2}{3}} \right)^6}{\left( {\frac{1}{3}} \right)^6}\) or \({\left( {\begin{array}{*{20}{c}}
6 \\
4
\end{array}} \right)^2}{\left( {\frac{2}{3}} \right)^6}{\left( {\frac{1}{3}} \right)^6}\) A1
r = 2 or 4, s = t = 6
(ii) P(Total = 6) =
P(0, 6) + P(1, 5) + P(2, 4) + P(3, 3) + P(4, 2) + P(5, 1) + P(6, 0) (M1)
\( = {\left( {\begin{array}{*{20}{c}}
6 \\
0
\end{array}} \right)^2}{\left( {\frac{2}{3}} \right)^6}{\left( {\frac{1}{3}} \right)^6} + {\left( {\begin{array}{*{20}{c}}
6 \\
1
\end{array}} \right)^2}{\left( {\frac{2}{3}} \right)^6}{\left( {\frac{1}{3}} \right)^6} + … + {\left( {\begin{array}{*{20}{c}}
6 \\
6
\end{array}} \right)^2}{\left( {\frac{2}{3}} \right)^6}{\left( {\frac{1}{3}} \right)^6}\) A2
\( = \frac{{{2^6}}}{{{3^{12}}}}\left( {{{\left( {\begin{array}{*{20}{c}}
6 \\
0
\end{array}} \right)}^2} + {{\left( {\begin{array}{*{20}{c}}
6 \\
1
\end{array}} \right)}^2} + {{\left( {\begin{array}{*{20}{c}}
6 \\
2
\end{array}} \right)}^2} + {{\left( {\begin{array}{*{20}{c}}
6 \\
3
\end{array}} \right)}^2} + {{\left( {\begin{array}{*{20}{c}}
6 \\
4
\end{array}} \right)}^2} + {{\left( {\begin{array}{*{20}{c}}
6 \\
5
\end{array}} \right)}^2} + {{\left( {\begin{array}{*{20}{c}}
6 \\
6
\end{array}} \right)}^2}} \right)\)
Note: Accept any valid sum of 7 probabilities.
(iii) use of \(\left( {\begin{array}{*{20}{c}}
6 \\
i
\end{array}} \right) = \left( {\begin{array}{*{20}{l}}
6 \\
{6 – i}
\end{array}} \right)\) (M1)
(can be used either here or in (c)(ii))
P(wins 6 out of 12) \( = \left( {\begin{array}{*{20}{c}}
{12} \\
6
\end{array}} \right) \times {\left( {\frac{2}{3}} \right)^6} \times {\left( {\frac{1}{3}} \right)^6} = \frac{{{2^6}}}{{{3^{12}}}}\left( {\begin{array}{*{20}{c}}
{12} \\
6
\end{array}} \right)\) A1
\( = \frac{{{2^6}}}{{{3^{12}}}}\left( {{{\left( {\begin{array}{*{20}{c}}
6 \\
0
\end{array}} \right)}^2} + {{\left( {\begin{array}{*{20}{c}}
6 \\
1
\end{array}} \right)}^2} + {{\left( {\begin{array}{*{20}{c}}
6 \\
2
\end{array}} \right)}^2} + {{\left( {\begin{array}{*{20}{c}}
6 \\
3
\end{array}} \right)}^2} + {{\left( {\begin{array}{*{20}{c}}
6 \\
4
\end{array}} \right)}^2} + {{\left( {\begin{array}{*{20}{c}}
6 \\
5
\end{array}} \right)}^2} + {{\left( {\begin{array}{*{20}{c}}
6 \\
6
\end{array}} \right)}^2}} \right) = \frac{{{2^6}}}{{{3^{12}}}}\left( {\begin{array}{*{20}{c}}
{12} \\
6
\end{array}} \right)\) A1
therefore \({\left( {\begin{array}{*{20}{c}}
6 \\
0
\end{array}} \right)^2} + {\left( {\begin{array}{*{20}{c}}
6 \\
1
\end{array}} \right)^2} + {\left( {\begin{array}{*{20}{c}}
6 \\
2
\end{array}} \right)^2} + {\left( {\begin{array}{*{20}{c}}
6 \\
3
\end{array}} \right)^2} + {\left( {\begin{array}{*{20}{c}}
6 \\
4
\end{array}} \right)^2} + {\left( {\begin{array}{*{20}{c}}
6 \\
5
\end{array}} \right)^2} + {\left( {\begin{array}{*{20}{c}}
6 \\
6
\end{array}} \right)^2} = \left( {\begin{array}{*{20}{c}}
{12} \\
6
\end{array}} \right)\) AG
[9 marks]
(i) \({\text{E}}(A) = \sum\limits_{r = 0}^n {r\left( {\begin{array}{*{20}{c}}
n \\
r
\end{array}} \right)} {\left( {\frac{2}{3}} \right)^r}{\left( {\frac{1}{3}} \right)^{n – r}} = \sum\limits_{r = 0}^n {r\left( {\begin{array}{*{20}{c}}
n \\
r
\end{array}} \right)} \frac{{{2^r}}}{{{3^n}}}\)
(a = 2, b = 3) M1A1
Note: M0A0 for a = 2, b = 3 without any method.
(ii) \(n{(1 + x)^{n – 1}} = \sum\limits_{r = 1}^n {\left( {\begin{array}{*{20}{c}}
n \\
r
\end{array}} \right)} r{x^{r – 1}}\) A1A1
(sigma notation not necessary)
(if sigma notation used also allow lower limit to be r = 0)
let x = 2 M1
\(n{3^{n – 1}} = \sum\limits_{r = 1}^n {\left( {\begin{array}{*{20}{c}}
n \\
r
\end{array}} \right)} r{2^{r – 1}}\)
multiply by 2 and divide by \({3^n}\) (M1)
\(\frac{{2n}}{3} = \sum\limits_{r = 1}^n {\left( {\begin{array}{*{20}{c}}
n \\
r
\end{array}} \right)} r\frac{{{2^r}}}{{{3^n}}}\left( { = \sum\limits_{r = 0}^n {\left( {\begin{array}{*{20}{c}}
n \\
r
\end{array}} \right)} \frac{{{2^r}}}{{{3^n}}}} \right)\) AG
[6 marks]
Examiners report
This question linked the binomial distribution with binomial expansion and coefficients and was generally well done.
(a) Candidates need to be aware how to work out binomial coefficients without a calculator
This question linked the binomial distribution with binomial expansion and coefficients and was generally well done.
(b) (ii) A surprising number of candidates chose to work out the values of all the binomial coefficients (or use Pascal’s triangle) to make a total of 64 rather than simply putting 1 into the left hand side of the expression.
This question linked the binomial distribution with binomial expansion and coefficients and was generally well done.
This question linked the binomial distribution with binomial expansion and coefficients and was generally well done.
(d) This was poorly done. Candidates were not able to manipulate expressions given using sigma notation.
Question
A mathematics test is given to a class of 20 students. One student scores 0, but all the other students score 10.
a.Find the mean score for the class.[2]
b.Write down the median score.[1]
c.Write down the number of students who scored
(i) above the mean score;
(ii) below the median score.[2]
▶️Answer/Explanation
Markscheme
\(\bar x = \frac{{1 \times 0 + 19 \times 10}}{{20}} = 9.5\) (M1)A1
[2 marks]
median is \(10\) A1
[1 mark]
(i) \(19\) A1
(ii) \(1\) A1
[2 marks]
Total [5 marks]
Examiners report
Well done.
Well done.
Both parts well done.
Question
A mathematics test is given to a class of 20 students. One student scores 0, but all the other students score 10.
a.Find the mean score for the class.[2]
b.Write down the median score.[1]
c.Write down the number of students who scored
(i) above the mean score;
(ii) below the median score.[2]
▶️Answer/Explanation
Markscheme
\(\bar x = \frac{{1 \times 0 + 19 \times 10}}{{20}} = 9.5\) (M1)A1
[2 marks]
median is \(10\) A1
[1 mark]
(i) \(19\) A1
(ii) \(1\) A1
[2 marks]
Total [5 marks]
Examiners report
Well done.
Well done.
Both parts well done.
Question
At a skiing competition the mean time of the first three skiers is 34.1 seconds. The time for the fourth skier is then recorded and the mean time of the first four skiers is 35.0 seconds. Find the time achieved by the fourth skier.
▶️Answer/Explanation
Markscheme
total time of first 3 skiers \( = 34.1 \times 3 = 102.3\) (M1)A1
total time of first 4 skiers \( = 35.0 \times 4 = 140.0\) A1
time taken by fourth skier \( = 140.0 – 102.3 = 37.7{\text{ (seconds)}}\) A1
[4 marks]
Examiners report
This was done successfully by almost all candidates.
Question
The discrete random variable X has the following probability distribution, where p is a constant.
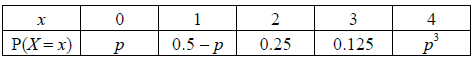
a.Find the value of p.[2]
b.i.Find μ, the expected value of X.[2]
b.ii.Find P(X > μ).[2]
▶️Answer/Explanation
Markscheme
equating sum of probabilities to 1 (p + 0.5 − p + 0.25 + 0.125 + p3 = 1) M1
p3 = 0.125 = \(\frac{1}{8}\)
p= 0.5 A1
[2 marks]
μ = 0 × 0.5 + 1 × 0 + 2 × 0.25 + 3 × 0.125 + 4 × 0.125 M1
= 1.375 \(\left( { = \frac{{11}}{8}} \right)\) A1
[2 marks]
P(X > μ) = P(X = 2) + P(X = 3) + P(X = 4) (M1)
= 0.5 A1
Note: Do not award follow through A marks in (b)(i) from an incorrect value of p.
Note: Award M marks in both (b)(i) and (b)(ii) provided no negative probabilities, and provided a numerical value for μ has been found.
[2 marks]
Examiners report
[N/A]
[N/A]
[N/A]
Question
The discrete random variable X has the following probability distribution, where p is a constant.
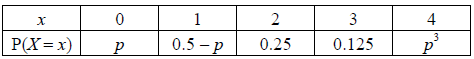
a.Find the value of p.[2]
b.i.Find μ, the expected value of X.[2]
b.ii.Find P(X > μ).[2]
▶️Answer/Explanation
Markscheme
equating sum of probabilities to 1 (p + 0.5 − p + 0.25 + 0.125 + p3 = 1) M1
p3 = 0.125 = \(\frac{1}{8}\)
p= 0.5 A1
[2 marks]
μ = 0 × 0.5 + 1 × 0 + 2 × 0.25 + 3 × 0.125 + 4 × 0.125 M1
= 1.375 \(\left( { = \frac{{11}}{8}} \right)\) A1
[2 marks]
P(X > μ) = P(X = 2) + P(X = 3) + P(X = 4) (M1)
= 0.5 A1
Note: Do not award follow through A marks in (b)(i) from an incorrect value of p.
Note: Award M marks in both (b)(i) and (b)(ii) provided no negative probabilities, and provided a numerical value for μ has been found.
[2 marks]
Examiners report
[N/A]
[N/A]
[N/A]
Question
The discrete random variable X has the following probability distribution, where p is a constant.
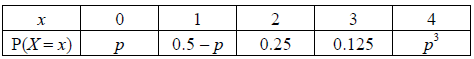
a.Find the value of p.[2]
b.i.Find μ, the expected value of X.[2]
b.ii.Find P(X > μ).[2]
▶️Answer/Explanation
Markscheme
equating sum of probabilities to 1 (p + 0.5 − p + 0.25 + 0.125 + p3 = 1) M1
p3 = 0.125 = \(\frac{1}{8}\)
p= 0.5 A1
[2 marks]
μ = 0 × 0.5 + 1 × 0 + 2 × 0.25 + 3 × 0.125 + 4 × 0.125 M1
= 1.375 \(\left( { = \frac{{11}}{8}} \right)\) A1
[2 marks]
P(X > μ) = P(X = 2) + P(X = 3) + P(X = 4) (M1)
= 0.5 A1
Note: Do not award follow through A marks in (b)(i) from an incorrect value of p.
Note: Award M marks in both (b)(i) and (b)(ii) provided no negative probabilities, and provided a numerical value for μ has been found.
[2 marks]
Examiners report
[N/A]
[N/A]
[N/A]
