Question
(a) Using l’Hopital’s Rule, show that \(\mathop {\lim }\limits_{x \to \infty } x{{\text{e}}^{ – x}} = 0\) .
(b) Determine \(\int_0^a {x{{\text{e}}^{ – x}}{\text{d}}x} \) .
(c) Show that the integral \(\int_0^\infty {x{{\text{e}}^{ – x}}{\text{d}}x} \) is convergent and find its value.
Answer/Explanation
Markscheme
(a) \(\mathop {\lim }\limits_{x \to \infty } \frac{x}{{{{\text{e}}^x}}} = \mathop {\lim }\limits_{x \to \infty } \frac{1}{{{{\text{e}}^x}}}\) M1A1
= 0 AG
[2 marks]
(b) Using integration by parts M1
\(\int_0^a {x{{\text{e}}^{ – x}}{\text{d}}x} = \left[ { – x{{\text{e}}^{ – x}}} \right]_0^a + \int_0^a {{{\text{e}}^{ – x}}{\text{d}}x} \) A1A1
\( = – a{{\text{e}}^{ – a}} – \left[ {{e^{ – x}}} \right]_0^a\) A1
\( = 1 – a{{\text{e}}^{ – a}} – {{\text{e}}^{ – a}}\) A1
[5 marks]
(c) Since \({{\text{e}}^{ – a}}\) and \(a{{\text{e}}^{ – a}}\) are both convergent (to zero), the integral is convergent. R1
Its value is 1. A1
[2 marks]
Total [9 marks]
Examiners report
Most candidates made a reasonable attempt at (a). In (b), however, it was disappointing to note that some candidates were unable to use integration by parts to perform the integration. In (c), while many candidates obtained the correct value of the integral, proof of its convergence was often unconvincing.
Question
Find the value of \(\mathop {\lim }\limits_{x \to 1} \left( {\frac{{\ln x}}{{\sin 2\pi x}}} \right)\).
By using the series expansions for \({{\text{e}}^{{x^2}}}\) and cos x evaluate \(\mathop {\lim }\limits_{x \to 0} \left( {\frac{{1 – {{\text{e}}^{{x^2}}}}}{{1 – \cos x}}} \right).\).
Answer/Explanation
Markscheme
Using l’Hopital’s rule,
\(\mathop {\lim }\limits_{x \to 1} \left( {\frac{{\ln x}}{{\sin 2\pi x}}} \right) = \mathop {\lim }\limits_{x \to 1} \left( {\frac{{\frac{1}{x}}}{{2\pi \cos 2\pi x}}} \right)\) M1A1
\( = \frac{1}{{2\pi }}\) A1
[3 marks]
\(\mathop {\lim }\limits_{x \to 0} \left( {\frac{{1 – {{\text{e}}^{{x^2}}}}}{{1 – \cos x}}} \right) = \mathop {\lim }\limits_{x \to 0} \left( {\frac{{1 – \left( {1 + {x^2} + \frac{{{x^4}}}{{2!}} + \frac{{{x^6}}}{{3!}} + …} \right)}}{{1 – \left( {1 – \frac{{{x^2}}}{{2!}} + \frac{{{x^4}}}{{4!}} – …} \right)}}} \right)\) M1A1A1
Note: Award M1 for evidence of using the two series.
\( = \mathop {\lim }\limits_{x \to 0} \left( {\frac{{\left( { – {x^2} – \frac{{{x^4}}}{{2!}} – \frac{{{x^6}}}{{3!}} – …} \right)}}{{\left( {\frac{{{x^2}}}{{2!}} – \frac{{{x^4}}}{{4!}} + …} \right)}}} \right)\) A1
EITHER
\( = \mathop {\lim }\limits_{x \to 0} \left( {\frac{{\left( { – 1 – \frac{{{x^2}}}{{2!}} – \frac{{{x^4}}}{{3!}} – …} \right)}}{{\left( {\frac{1}{{2!}} – \frac{{{x^2}}}{{4!}} + …} \right)}}} \right)\) M1A1
\( = \frac{{ – 1}}{{\frac{1}{2}}} = – 2\) A1
OR
\( = \mathop {\lim }\limits_{x \to 0} \left( {\frac{{\left( { – 2x – \frac{{4{x^3}}}{{2!}} – \frac{{6{x^5}}}{{3!}} – …} \right)}}{{\left( {\frac{{2x}}{{2!}} – \frac{{4{x^3}}}{{4!}} + …} \right)}}} \right)\) M1A1
\( = \mathop {\lim }\limits_{x \to 0} \left( {\frac{{\left( { – 2 – \frac{{4{x^2}}}{{2!}} – \frac{{6{x^4}}}{{3!}} – …} \right)}}{{\left( {1 – \frac{{4{x^2}}}{{4!}} + …} \right)}}} \right)\)
\( = \frac{{ – 2}}{1} = – 2\) A1
[7 marks]
Examiners report
Part (a) was well done but too often the instruction to use series in part (b) was ignored. When this hint was observed correct solutions followed.
Part (a) was well done but too often the instruction to use series in part (b) was ignored. When this hint was observed correct solutions followed.
Question
Find the value of \(\mathop {\lim }\limits_{x \to 1} \left( {\frac{{\ln x}}{{\sin 2\pi x}}} \right)\).
By using the series expansions for \({{\text{e}}^{{x^2}}}\) and cos x evaluate \(\mathop {\lim }\limits_{x \to 0} \left( {\frac{{1 – {{\text{e}}^{{x^2}}}}}{{1 – \cos x}}} \right).\).
Answer/Explanation
Markscheme
Using l’Hopital’s rule,
\(\mathop {\lim }\limits_{x \to 1} \left( {\frac{{\ln x}}{{\sin 2\pi x}}} \right) = \mathop {\lim }\limits_{x \to 1} \left( {\frac{{\frac{1}{x}}}{{2\pi \cos 2\pi x}}} \right)\) M1A1
\( = \frac{1}{{2\pi }}\) A1
[3 marks]
\(\mathop {\lim }\limits_{x \to 0} \left( {\frac{{1 – {{\text{e}}^{{x^2}}}}}{{1 – \cos x}}} \right) = \mathop {\lim }\limits_{x \to 0} \left( {\frac{{1 – \left( {1 + {x^2} + \frac{{{x^4}}}{{2!}} + \frac{{{x^6}}}{{3!}} + …} \right)}}{{1 – \left( {1 – \frac{{{x^2}}}{{2!}} + \frac{{{x^4}}}{{4!}} – …} \right)}}} \right)\) M1A1A1
Note: Award M1 for evidence of using the two series.
\( = \mathop {\lim }\limits_{x \to 0} \left( {\frac{{\left( { – {x^2} – \frac{{{x^4}}}{{2!}} – \frac{{{x^6}}}{{3!}} – …} \right)}}{{\left( {\frac{{{x^2}}}{{2!}} – \frac{{{x^4}}}{{4!}} + …} \right)}}} \right)\) A1
EITHER
\( = \mathop {\lim }\limits_{x \to 0} \left( {\frac{{\left( { – 1 – \frac{{{x^2}}}{{2!}} – \frac{{{x^4}}}{{3!}} – …} \right)}}{{\left( {\frac{1}{{2!}} – \frac{{{x^2}}}{{4!}} + …} \right)}}} \right)\) M1A1
\( = \frac{{ – 1}}{{\frac{1}{2}}} = – 2\) A1
OR
\( = \mathop {\lim }\limits_{x \to 0} \left( {\frac{{\left( { – 2x – \frac{{4{x^3}}}{{2!}} – \frac{{6{x^5}}}{{3!}} – …} \right)}}{{\left( {\frac{{2x}}{{2!}} – \frac{{4{x^3}}}{{4!}} + …} \right)}}} \right)\) M1A1
\( = \mathop {\lim }\limits_{x \to 0} \left( {\frac{{\left( { – 2 – \frac{{4{x^2}}}{{2!}} – \frac{{6{x^4}}}{{3!}} – …} \right)}}{{\left( {1 – \frac{{4{x^2}}}{{4!}} + …} \right)}}} \right)\)
\( = \frac{{ – 2}}{1} = – 2\) A1
[7 marks]
Examiners report
Part (a) was well done but too often the instruction to use series in part (b) was ignored. When this hint was observed correct solutions followed.
Part (a) was well done but too often the instruction to use series in part (b) was ignored. When this hint was observed correct solutions followed.
Question
(a) Show that the solution of the differential equation
\[\frac{{{\text{d}}y}}{{{\text{d}}x}} = \cos x{\cos ^2}y{\text{,}}\]
given that \(y = \frac{\pi }{4}{\text{ when }}x = \pi {\text{, is }}y = \arctan (1 + \sin x){\text{.}}\)
(b) Determine the value of the constant a for which the following limit exists
\[\mathop {\lim }\limits_{x \to \frac{\pi }{2}} \frac{{\arctan (1 + \sin x) – a}}{{{{\left( {x – \frac{\pi }{2}} \right)}^2}}}\]
and evaluate that limit.
Answer/Explanation
Markscheme
(a) this separable equation has general solution
\(\int {{{\sec }^2}y{\text{d}}y = \int {\cos x{\text{d}}x} } \) (M1)(A1)
\(\tan y = \sin x + c\) A1
the condition gives
\(\tan \frac{\pi }{4} = \sin \pi + c \Rightarrow c = 1\) M1
the solution is \(\tan y = 1 + \sin x\) A1
\(y = \arctan (1 + \sin x)\) AG
[5 marks]
(b) the limit cannot exist unless \(a = \arctan \left( {1 + \sin \frac{\pi }{2}} \right) = \arctan 2\) R1A1
in that case the limit can be evaluated using l’Hopital’s rule (twice) limit is
\(\mathop {\lim }\limits_{x \to \frac{\pi }{2}} \frac{{{{\left( {\arctan (1 + \sin x)} \right)}^\prime }}}{{2\left( {x – \frac{\pi }{2}} \right)}} = \mathop {\lim }\limits_{x \to \frac{\pi }{2}} \frac{{y’}}{{2\left( {x – \frac{\pi }{2}} \right)}}\) M1A1
where y is the solution of the differential equation
the numerator has zero limit (from the factor \(\cos x\) in the differential equation) R1
so required limit is
\(\mathop {\lim }\limits_{x \to \frac{\pi }{2}} \frac{{y”}}{2}\) M1A1
finally,
\(y” = – \sin x{\cos ^2}y – 2\cos x\cos y\sin y \times y'(x)\) M1A1
since \(\cos y\left( {\frac{\pi }{2}} \right) = \frac{1}{{\sqrt 5 }}\) A1
\(y” = – \frac{1}{5}{\text{ at }}x = \frac{\pi }{2}\) A1
the required limit is \( – \frac{1}{{10}}\) A1
[12 marks]
Total [17 marks]
Examiners report
Many candidates successfully obtained the displayed solution of the differential equation in part(a). Few complete solutions to part(b) were seen which used the result in part(a). The problem can, however, be solved by direct differentiation although this is algebraically more complicated. Some successful solutions using this method were seen.
Question
Find \(\mathop {\lim }\limits_{x \to 0} \frac{{\tan x}}{{x + {x^2}}}\) ;
Find \(\mathop {\lim }\limits_{x \to 1} \frac{{1 – {x^2} + 2{x^2}\ln x}}{{1 – \sin \frac{{\pi x}}{2}}}\) .
Answer/Explanation
Markscheme
\(\mathop {\lim }\limits_{x \to 0} \frac{{\tan x}}{{x + {x^2}}} = \mathop {\lim }\limits_{x \to 0} \frac{{{{\sec }^2}x}}{{1 + 2x}}\) M1A1A1
\(\mathop {\lim }\limits_{x \to 0} \frac{{\tan x}}{{x + {x^2}}} = \frac{1}{1} = 1\) A1
[4 marks]
\(\mathop {\lim }\limits_{x \to 1} \frac{{1 – {x^2} + 2{x^2}\ln x}}{{1 – \sin \frac{{\pi x}}{2}}} = \mathop {\lim }\limits_{x \to 1} \frac{{ – 2x + 2x + 4x\ln x}}{{ – \frac{\pi }{2}\cos \frac{{\pi x}}{2}}}\) M1A1A1
\( = \mathop {\lim }\limits_{x \to 1} \frac{{4 + 4\ln x}}{{\frac{{{\pi ^2}}}{4}\sin \frac{{\pi x}}{2}}}\) M1A1A1
\(\mathop {\lim }\limits_{x \to 1} \frac{{1 – {x^2} + 2{x^2}\ln x}}{{1 – \sin \frac{{\pi x}}{2}}} = \frac{4}{{\frac{{{\pi ^2}}}{4}}} = \frac{{16}}{{{\pi ^2}}}\) A1
[7 marks]
Examiners report
This question was accessible to the vast majority of candidates, who recognised that L’Hopital’s rule was required. A few of the weaker candidates did not realise that it needed to be applied twice in part (b). Many fully correct solutions were seen.
This question was accessible to the vast majority of candidates, who recognised that L’Hopital’s rule was required. A few of the weaker candidates did not realise that it needed to be applied twice in part (b). Many fully correct solutions were seen.
Question
The function f is defined by \(f(x) = {{\text{e}}^{({{\text{e}}^x} – 1)}}\) .
(a) Assuming the Maclaurin series for \({{\text{e}}^x}\) , show that the Maclaurin series for \(f(x)\)
is \(1 + x + {x^2} + \frac{5}{6}{x^3} + \ldots {\text{ .}}\)
(b) Hence or otherwise find the value of \(\mathop {\lim }\limits_{x \to 0} \frac{{f(x) – 1}}{{f'(x) – 1}}\) .
Answer/Explanation
Markscheme
(a) \({{\text{e}}^x} – 1 = x + \frac{{{x^2}}}{2} + \frac{{{x^2}}}{6} + \ldots \) A1
\({{\text{e}}^{{{\text{e}}^x} – 1}} = 1 + \left( {x + \frac{{{x^2}}}{2} + \frac{{{x^3}}}{6}} \right) + \frac{{{{\left( {x + \frac{{{x^2}}}{2} + \frac{{{x^3}}}{6}} \right)}^2}}}{2} + \frac{{{{\left( {x + \frac{{{x^2}}}{2} + \frac{{{x^3}}}{6}} \right)}^3}}}{6} + \ldots \) M1A1
\( = 1 + x + \frac{{{x^2}}}{2} + \frac{{{x^3}}}{6} + \frac{{{x^2}}}{2} + \frac{{{x^3}}}{2} + \frac{{{x^3}}}{6} + \ldots \) M1A1
\( = 1 + x + {x^2} + \frac{5}{6}{x^3} + \ldots \) AG
[5 marks]
(b) EITHER
\(f'(x) = 1 + 2x + \frac{{5{x^2}}}{2} + \ldots \) A1
\(\frac{{f(x) – 1}}{{f'(x) – 1}} = \frac{{x + {x^2} + 5{x^3}/6 + \ldots }}{{2x + 5{x^2}/2 + \ldots }}\) M1A1
\( = \frac{{1 + x + \ldots }}{{2 + 5x/2 + \ldots }}\) A1
\( \to \frac{1}{2}{\text{ as }}x \to 0\) A1
[5 marks]
OR
using l’Hopital’s rule, M1
\(\mathop {\lim }\limits_{x \to 0} \frac{{{{\text{e}}^{({{\text{e}}^x} – 1)}} – 1}}{{{{\text{e}}^{({{\text{e}}^x} – 1)}} – 1′ – 1}} = \mathop {\lim }\limits_{x \to 0} \frac{{{{\text{e}}^{({{\text{e}}^x} – 1)}} – 1}}{{{{\text{e}}^{({{\text{e}}^x} + x – 1)}} – 1}}\) M1A1
\( = \mathop {\lim }\limits_{x \to 0} \frac{{{{\text{e}}^{({{\text{e}}^x} + x – 1)}}}}{{{{\text{e}}^{({{\text{e}}^x} + x – 1)}} \times ({{\text{e}}^x} + 1)}}\) A1
\( = \frac{1}{2}\) A1
[5 marks]
Total [10 marks]
Examiners report
Many candidates obtained the required series by finding the values of successive derivatives at x = 0 , failing to realise that the intention was to start with the exponential series and replace x by the series for \({{\text{e}}^x} – 1\). Candidates who did this were given partial credit for using this method. Part (b) was reasonably well answered using a variety of methods.
Question
(a) Using the Maclaurin series for \({(1 + x)^n}\), write down and simplify the Maclaurin series approximation for \({(1 – {x^2})^{ – \frac{1}{2}}}\) as far as the term in \({x^4}\)
(b) Use your result to show that a series approximation for arccos x is
\[\arccos x \approx \frac{\pi }{2} – x – \frac{1}{6}{x^3} – \frac{3}{{40}}{x^5}.\]
(c) Evaluate \(\mathop {\lim }\limits_{x \to 0} \frac{{\frac{\pi }{2} – \arccos ({x^2}) – {x^2}}}{{{x^6}}}\).
(d) Use the series approximation for \(\arccos x\) to find an approximate value for
\[\int_0^{0.2} {\arccos \left( {\sqrt x } \right){\text{d}}x} ,\]
giving your answer to 5 decimal places. Does your answer give the actual value of the integral to 5 decimal places?
Answer/Explanation
Markscheme
(a) using or obtaining \({(1 + x)^n} = 1 + nx + \frac{{n(n – 1)}}{2}{x^2} + \ldots \) (M1)
\({(1 – {n^2})^{ – \frac{1}{2}}} = 1 + ( – {x^2}) \times \left( { – \frac{1}{2}} \right) + \frac{{{{( – {x^2})}^2}}}{2} \times \left( { – \frac{1}{2}} \right) \times \left( { – \frac{3}{2}} \right) + \ldots \) (A1)
\( = 1 + \frac{1}{2}{x^2} + \frac{3}{8}{x^4} + \ldots \) A1
[3 marks]
(b) integrating, and changing sign
\(\arccos x = – x – \frac{1}{6}{x^3} – \frac{3}{{40}}{x^5} + C + \ldots \) M1A1
put x = 0,
\(\frac{\pi }{2} = C\) M1
\(\left( {\arccos x \approx \frac{\pi }{2} – x – \frac{1}{6}{x^3} – \frac{3}{{40}}{x^5}} \right)\) AG
[3 marks]
(c) EITHER
using \(\arccos {x^2} \approx \frac{\pi }{2} – {x^2} – \frac{1}{6}{x^6} – \frac{3}{{40}}{x^{10}}\) M1A1
\(\mathop {\lim }\limits_{x \to 0} \frac{{\frac{\pi }{2} – \arccos {x^2} – {x^2}}}{{{x^6}}} = \mathop {\lim }\limits_{x \to 0} \frac{{\frac{{{x^6}}}{6} + {\text{higher powers}}}}{{{x^6}}}\) M1A1
\( = \frac{1}{6}\) A1
OR
using l’Hôpital’s Rule M1
\({\text{limit}} = \mathop {\lim }\limits_{x \to 0} \frac{{\frac{1}{{\sqrt {1 – {x^4}} }} \times 2x – 2x}}{{6{x^5}}}\) M1
\( = \mathop {\lim }\limits_{x \to 0} \frac{{\frac{1}{{\sqrt {1 – {x^4}} }} – 1}}{{3{x^4}}}\) A1
\( = \mathop {\lim }\limits_{x \to 0} \frac{{ – \frac{1}{2} \times \frac{1}{{{{(1 – {x^4})}^{3/2}}}} \times – 4{x^3}}}{{12{x^3}}}\) M1
\( = \frac{1}{6}\) A1
[5 marks]
(d) \(\int_0^{0.2} {\arccos \sqrt x {\text{d}}x \approx \int_0^{0.2} {\left( {\frac{\pi }{2} – {x^{\frac{1}{2}}} – \frac{1}{6}{x^{\frac{3}{2}}} – \frac{3}{{40}}{x^{\frac{5}{2}}}} \right){\text{d}}x} } \) M1
\( = \left[ {\frac{\pi }{2}x – \frac{2}{3}{x^{\frac{3}{2}}} – \frac{1}{{15}}{x^{\frac{5}{2}}} – \frac{3}{{140}}{x^{\frac{7}{2}}}} \right]_0^{0.2}\) (A1)
\( = \frac{\pi }{2} \times 0.2 – \frac{2}{3} \times {0.2^{\frac{3}{2}}} – \frac{1}{{15}} \times {0.2^{\frac{5}{2}}} – \frac{3}{{140}} \times {0.2^{\frac{7}{2}}}\) (A1)
= 0.25326 (to 5 decimal places) A1
Note: Accept integration of the series approximation using a GDC.
using a GDC, the actual value is 0.25325 A1
so the approximation is not correct to 5 decimal places R1
[6 marks]
Total [17 marks]
Examiners report
Many candidates ignored the instruction in the question to use the series for \({(1 + x)^n}\) to deduce the series for \({(1 – {x^2})^{ – 1/2}}\) and attempted instead to obtain it by successive differentiation. It was decided at the standardisation meeting to award full credit for this method although in the event the algebra proved to be too difficult for many. Many candidates used l’Hopital’s Rule in (c) – this was much more difficult algebraically than using the series and it usually ended unsuccessfully. Candidates should realise that if a question on evaluating an indeterminate limit follows the determination of a Maclaurin series then it is likely that the series will be helpful in evaluating the limit. Part (d) caused problems for many candidates with algebraic errors being common. Many candidates failed to realise that the best way to find the exact value of the integral was to use the calculator.
Question
Find \(\mathop {\lim }\limits_{x \to 0} \left( {\frac{{1 – \cos {x^6}}}{{{x^{12}}}}} \right)\).
Answer/Explanation
Markscheme
METHOD 1
\(f(0) = \frac{0}{0}\), hence using l’Hôpital’s Rule, (M1)
\(g(x) = 1 – \cos ({x^6}),{\text{ }}h(x) = {x^{12}};{\text{ }}\frac{{g'(x)}}{{h'(x)}} = \frac{{6{x^5}\sin ({x^6})}}{{12{x^{11}}}} = \frac{{\sin ({x^6})}}{{2{x^6}}}\) A1A1
EITHER
\(\frac{{g'(0)}}{{h'(0)}} = \frac{0}{0}\), using l’Hôpital’s Rule again, (M1)
\(\frac{{g”(x)}}{{h”(x)}} = \frac{{6{x^5}\cos ({x^6})}}{{12{x^5}}} = \frac{{\cos ({x^6})}}{2}\) A1A1
\(\frac{{g”(0)}}{{h”(0)}} = \frac{1}{2}\), hence the limit is \(\frac{1}{2}\) A1
OR
So \(\mathop {\lim }\limits_{x \to 0} \frac{{1 – \cos {x^6}}}{{{x^{12}}}} = \mathop {\lim }\limits_{x \to 0} \frac{{\sin {x^6}}}{{2{x^6}}}\) A1
\( = \frac{1}{2}\mathop {\lim }\limits_{x \to 0} \frac{{\sin {x^6}}}{{2{x^6}}}\) A1
\( = \frac{1}{2}{\text{ since }}\mathop {\lim }\limits_{x \to 0} \frac{{\sin {x^6}}}{{2{x^6}}} = 1\) A1 (R1)
METHOD 2
substituting \({{x^6}}\) for x in the expansion \(\cos x = 1 – \frac{{{x^2}}}{2} + \frac{{{x^4}}}{{24}} \ldots \) (M1)
\(\frac{{1 – \cos {x^6}}}{{{x^{12}}}} = \frac{{1 – \left( {1 – \frac{{{x^{12}}}}{2} + \frac{{{x^{24}}}}{{24}}} \right) \ldots }}{{{x^{12}}}}\) M1A1
\( = \frac{1}{2} – \frac{{{x^{12}}}}{{24}} + …\) A1A1
\(\mathop {\lim }\limits_{x \to 0} \frac{{1 – \cos {x^6}}}{{{x^{12}}}} = \frac{1}{2}\) M1A1
Note: Accept solutions using Maclaurin expansions.
[7 marks]
Examiners report
Surprisingly, some weaker candidates were more successful in answering this question than stronger candidates. If candidates failed to simplify the expression after the first application of L’Hôpital’s rule, they generally were not successful in correctly differentiating the expression a \({2^{{\text{nd}}}}\) time, hence could not achieve the final three A marks.
Question
Find the first three terms of the Maclaurin series for \(\ln (1 + {{\text{e}}^x})\) .
Hence, or otherwise, determine the value of \(\mathop {\lim }\limits_{x \to 0} \frac{{2\ln (1 + {{\text{e}}^x}) – x – \ln 4}}{{{x^2}}}\) .
Answer/Explanation
Markscheme
METHOD 1
\(f(x) = \ln (1 + {{\text{e}}^x});{\text{ }}f(0) = \ln 2\) A1
\(f'(x) = \frac{{{{\text{e}}^x}}}{{1 + {{\text{e}}^x}}};{\text{ }}f'(0) = \frac{1}{2}\) A1
Note: Award A0 for \(f'(x) = \frac{1}{{1 + {{\text{e}}^x}}};{\text{ }}f'(0) = \frac{1}{2}\)
\(f”(x) = \frac{{{{\text{e}}^x}(1 + {{\text{e}}^x}) – {{\text{e}}^{2x}}}}{{{{(1 + {{\text{e}}^x})}^2}}};{\text{ }}f”(0) = \frac{1}{4}\) M1A1
Note: Award M0A0 for \(f”(x){\text{ if }}f'(x) = \frac{1}{{1 + {{\text{e}}^x}}}\) is used
\(\ln (1 + {{\text{e}}^x}) = \ln 2 + \frac{1}{2}x + \frac{1}{8}{x^2} + …\) M1A1
[6 marks]
METHOD 2
\(\ln (1 + {{\text{e}}^x}) = \ln (1 + 1 + x + \frac{1}{2}{x^2} + …)\) M1A1
\( = \ln 2 + \ln (1 + \frac{1}{2}x + \frac{1}{4}{x^2} + …)\) A1
\( = \ln 2 + \left( {\frac{1}{2}x + \frac{1}{4}{x^2} + …} \right) – \frac{1}{2}{\left( {\frac{1}{2}x + \frac{1}{4}{x^2} + …} \right)^2} + …\) A1
\( = \ln 2 + \frac{1}{2}x + \frac{1}{4}{x^2} – \frac{1}{8}{x^2} + …\) A1
\( = \ln 2 + \frac{1}{2}x + \frac{1}{8}{x^2} + …\) A1
[6 marks]
METHOD 1
\(\mathop {\lim }\limits_{x \to 0} \frac{{2\ln (1 + {{\text{e}}^x}) – x – \ln 4}}{{{x^2}}} = \mathop {\lim }\limits_{x \to 0} \frac{{2\ln 2 + x + \frac{{{x^2}}}{4} + {x^3}{\text{ terms & above}} – x – \ln 4}}{{{x^2}}}\) M1A1
\( = \mathop {\lim }\limits_{x \to 0} \left( {\frac{1}{4} + {\text{powers of }}x} \right) = \frac{1}{4}\) M1A1
Note: Accept + … as evidence of recognition of cubic and higher powers.
Note: Award M1AOM1A0 for a solution which omits the cubic and higher powers.
[4 marks]
METHOD 2
using l’Hôpital’s Rule
\(\mathop {\lim }\limits_{x \to 0} \frac{{2\ln (1 + {{\text{e}}^x}) – x – \ln 4}}{{{x^2}}} = \mathop {\lim }\limits_{x \to 0} \frac{{2{{\text{e}}^x} \div (1 + {{\text{e}}^x}) – 1}}{{2x}}\) M1A1
\( = \mathop {\lim }\limits_{x \to 0} \frac{{2{{\text{e}}^x} \div {{(1 + {{\text{e}}^x})}^2}}}{2} = \frac{1}{4}\) M1A1
[4 marks]
Examiners report
In (a), candidates who found the series by successive differentiation were generally successful, the most common error being to state that the derivative of \(\ln (1 + {{\text{e}}^x})\) is \({(1 + {{\text{e}}^x})^{ – 1}}\). Some candidates assumed the series for \(\ln (1 + x)\) and \({{\text{e}}^x}\) attempted to combine them. This was accepted as an alternative solution but candidates using this method were often unable to obtain the required series.
In (b), candidates were equally split between using the series or using l’Hopital’s rule to find the limit. Both methods were fairly successful, but a number of candidates forgot that if a series was used, there had to be a recognition that it was not a finite series.
Question
Use L’Hôpital’s Rule to find \(\mathop {\lim }\limits_{x \to 0} \frac{{{{\text{e}}^x} – 1 – x\cos x}}{{{{\sin }^2}x}}\) .
Answer/Explanation
Markscheme
apply l’Hôpital’s Rule to a \(0/0\) type limit
\(\mathop {\lim }\limits_{x \to 0} \frac{{{{\text{e}}^x} – 1 – x\cos x}}{{{{\sin }^2}x}} = \mathop {\lim }\limits_{x \to 0} \frac{{{{\text{e}}^x} – \cos x + x\sin x}}{{2\sin x\cos x}}\) M1A1
noting this is also a \(0/0\) type limit, apply l’Hôpital’s Rule again (M1)
obtain \(\mathop {\lim }\limits_{x \to 0} \frac{{{{\text{e}}^x} + \sin x + x\cos x + \sin x}}{{2\cos 2x}}\) A1
substitution of x = 0 (M1)
= 0.5 A1
[6 marks]
Examiners report
The vast majority of candidates were familiar with L’Hôpitals rule and were also able to apply the technique twice as required by the problem. The errors that occurred were mostly due to difficulty in applying the differentiation rules correctly or errors in algebra. A small minority of candidates tried to use the quotient rule but it seemed that most candidates had a good understanding of L’Hôpital’s rule and its application to finding a limit.
Question
Consider the infinite spiral of right angle triangles as shown in the following diagram.
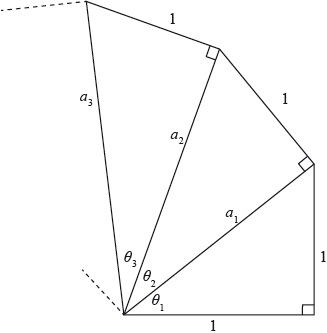
The \(n{\text{th}}\) triangle in the spiral has central angle \({\theta _n}\), hypotenuse of length \({a_n}\) and opposite side of length 1, as shown in the diagram. The first right angle triangle is isosceles with the two equal sides being of length 1.
Consider the series \(\sum\limits_{n = 1}^\infty {{\theta _n}} \).
Using l’Hôpital’s rule, find \(\mathop {\lim }\limits_{x \to \infty } \left( {\frac{{\arcsin \left( {\frac{1}{{\sqrt {(x + 1)} }}} \right)}}{{\frac{1}{{\sqrt x }}}}} \right)\).
(i) Find \({a_1}\) and \({a_2}\) and hence write down an expression for \({a_n}\).
(ii) Show that \({\theta _n} = \arcsin \frac{1}{{\sqrt {(n + 1)} }}\).
Using a suitable test, determine whether this series converges or diverges.
Answer/Explanation
Markscheme
\(\mathop {\lim }\limits_{x \to \infty } \left( {\frac{{\arcsin \left( {\frac{1}{{\sqrt {(x + 1)} }}} \right)}}{{\frac{1}{{\sqrt x }}}}} \right)\) is of the form \(\frac{0}{0}\)
and so will equal the limit of \(\frac{{\frac{{\frac{{ – 1}}{2}{{(x + 1)}^{ – \frac{3}{2}}}}}{{\sqrt {1 – \left( {\frac{1}{{x + 1}}} \right)} }}}}{{\frac{{ – 1}}{2}{x^{ – \frac{3}{2}}}}}\) M1M1A1A1
Note: M1 for attempting differentiation of the top and bottom, M1A1 for derivative of top (only award M1 if chain rule is used), A1 for derivative of bottom.
\( = \mathop {\lim }\limits_{x \to \infty } \frac{{{{\left( {\frac{x}{{(x + 1)}}} \right)}^{\frac{3}{2}}}}}{{\sqrt {\frac{x}{{x + 1}}} }} = \mathop {\lim }\limits_{x \to \infty } \left( {\frac{x}{{x + 1}}} \right)\) M1
Note: Accept any intermediate tidying up of correct derivative for the method mark.
\( = 1\) A1
[6 marks]
(i) \({a_1} = \sqrt 2 ,{\text{ }}{a_2} = \sqrt 3 \) A1
\({a_n} = \sqrt {n + 1} \) A1
(ii) \(\sin {\theta _n} = \frac{1}{{{a_n}}} = \frac{1}{{\sqrt {n + 1} }}\) A1
Note: Allow \({\theta _n} = \arcsin \left( {\frac{1}{{{a_n}}}} \right)\) if \({a_n} = \sqrt {n + 1} \) in b(i).
so \({\theta _n} = \arcsin \frac{1}{{\sqrt {(n + 1)} }}\) AG
[3 marks]
for \(\sum\limits_{n = 1}^\infty {\arcsin \frac{1}{{\sqrt {(n + 1)} }}} \) apply the limit comparison test (since both series of positive terms) M1
with \(\sum\limits_{n = 1}^\infty {\frac{1}{{\sqrt n }}} \) A1
from (a) \(\mathop {\lim }\limits_{n \to \infty } \frac{{\arcsin \frac{1}{{\sqrt {(n + 1)} }}}}{{\frac{1}{{\sqrt n }}}} = 1\), so the two series either both converge or both diverge M1R1
\(\sum\limits_{n = 1}^\infty {\frac{1}{{\sqrt 2 }}} \) diverges (as is a \(p\)-series with \(p = \frac{1}{2}\)) A1
hence \(\sum\limits_{n = 1}^\infty {{\theta _n}} \) diverges A1
[6 marks]
Examiners report
[N/A]
[N/A]
[N/A]
Question
The function f is defined on the domain \(\left] { – \frac{\pi }{2},\frac{\pi }{2}} \right[{\text{ by }}f(x) = \ln (1 + \sin x)\) .
Show that \(f”(x) = – \frac{1}{{(1 + \sin x)}}\) .
(i) Find the Maclaurin series for \(f(x)\) up to and including the term in \({x^4}\) .
(ii) Explain briefly why your result shows that f is neither an even function nor an odd function.
Determine the value of \(\mathop {\lim }\limits_{x \to 0} \frac{{\ln (1 + \sin x) – x}}{{{x^2}}}\).
Answer/Explanation
Markscheme
\(f'(x) = \frac{{\cos x}}{{1 + \sin x}}\) A1
\(f”(x) = \frac{{ – \sin x(1 + \sin x) – \cos x\cos x}}{{{{(1 + \sin x)}^2}}}\) M1A1
\( = \frac{{ – \sin x – ({{\sin }^2}x + {{\cos }^2}x)}}{{{{(1 + \sin x)}^2}}}\) A1
\( = – \frac{1}{{1 + \sin x}}\) AG
[4 marks]
(i) \(f”'(x) = \frac{{\cos x}}{{{{(1 + \sin x)}^2}}}\) A1
\({f^{(4)}}(x) = \frac{{ – \sin x{{(1 + \sin x)}^2} – 2(1 + \sin x){{\cos }^2}x}}{{{{(1 + \sin x)}^4}}}\) M1A1
\(f(0) = 0,{\text{ }}f'(0) = 1,{\text{ }}f”(0) = – 1\) M1
\(f”'(0) = 1,{\text{ }}{f^{(4)}}(0) = – 2\) A1
\(f(x) = x – \frac{{{x^2}}}{2} + \frac{{{x^3}}}{6} – \frac{{{x^4}}}{{12}} + \ldots \) A1
(ii) the series contains even and odd powers of x R1
[7 marks]
\(\mathop {\lim }\limits_{x \to 0} \frac{{\ln (1 + \sin x) – x}}{{{x^2}}} = \mathop {\lim }\limits_{x \to 0} \frac{{x – \frac{{{x^2}}}{2} + \frac{{{x^3}}}{6} + \ldots – x}}{{{x^2}}}\) M1
\( = \mathop {\lim }\limits_{x \to 0} \frac{{\frac{{ – 1}}{2} + \frac{x}{6} + \ldots }}{1}\) (A1)
\( = – \frac{1}{2}\) A1
Note: Use of l’Hopital’s Rule is also acceptable.
[3 marks]
Examiners report
[N/A]
[N/A]
[N/A]

