Question
An electron enters a region of uniform magnetic field at a speed v. The direction of the electron is perpendicular to the magnetic field. The path of the electron inside the magnetic field is circular with radius r.

The speed of the electron is varied to obtain different values of r.
Which graph represents the variation of speed v with r?

▶️Answer/Explanation
Ans:A
When an electron enters a region of a uniform magnetic field with a velocity perpendicular to the magnetic field lines, it experiences a magnetic force that acts as a centripetal force, causing the electron to move in a circular path. This is due to the interaction of the magnetic field with the moving charge of the electron.
The magnetic force (\(F_{\text{mag}}\)) on the electron is given by:
\[F_{\text{mag}} = qvB\]
This magnetic force provides the centripetal force required to keep the electron in a circular path. The centripetal force (\(F_{\text{cent}}\)) for an object moving in a circle of radius \(r\) with speed \(v\) is given by:
\[F_{\text{cent}} = \frac{mv^2}{r}\]
Since the magnetic force is acting as the centripetal force, we can equate the two expressions:
\[qvB = \frac{mv^2}{r}\]
Now, we can solve for the radius (\(r\)) of the circular path:
\[r = \frac{mv}{qB}\]
So, as the speed of the electron (\(v\)) is varied, the radius of the circular path (\(r\)) will change accordingly. The greater the speed, the larger the radius of the circular path, and vice versa. This relationship shows that the radius of the circular path is directly proportional to the speed of the electron, provided that the magnetic field strength and the charge of the electron remain constant.
Question
An electron is accelerated from rest through a potential difference \(V\).
What is the maximum speed of the electron?
A. \(\sqrt{\frac{2 e V}{m_e}}\)
B. \(\frac{e V}{m_e}\)
C. \(\frac{2 e V}{m_\theta}\)
D. \(\sqrt{\frac{2 V}{m_e}}\)
▶️Answer/Explanation
Ans:A
The maximum speed of an electron accelerated through a potential difference \(V\) can be calculated using the following formula:
\[v_{\text{max}} = \sqrt{\frac{2eV}{m_e}}\]
Where:
- \(v_{\text{max}}\) is the maximum speed of the electron.
- \(e\) is the charge of an electron.
- \(V\) is the potential difference.
- \(m_e\) is the mass of an electron.
So, the correct answer is A. \(\sqrt{\frac{2eV}{m_e}}\).
Question
A negatively charged sphere is falling through a magnetic field.
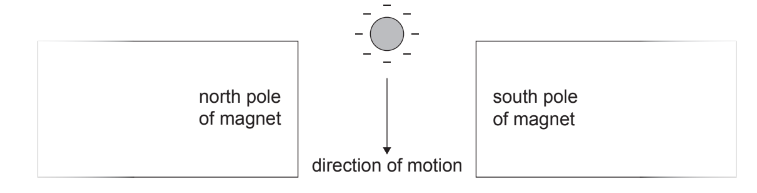
What is the direction of the magnetic force acting on the sphere?
A. To the left of the page
B. To the right of the page
C. Out of the page
D. Into the page
▶️Answer/Explanation
Ans:D
Current I will be opposite to flow of electron and magnetic field will be from north to south pole,

FLEMING’S LEFT HAND RULE
Fleming’s left hand rule states that, when \(u\) keep the thumb, index finger and middle finger of the left hand right angle to each other, if the middle finger shows the direction of current, index finger shows the direction of magnetic field, then the thumb will show the direction of motion. This law explains the working of a DC motor.

From this , magnetic force acting on the sphere is acting Into the page
Question
Two currents of 3 A and 1 A are established in the same direction through two parallel straight wires R and S.

What is correct about the magnetic forces acting on each wire?
A Both wires exert equal magnitude attractive forces on each other.
B Both wires exert equal magnitude repulsive forces on each other.
C Wire R exerts a larger magnitude attractive force on wire S.
D Wire R exerts a larger magnitude repulsive force on wire S.
Answer/Explanation
Answer – A

Question
Magnetic field lines are an example of
A a discovery that helps us understand magnetism.
B a model to aid in visualization.
C a pattern in data from experiments.
D a theory to explain concepts in magnetism.
Answer/Explanation
Ans: B
Field lines can be visualized quite easily in the real world. This is commonly done with iron filings dropped on a surface near something magnetic. Each filing behaves like a tiny magnet with a north and south pole. The filings naturally separate from each other because similar poles repel each other. The result is a pattern that resembles field lines. While the general pattern will always be the same, the exact position and density of lines of filings depends on how the filings happened to fall, their size and magnetic properties.
