Question
This interaction between a proton and a pion violates two or more conservation laws.
$
\mathrm{p}+\pi^{-} \rightarrow \mathrm{K}^{-}+\pi^{+}
$
Quark composition of particles:
$
\pi^{-}=\mathrm{du}, \pi^{+}=\mathrm{u} \overline{\mathrm{d}}, \mathrm{K}^{-}=\mathrm{su}, \mathrm{p}=\mathrm{uud}
$
Which laws are violated by this interaction?
I. Conservation of charge
II. Conservation of strangeness
III. Conservation of baryon number
A. I and II only
B. I and III only
C. II and III only
D. I, II and III
▶️Answer/Explanation
Ans:C
In this interaction, the initial and final states involve different particles, and we need to consider the conservation of various quantum numbers. Let’s evaluate the conservation laws:
I. Conservation of charge:
- Initial state: p (proton) has a charge of +1, and π⁻ (pion) has a charge of -1. So, the total charge of the initial state is 1 – 1 = 0.
- Final state: K⁻ (kaon) has a charge of -1, and π⁺ (pion) has a charge of +1. So, the total charge of the final state is -1 + 1 = 0.
- The conservation of charge is not violated in this interaction.
II. Conservation of strangeness:
- Strangeness is not conserved in this interaction. The initial state has a strangeness of 0 (since p and π⁻ have no strangeness), while the final state has a strangeness of -1 (K⁻ has strangeness -1, and π⁺ has strangeness 0).
III. Conservation of baryon number:
- Baryon number is not conserved in this interaction. The initial state has a baryon number of 1 (since p is a baryon), while the final state has a baryon number of 0 (since there are no baryons in the final state).
So, the correct answer is: C. II and III only
Conservation of charge (I) is not violated, but both the conservation of strangeness (II) and the conservation of baryon number (III) are violated in this interaction.
Question
In nuclear fission, a nucleus of element X absorbs a neutron (n) to give a nucleus of element Y and a nucleus of element Z.
X + n → Y + Z + 2n
What is \(\frac{{{\text{magnitude of the binding energy per nucleon of Y}}}}{{{\text{magnitude of the binding energy per nucleon of X}}}}\) and \(\frac{{{\text{total binding energy of Y and Z}}}}{{{\text{total binding energy of X}}}}\)?
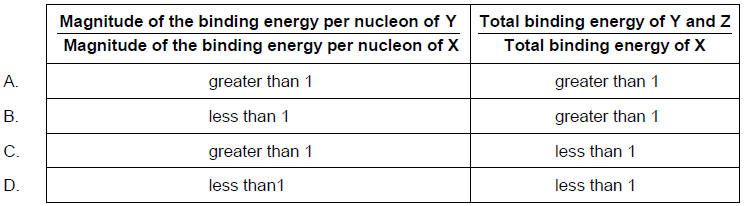
Answer/Explanation
Markscheme
A
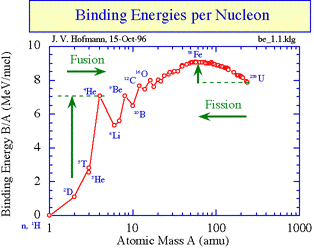
Energy is released when the binding energy per nucleon increases as this results in greater stability Nuclear reactions occur when the reactants have lower binding energy per nucleon than the products. If you look at the graph you can see that this can happen in two ways: if nuclei with very small values of A join together (fusion) they produce nuclei with larger values of A that have greater binding energy per nucleon,or large nuclei can split to form smaller nuclei (fission) that are higher up on the graph line (see arrows). Binding energy per nucleon increases by movement towards peak of graph.
Hence Binding energy of products are higher than reactant in case of Nuclear Fission reaction.
As mass of product combined together is less than mass of reactant , as this mass change is converted into nuclear energy and hence Binding energy of product is more than Binding Energy of reactant. Ans hence Product is more stable than reactant.
Question
What are the principal roles of a moderator and of a control rod in a thermal nuclear reactor?
| Role of moderator | Role of control rod |
A. | increases kinetic energy of neutrons | maintains a constant rate of reaction |
B. | increases kinetic energy of neutrons | absorbs energy transferred in the reactor |
C. | reduces kinetic energy of neutrons | maintains a constant rate of reaction |
D. | reduces kinetic energy of neutrons | absorbs energy transferred in the reactor |
Answer/Explanation
Ans: C
The moderator helps slow down the neutrons produced by fission to sustain the chain reaction. Control rods can then be inserted into the reactor core to reduce the reaction rate or withdrawn to increase it.
A rod, plate, or tube containing a material such as hafnium, boron, etc., used to control the power of a nuclear reactor. By absorbing neutrons, a control rod prevents the neutrons from causing further fissions and maintains a constant rate at which reaction happen.
What is the definition of the unified atomic mass unit?
A. \(\frac{1}{{12}}\) the mass of a neutral atom of carbon-12
B. The mass of a neutral atom of hydrogen-1
C. \(\frac{1}{{12}}\) the mass of a nucleus of carbon-12
D. The mass of a nucleus of hydrogen-1
Answer/Explanation
Markscheme
A
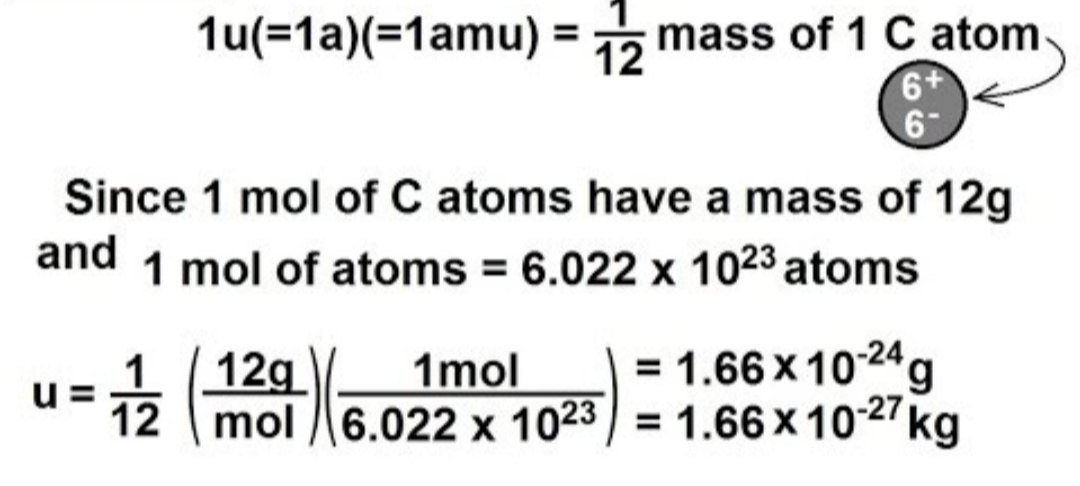
The dalton or unified atomic mass unit is a unit of mass widely used in physics and chemistry. It is defined as 1⁄12 of the mass of an unbound neutral atom of carbon-12 in its nuclear and electronic ground state and at rest. The atomic mass constant, denoted mᵤ, is defined identically, giving \(mᵤ = \frac{m}{12} = 1 Da\)