Question
Some cells, such as intestinal cells, exchange a lot of material with their surroundings. The surface-to-volume ratio of these cells affects the efficiency of material exchange.
The table provides measurements of four different eukaryotic cells.
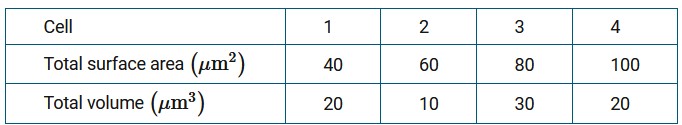
A. Cell 1
B. Cell 2
C. Cell 3
D. Cell 4
▶️Answer/Explanation
Ans: B
The surface area to volume calculation is \(\frac{60}{10}\)=6. Of the four cells, this one has the highest ratio of surface area to volume and is likely to be most effective in the exchange of materials.
Question
Hereditary spherocytosis (HS) is a disorder of red blood cells that causes the cells to be smaller and spherical instead of having the usual flattened, biconcave shape. The average diameter of normal red blood cells is 7.2μm, and the average diameter of red blood cells in a person with HS was found to be 6.7μm. The normal red blood cell has an average surface area of 136μm2 and an average volume of 91μ\(m^3\).
Which of the following provides an accurate calculation of the surface area to volume ratio of an HS red blood cell, as well as a prediction of its effect on the efficient transferring of oxygen compared to a normal red blood cell?
A. The ratio is 0.45, and the cells are more efficient at transferring oxygen.
B. The ratio is 1.12, and the cells are less efficient at transferring oxygen.
C. The ratio is 0.89, and the cells are less efficient at transferring oxygen.
D. The ratio is 141, and the cells are more efficient at transferring oxygen.
▶️Answer/Explanation
Ans: C
The correct calculation of the surface area to volume ratio of the HS cell is 0.89. This ratio is less than the ratio found in a normal red blood cell, 1.49, so the cell would be less efficient at transferring oxygen.
Question
Stomata are pores on the surfaces of the leaves and stems of plants that regulate gas exchange between the plants and the atmosphere.
Researchers found that the stomata density on the leaves of a species of plant change as the concentration of \(CO_2\) in the atmosphere changes. When grown at 350 ppm \(CO_2\) the plant has an average density of 300 stomata per mm2, but when grown at 400 ppm \(CO_2\) the plant has an average density of 250 stomata per \(mm^2\).
Which of the following best describes how the ratio of the density of stomata (stomata per mm2) per \(CO_2\) concentration (ppm \(CO_2\)) changes as the \(CO_2\) concentration increases?
A. The ratio decreases from 0.86 to 0.63, because fewer stomata are needed at higher \(CO_2\) concentrations.
B. The ratio decreases from 1.6 to 1.2, because fewer stomata are needed at higher \(CO_2\) concentrations.
C. The ratio increases from 0.63 to 0.86, because more stomata are needed at higher \(CO_2\) concentrations.
D. The ratio increases from 1.2 to 1.6, because more stomata are needed at higher \(CO_2\) concentrations.
▶️Answer/Explanation
Ans: A
The ratio of 300 stomata per \(mm^2\) to 350 ppm \(CO_2\) is 0.86, and the ratio of 250 stomata per mm2 to 400 ppm \(CO_2\) is 0.63. This reflects that fewer stomata are needed as the concentration of \(CO_2\) increases.
Question
Researchers propose a model to explain variation in phytoplankton cell sizes in a marine environment. They base their model on the idea that smaller cells absorb nutrients more efficiently. The researchers predict that the mean diameter of phytoplankton cells will change by 50 micrometers for every 5-kilometer increase in distance from the shore because of a gradual decrease in nutrient availability. To test their model, the researchers determine that the phytoplankton cells found closest to shore have a mean diameter of 900 micrometers.
Based on the model, what will be the mean diameter of the phytoplankton cells that are found 25 kilometers from shore?
A. 650 micrometers
B. 875 micrometers
C. 925 micrometers
D. 1150 micrometers
▶️Answer/Explanation
Ans: A
The model predicts that phytoplankton cell size will decrease with increasing distance from the shore, resulting in increased efficiency of nutrient absorption. The correct value was arrived at by subtracting 250 from 900.
Question
A spherical bacterial cell has a radius of 3μm. The human egg cell has a radius of 100μm.
Which statement correctly indicates the cell that is able to more efficiently exchange materials with the external environment and provides a correct explanation?
A. The egg cell, because it has the smallest surface-to-volume ratio.
B. The egg cell, because it has the largest surface-to-volume ratio.
C. The bacterial cell, because it has the smallest surface-to-volume ratio.
D. The bacterial cell, because it has the largest surface-to-volume ratio.
▶️Answer/Explanation
Ans: D
The bacterial cell is more efficient in the exchange of materials with the external environment. While the egg cell is much larger than the bacterial cell, the egg cell has a surface-to-volume ratio that is 33 times smaller than the bacterial cell’s surface-to-volume ratio.
