Question:
On a certain workday, the rate, in tons per hour, at which unprocessed gravel arrives at a gravel processing plant is modeled by \(G(t)=90+45 Cos\left ( \frac{t^{2}}{18} \right ),\) where t is measured in hours and 0 ≤ t ≤ 8. At the beginning of the workday (t = 0), the plant has 500 tons of unprocessed gravel. During the hours of operation,0 ≤ t ≤ 8 the plant processes gravel at a constant rate of 100 tons per hour.
(a) Find G’(5). Using correct units, interpret your answer in the context of the problem.
(b) Find the total amount of unprocessed gravel that arrives at the plant during the hours of operation on this workday.
(c) Is the amount of unprocessed gravel at the plant increasing or decreasing at time t = 5 hours? Show the work that leads to your answer.
(d) What is the maximum amount of unprocessed gravel at the plant during the hours of operation on this workday? Justify your answer.
▶️Answer/Explanation
Ans:
(a)
\(G(t)=90+45 Cos\frac{t^{2}}{18}\)
\(G'(t)=-5t sin\left ( \frac{t^{2}}{18} \right )\)
G’(5) = – 24.588 tons/ hr2
This means that the rate at which unprocessed gravel arrives at the processing plant is changing by -24.588 tons per hour per hour, or decreasing by 24.588 tons per hour per hour, at t = 5 hours.
(b)
\(\int_{0}^{8}\left [ 90+45 cos\left ( \frac{t^{2}}{18} \right ) \right ]dt= 825.551 tons\)
(c)
Let V(t) be the amount of unprocessed gravel.
V’(t) is the rate of which the amount of unprocessed gravel is changing.
V’(t) = G(t) – 100
V’(5) = G(5) – 100
\(=90+45 cos \left ( \frac{52}{18} \right )-100\)
V’(5) = – 1.859
Since V’(5) is negative the amount of unprocessed gravel is decreasing at time t = 5 hours
(d)
V’(t) = 0 at t = ?
0 = G(t) – 100
\(100=90+45 cos \left ( \frac{t^{2}}{18} \right )\)
\(10=45 cos \left ( \frac{t^{2}}{18} \right )\)
T = 4.923
\(v(t)-v(0)=\int_{0}^{t}\left ( G(x)-100 \right )dx\)
\(v(t)=\int_{0}^{t}\left ( G(x)-100 \right )dx+v(0)\)
V (0) = 500
V(4.923) = 635.76
V(8) = 525.551
Since v(t) is on a closed interval [0, 8 ], the maximum amount must occur at an endpoint or at a critical value after evaluating the amount of unprocessed gravel at t = 0, t = 4.923, and t = 8, the amount of unprocessed gravel is highest at t = 4.923, with 635.376 tons of unprocessed gravel.
Question
The rate at which raw sewage enters a treatment tank is given by E (t ) gallons per hour for \(0\leq t\leq 4\), hours. Treated sewage is removed from the tank at the constant rate of 645 gallons per hour. The treatment tank is empty at time t =0.
(a) How many gallons of sewage enter the treatment tank during the time interval \(0\leq t\leq 4\) Round your answer to the nearest gallon.
(b) For\( 0\leq t\leq 4,\) at what time t is the amount of sewage in the treatment tank greatest? To the nearest gallon, what is the maximum amount of sewage in the tank? Justify your answers.
(c) For \(0\leq t\leq 4?\) the cost of treating the raw sewage that enters the tank at time t is ( 0.15 -0.02t ) dollars per gallon. To the nearest dollar, what is the total cost of treating all the sewage that enters the tank during the time interval\( 0\leq t\leq 4?\)
▶️Answer/Explanation
(a) \(\int_{0}^{4}E(t) dt=3981 \)gallons
(b) Let S (t ) be the amount of sewage in the treatment tank at time t. Then \(S'(t)=E(t)-645 and S'(t)=0\) when
E ( t) = 645. On the interval \(0\leq t\leq 4\)= 645
when t = 2.309 and t= 3.559.
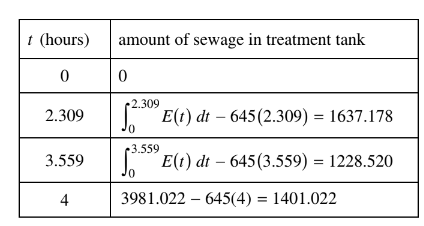
The amount of sewage in the treatment tank is greatest at
t = 2.309 hours. At that time, the amount of sewage in the tank, rounded to the nearest gallon, is 1637 gallons.
(c) Total cost= \(\int_{0}^{4}(0.15-0.02t)E(t)dt=474.320\) The total cost of treating the sewage that enters the tank
during the time interval \(0\leq t\leq 4\) to the nearest dollar, is $474.
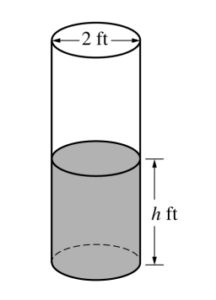
Question
A cylindrical barrel with a diameter of 2 feet contains collected rainwater, as shown in the figure above. The water drains out through a valve (not shown) at the bottom of the barrel. The rate of change of the height h of the water in the barrel with respect to time t is modeled by \(\frac{dh}{dt}=-\frac{1}{10}\sqrt{h},\) where h is measured in feet and
t is measured in seconds. (The volume V of a cylinder with radius r and height h is V = πr2h.)
(a) Find the rate of change of the volume of water in the barrel with respect to time when the height of the water is 4 feet. Indicate units of measure.
(b) When the height of the water is 3 feet, is the rate of change of the height of the water with respect to time increasing or decreasing? Explain your reasoning.
(c) At time t = 0 seconds, the height of the water is 5 feet. Use separation of variables to find an expression for h in terms of t.
▶️Answer/Explanation
Ans:
(a) \(V = \pi r^{2}h=\pi (1)^{2}h=\pi h\)
\(\frac{dV}{dt}|_{h=4}=\pi \frac{dh}{dt}|_{h=4}=\pi \left ( -\frac{1}{10}\sqrt{4} \right )=-\frac{\pi }{5}\) cubic feet per second
(b) \(\frac{d^{2}h}{dt^{2}}=-\frac{1}{20\sqrt{h}}\cdot \frac{dh}{dt}=-\frac{1}{20\sqrt{h}}\cdot \left ( -\frac{1}{10} \sqrt{h}\right )=\frac{1}{200}\)
Because \(\frac{d^{2}h}{dt^{2}}=\frac{1}{200}>0\) for h > 0, the rate of change of the height is increasing when the height of the water is 3 feet.
(c) \(\frac{dh}{\sqrt{h}}=-\frac{1}{10}dt\)
\(\int \frac{dh}{\sqrt{h}}=\int -\frac{1}{10}dt\)
\(2\sqrt{h}=-\frac{1}{10}t+C\)
\(2\sqrt{5}=-\frac{1}{10}\cdot 0+C\Rightarrow C = 2\sqrt{5}\)
\(2\sqrt{h}=-\frac{1}{10}t+2\sqrt{5}\)
\(h(t)=\left ( -\frac{1}{20}t+\sqrt{5} \right )^{2}\)