AP Calculus AB: 5.7 Using the Second Derivative Test to Determine Extrema

AP PhysicsAP Calculus AP ChemistryAP Biology

Question

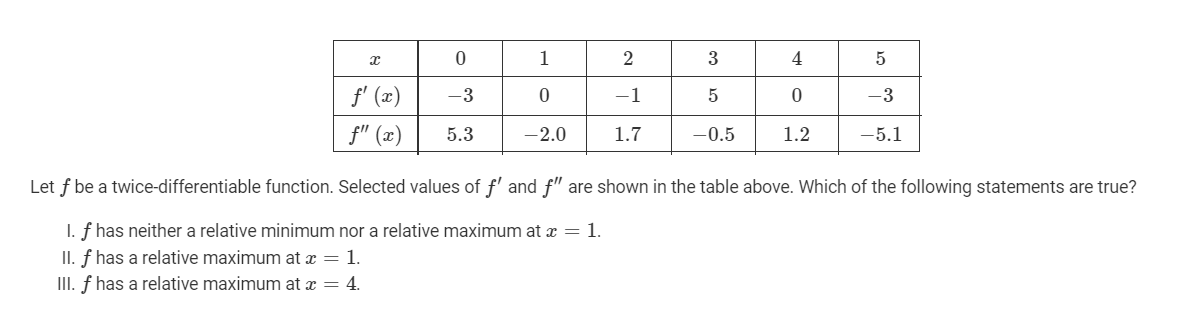
A I only

B ii ONLY

C III only

D I and III only

▶️Answer/Explanation

Ans:B

Since $f prime of 1, equals 0$ and $f double prime of 1, is less than 0$ , there is a relative maximum at $x equals 1$ by the Second Derivative Test. Therefore, statement II is true.Statement I is false since there is a relative maximum at $x equals 1$

x = 1

.Statement III is false since $f prime of 4, equals 0$ and $f double prime of 4, is greater than 0$ imply that there is a relative minimum at $x equals 4$ by the Second Derivative Test, not a relative maximum.

Question

Let $f$

$f$

be a twice-differentiable function. Selected values of $f^{'}$

$'$

and $f^{″}$

$″$

are shown in the table above. Which of the following statements are true?

$I. f$

has neither a relative minimum nor a relative maximum at $x equals 2$ .

$f$ has a relative maximum $x equals 2$ .

$f$ has a relative maximum $x equals 8$ .

A I only

B II only

C III only

D I and III only

▶️Answer/Explanation

Ans:B

Since $f prime of 2, equals 0$ and $f double prime of 2, is less than 0$ , there is a relative maximum at $x equals 2$ by the Second Derivative Test. Therefore, statement II is true.Statement I is false since there is a relative maximum at $x equals 2$

x = 2

.Statement III is false since $f prime of 8, equals 0$ and $f double prime of 8, is greater than 0$ implies that there is a relative minimum at $x equals 8$ by the Second Derivative Test, not a relative maximum.

Question

The derivative of
$f(x)=\frac{x^{4}}{3}-\frac{x^{5}}{5}$ attains its maximum value at x =

(A) –1 (B) 0 (C) 1 (D)$-\frac{1}{8} $ (E)$-\frac{1}{2}$

▶️Answer/Explanation

Ans:C

f”>0 for x <1 and f ‘ >0 for x>1 ⇒ f’ has its maximum at x = 1.

Question

An equation of the line tangent to $y=x^{3}+3x^{2}+2$ at its point of inflection is

(A)y=-6x-6 (B)y=-3x+1 (C)y=2x+10 (D)y=3x-1 (E)y=4x+1

▶️Answer/Explanation

Ans:E

AP Calculus AB: 5.7 Using the Second Derivative Test to Determine Extrema – Exam Style questions with Answer- MCQ

Question

Question

Question

Question

Resources

Members

Company