Question:
Consider the differential equation \(\frac{dy}{dx}= (3-y)cos x.\) Let y = f(x) be the particular solution to the differential equation with the initial condition f(0) = 1 . The function f is defined for all real numbers.
(a) A portion of the slope field of the differential equation is given below. Sketch the solution curve through the point (0, 1).
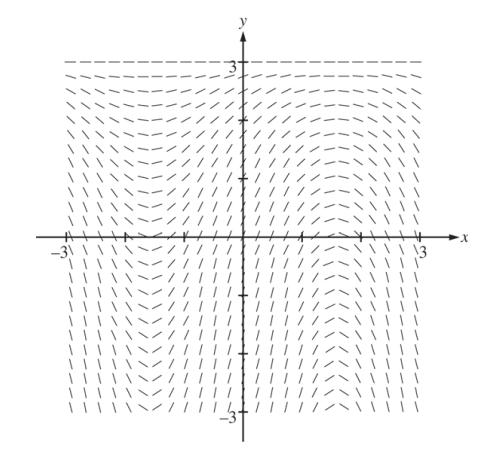
(b) Write an equation for the line tangent to the solution curve in part (a) at the point (0, 1) . Use the equation to approximate f(0.2) .
(c) Find y = f (x), the particular solution to the differential equation with the initial condition f(0) = 1 .
Answer/Explanation
Ans:
(a)
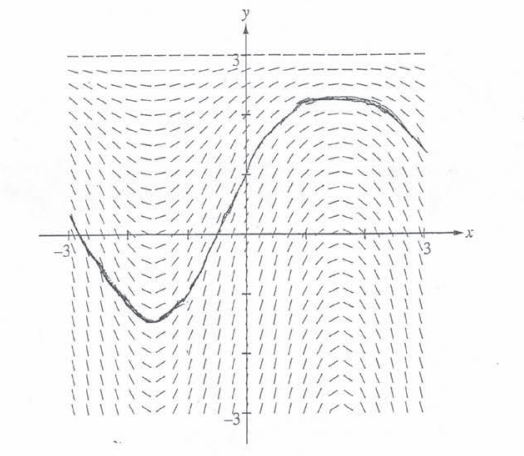
(b)
\(\frac{dy}{dx}= (3-y)cos x = m\)
\(\frac{dy}{dx}= (3-1)cos (0) = m\)
\(m = 2\)
\(y – 1 = 2(x-0)\)
\(y -1 = 2x\)
\(y = 2x + 1\)
\(y – 1 = 2(0.2 – 0)\)
\(y – 1 = 0.4\)
\(y = 1.4\)
\(f(0.2) ≈ 1.4\)
(c)
\(\int \frac{dy}{3-y}=\int cos x dx\) \(3 – y = u\)
\(-\int \frac{du}{u}=sin x + c\) \(du = -dx\)
\(- In |u| = sin x + c\)
\(- In |3-y| = sin x + c\) \(- In |3-y| = sin x – In 2\)
\(- In |3-1| = sin (0) + c \) \(In |3-y| = In 2 – sin x\)
\(- In |2| = 0 + c\) |3 – y| = eIn2 – sin x
\(c = – In 2\) \(\left | 3-y \right |= \frac{e^{In2}}{e^{sinx}}\)
|3-y| = 2e-sinx
3-y = 2e-sinx
-y = 2e-sinx 3
y = -2e-sinx + 3
Question:
Consider the differential equation \(\frac{dy}{dx}=2x – y.\)
(a) On the axes provided, sketch a slope field for the given differential equation at the six points indicated.
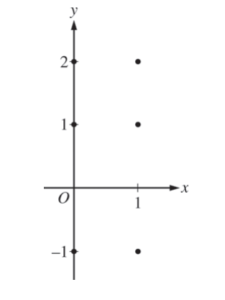
(b) Find \(\frac{d^{2}y}{dx^{2}}\) in terms of x and y. Determine the concavity of all solution curves for the given differential equation in Quadrant II. Give a reason for your answer.
(c) Let y = f(x) be the particular solution to the differential equation with the initial condition f (2) = 3.
Does f have a relative minimum, a relative maximum, or neither at x = 2 ? Justify your answer.
(d) Find the values of the constants m and b for which y = mx + b is a solution to the differential equation.
Answer/Explanation
Ans:
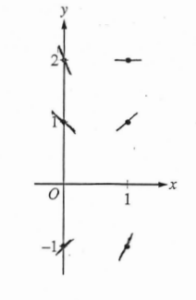
(b)
\(\frac{dy}{dx}= 2x – y\)
\(\frac{d^{2}y}{dx^{2}}= 2-\frac{dy}{dx}=2-(2x-y)\)
\(\frac{d^{2}y}{dx^{2}}= 2-2x+y\)
In Quadrant II, x < 0 and y > 0, So \(\frac{d^{2}y}{dx^{2}}= 2-2x+y>0,\)
Thus all solution curves in Quadrant II are concave up.
(c)
\(\frac{dy}{dx}= 2x-y=2.2-3 =1\)
Neither, as \(\frac{dy}{dx}\) ≠ 0 at x = 2.
(d)
\(\frac{dy}{dx}= 2x-y\), y = mx + b
\(\frac{dy}{dx}= m = 2x – y\)
m = 2x – (mx + b)
m = (2-m)x – b, equate coefficients
2 – m = 0
m = 2
-b = m
b = -m = -2.
m = 2, b = -2
Question:
Consider the differential equation \(\frac{dy}{dx}= \frac{y^{2}}{x – 1}.\)
(a) On the axes provided, sketch a slope field for the given differential equation at the six points indicated.
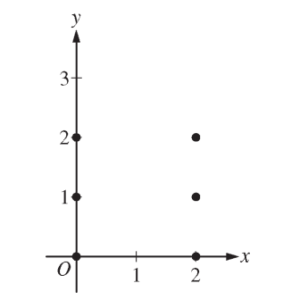
(b) Let y = f(x) be the particular solution to the given differential equation with the initial condition f (2) = 3. Write an equation for the line tangent to the graph of y = f(x) at x = 2.
Use your equation to approximate f(2.1).
(c) Find the particular solution y = f(x) to the given differential equation with the initial condition f (2) = 3.
Answer/Explanation
Ans:
(a)
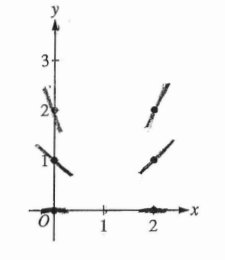
(b)
\(\frac{dy}{dx}= \frac{y^{2}}{x – 1}|_{(x,y)=(2, 3)}= \frac{9}{2-1}=\frac{9}{1}=9\)
y – 3 = 9 (x-2)
y = 9x – 18 + 3 = 9x – 15
y = 9(2.1) – 15 = 18.9 – 15 = 3.9
(c)
\(\frac{dy}{dx}= \frac{y^{2}}{x – 1}\)
\(\int \frac{dy}{y^{2}}=\int \frac{dx}{x-1}\)
\(\frac{y^{-1}}{-1}=ln|x-1| + c\)
\(\frac{-1}{y}=ln|x-1| + c\)
\(\frac{-1}{3}=ln|2-1| + c\)
\(\frac{-1}{3}=ln1 + c\)
\(c =\frac{-1}{3}\)
\(\frac{-1}{y} =ln(x-1)+\left ( \frac{-1}{3} \right )\)
\(-1 = \left ( ln (x-1)-\frac{1}{3} \right )y\)
\(y = \frac{-1}{ln (x-1)-\frac{-1}{3}}\)
Question:
At time t = 0, a boiled potato is taken from a pot on a stove and left to cool in a kitchen. The internal temperature of the potato is 91 degrees Celsius (°C) at time t = 0, and the internal temperature of the potato is greater than 27 C° for all times t > 0. The internal temperature of the potato at time t minutes can be modeled by the function H that satisfies the differential equation \(\frac{dH}{dt}=-\frac{1}{4}(H-27)\), where H(t) is measured in degrees Celsius and H( 0) = 91 .
(a) Write an equation for the line tangent to the graph of H at t = 0. Use this equation to approximate the internal temperature of the potato at time t = 3.
(b) Use \(\frac{d^{2}H}{dt^{2}}\) to determine whether your answer in part (a) is an underestimate or an overestimate of the internal temperature of the potato at time t = 3.
(c) For t < 10, an alternate model for the internal temperature of the potato at time t minutes is the function G that satisfies the differential equation \(\frac{dG}{dt} = -(G-27)^{2/3},\) where G(t) is measured in degrees Celsius and G(0) = 91. Find an expression for G (t). Based on this model, what is the internal temperature of the potato at time t = 3 ?
Answer/Explanation
Ans:
(a)
\(\frac{dH}{dt}_{H=91} = -\frac{1}{4}(91-27)=\left ( -\frac{1}{4} \right )(64)=-16\)
A(t) = -16 (t-0) + 91
A(t) = -16t + 91
A(3) = – 16(3) + 91
= -48 + 91
430C
at t = 3 minutes
(b)
\(\frac{dH}{dt} = -\frac{1}{4}(H-27)\) H > 27 for all t > 0
\(\frac{d^{2}H}{dt^{2}} = -\frac{1}{4}\left ( \frac{dH}{dt} \right )\) \(\frac{d^{2}H}{dt^{2}}\) is always positive,
\(-\frac{1}{4}\left ( -\frac{1}{4} \right )(H-24)\) So part (a) is an underestimate.
\(\frac{d^{2}H}{dt^{2}} = \frac{1}{16}(H-24)\)
(c)
\(\frac{dG}{dt} = -(G-27)^{2/3}\)
\(\int \frac{dG}{(G-27)^{2/3}}=\int -1dt\)
\(\int (G-27)^{-2/3}dt = -t+c\)
3 (G-27) 1/3 = -t + c1
\((G-27)^{-2/3} = -\frac{t}{3}+c_{2}\)
\(G-27 = \left ( -\frac{t}{3}+c_{2} \right )^{3}\)
\(G = \left ( -\frac{t}{3}+c_{2} \right )^{3}+27\)
at (0, 91)
\(91= (0+c_{2})^{3}+27\)
91 = (c2)3 + 27
64 = (c2)3 →c2 = 4
\(G(t)= \left ( -\frac{t}{3}+4 \right )^{3}+27\)
\(G(3)= \left ( \frac{-3}{3}+4 \right )^{3}+27\)
= (3)3 + 27
= 27 + 27
G(3) = 540C
Question:
Consider the differential equation \(\frac{dy}{dx}=\frac{1}{3}x(y-2)^{2}.\)
(a) A slope field for the given differential equation is shown below. Sketch the solution curve that passes through the point (0, 2), and sketch the solution curve that passes through the point (1, 0).
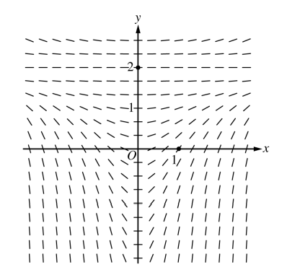
(b) Let y = f (x) be the particular solution to the given differential equation with initial condition f (1) = 0. Write an equation for the line tangent to the graph of y = f (x) at x = 1. Use your equation to approximate f (0.7).
(c) Find the particular solution y = f (x) to the given differential equation with initial condition f (1) = 0.
Answer/Explanation
Ans:
(a)
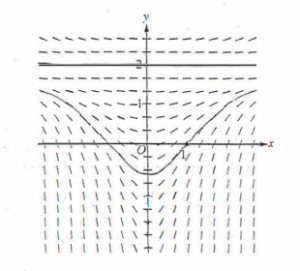
(b)
y = MT (x – 1) + 0 \(M_{T}=\frac{1}{3}\cdot 1(0-2)^{2}\)
y = 4/3 (x – 1) \(=\frac{1}{3}(4)\)
f(0.7) ≈ 4/3 (0.7 – 1) = 4/3
≈ 4/3 (-3/10)
≈ -4/10 = -2/5
(c)
u = y – 2
du = dy
\(\int \frac{dy}{(y-2)^{2}}=\int \frac{1}{3\times dx}\)
\(\int \frac{du}{u^{2}}=\frac{1}{3}\int x dx\)
\(\frac{-1}{4}=\frac{1}{3}\left ( \frac{x^{2}}{2} \right )+c\)
\(\frac{-1}{(y-2)}=\frac{x^{2}}{6}+c\)
point : x = 1
y = 0
\(c = \frac{1}{2}-\frac{1}{6}=\frac{3}{6}-\frac{1}{6}=\frac{2}{6}=\frac{1}{3}\)
\(\therefore \frac{-1}{y-2}=\frac{x^{2}}{6}+\frac{1}{3}\)
\(\frac{1}{y-2}=\frac{-x^{2}}{6}-\frac{1}{3}\)
\(y – 2 = \left ( \frac{1}{\frac{-x^{2}}{6}-\frac{2}{6}} \right )^{6/6}\)
\(y – 2 = \frac{b}{-(x^{2}+2)}\)
\(y = \frac{6}{-(x^{2}+2)}+2\)
Question:
A medication is administered to a patient. The amount, in milligrams, of the medication in the patient at time t hours is modeled by a function y = A(t ) that satisfies the differential equation \(\frac{dy}{dt}=\frac{12-y}{3}.\) At time t = 0 hours, there are 0 milligrams of the medication in the patient.
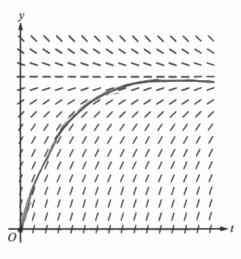
(a) A portion of the slope field for the differential equation \(\frac{dy}{dt}=\frac{12-y}{3}\) is given below. Sketch the solution curve through the point (0, 0).
(b) Using correct units, interpret the statement \(\lim_{t\rightarrow \infty }A(t)=12\) in the context of this problem.
(c) Use separation of variables to find y = A(t), the particular solution to the differential equation \(\frac{dy}{dt}=\frac{12-y}{3}\) with initial condition A(0) = 0.
(d) A different procedure is used to administer the medication to a second patient. The amount, in milligrams, of the medication in the second patient at time t hours is modeled by a function y = B(t) that satisfies the differential equation \(\frac{dy}{dt}=3 – \frac{y}{t+2}.\) At time t = 1 hour, there are 2.5 milligrams of the medication in the second patient. Is the rate of change of the amount of medication in the second patient increasing or decreasing at time t = 1 ? Give a reason for your answer.
Answer/Explanation
Ans:
(a)
(b)
As time goes to infinity, the amount of medication in the patient, in milligrams, is approaching 12.
(c)
\(\frac{dy}{dt}=\frac{12-y}{3}\)
\(\int \frac{dy}{12-y}=\int \frac{dt}{3}\)
\(-en|12-y| = \frac{1}{3}t+c\) \(-en|12-y| = \frac{1}{3}t-en 12\)
\(-en|12-0| = \frac{1}{3}(0)+c\) \(en|12-y| = \frac{1}{3}t+en 12\)
c = -en 12 \(e^{en|12-y|} = e^{-\frac{1}{3}t+en 12}\)
\(12-y = e^{-\frac{1}{3}t}.e^{en 12}\)
\(12-y = 12e^{-\frac{1}{3}t}\)
\(-y = 12e^{-\frac{1}{3}t}-12\)
\(y = -12e^{-\frac{1}{3}t}+12\)
(d)
\(\frac{dy}{dt}=3 – \frac{y}{t+2}\)
\(\frac{d^{2}y}{dt^{2}}=-\frac{dy/dx(t+2)-1(y)}{(t+2)^{2}}\)
\(\frac{d^{2}y}{dt^{2}}=-\frac{\left ( 3-\frac{y}{t+2} \right )(t+2)-y}{(t+2)^{2}}\)
\(\frac{d^{2}y}{dt^{2}}|_{(1, 2.5)}=-\frac{\left ( 3-\frac{2.5}{1+2} \right )(1+2)-2.5}{(1+2)^{2}}=\frac{\left ( 3-\frac{5}{3} \right )(3)-2.5}{3^{2}}=\sim \) #
