Question:
A particle moves along the x-axis with velocity given by \(v(t)=\frac{10 sin(0.4t^{2})}{t^{2}-t+3}\) for time 0 ≤ t ≤ 3.5. The particle is at position x = −5 at time t = 0.
(a) Find the acceleration of the particle at time t = 3.
(b) Find the position of the particle at time t = 3.
(c) Evaluate \(\int_{0}^{3.5}v(t)dt,\) and evaluate \(\int_{0}^{3.5}|v(t)|dt.\) Interpret the meaning of each integral in the context of the problem.
(d) A second particle moves along the x-axis with position given by x2(t) = t2 − t for 0 ≤ t ≤ 3.5 . At what time t are the two particles moving with the same velocity?
▶️Answer/Explanation
Ans:
(a)
v'(3) = -2.1182
(b)
\(-5+\int_{0}^{3}v(t)dt=-1.7602\)
(c)
\(\int_{0}^{3.5}v(t)dt=2.8439,\) which is the displacement of the particle from t = 0 to t = 3.5
\(\int_{0}^{3.5}|v(t)|dt=3.7371,\) which is the total distance the particle traveled from t = 0 to t = 3.5
(d)
v(t) = 2t – 1
t = 1.5705
Question:
For 0 ≤ t ≤ 6, a particle is moving along the x-axis. The particle’s position, x (t), is not explicitly given.
The velocity of the particle is given by \(v(t)=2sin\left ( e^{t/4} \right )+1.\) The acceleration of the particle is given by \(a(t)=\frac{1}{2}e^{t/4}cos\left ( e^{t/4} \right )\) and x(0) = 2.
(a) Is the speed of the particle increasing or decreasing at time t = 5.5 ? Give a reason for your answer.
(b) Find the average velocity of the particle for the time period 0 ≤ t ≤ 6.
(c) Find the total distance traveled by the particle from time t = 0 to t = 6.
(d) For 0 ≤ t ≤ 6,the particle changes direction exactly once. Find the position of the particle at that time.
▶️Answer/Explanation
Ans:
(a) The speed is increasing at t = 5.5 because v(5.5) < 0 and a(5.5) < 0
v (5.5) = -0.453
a (5.5) = -1.358
(b)
\(\frac{1}{6-0}\int_{0}^{6}v(t)dt = 1.949\)
(c)
\(\int_{0}^{6}\left | v(t) \right |dt = 12.573\)
(d)
v(t) = 0 at t=5.1955223 and v(t) changes sign at t = 5.1955223
\(X(5.195223)= 2+\int_{0}^{5.195523}v(t)dt\)
Position at t = 5.195523 is 14.134
Question:
For 0 ≤ t ≤ 12, a particle moves along the x-axis. The velocity of the particle at time t is given by \(v(t)=cos(\frac{\pi }{6}t)\). The particle is at position x = -2 at time t = 0.
(a) For 0 ≤ t ≤ 12, when is the particle moving to the left?
(b) Write, but do not evaluate, an integral expression that gives the total distance traveled by the particle from time t = 0 to time t = 6.
(c) Find the acceleration of the particle at time t. Is the speed of the particle increasing, decreasing, or neither at time t = 4 ? Explain your reasoning.
(d) Find the position of the particle at time t = 4.
▶️Answer/Explanation
Ans:
x(0) = -2
(a)
\(cos (\frac{\pi }{6}t)=0\) when x = 3 , x = 9
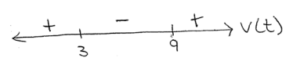
The particle is moving left on (3, 9)
(b)
\(D = \int_{0}^{6}\left | cos(\frac{\pi }{6}t) \right |dt\)
(c)
\(a(t)=v'(t)=-sin(\frac{\pi }{6}t)\cdot \frac{\pi }{6}=-\frac{\pi }{6}sin(\frac{\pi }{6}t)\)
\(a(4)=-\frac{\pi }{6} sin (\frac{2\pi }{3})=-\frac{\pi }{6}\left ( \frac{\sqrt{3}}{2} \right )=\frac{-\pi \sqrt{3}}{12}\)
\(v(4)= cos \left ( \frac{2\pi }{3} \right )=\frac{-1}{2}\)
The speed is increasing because a(t) and v(t) have the same sign (-) at t = 4
(d)
\(u = \frac{\pi }{6}t\)
\(du = \frac{\pi }{6}dt\)
\(x(t)=\int cos(\frac{\pi }{6}t)dt\)
\(x(t)=\frac{\pi }{6}\int cos u du\)
\(x(t)=\frac{\pi }{6}sin(\frac{\pi }{6}t)+C\)
\(\frac{\pi }{6}sin(\frac{\pi }{6}t)+C = -2 \) when x = 0
\(\frac{\pi }{6}sin(0)+C = -2 \)
C = -2
\(x(t)=\frac{\pi }{6}sin(\frac{\pi }{6}t) -2 \)
\(x(4)=\frac{\pi }{6}sin(\frac{2\pi }{3}) -2 \)
\(x(4)=\frac{\pi }{6}\left ( \frac{\sqrt{3}}{2} \right ) -2 \)
\(x(4)=\frac{2\sqrt{3}}{\pi} -2 \)
Question:
A particle moves along a straight line. For 0 ≤ t ≤ 5, the velocity of the particle is given by \(v(t)= -2 + (t^{2}+3t)^{6/5} – t^{3}\), and the position of the particle is given by s(t). It is known that s(0) = 10.
(a) Find all values of t in the interval 0 ≤ t ≤ 4 for which the speed of the particle is 2.
(b) Write an expression involving an integral that gives the position s(t). Use this expression to find the position of the particle at time t = 5.
(c) Find all times t in the interval 0 ≤ t ≤ 5 at which the particle changes direction. Justify your answer.
(d) Is the speed of the particle increasing or decreasing at time t = 4 ? Give a reason for your answer.
▶️Answer/Explanation
Ans:
(a)
|v(t) | = 2 2 ≤ t ≤ 4
t = 3.128, 3.473
(b)
\(s(t)= 10 + \int_{0}^{t}v(x)dx\)
\(s(5)= 10 + \int_{0}^{5}v(x)dx = -9.207\)
(c)
v(t) = 0
t = 0.536 , 3.318
the particle changes direction at 0.536 because v(t) < 0 for (0, 0.536) and v(t) > 0 for (0.536, 3.318). The particle changes direction at 3.318 because v(t) > 0 for (0.536, 3.318) and v(t) < 0 for t> 3.318
(d)
v(4) < 0
a(4) = v'(4) <0
The speed is increasing at t = 4 because both v(4) and a(4) = v'(4) are negative.
Question:
t (minutes) | 0 | 2 | 5 | 8 | 12 |
vA (t) (meters/ minute) | 0 | 100 | 40 | -120 | -150 |
Train A runs back and forth on an east-west section of railroad track. Train A’s velocity, measured in meters per minute, is given by a differentiable function vA (t) where time t is measured in minutes. Selected values for vA (t) are given in the table above.
(a) Find the average acceleration of train A over the interval 2 ≤ t ≤ 8.
(b) Do the data in the table support the conclusion that train A’s velocity is -100 meters per minute at some time t with 5 < t < 8 ? Give a reason for your answer.
(c) At time t = 2, train A’s position is 300 meters east of the Origin Station, and the train is moving to the east. Write an expression involving an integral that gives the position of train A, in meters from the Origin Station, at time t = 12. Use a trapezoidal sum with three subintervals indicated by the table to approximate the position of the train at time t = 12.
(d) A second train, train B, travels north from the Origin Station. At time t the velocity of train B is given by vB (t) = -5t2 + 60t + 25, and at time t = 2 the train is 400 meters north of the station. Find the rate, in meters per minute, at which the distance between train A and train B is changing at time t = 2.
▶️Answer/Explanation
Ans:
(a)
\(\frac{v(8)-v(2)}{8-2}\rightarrow \frac{-120-100}{6}\rightarrow \frac{-220}{6}\rightarrow \frac{-110}{3}m/min^{2}\)
(b)
Yes, because v(8) = -120 and v(5) = 40 and the function is differentiable and this continuous the train’s velocity must be -100 m/min at some point between 5 < t < 8 accordingly to the intermediate value theorem.
(c)
\(x(12)= \int_{2}^{12}{v_{A}}^{(t)}dt + x(2)\rightarrow x(12)=\int_{2}^{12}{v_{A}}^{(t)}dt +300\)
\(x(12)\approx 3\cdot \frac{1}{2}\cdot (140)+3\cdot \frac{1}{2}\cdot (-80)+4\cdot \frac{1}{2}\cdot (-270)+300\)
210 – 120 – 540 + 300
210 – 240 – 120
-30-120
150 meaning it is 150 m W of origin station
(d)
\(\frac{dB}{dt}= -5t^{2}+60t + 25 \rightarrow 125 m/min\)
-20 + 120 +25
120 + 5 → 135
\(\frac{dA}{dt}= 100 m/min\)
A2 + B2 + = D2
\(2A\frac{dA}{dt}+2B\frac{dB}{dt}=2D\frac{dD}{dt}\)
600(100) + 800 (125) = 1000 \(\frac{dD}{dt}\)
6000 + 100000 = 1000 \(\frac{dD}{dt}\)
\(\frac{160,000}{1000}=\frac{dD}{dt}\rightarrow \frac{dD}{dt}=160 m/min\)
Question:
| t (minutes) | 0 | 12 | 20 | 24 | 40 |
| v (t) (meters per minute) | 0 | 200 | 240 | -220 | 150 |
Johanna jogs along a straight path. For 0 ≤ t ≤ 40, Johanna’s velocity is given by a differentiable function v.
Selected values of v (t), where t is measured in minutes and v (t) is measured in meters per minute, are given in the table above.
(a) Use the data in the table to estimate the value of v'(16).
(b) Using correct units, explain the meaning of the definite integral \(\int_{0}^{40}|v(t)|dt\) in the context of the problem.
Approximate the value of \(\int_{0}^{40}|v(t)|dt\) using a right Riemann sum with the four subintervals indicated in the table.
(c) Bob is riding his bicycle along the same path. For 0 ≤ t ≤ 10, Bob’s velocity is modeled by B(t) = t3 – 6t + 300, where t is measured in minutes and B (t) is measured in meters per minute.
Find Bob’s acceleration at time t = 5.
(d) Based on the model B from part (c), find Bob’s average velocity during the interval0 ≤ t ≤ 10.
▶️Answer/Explanation
Ans:
(a)
\(V'(14)\approx \frac{v(20)-v(12)}{20-12}=\frac{240-200}{8}=\frac{40}{8}=\frac{20}{4}=5\frac{m}{min^{2}}\)
(b)
\(\int_{0}^{40}|v(t)|dt\) represents the total distance in meters Johanna traveled between times. t = 0 and t = 40 minutes.
\(\int_{0}^{40}|v(t)|dt\approx \left [ 12(200)+8(240)+4(220)+16(150) \right ]\)
= 2400 + 1920 + 880 + 2400
= 7600 meters
(c)
acceleration = B'(t) = 3t2 – 12t
B'(5) = 3(5)2 – 12(5)
= 75-60 = 15 \(\frac{m}{min^{2}}\)
(d)
Avg. Velocity = \(\frac{1}{10}\int_{0}^{10}(t^{3}-6t^{2}+300)dt\)
\(=\frac{1}{10}\cdot \left [ \frac{t^{4}}{4} -2t^{3}+300t\right ]_{0}^{10}\)
\(=\frac{1}{10}\cdot \left [ \frac{10000}{4} -2000+300t\right ]\)
\(=\frac{1}{10}\left [ 3500\right ]=350\frac{m}{min}\)
Question:
For t ≥ 0, a particle moves along the x-axis. The velocity of the particle at time t is given by \(v(t)=1+2 sin\left ( \frac{t^{2}}{2} \right )\) The particle is at position x = 2 at time t = 4.
(a) At time t = 4, is the particle speeding up or slowing down?
(b) Find all times t in the interval 0 < t < 3 when the particle changes direction. Justify your answer.
(c) Find the position of the particle at time t = 0.
(d) Find the total distance the particle travels from time t = 0 to time t = 3.
▶️Answer/Explanation
Ans:
(a)
\(v(4)=1+2 sin\left ( \frac{4^{2}}{2} \right )\)
v(4) = 2.979
\(v'(t)=2 cos\left ( \frac{t^{2}}{2} \right )(t)\)
\(v'(4)=2 cos\left ( \frac{4^{2}}{2} \right )(4)\)
v'(4) = -1.164
Showing down because v(A) is positive and v'(4) is negative.
(b)
\(1+2sin\left ( \frac{t^{2}}{2} \right )=0\)
t = 2.707
The particle changes direction one time at t = 2.707 because v(t) = 0 and v(t) changes from positive to negative.
(c)
\(2+\int_{4}^{0}v(t)dt = -3.815\)
(d)
\(2+\int_{0}^{3}|v(t)|dt = 5.301\)
Question:
Two particles move along the x-axis. For 0 ≤ t ≤ 8, the position of particle P at time t is given by xp(t) = ln(t2 − 2t + 10 ) , while the velocity of particle Q at time t is given by vQ(t) = t2 − 8t + 15 . Particle Q is at position x = 5 at time t = 0.
(a) For 0 ≤ t ≤ 8, when is particle P moving to the left?
(b) For 0 ≤ t ≤ 8, find all times t during which the two particles travel in the same direction.
(c) Find the acceleration of particle Q at time t = 2. Is the speed of particle Q increasing, decreasing, or neither at time t = 2 ? Explain your reasoning.
(d) Find the position of particle Q the first time it changes direction.
▶️Answer/Explanation
Ans:
(a)
\({v_{p}}(t)\) is neg.
\({v_{p}}(t)= {x_{p}}'(t)=\frac{1}{t^{2}+2t+10}\cdot (2t-2)\)
\(0=\frac{2t-2}{t^{2}+2t+10}\)
2t – 2 = 0
t = 1
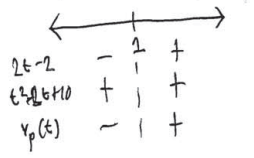
Particle p is moving to the left on 0 ≤ t < 1.
(b)
t2 – 8t + 15 = 0
(t-3)(t-5) = 0
t = 3.5
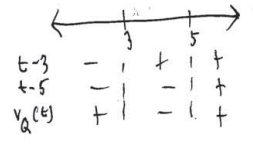
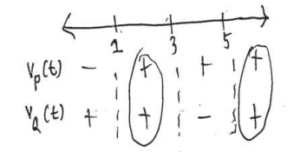
Particles P and Q are moving in the same direction when 1 < t < 3 and 5 < t ≤ 8.
(c)
aQ(t) = 2t – 8
aQ(2) = 2(2) – 8 = -4
The speed of particle Q is decreasing because the particle has a positive velocity but negative acceleration when t = 2.
(d)
@ t = 3, particle Q first changes direction
\({x_{Q}}^{(t)}=\int \left ( t^{2}-8t+15 \right )dt = \frac{t^{3}}{3}-4t^{2}+15t+c\)
\({x_{Q}}^{(0)}=c = 5\Rightarrow {x_{Q}}^{(t)} = \frac{t^{3}}{3}-4t^{2}+15t+5\)
\({x_{Q}}^{(3)}=\frac{3^{3}}{3} -4(3)^{2}+15(3)+5= 9-35+45+5=23\)
Question:
Fish enter a lake at a rate modeled by the function E given by \(E(t)=20 + 15 sin\left ( \frac{\pi t}{6} \right ).\) Fish leave the lake at a rate modeled by the function L given by L(t) = 4 + 20.1t2. Both E (t) and L (t) are measured in fish per
hour, and t is measured in hours since midnight (t = 0).
(a) How many fish enter the lake over the 5-hour period from midnight (t = 0) to 5 A.M. (t = 5) ? Give your answer to the nearest whole number.
(b) What is the average number of fish that leave the lake per hour over the 5-hour period from midnight (t = 0) to 5 A.M. (t = 5) ?
(c) At what time t, for 0 ≤ t ≤ 8, is the greatest number of fish in the lake? Justify your answer.
(d) Is the rate of change in the number of fish in the lake increasing or decreasing at 5 A.M. (t = 5) ? Explain your reasoning.
▶️Answer/Explanation
Ans:
(a)
\(\int_{0}^{5}E(t)dt\approx 153 fish\)
(b)
\(\frac{1}{5}\int_{0}^{5}L(t)dt\approx \frac{30.295}{5}\approx 6.059\) fish per hour leave the lake
(c)
E(t) – L(t) = 0
t ≈ 6.204
E(t) – L(t) is the rate at which the number of fish is changing
At time t = 6.204, the greatest number of fish in the 8 hour period are in the lake. This is because E(t) – L(t) is positive from t = 0 + 0 t = 6.204 indicating that the number of fish in the lake is increasing over (0, 3.204), but E(t) – L(t) is negative from t = 6.204 to t = 8, which means the number of fish are decreasing in this time period, so the number of fish in the lake is greatest at t = 6.204 hours
(d)
\(\frac{d}{dt}\left ( 16+15 sin(\frac{\pi t}{6})-2^{0.1t2} \right )|_{t=5}\approx -10.723 fish / hour^{2}\)
Since the derivative of E(t) – L(t) at t = 5 is negative, the rate of change in the number of fish in the lake is decreasing
Question:
| t(hours) | 0 | 0.3 | 1.7 | 2.8 | 4 |
| \(v_P(t)\) (meters per hour) | 0 | 55 | -29 | 55 | 48 |
The velocity of a particle, P, moving along the x-axis is given by the differentiable function vp , where \(v_P(t)\) is measured in meters per hour and t is measured in hours. Selected values of \(v_P(t)\) are shown in the table above. Particle P is at the origin at time t = 0.
(a) Justify why there must be at least one time t, for 0.3 ≤ t ≤ 2.8, at which \(v_P(t)\) , the acceleration of particle P, equals 0 meters per hour per hour.
(b) Use a trapezoidal sum with the three sub-intervals [0, 0.3], [0.3, 1.7], and [1.7, 2.8] to approximate the value of \(\int_{0}^{2.8}v_{p}(t)dt.\)
(c) A second particle, Q, also moves along the x-axis so that its velocity for 0 ≤ t ≤ 4 is given by \(v_{Q}(t)=45\sqrt{t}cos(0.063t^{2})\) meters per hour. Find the time interval during which the velocity of particle Q is at least 60 meters per hour. Find the distance traveled by particle Q during the interval when the velocity of particle Q is at least 60 meters per hour.
(d) At time t = 0, particle Q is at position x = −90. Using the result from part (b) and the function vQ from part (c), approximate the distance between particles P and Q at time t = 2.8.
▶️Answer/Explanation
Ans:
(a)
\({v_{p}}'(t)=9_{p}(t)\) \(\frac{v_{p}(2.8)-v_{p}(0.3)}{2.8-0.3}\)
Since \(v_P(t)\) is a continuous and differentiable function, MVT states that there must be at least one time t, for 0.3 ≤ t ≤ 2.8 at which \({v_{p}}'(t)=\frac{v_{p}(2.8)-v_{p}(0.3)}{2.8-0.3}=\frac{55-55}{2.5}=0.\)
(b)
\(0.3\left ( \frac{55+0}{2} \right )+1.4\left ( \frac{-29+55}{2} \right )+1.1\left ( \frac{55+(-29)}{2} \right )\)
8.25 + 18.2 + 14.3
\(\int_{0}^{2.8}{v_{p}}^{(t)}=40.75\)
(c)
\(60 = 45\sqrt{t}cos (0.053t^{2})\)
t = 1.866, 3.519
1.866 ≤ t ≤ 3.519
\(\int_{1.866}^{3.519}45\sqrt{t}cos (0.063t^{2})dt\)
= 106.109
106.109 meters
(d)
\(X_p(2.8)= 40.75\)
\(x_{Q}(2.8) = -90 +\int_{0}^{2.8}45\sqrt{t}cos (0.063t^{2})dt\)
= -90 + 135.938 = 45.938
45.938 – 40.75 = 5.188
The distance between particles P and Q at t = 2.8 is 5.188 meters
Question:
A particle, P, is moving along the x-axis. The velocity of particle P at time t is given by \(v_{p}(t)= sin(t^{1.5})\) for 0 ≤ t ≤ π. At time t = 0, particle P is at position x = 5.
A second particle, Q, also moves along the x-axis. The velocity of particle Q at time t is given by \(v_{Q}(t)\) = (t – 1.8) • 1.25t for 0 ≤ t ≤ π. At time t = 0, particle Q is at position x = 10.
(a) Find the positions of particles P and Q at time t = 1.
(b) Are particles P and Q moving toward each other or away from each other at time t = 1 ? Explain your reasoning.
(c) Find the acceleration of particle Q at time t = 1. Is the speed of particle Q increasing or decreasing at time t = 1 ? Explain your reasoning.
(d) Find the total distance traveled by particle P over the time interval 0 ≤ t ≤ π.
▶️Answer/Explanation
Ans:
(a)
position of \(p = x_{p}=5+\int_{0}^{1}sin(t^{1.5})dt=5+0.37066\)
position of \(Q = x_{Q}=10+\int_{0}^{1}{v_{Q}}^{(t)}dt=8.56435\)
At time t=1, the position of particle p is 5.37066 and the position of particle Q is 8.56435
(b)
\(v_{Q}(1)= -1\) \(X_{Q}(1)= 5.37066\)
\(v_{P}(2) = 0.84147\) \(X_{P}(2) = 8.80035\)
At time t=1 the 2 particles are moving closer to each other because xp(1) = 5.37066 and xQ(1) = 8.56435, which means that particle Q is to the right of particle p at t = 1, and since particle Q has a negative velocity it is moving left and particle p has a positive velocity it is moving right, so the particles are moving toward each other at time t=1.
(c)
\(a_Q\)= acceleration of particle Q
\(a_Q = {v_{Q}}'(1)\) \({v_{Q}}'(1)= 1.02686.\)
\(v_{a}(1)= -1\) Since the \(a_{Q}(1)\) is 1.02686 and is positive and and \(v_{Q}(1)\) is negative, the speed of particle Q at time t=1 must be decreasing.
(d)
Total distance particle P travelled from \(0\leq t\leq \pi =\int_{0}^{\pi }|{v_{p}}^{(t)}|dt=1.93148\)
The total distance particle p travelled from 0 ≤ t ≤ π is 1.93148.
