Tangent Line Approximation and Differentials
The line tangent to a curve at a point is the line that best approximates the curve near that point. An equation for the tangent line at the point $(c, f(c))$ is given by $y-f(c)=f^{\prime}(c)(x-c)$ or $y=f(c)+f^{\prime}(c)(x-c)$ and the approximation
$
f(x) \approx f(c)+f^{\prime}(c)(x-c)
$
is called the tangent line approximation of $f$ at $c$. The linear function
$
L(x)=f(c)+f^{\prime}(c)(x-c)
$
is called the linearization of $f$ at $c$.
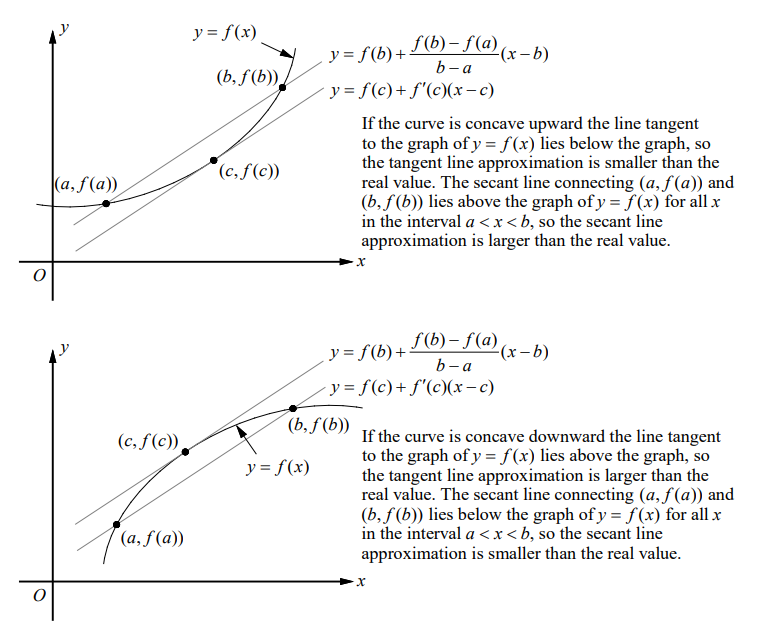
Definition of Differentials
Let $y=f(x)$ be a differentiable function. The differential $d x$ is an independent variable. The differential $\boldsymbol{d} \boldsymbol{y}$ is
$
d y=f^{\prime}(x) d x
$
Estimating with Differentials
Differentials can be used to approximate function values.
$
f(x+\Delta x) \approx f(x)+d y \approx f(x)+f^{\prime}(x) d x
$
Example 1
- (a) Find the tangent line approximation of $f(x)=\sqrt{x-1}$ at $c=5$ and approximate the number $\sqrt{3.95}$.
(b) Find the tangent line approximation of $f(x)=\tan x$ at $c=\pi / 4$ and approximate the number $\tan 47^{\circ}$.
▶️Answer/Explanation
Solution
(a) $f(x)=\sqrt{x-1} \Rightarrow f(5)=\sqrt{5-1}=2$
$
f^{\prime}(x)=\frac{1}{2 \sqrt{x-1}} \Rightarrow f^{\prime}(5)=\frac{1}{2 \sqrt{5-1}}=\frac{1}{4}
$
$\begin{array}{ll}y=2+\frac{1}{4}(x-5) & \text { Tangent line approximation } \\ y=\frac{1}{4} x+\frac{3}{4} & \text { Simplify. } \\ \sqrt{x-1} \approx \frac{1}{4} x+\frac{3}{4} & f(x) \approx f(c)+f^{\prime}(c)(x-c) \\ \sqrt{3.95}=\sqrt{4.95-1} & x=4.95 \\ \approx \frac{1}{4}(4.95)+\frac{3}{4}=1.9875 & \end{array}$
(b) $
\begin{aligned}
& f(x)=\tan x \Rightarrow f\left(\frac{\pi}{4}\right)=\tan \left(\frac{\pi}{4}\right)=1 \\
& f^{\prime}(x)=\sec ^2 x \Rightarrow f^{\prime}\left(\frac{\pi}{4}\right)=\sec ^2\left(\frac{\pi}{4}\right)=2
\end{aligned}
$
$\begin{array}{ll}y=1+2\left(x-\frac{\pi}{4}\right) & \text { Tangent line approximation } \\ y=2 x-\frac{\pi}{2}+1 & \text { Simplify. } \\ \tan x \approx 2 x-\frac{\pi}{2}+1 & f(x) \approx f(c)+f^{\prime}(c)(x-c) \\ \tan 47^{\circ} \approx 2\left(47^{\circ} \cdot \frac{\pi}{180}\right)-\frac{\pi}{2}+1 & x=47^{\circ} \text { and } 47^{\circ}=47 \cdot \frac{\pi}{180} \text { radian } \\ \approx 1.0698 & \end{array}$
Exercises – Tangent Line Approximation and Differentials
Multiple Choice Questions
- 1. For small values of $h$, the function $h(x)=\sqrt[3]{8+h}$ is best approximated by which of the following?
(A) $\frac{h}{12}$
(B) $2-\frac{h}{12}$
(C) $2+\frac{h}{12}$
(D) $3+\frac{h}{12}$
▶️Answer/Explanation
Ans:C
Example
- 2. The approximate value of $y=\sqrt{1-\sin x}$ at $x=-0.1$, obtained from the line tangent to the graph at $x=0$, is
(A) 0.9
(B) 0.95
(C) 1.01
(D) 1.05
▶️Answer/Explanation
Ans:D
Example
- 3. Let $y=x^2 \ln x$. When $x=e$ and $d x=0.1$, the value of $d y$ is
(A) $\frac{e}{10}$
(B) $\frac{e}{5}$
(C) $\frac{3 e}{10}$
(D) $\frac{2 e}{5}$
▶️Answer/Explanation
Ans:C
Example
- 4. Let $f$ be a differentiable function such that $f(2)=\frac{5}{2}$ and $f^{\prime}(2)=\frac{1}{2}$. If the line tangent to the graph of $f$ at $x=2$ is used to find an approximation of a zero of $f$, that approximation is
(A) -3
(B) -2.4
(C) -1.8
(D) -1.2
▶️Answer/Explanation
Ans:A
Example
- 5. The approximate value of $y=\frac{1}{\sqrt{x}}$ at $x=4.1$, obtained from the line tangent to the graph at $x=4$ is
(A) $\frac{39}{80}$
(B) $\frac{79}{160}$
(C) $\frac{1}{2}$
(D) $\frac{81}{160}$
▶️Answer/Explanation
Ans:B
