Question
Which of the following limits does not yield an indeterminate form?
A \(\lim_{x\rightarrow 0}\frac{4x^{3}}{4(x)-1}\)
B \(\lim_{x\rightarrow 3}\frac{\ln (\frac{x}{3})}{x^{2}-7x+12}\)
C \(\lim_{x\rightarrow \pi }\frac{\pi -x}{\sin (2x)-1}\)
D \(\lim_{x\rightarrow \infty }\frac{x^{10}}{e^{2x}+x}\)
Answer/Explanation
Ans:C
Question
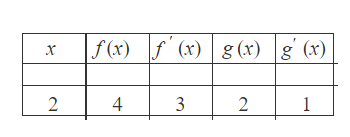
Selected values of the twice-differentiable functions f and g and their derivatives are given in the table above. The value of \(\lim_{x\rightarrow 2}\frac{x^{2}f(x)-16}{g(x)-2}\) is
A −28
B −12
C 28
D nonexistent
Answer/Explanation
Ans:C
Both f and g are continuous because they are differentiable. Since \(\lim_{x\rightarrow 2}(x^{2}f(x)-16)\)=4⋅f(2)−16=4⋅4−16=0 and \(\lim_{x\rightarrow 2}(g(x)-2)\)=2−2=0, the limit is of the indeterminate form \(\frac{0}{0}\) . Therefore, L’Hospital’s Rule can be used to find the limit. Both f′ and g′ are continuous because they are differentiable.
Question
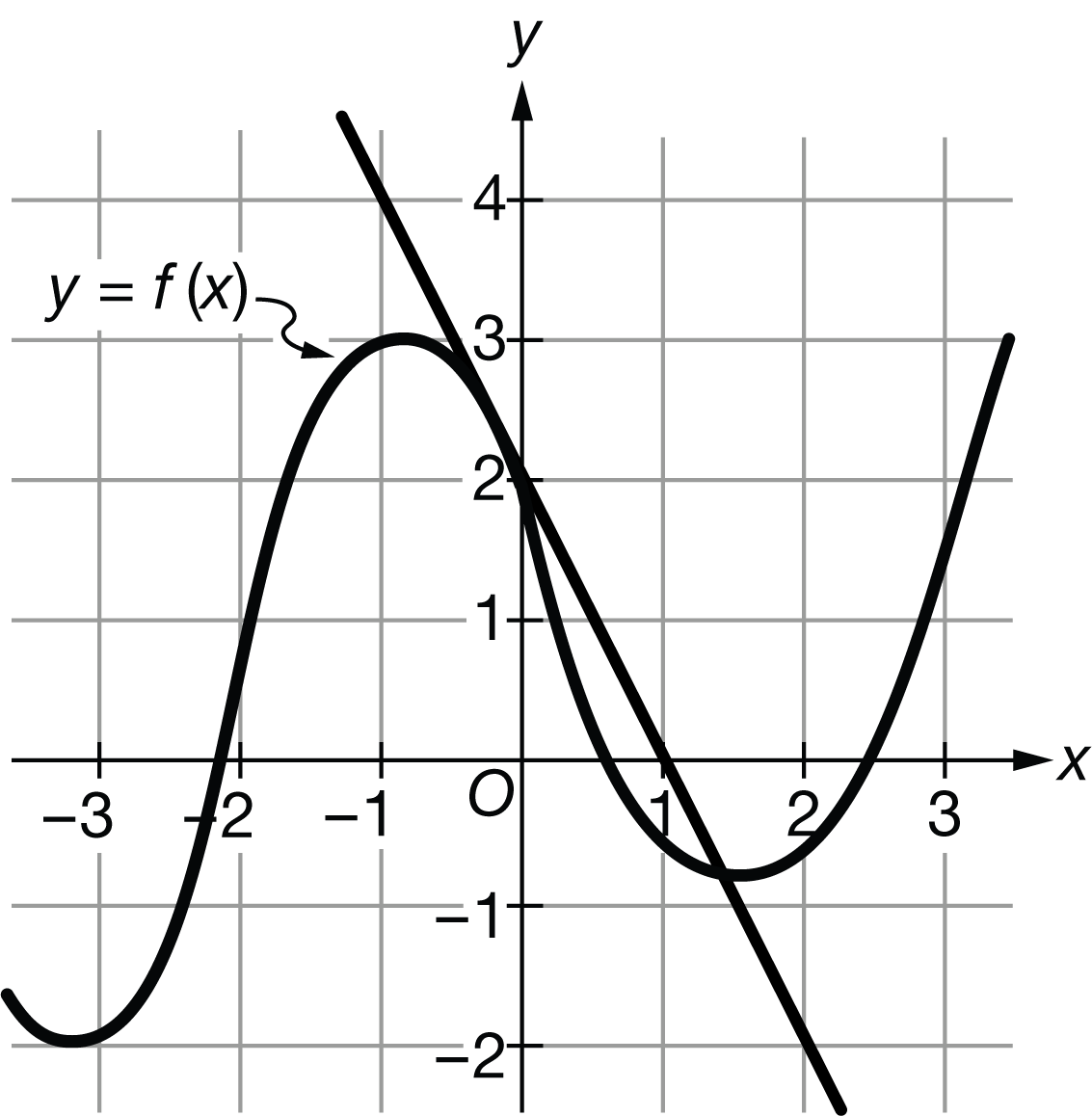
The figure above shows the graph of the twice-differentiable function f and the line tangent to the graph of f at the point (0,2). The value of \(\lim_{x\rightarrow 0}\frac{f(x)e^{-x}-2}{x^{2}-2x}\) is
A −1
B 0
C2
D nonexistent
Answer/Explanation
Ans:C
Substituting 0 into the fraction yields the indeterminate form 00 because both the value of the numerator and the value of the denominator are equal to 0. From the graph, f(0)=2. Therefore, L’Hospital’s Rule can be used to find the limit.
\(\lim_{x\rightarrow 0}\frac{f(x)e^{-x}-2}{x^{2}-2x}=\lim_{x\rightarrow 0}\frac{f'(x)e^{-x}-f(x)e^{-x}}{2x-2}\)
Question
what is \(\lim_{h\rightarrow 0}\frac{8\left ( \frac{1}{2}+h \right )^{8}-8\left ( \frac{1}{2} \right )^{8}}{h}\)?
(A) 0 (B)\(\frac{ 1}{2}\) (C) 1 (D) The limit does not exist. (E) It cannot be determined from the information given.
Answer/Explanation
Ans:B
