Question
Find an inflection point for the function \(f(x)=2x(x+4)^{3}\)
(A) (0, 0)
(B) (-4, 0)
(C) (-2, 4)
(D) (4, 0)
Answer/Explanation
Ans:(B)
Use the second derivative to find points of inflection. \(f'(x)=6x(x+4)^{2}+2(x+4)^{3}\).Then we find \(f”(x)=12x(x+4)+6(x+4)^{2}+6(x+4)^{2}=12x(x+4)+12(x+4)^{2}\).You can
Question
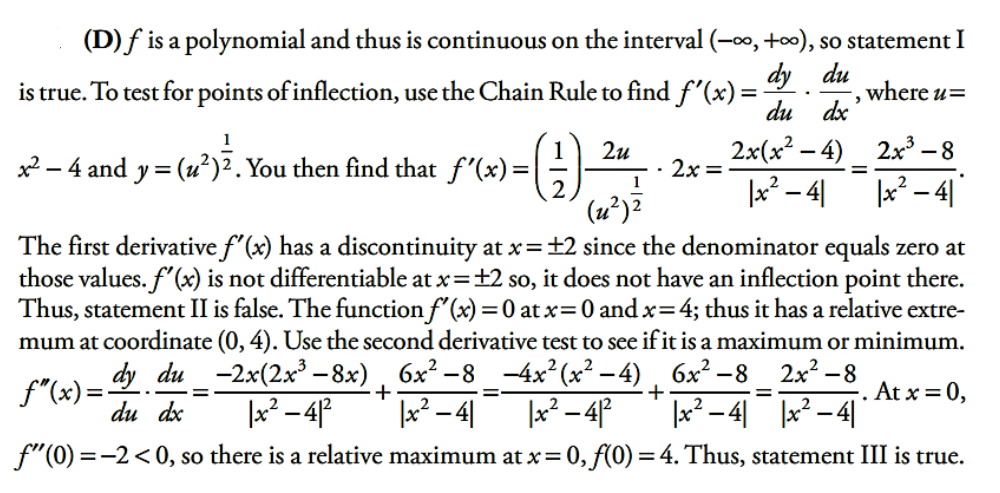
If \(f(x)=\left | x^{2} -4\right |\), which of the following statements about f is/are true?
I. f is continuous on the interval (-∞, +∞).
II. f has points of inflection at x = ±2.
III. f has a relative maximum at (0, 4).
(A) I only
(B) II only
(C) III only
(D) I and III
Answer/Explanation
Ans:(D)
Question
The derivative of
\(f(x)=\frac{x^{4}}{3}-\frac{x^{5}}{5}\) attains its maximum value at x =
(A) –1 (B) 0 (C) 1 (D)\(-\frac{1}{8} \) (E)\(-\frac{1}{2}\)
Answer/Explanation
Ans:C
![]()
Question
An equation of the line tangent to \(y=x^{3}+3x^{2}+2\) at its point of inflection is
(A)y=-6x-6 (B)y=-3x+1 (C)y=2x+10 (D)y=3x-1 (E)y=4x+1
Answer/Explanation
Ans:E
Use the technique of antiderivatives by part:
\(u sin^{-1}x dv=dx\)
\(du=\frac{dx}{\sqrt{1-x^2}} v=x\)
