Question
The base of a solid is the region enclosed by the graph of \(y=e^{-x}\) , the coordinate axes, and the line
x = 3. If all plane cross sections perpendicular to the x-axis are squares, then its volume is
(A)\(\frac{\left ( 1-e^{-6} \right )}{2}\)
(B)\(\frac{1}{2}e^{-6}\)
(C)\(e^{-6}\)
(D)\(e^{-3}\)
(E)\(1-e^{-3}\)
Answer/Explanation
Question
The base of a solid is the region in the first quadrant enclosed by the parabola\( y = 4x^{3}\), the line x =1, and the x-axis. Each plane section of the solid perpendicular to the x-axis is a square. The volume of the solid is
(A) \(\frac{4π}{3}\) (B) \(\frac{16π}{5}\) (C) \(\frac{4}{3}\) (D) \(\frac{16}{5}\) (E)\(\frac{64}{5}\)
Answer/Explanation
Ans:D
Square cross – sections :\(\sum y^2\Delta x\) where \(y=4x^2\).
Question
The base of a solid is the region enclosed by the graph of \(y=e^{-x}\) , the coordinate axes, and the line
x = 3. If all plane cross sections perpendicular to the x-axis are squares, then its volume is
(A)\(\frac{\left ( 1-e^{-6} \right )}{2}\)
(B)\(\frac{1}{2}e^{-6}\)
(C)\(e^{-6}\)
(D)\(e^{-3}\)
(E)\(1-e^{-3}\)
Answer/Explanation
Ans:A
Square cross sections: 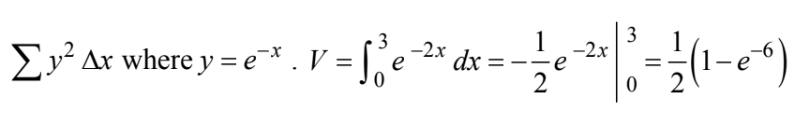
Question
Consider a solid S whose base is the region enclosed by the curve \(x=y^{2}\)and the line x = 3, and whose parallel cross sections perpendicular to the x-axis are squares. Find the volume of S.
(A) 6
(B) 9
(C) 18
(D) 27
Answer/Explanation
Ans:(C)
The length of each square cross section is a function of x given by \(s(x)=2\sqrt{x}\) ,and therefore each square has area A(x)=4x. The volume of the described solid is thus
