Question
The region in the first quadrant bounded by the graph of\( y=secx,x=\frac{\pi }{4}\) , and the axes is rotated about the x-axis. What is the volume of the solid generated?
(A)\(\frac{\pi ^{2}}{4}\) (B)\(\pi -1 \) (C)\(\pi\) (D)\(2\pi\) (E)\(\frac{8\pi }{3}\)
Answer/Explanation
Question
The region R in the first quadrant is enclosed by the lines x = 0 and y = 5 and the graph of \(y= x^{2}+1\). The volume of the solid generated when R is revolved about the y-axis is
(A) 6π (B) 8π (C) \(\frac{34π}{3}\) (D) 16π (E) \frac{544π}{15}\)
Answer/Explanation
Ans:B
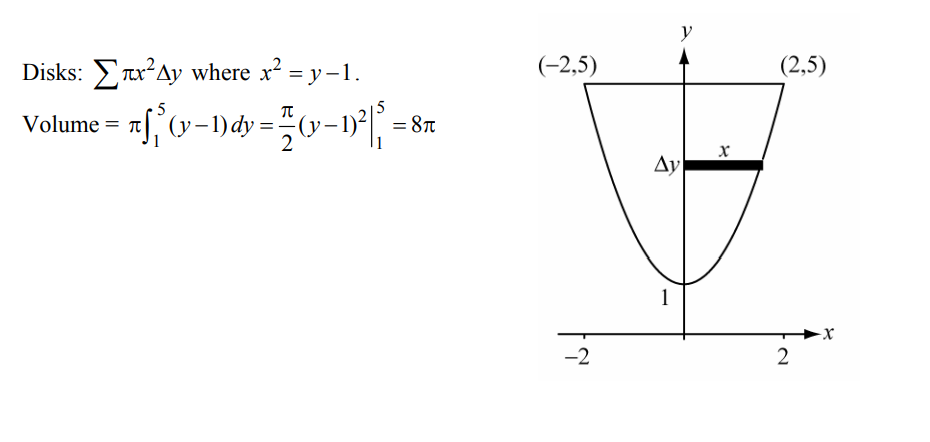
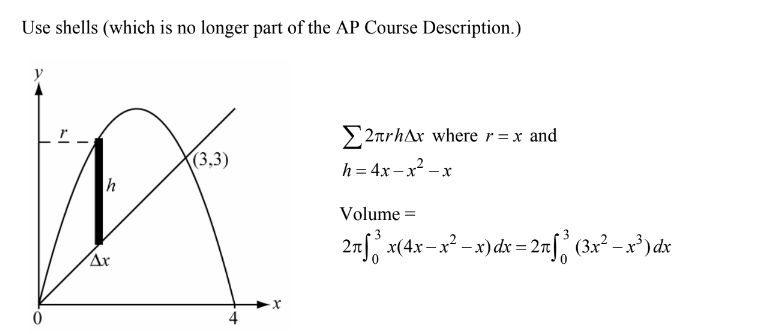
Question
When the region enclosed by the graphs of y = x and \(y=4x-x^{2}\) is revolved about the y-axis, the volume of the solid generated is given by
(A)\(\pi\int_{0}^{3}(x^3-3x^2)dx\)
(B)\(\pi\int_{0}^{3}\left ( x^2\left ( 4x-x^2 \right ) \right )dx\)
(C)\(\pi\int_{0}^{3}(3x-x^2)^{2}dx\)
(D)\(2\pi\int_{0}^{3}(x^3-3x^2)dx\)
(E)\(2\pi\int_{0}^{3}(3x^3-x^2)dx\)
Answer/Explanation
Ans:E
Question
The base of a solid is the region in the first quadrant enclosed by the graph of\( y=2-x^2\) and the coordinate axes. If every cross section of the solid perpendicular to the y-axis is a square, the
volume of the solid is given by
(A)\(\pi\int_{0}^{2}(2-y)^{2}dy\)
(B)\(\int_{0}^{2}(2-y)^{2}dy\)
(C)\(\pi\int_{0}^{\sqrt{2}}\left ( 2-x^{2} \right )^{2}dx\)
(D)\(\int_{0}^{\sqrt{2}}\left ( 2-x^{2} \right )^{2}dx\)
(E)\(\int_{0}^{\sqrt{2}}\left ( 2-x^{2} \right )dx\)
Answer/Explanation
Ans:B
