Question
A student is given a 25.0 mL sample of a solution of an unknown monoprotic acid and asked to determine the concentration of the acid by titration. The student uses a standardized solution of 0.110 M NaOH(aq), a buret, a flask, an appropriate indicator, and other laboratory equipment necessary for the titration.
(a) The images below show the buret before the titration begins (below left) and at the end point (below right). What should the student record as the volume of NaOH(aq) delivered to the flask?
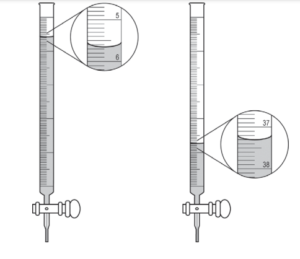
(b) Based on the given information and your answer to part (a), determine the value of the concentration of the acid that should be recorded in the student’s lab report.
(c) In a second trial, the student accidentally added more NaOH(aq) to the flask than was needed to reach the end point, and then recorded the final volume. Would this error increase, decrease, or have no effect on the calculated acid concentration for the second trial? Justify your answer.
▶️Answer/Explanation
Ans:
(a)
| 37.30 mL – 5.65 mL = 31.65 mL |
(b)
| At the equivalence point, moles OH– added = moles of H+ consumed. Because HA is monoprotic: \((0.110 M)(0.03165L)\times \frac{1 mol HA}{1 mol NaOH}\times \frac{1}{0.0250 L}=0.139 M\) OR moles of H+ consumed = MaVa MaVa = MbVb Therefore, \(M_{a}=\frac{M_{b}V_{b}}{V_{a}}=\frac{(0.110 M)(0.03165 L)}{0.0250 L}=0.139 M\) |
(c)
| The error would increase the calculated acid concentration. A volume of NaOH(aq) larger than the actual volume needed to reach the equivalence point, would lead to a calculation of moles of base that would be greater than the moles of acid actually present in the solution. The assumption that the moles of acid are the same as the moles of base would lead to a calculated concentration of acid that would be higher than the actual concentration |
