Question
\(CaCO_{3}(s)\rightleftharpoons CaO(s)+CO_{2}(g)\)
When heated, calcium carbonate decomposes according to the equation above. In a study of the decomposition of calcium carbonate, a student added a 50.0 g sample of powdered \(CaCO_{3}\)(s) to a 1.00 L rigid container. The student sealed the container, pumped out all the gases, then heated the container in an oven at 1100 K. As the container was heated, the total pressure of the \(CO_2\)(g) in the container was measured over time. The data are plotted in the graph below.
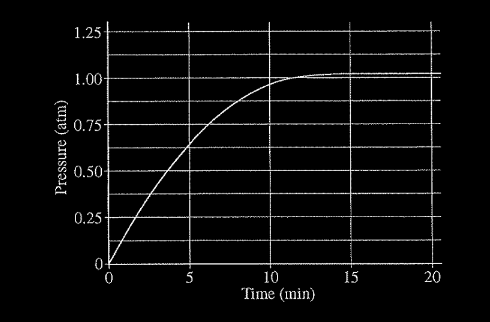
The student repeated the experiment, but this time the student used a 100.0 g sample of powdered \(CaCO_3\)(s). In this experiment, the final pressure in the container was 1.04 atm, which was the same final pressure as in the first experiment.
(a) Calculate the number of moles of \(CO_{2}(g)\) present in the container after 20 minutes of heating.
(b) The student claimed that the final pressure in the container in each experiment became constant because all of the\( CaCO_{3}\)(s) had decomposed. Based on the data in the experiments, do you agree with this claim? Explain.
(c) After 20 minutes some \(CO_2\)(g) was injected into the container, initially raising the pressure to 1.5 atm. Would the final pressure inside the container be less than, greater than, or equal to 1.04 atm? Explain your reasoning.
(d) Are there sufficient data obtained in the experiments to determine the value of the equilibrium constant, K,for the decomposition of \(CaCO_3\)(s) at 1100 K? Justify your answer.
▶️Answer/Explanation
(a) \(PV=nRT\)
\(\frac{PV}{RT}=n=\frac{(1.04 atm)(1.00 L)}{(0.0821\frac{L atm}{mol K})(1100 K)}== 0.0115 mol CO_2\)
(b) Do not agree with claim
Explanation I: In experiment 1, the moles of \(CaCO_3 = 50.0 g/100.09 g/mol = 0.500 mol CaCO_3\). If the reaction had gone to completion, 0.500 mol of \(CO_2\) would have been produced. From part (a) only 0.0115 mol was produced. Hence, the student’s claim was false.
Explanation II: The two different experiments (one with 50.0 g of \(CaCO_3\) and one with 100.0 g of \(CaCO_3\)) reached the same constant, final pressure of 1.04 atm. Since increasing the amount of reactant did not produce more product, there is no way that all of the \(CaCO\), reacted. Instead, an equilibrium condition has been achieved and there must be some solid \(CaCO_3\) in the container.
(c) The final pressure would be equal to 1.04 atm. Equilibrium was reached in both experiments; the equilibrium pressure at this temperature is 1.04 atm. As the reaction shifts toward the reactant, the amount of \(CO_2\)(g) in the container will decrease until the pressure returns to 1.04 atm.
(d) Yes. For the equilibrium reaction represented by the chemical equation in this problem, at a given temperature the equilibrium pressure of \(CO_2\) determines the equilibrium constant. Since the measured pressure of \(CO_2\) is also the equilibrium pressure of \(CO_2\), \(K_p= P_{co_2} =1.04\).
Note: If the response in part (b) indicates “yes”, that all of the \(CaCO_3\)(s) had decomposed, then the point can be earned by stating that the system did not reach equilibrium in either experiment and hence the value of \(K_p\), cannot be calculated from the data.
Question

The half-life (1/2) of the catalyzed isomerization of cis-2-butene gas to produce trans-2-butene gas, represented above, was measured under various conditions, as shown in the table below.
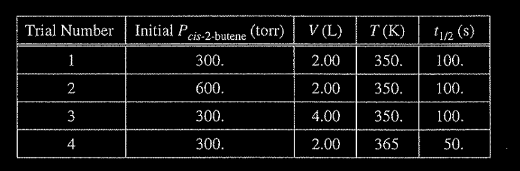
(a) The reaction is first order. Explain how the data in the table are consistent with a first-order reaction.
(b) Calculate the rate constant, k, for the reaction at 350. K. Include appropriate units with your answer.
(c) Is the initial rate of the reaction in trial I greater than, less than, or equal to the initial rate in trial 2? Justify your answer.
(d) The half-life of the reaction in trial 4 is less than the half-life in trial 1. Explain why, in terms of activation energy.
▶️Answer/Explanation
(a) For a first-order reaction, the half-life is independent of reactant concentration (or pressure) at constant 7, as shown in trials 1, 2, and 3.
(b) \(k=\frac{0.693}{t_{1/2}}=\frac{ 0.693}{ 100 s} = 0.00693 s^{-1}\)
(c) The initial rate in trial I is less than that in trial 2 because rate = k[cis-2-butene] or rate \(= k _{Pcis-2-butene}\) (with reference to values from both trials). OR because the initial concentration of cis-2-butene in trial 1 is less than that in trial 2 and it is constant.
(d) The temperature is higher in trial 4, meaning that the \(KE_{avg}\) of the molecules is greater. Consequently, in this trial a greater fraction of collisions have sufficient energy to overcome the activation energy barrier, thus the rate is greater.
Question
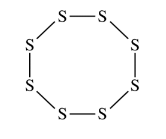
Elemental sulfur can exist as molecules with the formula \(S_8\) . The \(S_8\) molecule is represented by the incomplete Lewis diagram above.
(a) The diagram of \(S_8\) shows only bonding pairs of electrons. How many lone pairs of electrons does each S atom in the molecule have?
(b) Based on your answer to part (a), determine the expected value of the S–S–S bond angles in the S8 molecule.
(c) Write the electron configuration for the S atom in its ground state.
(d) The complete photoelectron spectrum for the element chlorine is represented below. Peak X in the spectrum corresponds to the binding energy of electrons in a certain orbital of chlorine atoms. The electrons in this orbital of chlorine have a binding energy of 273 MJ/mol, while the electrons in the same orbital of sulfur atoms have a binding energy of 239 MJ/mol.

(i) Identify the orbital and explain the difference between the binding energies in terms of Coulombic forces.
(ii) Peak Y corresponds to the electrons in certain orbitals of chlorine atoms. On the spectrum shown, carefully draw the peak that would correspond to the electrons in the same orbitals of sulfur atoms.
\(3S_{8}+8OH^{-}\rightarrow 8S_{3}^{-}+4HOOH\)
In an experiment, a student studies the kinetics of the reaction represented above and obtains the data shown in the following table.
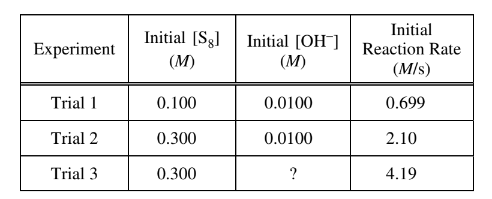
(e) Use the data in the table to do the following.
(i) Determine the order of the reaction with respect to \(S_8\) . Justify your answer.
(ii) Determine the value of\( [OH^−]\) that was used in trial 3, considering that the reaction is first order with respect to \(OH^−\). Justify your answer. The next day the student conducts trial 4 using the same concentrations of S 8 and \(OH^−\) as in trial 1, but the reaction occurs at a much slower rate than the reaction in trial 1. The student observes that the temperature in the lab is lower than it was the day before.
(f) Using particle-level reasoning, provide TWO explanations that help to account for the fact that the reaction rate is slower in trial 4.
▶️Answer/Explanation
(a) Two
(b)\(109.5^{\circ}\)
Acceptable range: \(104^{\circ}\leq angle \leq 110^{\circ}\).
(The experimentally determined angle is \(107^{\circ}8\)
(c) \(1s^{2} 2s^{2}2p^63s^2 3p^4\)
OR [Ne]\(3s^23p^4\)
(i) Peak X represents electrons in a 1s orbital. A Cl atom has one more proton in its nucleus than does a S atom; therefore, the electrons in Cl are more strongly attracted to the nucleus, and the binding energy of the 1s electrons in the Cl atom is greater than that of the 1s electrons in the S atom.
(ii) See example of a correct response (dashed peak) above.
(e)(i)
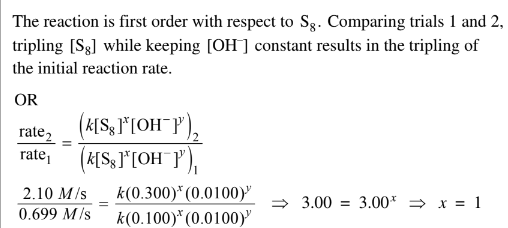
e(ii) Comparing trials 2 and 3, [S8] is kept constant and the initial reaction rate doubles. Since the reaction is first order with respect to\( OH^{-}\), the concentration of \(OH^{-}\) intrial 3 must be\( 2 \times 0.0100 M \)= 0.0200 M.
(f) The temperature was lower on the second day so the average kinetic energy of the reactant particles was lower. Therefore, there were fewer collisions between particles with sufficient
energy to react. Since the temperature was lower, the kinetic energy was lower and the average speed of the particles was lower. At the lower speeds, the reactant particles collided less frequently.
