Question
\(C_2H_6(g)+\frac{7}{2}O_2(g)→2CO_2(g)+3H_2O(l)\) ΔH°=−1560kJ
\( C(graphite)+O_2(g)→CO_2(g)\) ΔH°=−394kJ
Given the equations and the values of ΔH° for the combustion of \(C_2H_6\)(g) and the combustion of C(graphite) represented above, which of the following additional information is needed to determine the values of ΔH° for the overall reaction \(2C(graphite)+3H_2(g)→C_2H_6(g)\) ?
A \(C(diamond)→C(graphite) \) ΔH°=−2kJ
B \(C_2H_4(g)+H_2(g)→C_2H_6(g)\) ΔH°=−134kJ
C \(H_2(g)+\frac{1}{2}O2(g)→H_2O(l)\) ΔH°=−286kJ
D \(H_2O(l)→H_2O(g) \) ΔH°=+41kJ
▶️Answer/Explanation
Ans:C
Because the overall equation contains H2(g) as a reactant and the equations for the other steps do not, the missing equation must include H2(g) and should not include other substances that are not in the other equations; thus, \(H_2(g)+O_2(g)→H_2O(l)\) is the missing equation. Based on Hess’s law, ΔH° for \(2C(graphite)+2H_2(g)→C_2H_6(g)\) is equal to \((1560kJ)+2(−394kJ)+3(−286kJ)\).
Question
Reaction 1: \(2O_3(g)→3O_2(g)\)
Reaction 2: ?
Overall reaction: \(2O_3(g)+2NO(g)→2NO_2(g)+2O_2(g)\)
The enthalpy of the overall reaction represented above can be determined by adding the enthalpies of reactions 1 and 2. Which of the following could be reaction 2 ?
A \(NO(g)+O(g)→NO_2(g) \)
B \((NO)_2(g)→2NO(g)\)
C \(2NO(g)+O_2(g)→2NO_2(g)\)
D \(2NO_2(g)+O_3(g)→N_2O_5(g)+O_2(g)\)
▶️Answer/Explanation
Ans:C
Adding \(2NO(g)+O_2(g)→2NO_2(g)\) to \(2O_3(g)→3O_2(g)\) gives \(2NO(g)+O_2(g)+2O_3(g)→2NO_2(g)+3O_2(g)\). Simplifying yields the net reaction\(2O_3(g)+2NO(g)→2NO_2(g)+2O_2(g)\). Hence, the enthalpy of the net reaction can therefore be determined by adding the enthalpies of reaction 1 and 2.
Question
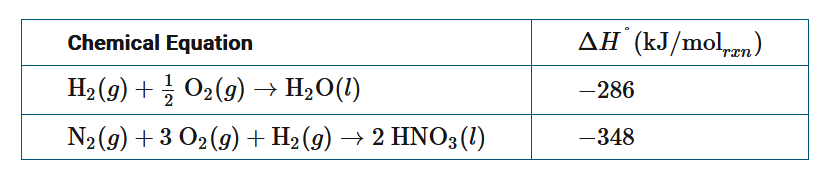
The table above provides the values for the standard enthalpy change for two chemical reactions. A student needs to calculate the enthalpy change for the reaction \(2HNO_3(l)→N_2O_5(g)+H_2O(l)\) under standard conditions using Hess’s law. In addition to the values given in the table, what other information will the student need to perform the calculation?
A \(\Delta H^0_f\) for \(N_2(l)\)
B \(\Delta H^0_f\) for \(N_2O_5(g)\)
C \(\Delta H^0_f\) for \(H_2O(g)\)
D \(\Delta H^0_f\) for \(H_2(l)\)
▶️Answer/Explanation
Ans:B
Inverting the second equation in the table, adding it to the first, and simplifying gives: \(2HNO_3(l)→N_2(g)+\frac{5}{2}O_2(g)+H_2O(l)\). Since the standard enthalpy of formation of \(N_2O_5(g)\) is the enthalpy change for the production of 1 mole of the substance from its elements in their standard states, this equation is \(N_2(g)+\frac{5}{2}O_2(g)→N_2O_5(g)\). Adding these two equations yields the chemical equation for the target reaction and allows the enthalpy change to be determined by adding \(−286+[−(−348)]+\Delta H^0_f(N_2O_5)\).
