Question.
\(H_{3}BO_{3}(aq)+4HF(g)\rightarrow H_{3}O^{+}(aq)+BF_{4}^{-}(aq)+2H_{2}O(l)\)
Tetrafluoroboric acid is a strong acid with the formula\( HBF_4\). The acid can be prepared by reacting the weak acid \(H_3BO_3\) (molar mass 61.83 g/mol) with HF according to the equation above.
(a) To prepare a solution of \(BF_4^{-}\)(aq), HF(g) is bubbled into a solution containing 50.0 g of\( H_3BO_3\) in a 1 L reaction vessel.
(i) Calculate the maximum number of moles of\( BF_4^{-}\)(aq) that can be produced.
(ii) Calculate the number of liters of HF(g), measured at 273 K and 1.00 atm, that will be consumed if all the\( H_3BO_3\) reacts.
(iii) Will the pH of the solution increase, decrease, or remain the same during the course of the reaction? Justify your answer. In another experiment, a 0.150 M \(BF_4^-\)(aq) solution is prepared by dissolving \(NaBF_4\)(s) in distilled water. The\( BF_{4}^-\)(aq) ions in the solution slowly react with H2O(l) in the reversible reaction represented below.
\(BF_4^-(aq)+H_{2}O(l)\rightleftharpoons BF_{3}OH^{-}(aq)+HF(aq)\)
The concentration of HF is monitored over time, as shown in the graph below.

[HF] reaches a constant value of 0.0174 M when the reaction reaches equilibrium. For the forward reaction, the rate law is rate = kf \([BF_4^{-}\)]. The value of the rate constant kf was experimentally determined to be \(9.00\times 10^{-4}min^{-1}\).
(b) Calculate the rate of the forward reaction after 600. minutes. Include units with your answer. The rate law for the reverse reaction is rate = \(kr [BF_3OH^-]\)[HF].
(c) A student claims that the initial rate of the reverse reaction is equal to zero. Do you agree or disagree with this claim? Justify your answer in terms of the rate law for the reverse reaction.
(d) At equilibrium the forward and reverse reaction rates are equal. Calculate the value of the rate constant for the reverse reaction.
\(H_{3}BO_{3}(aq)+4HF(g)\rightarrow H_{3}O^{+}(aq)+BF_{4}^{-}(aq)+2H_{2}O(l)\)
Tetrafluoroboric acid is a strong acid with the formula \(HBF_4\). The acid can be prepared by reacting the weak acid \(H_3BO_3\) (molar mass 61.83 g/mol) with HF according to the equation above.
▶️Answer/Explanation
a(i) \(50.0gH_{3}BO_{3}\times \frac{1molH_{3}BO_{3}}{61.83g}\times \frac{1molBF_{4}^{-}}{1molH_{3}BO_{3}}\)
a(ii)\( 0.809 mol \times 4 = 3.24 mol\)
PV= nRT
\(V=\frac{nRT}{p}=\frac{(3.24mol)(0.08206Latm mol^{-1}K^{-1})(273K)}{1.00atm}\)
=72.6L
OR
\(0.809 mol \times 4 = 3.24 mol\)
\(\frac{22.4L}{1mol}\times 3.24mol=72.6 L\)
a(iii) As the reaction proceeds,\( H_3O^{+}\) is produced, so the pH
will decrease.
(b) \([BF_4^{-}] = 0.150 M – 0.0174 M = 0.133 M\)
\(rate = (9.00\times 10^{-4}min^{-1})(0.133M)=1.20\times 10^{-4}M min^{-1}\)
(c) Agree. The initial concentration of each product is zero, so the initial rate of the reverse reaction is zero.
(d ) 
Question
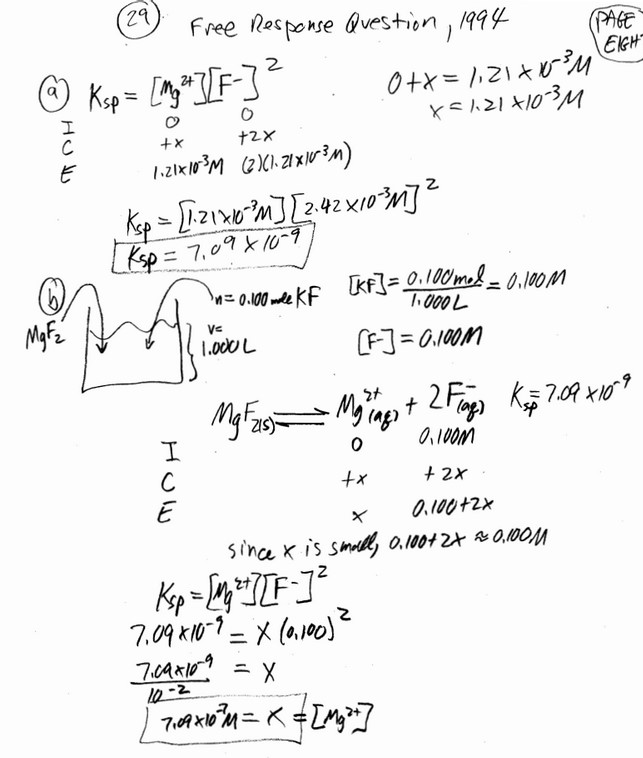
\(MgF_2(s) \Leftrightarrow Mg^{2+}(aq) + 2 F^{ -}(aq)\)
In a saturated solution of \(MgF_2\) at 18° C, the concentration of Mg2+ is \(1.21 x 10^ {-3}\) molar. The equilibrium is represented by the equation above.
(a) Write the expression for the solubility-product constant, \(K_{sp}\), and calculate its value at 18° C.
(b) Calculate the equilibrium concentration of \(Mg^{2+}\) in 1.000 liter of saturated \(MgF_2\) solution at 18°C to which 0.100 mole of solid KF has been added. The KF dissolves completely. Assume the volume change is negligible.
(c) Predict whether a precipitate of \(MgF_2\) will form when 100.0 milliliters of a \(3.00 x 10 ^{-3}\) molar \(Mg(NO_3)_2\) solution is mixed with 200.0 milliliters of a \(2.00 x 10 ^{-3}\) molar NaF solution at 18°C.
Calculations to support your prediction must be shown.
(d) At 27°C the concentration of \(Mg^{2+}\) in a saturated solution of \(MgF_2\) is \(1.17 x 10 ^{-3}\) molar. Is the dissolving of \(MgF_2\) in water an endothermic or an exothermic process? Give an explanation to support
your conclusion.
▶️Answer/Explanation
Answer: