Question
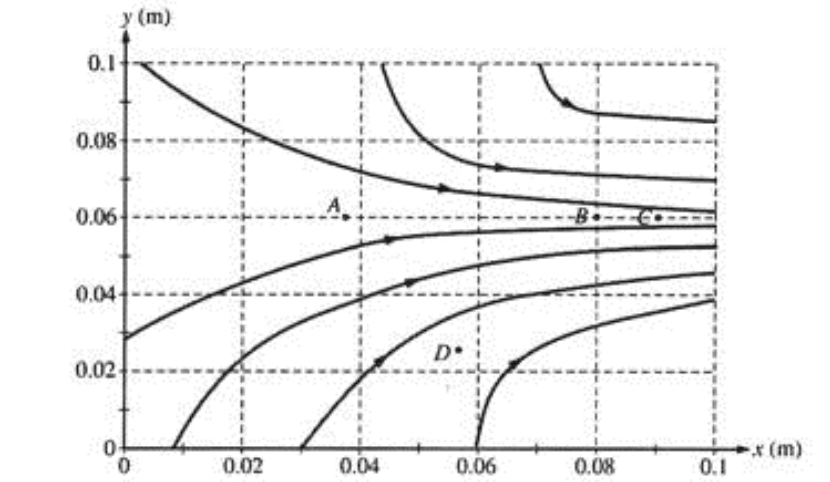
Consider the electric field diagram above.
a. Points A, B, and C are all located at y = 0.06 m .
i. At which of these three points is the magnitude of the electric field the greatest? Justify your answer.
ii. At which of these three points is the electric potential the greatest? Justify your answer.
b. An electron is released from rest at point B.
i. Qualitatively describe the electron’s motion in terms of direction, speed, and acceleration.
ii. Calculate the electron’s speed after it has moved through a potential difference of 10 V.
c. Points B and C are separated by a potential difference of 20 V. Estimate the magnitude of the electric field midway between them and state any assumptions that you make.
d. On the diagram, draw an equipotential line that passes through point D and intersects at least three electric field lines.
▶️Answer/Explanation
Ans:
a. i. The magnitude of the field is greatest at point C because this is where the field lines are closest together.
ii. The potential is greatest at point A. Electric field lines point from high to low potential.
b. i. The electron moves to the left, against the field lines. As the field gets weaker the electron’s acceleration to the left decreases in magnitude, all the while gaining speed to the left.
ii. W = qΔV = ½ mv2 gives v = 1.9 × 106 m/s
c. If we assume the field is nearly uniform between B and C we can use E = ΔV/d where the distance between B and C d = 0.01 m giving E = 20 V/0.01 m = 2000 V/m
d. 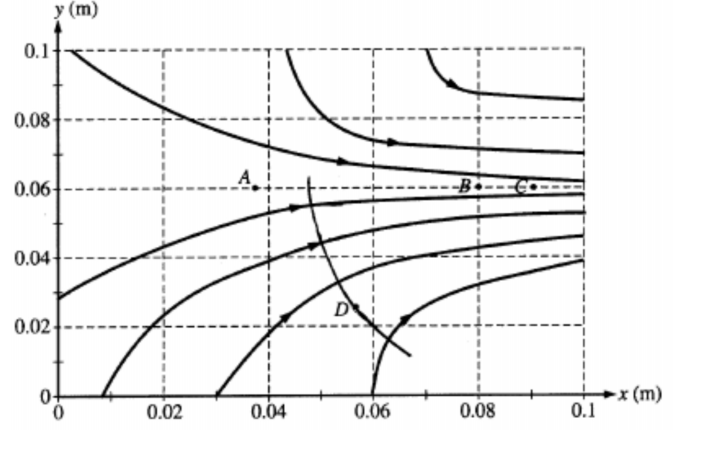
Question
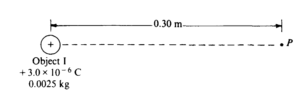
Object I, shown above, has a charge of + 3 x 10–6 coulomb and a mass of 0.0025 kilogram.
a. What is the electric potential at point P, 0.30 meter from object I?
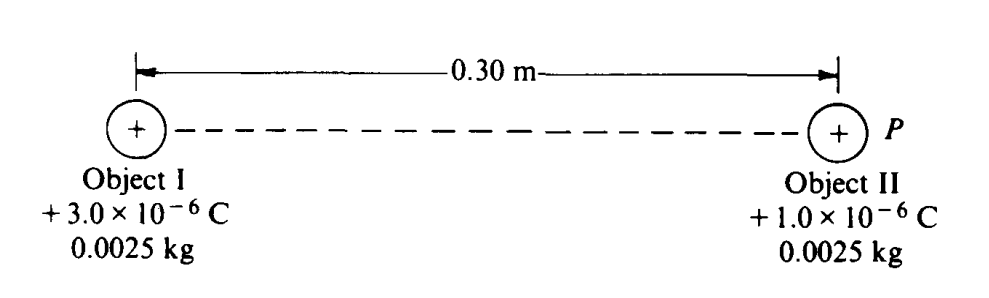
Object II, of the same mass as object I, but having a charge of + 1 x 10–6 coulomb, is brought from infinity to point P, as shown above.
b. How much work must be done to bring the object II from infinity to point P?
c. What is the magnitude of the electric force between the two objects when they are 0.30 meter apart?
d. What are the magnitude and direction of the electric field at the point midway between the two objects?
The two objects are then released simultaneously and move apart due to the electric force between them. No other forces act on the objects.
e. What is the speed of object I when the objects are very far apart?
▶️Answer/Explanation
Ans:
a. V = kQ/r = 9 × 104 V
b. W = qΔV (where V at infinity is zero) = 0.09 J
c. F = kqQ/r2 = 0.3 N
d. Between the two charges, the fields from each charge point in opposite directions, making the resultant field the difference between the magnitudes of the individual fields.
E = kQ/r2 gives EI = 1.2 × 106 N/C to the right and EII = 0.4 × 106 N/C to the left
The resultant field is therefore E = EI – EII = 8 × 105 N/C to the right
e. From conservation of momentum mIvI = mIIvII and since the masses are equal we have vI = vII. Conservation of energy gives U = K = 2(½ mv2) = 0.09 J giving v = 6 m
Question: (12 points, suggested time 25 minutes)
Some students want to know what gets used up in an incandescent lightbulb when it is in series with a resistor:
current, energy, or both. They come up with the following two questions.
(1) In one second, do fewer electrons leave the bulb than enter the bulb?
(2) Does the electric potential energy of electrons change while inside the bulb?
The students have an adjustable power source, insulated wire, lightbulbs, resistors, switches, voltmeters, ammeters, and other standard lab equipment. Assume that the power supply and voltmeters are marked in 0.1 V increments and the ammeters are marked in 0.01 A increments.
(a) Describe an experimental procedure that could be used to answer questions (1) and (2) above. In your description, state the measurements you would make and how you would use the equipment to make them. Include a neat, labeled diagram of your setup.
(b)
i. Explain how data from the experiment you described can be used to answer question (1) above.
ii. Explain how data from the experiment you described can be used to answer question (2) above.
A lightbulb is nonohmic if its resistance changes as a function of current. Your setup from part (a) is to be used or modified to determine whether the lightbulb is nonohmic.
(c)
i. How, if at all, does the setup need to be modified?
ii. What additional data, if any, would need to be collected?
(d) How would you analyze the data to determine whether the bulb is nonohmic? Include a discussion of how the uncertainties in the voltmeters and ammeters would affect your argument for concluding whether the resistor is nonohmic.
▶️Answer/Explanation
Ans:
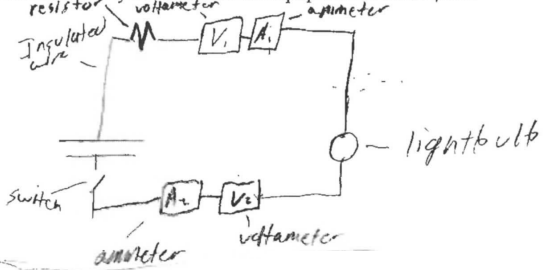
To answer questions 1 and 2, the students would use the voltameters and ammeters to measure the current and voltage of the circuit both before and after the lightbulb for 3 trials of 1 second. The student would record this information for calculations.
(b) i. The data from the experiment above can be used to answer question (i) by comparing the current in the circuit at the ammeter before and the ammeter after the light bulb. If a discrepancy between the two measurements in earn trial is found, fever electrons may have left the light bulb.
ii. The data from the experiment above can be used to answer question (2) by comparison the voltage measured by the voltmeters placed before and after the light bulb. If a discrepancy between the values from each voltmeter in a given trial is found, the electrical potential energy of the electrons may have changed which inside the bulb.
(c) i. The voltage of the adjustable power source must be varied for a given number of trials to create varying currents because current = \(\frac{voltage}{resistance}= I = \frac{V}{R}\)
ii. Additional data reavired would be a number of trials at given voltages from the adjustable power source. This would allow different data sets of voltage and current to be used to determinse the resistance of the bulb.
(d) To determine whether the bulb is nonohmic, I would determine the resistances of the lightbulb in each trial according to the formula \(I = \frac{\Delta V}{R}\) assuming that in series no current is lost in the lightbulb (or circuit for that matter). If the resistance of the lightbulb is found to vary, it may be assumed to be nonohmic assuming the uncertainties in the voltmeters and ammeters did not affect the resorts. Because the uncertainties exist, hoever, If the change in voltage is less than or eacal to. IV, The conclusion as to whether or not the lightbulb is nonohmic cannot be verified using the given ealipment.
Question

A scientist constructs a device shown in the figure. Region I consists of a charged parallel plate capacitor with vertical plates separated by a distance x. The left plate has a small opening that accelerated particles can pass through. Region II has two large horizontal capacitor plates with a separation of y and a magnetic field created by current-carrying solenoid coils, which are not pictured in the figure. The magnetic field is directed upward out of the page. In an experiment a single proton P is placed at the launch point near the right plate in Region I. The proton accelerates to the left through the hole and continues on a straight path through Region II as seen in the figure.
(A) What is the direction of the electric field in Region I? Justify your answer.
(B) Which capacitor plate has the higher potential in Region II? Justify your answer.
(C) The experiment is repeated, replacing the proton with an alpha particle that has a mass approximately four times larger than the proton and a charge two times larger than the proton. The alpha particle is released from the launch point and passes through the hole in the left plate. Compare the motion of the alpha particle to the motion of the proton through Regions I and II. Justify your reason mg. The Region I capacitor plates have a potential difference of 5400 V and a plate separation x of 0.14 m. The Region II capacitor plates have a separation y of 0.060 m and a magnetic field of 0.50 T.
(D) A proton is again placed at the launch point near the right plate in Region I. Derive an algebraic expression for the velocity of the proton as it passes through the hole in the left plate. Use your expression to calculate the numerical value of the velocity.
(E) Using your work from (D), derive an algebraic expression for the potential difference that must be applied to the capacitor in Region II so that the proton 1noves in a straight line through the region. Use your expression to calculate the numerical value of the potential difference.
(F) Keeping the potential difference the same as the calculated value from (E), the scientist places an unknown particle at the launch point and observes that it travels straight through both Regions I and II just as the proton did. Discuss what the scientist can deduce about the unknown particle. Justify your answer, making appropriate reference to the algebraic expressions derived in (D) and (E).
▶️Answer/Explanation
Ans:
Part (A)
Electric field is to the left. The electric field points in the direction of force on a positive charge. The proton accelerates to the left; therefore, the force is to the left and the electric field is also to the left.
Part (B)
The upper plate must have a higher potential. Utilizing the right-hand rule for forces on moving positive charges, and showing that the proton receives a magnetic force upward from the magnetic field. The electric force is equal and opposite in direction to the magnetic force so that the proton will travel in a straight line. Electric fields point from higher potential to lower potential. Thus, the upper plate is a higher potential.
Part (C)
The alpha particle will curve downward in Region II. The alpha particle will exit Region I with a slower velocity. The electric potential energy has doubled, but the mass has quadrupled leading to a smaller exit velocity. (Or, in Region I the electric force on the charge has doubled, but the mass has quadrupled, leading to a smaller acceleration and exit velocity.) The electric force will now be larger than the magnetic force causing the alpha particle to arc downward. The electric force will double as the change doubles: \(B_{E}=q_{E}\). The magnetic force will not increase as much as the electric force. Even though the charge doubles, the velocity is now slower: \(P_{M}=qvB\).
Part (D)
Conservation of energy: \(AR_{E}=K\)
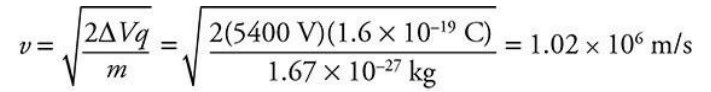
Part (E)
To move in a straight line: \(B_{E}=P_{M}\)
Magnetic force equal to electric force: Eq=qvB
\(E=\frac{\Delta V}{y}=vB\)
Equations with substitutions:
AK= yvB — (0.060)(1.02 x \(10^{6}\) m/s)(0.50 T)
AK= 30,600 V
Part (F)
From the equation derived in (E) AK= yvB, we know that the velocity of the particle is the same because AK, y, and B are all the same.
From the equation in (D) \(v=\sqrt{\frac{2\Delta Vq}{m}}\), we know that both v and AT are the same. Thus, we can deduce the charge to mass ratio q/m of the unknown particle.
