Question

Two charges, \(q_{1}\) = +3 nC and \(q_{2}\) = –12 nC, are fixed in place and separated by a distance of 30 cm.
(a) What is the magnitude of the electric force between the two charges? Is the force attractive or repulsive?
(b) At what point between the two charges is the electric field equal to 0?
(c) What is the electric field at the midpoint between the two charges?
(d) What is the magnitude of the electric force on a charge \(q_{3}\) = +2 nC placed in the middle of the two charges?
▶️Answer/Explanation
Ans:
(a) The magnitude of the electric force is given by the following equation:
\(F_{E}=\frac{1}{4\pi \varepsilon _{0}}\frac{q_{1}q_{2}}{r^{2}}=(9\times 10^{9}N\cdot \frac{m^{2}}{C^{2}})\frac{(3\times 10^{-9}nC)(-12\times 10^{-9nC})}{(0.3m)^{2}}=-3.6\times 10^{-6}N\)
The electric force between two opposite charges is attractive
(b) The electric field is given by the equation \(E=\frac{1}{4\pi \varepsilon _{0}}\frac{Q}{r^{2}}\). As there are two charges present, the electric field at any point is equal to the sum of the individual electric fields produced by each charge. As you are looking for the point where E = 0, set the total electric field equal to 0 and solve:
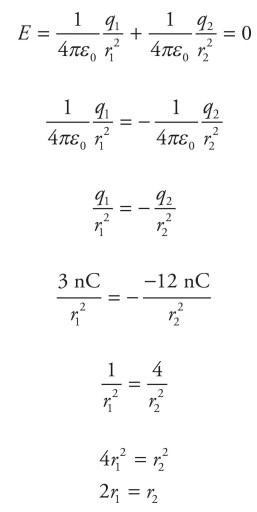
The electric field will be equal to 0 when \(2r_{1}=r_{2}\). As you are looking for a point between the two charges, it is also true that \(r_{1}+r_{2}=0.3m\)
Substituting in for \(r_{2}\), you get
\(r_{1}+2r_{1}=0.3m\Rightarrow 3r_{1}=0.3m\Rightarrow r_{1}=0.1m\)
\(r_{2}=2r_{1}=0.2m\)
The electric field is equal to 0 at the point 0.1 m from the \(q_{1}\) and 0.2 m from \(q_{1}\) between the two charges.
(c) At the midpoint between the two charges, \(r_{2}\) = \(r_{1}\) = 0.15 m. The electric field at the point right between the two charges is equal to
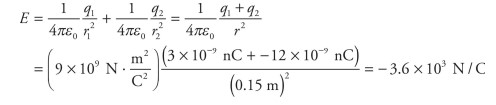
As electric fields point away from positive charges and toward negative charges, the direction of the electric field is toward \(q_{2}\).
(d) Given the electric field at a point, the electric force on a charge at that point is given by
F = qE = (2 × \(10^{-9}\) nC)(–3.6 × \(10^{3}\) N/C) = –7.2 × \(10^{-6}\) N
Question
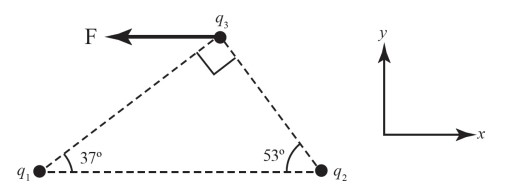
Three particles are fixed in place in a horizontal plane, as shown in the figure above. Particle 1 has a charge that has a magnitude of 4.0 ´ \(10^{-6}\) C and the sign of the charge is unknown. Particle 2 has a charge that has a magnitude of 1.7 ´ \(10^{-6}\) C and the sign of the charge is unknown. Particle 3 has a charge of +1.0 ´ \(10^{-6}\) C. The distance between \(q_{1}\) and \(q_{2}\) is 5.0 m, the distance between \(q_{2}\) and \(q_{3}\) is 3.0 m, and the distance between \(q_{1}\) and \(q_{3}\) is 4.0 m. The electrostatic force F on particle 3 due to the other two charges is shown in the negative x-direction.
(a) Determine the signs of the charges of \(q_{1}\) and \(q_{2}\) .
(b) On the diagram below, draw and label the force \(F_{1}\) of the force exerted by Particle 1 on Particle 3 and the force \(F_{2}\) of the force exerted by Particle 2 on Particle 3.
(c) Calculate the magnitude of the electrostatic force on Particle 3.
(d) Draw and label clearly where another positively charged particle could be placed so the net electrostatic force on Particle 3 is zero.

▶️Answer/Explanation
Ans:
(a) Because the force vector points to the left, the only logical solution is if \(q_{1}\) is negative and \(q_{2}\) is positive.
(b) 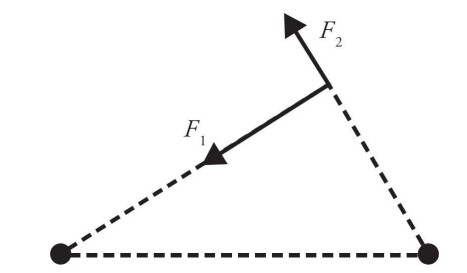
(c) Applying Coulomb’s Law to determine \(F_{1}\) and \(F_{2}\),
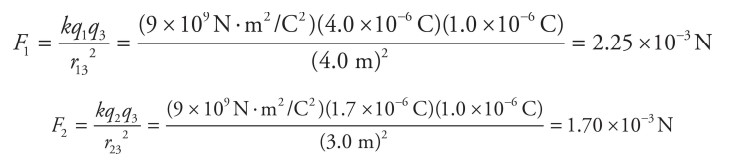
F is the vector sum of the two forces, \(F\) = \(F_{1}\) + \(F_{2}\). Since they are at right angles to each other, the magnitude can be found by applying the Pythagorean Theorem

(d) 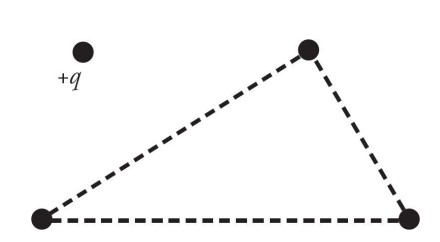
From the setup, you know that the current net force is entirely to the left. Therefore, you need to cancel this out with a force that is entirely to the right. The question specifies that you must use a positively charged particle to do this, and positive charges will create repulsive forces in this case because \(q_{3}\) is itself positive. So if you’re trying to repel an object and make it move right, you need to be to that object’s left. Without additional numerical information in the question, it’s impossible to determine exactly how far from \(q_{3}\) this charge should be placed, but it must be somewhere along the line that extends directly to the left of \(q_{3}\).
Question

In the above diagram, two small objects, each with a charge of –4.0 nC, are held together by a 0.020 m length of insulating string. The objects are initially at rest on a horizontal, non-conducting, frictionless surface. The effects of gravity on each other can be considered negligible.
(a) Calculate the tension in the string.
(b) Illustrate the electric field by drawing electric field lines for the two objects on the following diagram.
![]()
The masses of the objects are \(m_{1}\) = 0.030 kg and \(m_{2}\) = 0.060 kg. The string is now cut.
(c) Calculate the magnitude of the initial acceleration of each object.
(d) On the axes below, sketch a graph of the acceleration a of the object of mass m2 versus the distance d between the objects after the string has been cut.

(e) In a brief paragraph, describe the speed of the objects as time increases, assuming that the objects remain on the horizontal, non-conducting frictionless surface.
▶️Answer/Explanation
Ans:
(a) Applying Newton’s Second Law to one of the objects

(b) 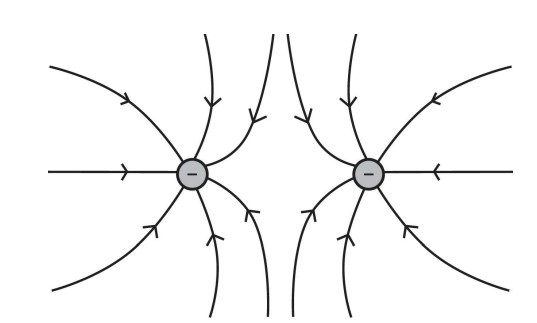
(c) The acceleration is caused by the electrostatic force, and initially that force has the same magnitude as the one calculated in part (A).
\(F_{net}=F_{E}=T\)
\(ma=T\)
Now calculate the acceleration on each object,
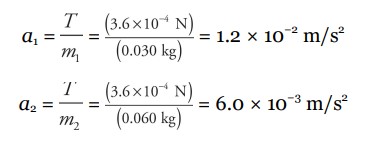
(d) 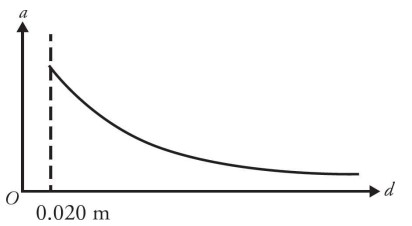
(e) As time increases, the speed of the objects increase. The objects move further apart, but their speeds increase at a slower rate. This is because the electrostatic force decreases as the objects move further apart. Hence, the acceleration also decreases. The speed approaches a constant value as the distance between the objects, d, approaches infinity.
