Question
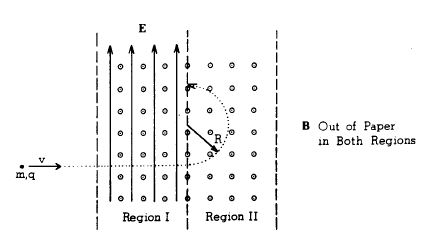
An ion of mass m and charge of known magnitude q is observed to move in a straight line through a region of space in which a uniform magnetic field B points out of the paper and a uniform
electric field E points toward the top edge of the paper, as shown in region I above. The particle travels into region II in which the same magnetic field is present, but the electric field is zero. In region II the ion moves in a circular path of radius R as shown.
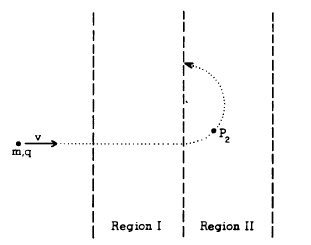
a. Indicate on the diagram below the direction of the force on the ion at point P2, in region II.
b. Is the ion positively or negatively charged? Explain clearly the reasoning on which you base your
conclusion.
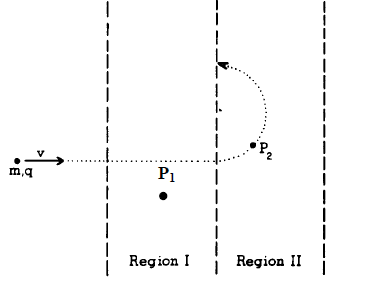
c. Indicate and label on the diagram below the forces which act on the ion at point P1 in region I.
d. Find an expression for the ion’s speed v at point P1 in terms of E and B.
e. Starting with Newton’s law, derive an expression for the mass m of the ion in terms of B, E, q, and R.
Answer/Explanation
Ans.
a. In order to move in a uniform circular path, the force must be directed toward the center of the circle.
b. Because the particle experiences an upward magnetic force while traveling to the right in a magnetic field
pointing out of the page, it is the LHR that provides the correct direction, which indicates a negative charge.
c. The magnetic force acts up and the electric force acts down
d. As there is no acceleration in region I, the net force must be zero, so the magnetic force is equal to the electric
force: qvB = qE, or v = E/B
e. In the circular region F = mv2/r = qvB with v = E/B, giving m = B2qR/E
Question
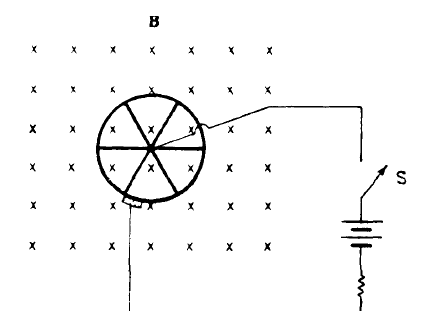
A wheel with six spokes is positioned perpendicular to a uniform magnetic field B of magnitude 0.5 tesla (weber per square meter). The field is directed into the plane of the paper and is present over the entire region of the wheel as shown above. When the switch S is closed, there is an initial current of 6 amperes between the axle and the rim, and the wheel begins to rotate. The resistance of the spokes and the rim may be neglected.
a. What is the direction of rotation of the wheel? Explain.
b. The radius of the wheel is 0.2 meters. Calculate the initial torque on the wheel.
Answer/Explanation
Ans.
a. Counterclockwise. The current flows radially outward, use RHR (force)
b. τ = rF for each spoke and let r = center of mass of each spoke:
Nr(BIL) = (6)(0.1 m)(0.5 T)(6 A)(0.2 m) = 0.06 N-m
Question
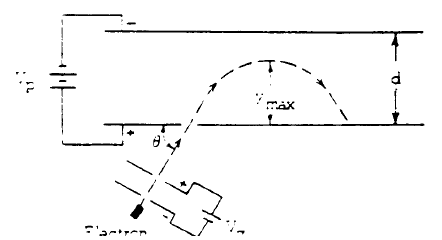
Electrons are accelerated from rest in an electron gun between two plates that have a voltage Vg across them. The electrons then move into the region between two other parallel plates of separation d that have voltage Vp across them. The electrons are projected into this region at an angle θ to the plates as shown above. Assume that the entire apparatus is in vacuum and that Vp > Vg. Display all results in terms that include d, Vg, Vp, θ, e (the magnitude of the electron charge), and mo (the electron mass).
a. Develop an equation for the speed ve with which the electrons leave the electron gun.
b. Develop an equation for the maximum distance ymax that the electrons travel above the lower plate.
Suppose that a magnetic field directed into the plane of the paper is introduced in the region between
the upper plates
c. How will the speed with which the electrons strike the lower plate be affected? Explain.
d. Sketch on the diagram a trajectory that an electron might follow with the magnetic field present.
Account qualitatively for the difference between the new and old trajectory.
Answer/Explanation
Ans.
a. ΔU = ΔK
qVg = ½ mve2 giving ve = (2eVg/m)1/2
b. Total energy upon entering = total energy at ymax
½ mve2 = ½ mve2cos2θ + e(VP/d)ymax where the second term is from W = qEd
thus ymax = d(Vg/VP)sin2θ
c. The speed at impact is unchanged, the magnetic force is always 90º to the velocity and thus does no work
d.