Question
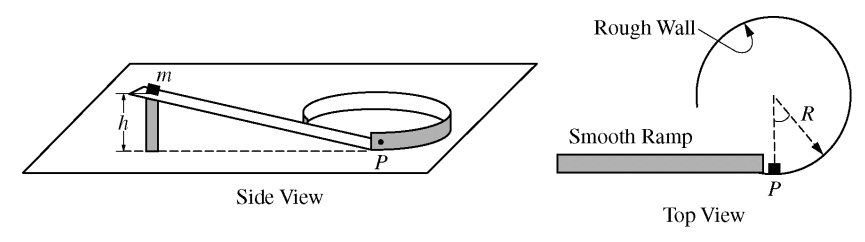
A small block of mass m starts from rest at the top of a frictionless ramp, which is at a height h above a horizontal tabletop, as shown in the side view above. The block slides down the smooth ramp and reaches point P with a speed v0 . After the block reaches point P at the bottom of the ramp, it slides on the tabletop guided by a circular vertical wall with radius R, as shown in the top view. The tabletop has negligible friction, and the coefficient of kinetic friction between the block and the circular wall is μ .
(a) Derive an expression for the height of the ramp h. Express your answer in terms of v0 , m, and fundamental constants, as appropriate.
A short time after passing point P, the block is in contact with the wall and moves with a speed of v .
(b)
i. Is the vertical component of the net force on the block upward, downward, or zero?
____ Upward ____ Downward ____ Zero
Justify your answer.
ii. On the figure below, draw an arrow starting on the block to indicate the direction of the horizontal component of the net force on the moving block when it is at the position shown.
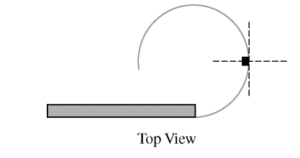
Justify your answer.
Express your answers to the following in terms of v0 , u , m, R, m , and fundamental constants, as appropriate.
(c) Determine an expression for the magnitude of the normal force N exerted on the block by the circular wall as a function of v .
(d) Derive an expression for the magnitude of the tangential acceleration of the block at the instant the block has attained a speed of v .
(e) Derive an expression for v(t) , the speed of the block as a function of time t after passing point P on the track.
Answer/Explanation
Ans:
(a)
WNC = ΔA + Δv
0 = \(\frac{1}{2}mv^{2}-mgh\)
\(\frac{1}{2}{mv_{0}}^{2}= mgh\)
\(\therefore h = \frac{{v_{0}}^{2}}{2g}\)
(b) i.
√ Zero
The block shows centripetal acceleration, but ∑F1 = 0 because it does not accelerate up or down. Thus, ms – N = 0.
ii.
The block is in circular motion about the center of the loop. Thus, it must accelerate toward the center of the loop. Furthermore, friction with the loop’s wall provides a regarding force (tangible acceleration). These 2 forces together provide the vector shown above.
(c) \(F_{c} = \frac{mv^{2}}{r}\rightarrow \therefore N = \frac{mv^{2}}{R}\)
(d)
\(\sum \overrightarrow{F}= ma\)
-μN = ma
\(-\mu \left ( \frac{mv^{2}}{R} \right )=ma\)
\(\therefore a = -\frac{\mu v^{2}}{R}\)
(e)
\(\frac{dv}{dt}= – \frac{\mu v^{2}}{R}\)
\(\int \frac{dv}{v^{2}}= \int -\frac{\mu }{R}dt\)
\(-\frac{1}{v}= -\frac{\mu t}{R}+C_{2}\)
When t = 0, v = v0
\(\therefore -\frac{1}{v_{0}}= 0 + c_{1}\)
\(\therefore c_{1}= -\frac{1}{v_{0}}\)
\(\therefore \frac{1}{v}= \frac{\mu t}{R}+\frac{1}{v_{0}}\)
\(\frac{1}{v}= \frac{\mu tv_{0}+R}{Rv_{0}}\)
\(\therefore v(t)= \frac{Rv_{0}}{\mu v_{0}t+R}\)
Question
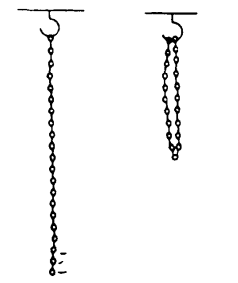
A uniform chain of mass M and length l hangs from a hook in the ceiling. The bottom link is now raised vertically and hung on the hook as shown above on the right.
a. Determine the increase in gravitational potential energy of the chain by considering the change in position of the center of mass of the chain.
b. Write an equation for the upward external force F(y) required to lift the chain slowly as a function of the vertical distance y.
c. Find the work done on the chain by direct integration of ∫ 𝐹𝑑𝑦.
Answer/Explanation
Ans:
a. using the ceiling as a reference point: Ui = – ½ mgl; Uf = – ¼ mgl
∆U = Uf – Ui = ¼ mgl
b.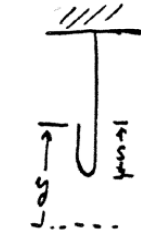 
λ = m/l, the weight of the piece being held is λsg = mgs/l; s = y/2 so F(y) = mgy/2l
c. \(\int_{0}^{l}Fdy = \frac{mg}{2l}\int_{0}^{l}ydy = \frac{mg}{2l}\frac{l^{2}}{2}= \frac{1}{4}mgl\)
Question
A car of mass M moves with an initial speed vo on a straight horizontal road. The car is brought to rest by braking in such a way that the speed of the car is given as a function of time t by v = (vo² – Rt/M)½ where R is a constant.
a. Develop an equation that expresses the time rate of change of kinetic energy.
Answer/Explanation
Ans:
a. K = ½ Mv2 = ½ M(v02 –Rt/M)
(the rest of this question is in the dynamics chapter)