Question
An object is stretched until it reaches the elastic limit.
Which statement must describe the stress on the object when it is at the elastic limit?
A It is the maximum stress for which the object obeys Hooke’s law.
B It is the maximum stress that can be applied to the object before it has elastic deformation.
C It is the maximum stress that can be applied to the object before it has plastic deformation.
D It is the maximum stress the object can withstand before it breaks.
Answer/Explanation
Ans:
Question
Two identical springs are connected in parallel. A weight of 8.0 N is hung from the combination, as shown.
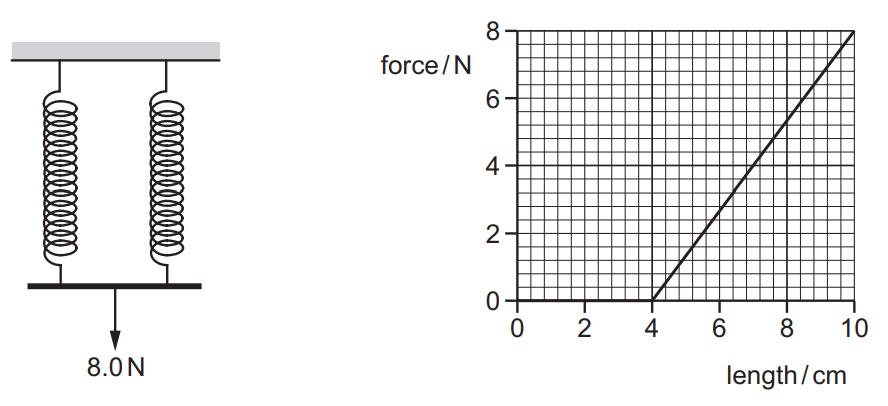
The graph shows the variation with length of the force applied to one of the springs. What is the strain energy in one of the springs?
A 0.060J B 0.12 J C 0.14 J D 0.24 J
Answer/Explanation
Ans :
Question
Forces are applied to the ends of a rod so that its length increases. The variation with force F of
the extension e of the rod is shown.

The point P is the elastic limit.
Which shaded area represents the work done during the plastic deformation of the rod?
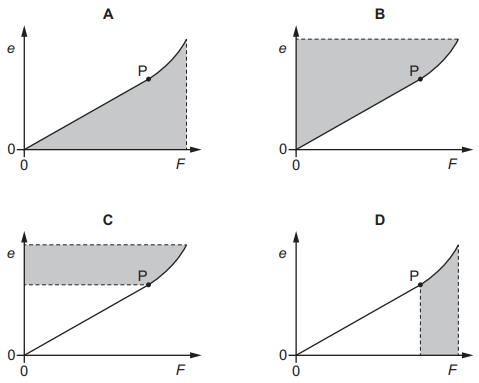
Answer/Explanation
Ans:
Question
An unstretched rubber cord is stretched by a force. The force F is plotted against the extension x.
F is slowly increased from zero, causing the cord to extend along path P. F is then reduced back
to zero along path Q.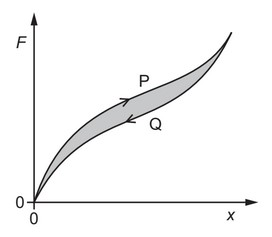
What is represented by the shaded area?
A the elastic energy stored in the rubber cord
B the energy that causes plastic deformation
C the energy dissipated as heat
D the work done to extend the rubber cord
Answer/Explanation
Question
A wire is stretched by applying increasing values of force F. For each value of force applied, the
extension x is recorded. A force–extension graph is plotted from the data obtained.
Which statement about the area under the graph must be correct?
A It can be calculated as ½ Fx .
B It is the elastic potential energy stored in the stretched sample.
C It is the work done in stretching the sample.
D It would be the same for any wire of the same material.
Answer/Explanation
Ans
