Question
(a) By reference to the direction of transfer of energy, state what is meant by a longitudinal wave. [1]
(b) A vehicle travels at constant speed around a wide circular track. It continuously sounds its
horn, which emits a single note of frequency 1.2kHz. An observer is a large distance away
from the track, as shown in the view from above in Fig. 4.1.

Fig. 4.2 shows the variation with time of the frequency f of the sound of the horn that is
detected by the observer. The time taken for the vehicle to travel once around the track is T.
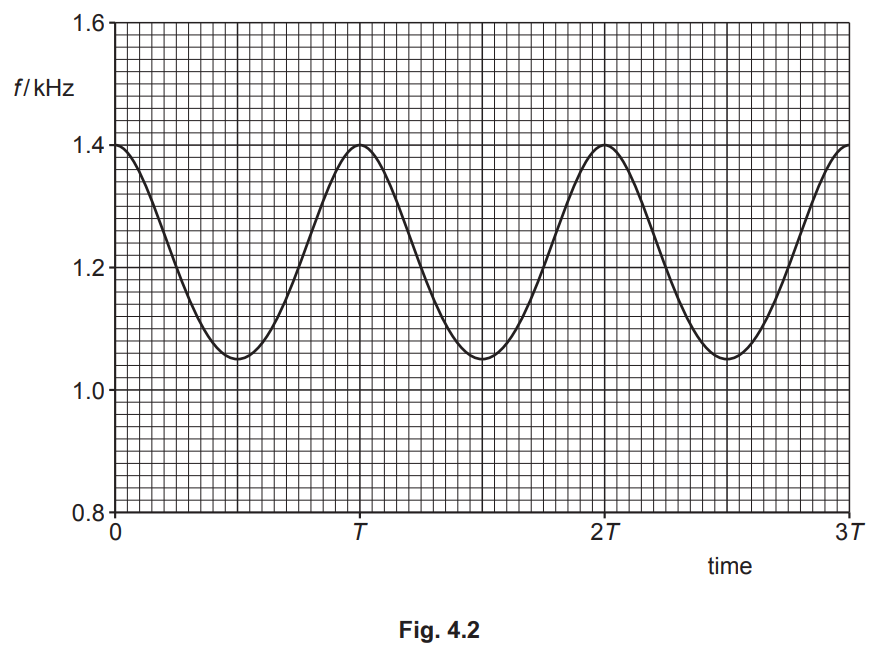
(i) Explain why the frequency of the sound detected by the observer is sometimes above
and sometimes below 1.2kHz. [2]
(ii) State the name of the phenomenon in (b)(i). [1]
(iii) On Fig. 4.1, mark with a letter X the position of the vehicle when it emitted the sound that
is detected at time T. [1]
(iv) On Fig. 4.1, mark with a letter Y the position of the vehicle when it emitted the sound that
is detected at time \( \frac{9T}{4}\) [1]
(c) The speed of the sound in the air is 320ms–1.
Use Fig. 4.2 to determine the speed of the vehicle in (b).
speed = ………………………………………… ms–1 [3]
[Total: 9]
Answer/Explanation
Ans
(a) oscillations (of particles) are parallel to (the direction of) energy transfer
(b) (i) (frequency varies as) vehicle moves relative to (stationary) observer
(vehicle) moving towards (observer) gives higher (observed) frequency (than 1.2 kHz) and (vehicle) moving away (from
observer) gives lower (observed) frequency (than 1.2 kHz)
(b) (ii) Doppler effect
(b) (iii) position of vehicle labelled ‘X’ at top (12 o’clock) position on track
(b) (iv) position of vehicle labelled ‘Y’ at right-hand edge (3 o’clock) position on track B1
(c) maximum frequency = 1.40 (kHz) or 1.40 × 103 (Hz)
1.40 = (1.2 × 320) / (320 –v)
v = 46ms–1
or
minimum frequency = 1.05 (kHz) or 1.05 × 103 (Hz)
1.05 = (1.2 × 320) / (320 + v)
v = 46ms–1
Question
(a) (i) By reference to the direction of propagation of energy, state what is meant by a longitudinal wave.
(ii) State the principle of superposition.
(b) The wavelength of light from a laser is determined using the apparatus shown in Fig. 4.1.
The light from the laser is incident normally on the plane of the double slit. The separation of the two slits is \(3.7×10^{–4}\)m. The screen is parallel to the plane of the double slit. The distance between the screen and the double slit is 2.3m. A pattern of bright fringes and dark fringes is seen on the screen. The separation of adjacent bright fringes on the screen is \(4.3×10^{–3}\)m.
(i) Calculate the wavelength, in nm, of the light.
wavelength = …………………………………………… nm
(ii) The intensity of the light passing through each slit was initially the same. The intensity of the light through one of the slits is now reduced.
Compare the appearance of the fringes before and after the change of intensity.
Answer/Explanation
Answer:
(a) (i) vibrations (of particles) are parallel to direction of energy propagation
(ii) waves meet/overlap (at a point)
(b)(i) λ = ax / D
\(= (3.7 × 10^{–4} × 4.3 × 10^{–3}) / 2.3\)
\( = 6.9 \times 10^{-7}\) (m)
= 690 nm
(ii) • no change to fringe separation/fringe width/number of fringes
• bright fringes are darker
• dark fringes are brighter
Any two marking points, 1 mark each
