Question
Find the least positive value of x for which cos \(\left ( \frac{x}{2} + \frac{\pi }{3} \right ) = \frac{1}{\sqrt{2}}.\)
▶️Answer/Explanation
Ans:
determines \(\frac{\pi }{4}\) ( or 450) as the first quadrant (reference) angle attempts to solve \(\frac{x }{2} + \frac{\pi }{3} = \frac{\pi }{4}\)
Note: Award M1 for attempting to solve \(\frac{x }{2} + \frac{\pi }{3} = \frac{\pi }{4}, \frac{7\pi }{4} (,…)\)
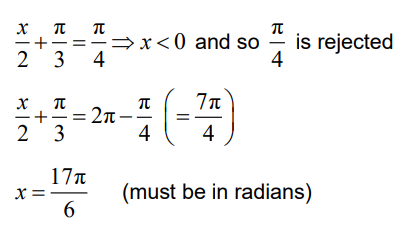
Question
A function f is defined by f(x) = \(\frac{2x – 1}{x + 1}\), where x ∈ R, x ≠ -1.
(a) The graph of y = f (x) has a vertical asymptote and a horizontal asymptote.
Write down the equation of
(i) the vertical asymptote;
(ii) the horizontal asymptote.
(b) On the set of axes below, sketch the graph of y = f (x) .
On your sketch, clearly indicate the asymptotes and the position of any points of intersection with the axes.
(c) Hence, solve the inequality \(0<\frac{2x – 1}{x + 1} <2.\)
(d) Solve the inequality \(0<\frac{2|x|-1}{|x| + 1} <2.\)
▶️Answer/Explanation
Ans:
(a) (i) x =−1
(ii) y = 2
(b) 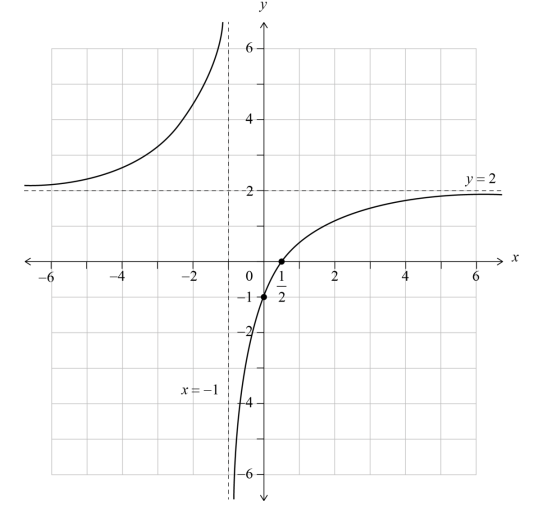
rational function shape with two branches in opposite quadrants, with two correctly positioned asymptotes and asymptotic behaviour shown axes intercepts clearly shown at \(x = \frac{1}{2} and y = -1\)
(c) x > \(\frac{1}{2}\)
Note: Accept correct alternative correct notation, such as \(\left ( \frac{1}{2}, \infty \right ) and ]\frac{1}{2}, \infty [.\)
(d) EITHER
attempts to sketch \(y = \frac{2|x| – 1}{|x|+1}\)
OR
attempts to solve 2|x| – 1 = 0
Note: Award the (M1) if \(x = \frac{1}{2} and x = -\frac{1}{2}\) are identified.
THEN
\(x <-\frac{1}{2} and x >\frac{1}{2}\)
Note: Accept the use of a comma. Condone the use of ‘and’. Accept correct alternative notation.
