Question
[Maximum mark: 5]
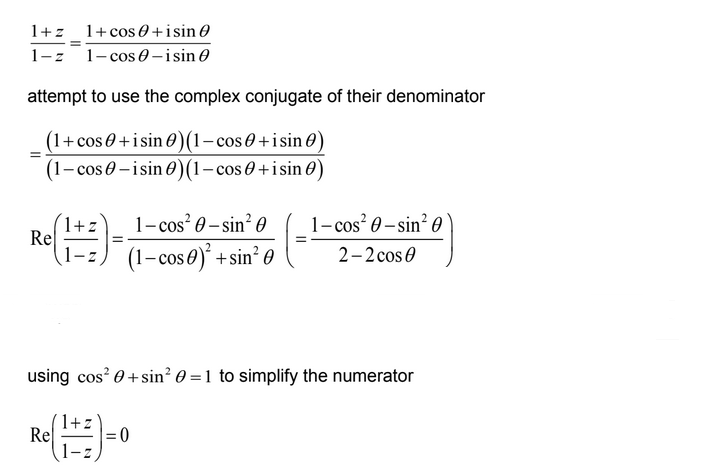
Consider \(z=\cos \Theta +i\sin \Theta \) where \(z\epsilon C ,z\neq 1.\)
Show that\(Re\left ( \frac{1+z}{1-z} \right )=0\)
▶️Answer/Explanation
Question
(a) Solve the equation \({z^3} = – 2 + 2{\text{i}}\), giving your answers in modulus-argument form.
(b) Hence show that one of the solutions is 1 + i when written in Cartesian form.
▶️Answer/Explanation
Markscheme
(a) \({z^3} = 2\sqrt 2 {{\text{e}}^{\frac{{3\pi {\text{i}}}}{4}}}\) (M1)(A1)
\({z_1} = \sqrt 2 {{\text{e}}^{\frac{{\pi {\text{i}}}}{4}}}\) A1
adding or subtracting \(\frac{{2\pi {\text{i}}}}{3}\) M1
\({z_2} = \sqrt 2 {{\text{e}}^{\frac{{\pi {\text{i}}}}{4} + \frac{{2\pi {\text{i}}}}{3}}} = \sqrt 2 {{\text{e}}^{\frac{{11\pi {\text{i}}}}{{12}}}}\) A1
\({z_3} = \sqrt 2 {{\text{e}}^{\frac{{\pi {\text{i}}}}{4} – \frac{{2\pi {\text{i}}}}{3}}} = \sqrt 2 {{\text{e}}^{ – \frac{{5\pi {\text{i}}}}{{12}}}}\) A1
Notes: Accept equivalent solutions e.g. \({z_3} = \sqrt 2 {{\text{e}}^{\frac{{19\pi {\text{i}}}}{{12}}}}\)
Award marks as appropriate for solving \({(a + b{\text{i}})^3} = – 2 + 2{\text{i}}\).
Accept answers in degrees.
(b) \(\sqrt 2 {{\text{e}}^{\frac{{\pi {\text{i}}}}{4}}}{\text{ }}\left( { = \sqrt 2 \left( {\frac{1}{{\sqrt 2 }} + \frac{{\text{i}}}{{\sqrt 2 }}} \right)} \right)\) A1
= 1 + i AG
Note: Accept geometrical reasoning.
[7 marks]
Examiners report
Many students incorrectly found the argument of \({z^3}\) to be \(\arctan \left( {\frac{2}{{ – 2}}} \right) = – \frac{\pi }{4}\). Of those students correctly finding one solution, many were unable to use symmetry around the origin, to find the other two. In part (b) many students found the cube of 1 + i which could not be awarded marks as it was not “hence”.
Question
Let \(\omega = \cos \theta + {\text{i}}\sin \theta \) . Find, in terms of \(\theta \) , the modulus and argument of \({(1 – {\omega ^2})^ * }\) .
▶️Answer/Explanation
Markscheme
METHOD 1
\({(1 – {\omega ^2})^ * } = {(1 – {\text{cis}}\,2\theta )^ * } = {\left( {(1 – \cos 2\theta ) – {\text{i}}\sin 2\theta } \right)^ * }\) M1A1
\( = (1 – \cos 2\theta ) + {\text{i}}\sin 2\theta \) A1
\(\left| {{{(1 – {\omega ^2})}^ * }} \right| = \sqrt {{{(1 – \cos 2\theta )}^2} + {{\sin }^2}2\theta } \left( { = \sqrt {{{(2{{\sin }^2}\theta )}^2} + {{(2\sin \theta \cos \theta )}^2}} } \right)\) M1
\( = \left| {2\sin \theta } \right|\) A1
\(\arg \left( {{{(1 – {\omega ^2})}^ * }} \right) = \alpha \Rightarrow \tan \alpha = \cot (\theta )\) M1
\(\alpha = \frac{\pi }{2} – \theta \) A1
therefore:
modulus is \(2\left| {\sin \theta } \right|\) and argument is \(\frac{\pi }{2} – \theta {\text{ or }}\frac{\pi }{2} – \theta \pm \pi \)
Note: Accept modulus is 2sinθ and argument is \(\frac{\pi }{2} – \theta \)
METHOD 2
EITHER
\({(1 – {\varpi ^2})^ * } = {(1 – {\text{cis}}\,2\theta )^ * } = {\left( {(1 – \cos 2\theta ) – {\text{i}}\sin 2\theta } \right)^ * }\) M1A1
\( = (1 – \cos 2\theta ) + {\text{i}}\sin 2\theta \) A1
\( = (1 – 1 + 2{\sin ^2}\theta ) + 2{\text{i}}\sin \theta \cos \theta \) M1
OR
\({(1 – {\varpi ^2})^ * } = {\left( {1 – {{(\cos \theta + {\text{i}}\sin \theta )}^2}} \right)^ * }\) M1A1
\( = {(1 – {\cos ^2}\theta + {\sin ^2}\theta – 2{\text{i}}\sin \theta \cos \theta )^ * }\) A1
\( = 2{\sin ^2}\theta + 2{\text{i}}\sin \theta \cos \theta \) M1
THEN
\( = 2\sin \theta (\sin \theta + {\text{i}}\cos \theta )\) (M1)
\( = 2\sin \theta \left( {\cos \left( {\frac{\pi }{2} – \theta } \right) + {\text{i}}\sin \left( {\frac{\pi }{2} – \theta } \right)} \right)\) A1A1
\( = 2\sin \theta \,{\text{cis}}\left( {\frac{\pi }{2} – \theta } \right)\)
therefore:
modulus is \(2\left| {\sin \theta } \right|\) and argument is \(\frac{\pi }{2} – \theta {\text{ or }}\frac{\pi }{2} – \theta \pm \pi \)
Note: Accept modulus is 2sinθ and argument is \(\frac{\pi }{2} – \theta \) .
[7 marks]
Examiners report
This was the most challenging question in part A with just a few candidates scoring full marks. This question showed that many candidates have difficulties with algebraic manipulations, application of De Moivre’s theorem and use of trigonometric identities. Although some candidates managed to calculate the square of a complex number, many failed to write down its conjugate or made algebraic errors which lead to wrong results in many cases. Just a few candidates were able to calculate the modulus and the argument of the complex number.
Question
Find, in its simplest form, the argument of \({\left( {\sin \theta + {\text{i}}(1 – \cos \theta )} \right)^2}\) where \(\theta \) is an acute angle.
▶️Answer/Explanation
Markscheme
\({\left( {\sin \theta + {\text{i}}(1 – \cos \theta )} \right)^2} = {\sin ^2}\theta – {(1 – \cos \theta )^2} + {\text{i}}2\sin \theta (1 – \cos \theta )\) M1A1
Let \(\alpha \) be the required argument.
\(\tan \alpha = \frac{{2\sin \theta (1 – \cos \theta )}}{{{{\sin }^2}\theta – {{(1 – \cos \theta )}^2}}}\) M1
\( = \frac{{2\sin \theta (1 – \cos \theta )}}{{(1 – {{\cos }^2}\theta ) – (1 – 2\cos \theta + {{\cos }^2}\theta )}}\) (M1)
\( = \frac{{2\sin \theta (1 – \cos \theta )}}{{2\cos \theta (1 – \cos \theta )}}\) A1
\( = \tan \theta \) A1
\(\alpha = \theta \) A1
[7 marks]
Examiners report
Very few candidates scored more than the first two marks in this question. Some candidates had difficulty manipulating trigonometric identities. Most candidates did not get as far as defining the argument of the complex expression.
