Question
(i) Sketch the graphs of \(y = \sin x\) and \(y = \sin 2x\) , on the same set of axes, for \(0 \leqslant x \leqslant \frac{\pi }{2}\) .
(ii) Find the x-coordinates of the points of intersection of the graphs in the domain \(0 \leqslant x \leqslant \frac{\pi }{2}\) .
(iii) Find the area enclosed by the graphs.[9]
Find the value of \(\int_0^1 {\sqrt {\frac{x}{{4 – x}}} }{{\text{d}}x} \) using the substitution \(x = 4{\sin ^2}\theta \) .[8]
The increasing function f satisfies \(f(0) = 0\) and \(f(a) = b\) , where \(a > 0\) and \(b > 0\) .
(i) By reference to a sketch, show that \(\int_0^a {f(x){\text{d}}x = ab – \int_0^b {{f^{ – 1}}(x){\text{d}}x} } \) .
(ii) Hence find the value of \(\int_0^2 {\arcsin \left( {\frac{x}{4}} \right){\text{d}}x} \) .[8]
▶️Answer/Explanation
Markscheme
(i)
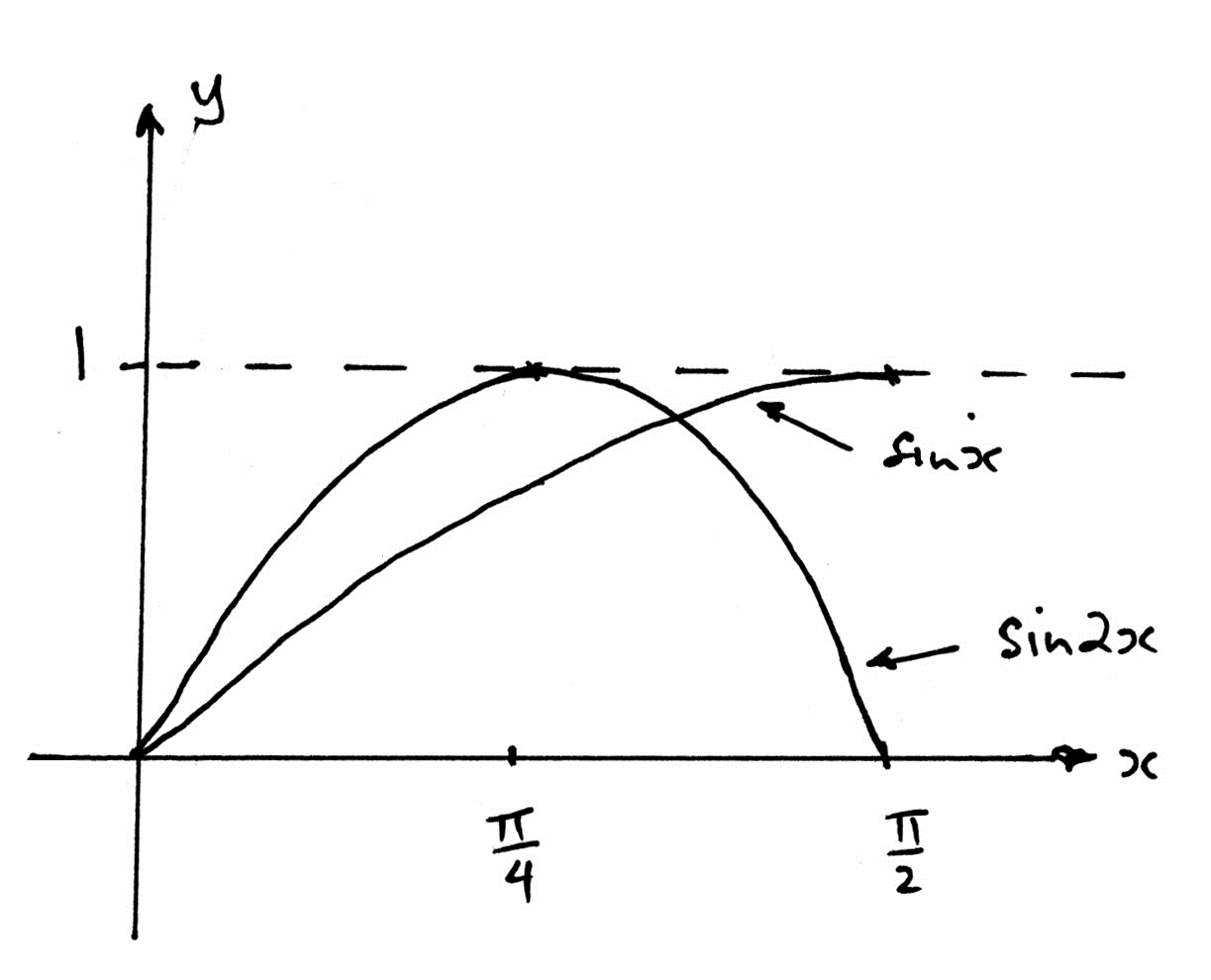 A2
A2
Note: Award A1 for correct \(\sin x\) , A1 for correct \(\sin 2x\) .
Note: Award A1A0 for two correct shapes with \(\frac{\pi }{2}\) and/or 1 missing.
Note: Condone graph outside the domain.
(ii) \(\sin 2x = \sin x\) , \(0 \leqslant x \leqslant \frac{\pi }{2}\)
\(2\sin x\cos x – \sin x = 0\) M1
\(\sin x(2\cos x – 1) = 0\)
\(x = 0,\frac{\pi }{3}\) A1A1 N1N1
(iii) area \( = \int_0^{\frac{\pi }{3}} {(\sin 2x – \sin x){\text{d}}x} \) M1
Note: Award M1 for an integral that contains limits, not necessarily correct, with \(\sin x\) and \(\sin 2x\) subtracted in either order.
\( = \left[ { – \frac{1}{2}\cos 2x + \cos x} \right]_0^{\frac{\pi }{3}}\) A1
\( = \left( { – \frac{1}{2}\cos \frac{{2\pi }}{3} + \cos \frac{\pi }{3}} \right) – \left( { – \frac{1}{2}\cos 0 + \cos 0} \right)\) (M1)
\( = \frac{3}{4} – \frac{1}{2}\)
\( = \frac{1}{4}\) A1
[9 marks]
\(\int_0^1 {\sqrt {\frac{x}{{4 – x}}} } {\text{d}}x = \int_0^{\frac{\pi }{6}} {\sqrt {\frac{{4{{\sin }^2}\theta }}{{4 – 4{{\sin }^2}\theta }}} \times 8\sin \theta \cos \theta {\text{d}}\theta } \) M1A1A1
Note: Award M1 for substitution and reasonable attempt at finding expression for dx in terms of \({\text{d}}\theta \) , first A1 for correct limits, second A1 for correct substitution for dx .
\(\int_0^{\frac{\pi }{6}} {8{{\sin }^2}\theta {\text{d}}\theta } \) A1
\(\int_0^{\frac{\pi }{6}} {4 – 4\cos 2\theta {\text{d}}\theta } \) M1
\( = [4\theta – 2\sin 2\theta ]_0^{\frac{\pi }{6}}\) A1
\( = \left( {\frac{{2\pi }}{3} – 2\sin \frac{\pi }{3}} \right) – 0\) (M1)
\( = \frac{{2\pi }}{3} – \sqrt 3 \) A1
[8 marks]
(i)
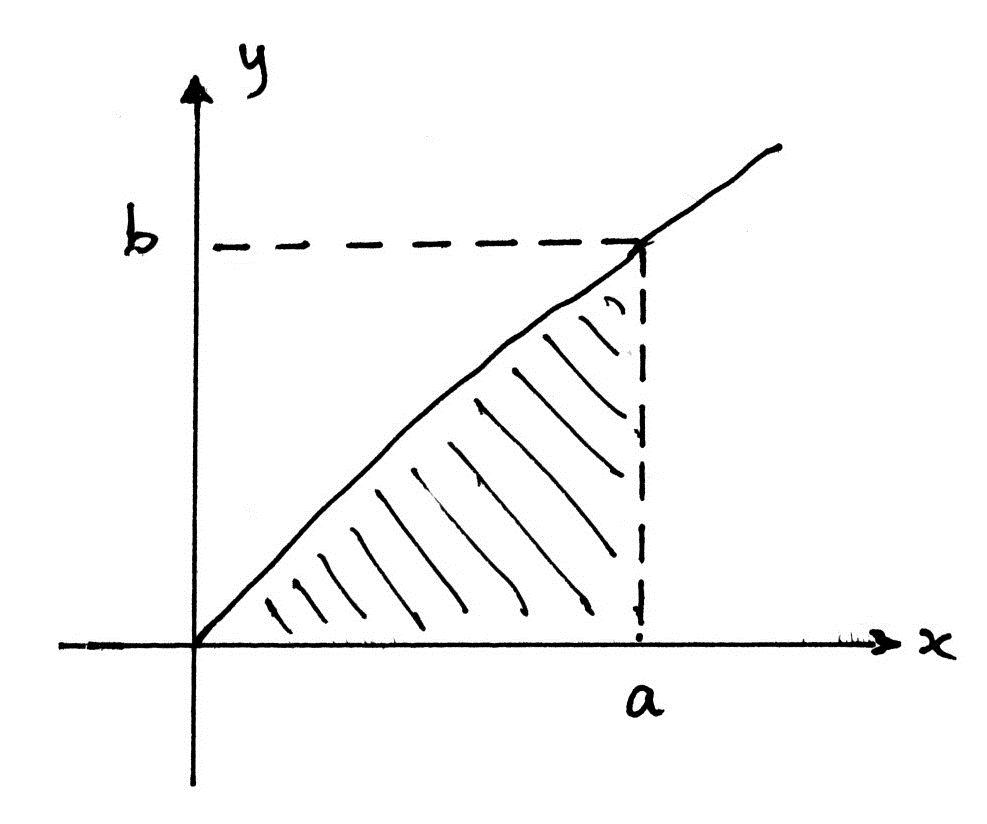 M1
M1
from the diagram above
the shaded area \( = \int_0^a {f(x){\text{d}}x = ab – \int_0^b {{f^{ – 1}}(y){\text{d}}y} } \) R1
\({ = ab – \int_0^b {{f^{ – 1}}(x){\text{d}}x} }\) AG
(ii) \(f(x) = \arcsin \frac{x}{4} \Rightarrow {f^{ – 1}}(x) = 4\sin x\) A1
\(\int_0^2 {\arcsin \left( {\frac{x}{4}} \right){\text{d}}x = \frac{\pi }{3} – \int_0^{\frac{\pi }{6}} {4\sin x{\text{d}}x} } \) M1A1A1
Note: Award A1 for the limit \(\frac{\pi }{6}\) seen anywhere, A1 for all else correct.
\( = \frac{\pi }{3} – [ – 4\cos x]_0^{\frac{\pi }{6}}\) A1
\( = \frac{\pi }{3} – 4 + 2\sqrt 3 \) A1
Note: Award no marks for methods using integration by parts.
[8 marks]
Question
Let \(f(x) = \frac{{\sin 3x}}{{\sin x}} – \frac{{\cos 3x}}{{\cos x}}\).
For what values of x does \(f(x)\) not exist?[2]
Simplify the expression \(\frac{{\sin 3x}}{{\sin x}} – \frac{{\cos 3x}}{{\cos x}}\).[5]
▶️Answer/Explanation
Markscheme
\(\cos x = 0{\text{, }}\sin x = 0\) (M1)
\(x = \frac{{n\pi }}{2},n \in \mathbb{Z}\) A1
EITHER
\(\frac{{\sin 3x\cos x – \cos 3x\sin x}}{{\sin x\cos x}}\) M1 A1
\( = \frac{{\sin (3x – x)}}{{\frac{1}{2}\sin 2x}}\) A1 A1
\( = 2\) A1
OR
\(\frac{{\sin 2x\cos x + \cos 2x\sin x}}{{\sin x}} – \frac{{\cos 2x\cos x – \sin 2x\sin x}}{{\cos x}}\) M1
\( = \frac{{2\sin x{{\cos }^2}x + 2{{\cos }^2}x\sin x – \sin x}}{{\sin x}} – \frac{{2{{\cos }^3}x – \cos x – {{\sin }^2}x\cos x}}{{\cos x}}\) A1 A1
\( = 4{\cos ^2}x – 1 – 2{\cos ^2}x + 1 + 2{\sin ^2}x\) A1
\( = 2{\cos ^2}x + 2{\sin ^2}x\)
\( = 2\) A1
[5 marks]
Question
In the triangle ABC, \({\rm{A\hat BC}} = 90^\circ\) , \({\text{AC}} = \sqrt {\text{2}}\) and AB = BC + 1.
Show that cos \(\hat A – \sin \hat A = \frac{1}{{\sqrt 2 }}\).[3]
By squaring both sides of the equation in part (a), solve the equation to find the angles in the triangle.[8]
Apply Pythagoras’ theorem in the triangle ABC to find BC, and hence show that \(\sin \hat A = \frac{{\sqrt 6 – \sqrt 2 }}{4}\).[6]
Hence, or otherwise, calculate the length of the perpendicular from B to [AC].[4]
▶️Answer/Explanation
Markscheme
\(\cos \hat A = \frac{{{\text{BA}}}}{{\sqrt 2 }}\) A1
\(\sin \hat A = \frac{{{\text{BC}}}}{{\sqrt 2 }}\) A1
\(\cos \hat A – \sin \hat A = \frac{{{\text{BA}} – {\text{BC}}}}{{\sqrt 2 }}\) R1
\( = \frac{1}{{\sqrt 2 }}\) AG
[3 marks]
\({\cos ^2}\hat A – 2\cos \hat A\sin \hat A + {\sin ^2}\hat A = \frac{1}{2}\) M1A1
\(1 – 2\sin \hat A\cos \hat A = \frac{1}{2}\) M1A1
\(\sin 2\hat A = \frac{1}{2}\) M1
\(2\hat A = 30^\circ \) A1
angles in the triangle are 15° and 75° A1A1
Note: Accept answers in radians.
[8 marks]
\({\text{B}}{{\text{C}}^2} + {({\text{BC}} + 1)^2} = 2\) M1A1
\(2{\text{B}}{{\text{C}}^2} + 2{\text{BC}} – 1 = 0\) A1
\({\text{BC}} = \frac{{ -2 + \sqrt {12} }}{4}\left( { = \frac{{\sqrt 3 – 1}}{2}} \right)\) M1A1
\(\sin \hat A = \frac{{{\text{BC}}}}{{\sqrt 2 }} = \frac{{\sqrt 3 – 1}}{{2\sqrt 2 }}\) A1
\( = \frac{{\sqrt 6 – \sqrt 2 }}{4}\) AG
[6 marks]
EITHER
\(h = {\text{ABsin}}\hat A\) M1
\( = ({\text{BC}} + 1)\sin \hat A\) A1
\( = \frac{{\sqrt 3 + 1}}{2} \times \frac{{\sqrt 6 – \sqrt 2 }}{4} = \frac{{\sqrt 2 }}{4}\) M1A1
OR
\(\tfrac{1}{2}AB.BC = \tfrac{1}{2}AC.h\) M1
\(\frac{{\sqrt 3 – 1}}{2} \cdot \frac{{\sqrt {3 + 1} }}{2} = \sqrt {2h} \) A1
\(\frac{2}{4} = \sqrt 2 h\) M1
\(h = \frac{1}{{2\sqrt 2 }}\) A1
[4 marks]
Question
The function f is defined on the domain \(\left[ {0,\,\frac{{3\pi }}{2}} \right]\) by \(f(x) = {e^{ – x}}\cos x\) .
State the two zeros of f .[1]
Sketch the graph of f .[1]
The region bounded by the graph, the x-axis and the y-axis is denoted by A and the region bounded by the graph and the x-axis is denoted by B . Show that the ratio of the area of A to the area of B is
\[\frac{{{e^\pi }\left( {{e^{\frac{\pi }{2}}} + 1} \right)}}{{{e^\pi } + 1}}.\][7]
▶️Answer/Explanation
Markscheme
\({e^{ – x}}\cos x = 0\)
\( \Rightarrow x = \frac{\pi }{2},{\text{ }}\frac{{3\pi }}{2}\) A1
[1 mark]
 A1
A1
Note: Accept any form of concavity for \(x \in \left[ {0,\frac{\pi }{2}} \right]\).
Note: Do not penalize unmarked zeros if given in part (a).
Note: Zeros written on diagram can be used to allow the mark in part (a) to be awarded retrospectively.
[1 mark]
attempt at integration by parts M1
EITHER
\(I = \int {{{\text{e}}^{ – x}}\cos x{\text{d}}x = – {{\text{e}}^{ – x}}\cos x{\text{d}}x – \int {{{\text{e}}^{ – x}}\sin x{\text{d}}x} } \) A1
\( \Rightarrow I = – {{\text{e}}^{ – x}}\cos x{\text{d}}x – \left[ { – {{\text{e}}^{ – x}}\sin x + \int {{{\text{e}}^{ – x}}\cos x{\text{d}}x} } \right]\) A1
\( \Rightarrow I = \frac{{{{\text{e}}^{ – x}}}}{2}(\sin x – \cos x) + C\) A1
Note: Do not penalize absence of C.
OR
\(I = \int {{{\text{e}}^{ – x}}\cos x{\text{d}}x = {{\text{e}}^{ – x}}\sin x + \int {{{\text{e}}^{ – x}}\sin x{\text{d}}x} } \) A1
\(I = {{\text{e}}^{ – x}}\sin x – {{\text{e}}^{ – x}}\cos x – \int {{{\text{e}}^{ – x}}\cos x{\text{d}}x} \) A1
\( \Rightarrow I = \frac{{{{\text{e}}^{ – x}}}}{2}(\sin x – \cos x) + C\) A1
Note: Do not penalize absence of C.
THEN
\(\int_0^{\frac{\pi }{2}} {{{\text{e}}^{ – x}}\cos x{\text{d}}x = \left[ {\frac{{{{\text{e}}^{ – x}}}}{2}(\sin x – \cos x)} \right]} _0^{\frac{\pi }{2}} = \frac{{{{\text{e}}^{ – \frac{\pi }{2}}}}}{2} + \frac{1}{2}\) A1
\(\int_{\frac{\pi }{2}}^{\frac{{3\pi }}{2}} {{{\text{e}}^{ – x}}\cos x{\text{d}}x = \left[ {\frac{{{{\text{e}}^{ – x}}}}{2}(\sin x – \cos x)} \right]_{\frac{\pi }{2}}^{\frac{{3\pi }}{2}} = – \frac{{{{\text{e}}^{ – \frac{{3\pi }}{2}}}}}{2} – \frac{{{{\text{e}}^{ – \frac{\pi }{2}}}}}{2}} \) A1
ratio of A:B is \(\frac{{\frac{{{{\text{e}}^{ – \frac{\pi }{2}}}}}{2} + \frac{1}{2}}}{{\frac{{{{\text{e}}^{ – \frac{{3\pi }}{2}}}}}{2} + \frac{{{{\text{e}}^{ – \frac{\pi }{2}}}}}{2}}}\)
\( = \frac{{{{\text{e}}^{\frac{{3\pi }}{2}}}\left( {{{\text{e}}^{ – \frac{\pi }{2}}} + 1} \right)}}{{{{\text{e}}^{\frac{{3\pi }}{2}}}\left( {{{\text{e}}^{ – \frac{{3\pi }}{2}}} + {{\text{e}}^{ – \frac{\pi }{2}}}} \right)}}\) M1
\( = \frac{{{{\text{e}}^\pi }\left( {{{\text{e}}^{\frac{\pi }{2}}} + 1} \right)}}{{{{\text{e}}^\pi } + 1}}\) AG
[7 marks]
