IBDP Maths AA - AHL 1.11 Partial fractions AA HL Paper 1- Exam Style Questions- New Syllabus
▶️ Answer/Explanation
For \(\frac{20x + 35}{(x + 4)^2}\), assume partial fractions: \(\frac{A}{x + 4} + \frac{B}{(x + 4)^2}\).
Multiply through by \((x + 4)^2\):
\(20x + 35 = A(x + 4) + B\).
Expand: \(20x + 35 = Ax + (4A + B)\).
Equate coefficients:
– \(x\): \(A = 20\).
– Constant: \(4A + B = 35 \implies 4 \cdot 20 + B = 35 \implies B = 35 – 80 = -45\).
Thus, \(\frac{20x + 35}{(x + 4)^2} = \frac{20}{x + 4} – \frac{45}{(x + 4)^2}\).
Markscheme
Form: \(\frac{A}{x + 4} + \frac{B}{(x + 4)^2} \quad \mathbf{M1} \)
Equation: \(20x + 35 = A(x + 4) + B \quad \mathbf{M1} \)
Coefficients: \(A = 20 \quad \mathbf{A1} \)
Result: \(B = -45 \), final form \(\frac{20}{x + 4} – \frac{45}{(x + 4)^2} \quad \mathbf{A1} \)
[4 marks]
▶️ Answer/Explanation
Factor the denominator using the factor theorem: \(x = -2\) gives \( (-2)^3 + 3(-2)^2 + 3(-2) + 2 = -8 + 12 – 6 + 2 = 0 \), so \(x + 2\) is a factor.
Divide \(x^3 + 3x^2 + 3x + 2\) by \(x + 2\): quotient is \(x^2 + x + 1\). Thus, \(x^3 + 3x^2 + 3x + 2 = (x + 2)(x^2 + x + 1)\).
Write: \(\frac{x^2 + 1}{(x + 2)(x^2 + x + 1)} = \frac{A}{x + 2} + \frac{Bx + C}{x^2 + x + 1}\).
Multiply by \((x + 2)(x^2 + x + 1)\):
\(x^2 + 1 = A(x^2 + x + 1) + (Bx + C)(x + 2)\).
Expand: \(x^2 + 1 = A x^2 + A x + A + B x^2 + 2B x + C x + 2C = (A + B)x^2 + (A + 2B + C)x + (A + 2C)\).
Equate coefficients:
– \(x^2\): \(A + B = 1\).
– \(x\): \(A + 2B + C = 0\).
– Constant: \(A + 2C = 1\).
Solve: From \(A + 2C = 1\) and \(A + B = 1\), set \(A = 1 – B\). Then \(A + 2C = 1 \implies (1 – B) + 2C = 1 \implies 2C = B\). From \(A + 2B + C = 0 \implies (1 – B) + 2B + C = 0 \implies 1 + B + C = 0 \implies C = -1 – B\).
Substitute \(2C = B\) into \(C = -1 – B\): \(2(-1 – B) = B \implies -2 – 2B = B \implies -2 = 3B \implies B = -\frac{2}{3}\).
Then \(C = -\frac{1}{3}\), \(A = 1 – \left(-\frac{2}{3}\right) = \frac{5}{3}\).
Thus, \(\frac{x^2 + 1}{x^3 + 3x^2 + 3x + 2} = \frac{\frac{5}{3}}{x + 2} – \frac{\frac{2}{3}x + \frac{1}{3}}{x^2 + x + 1} = \frac{5}{3(x + 2)} – \frac{2x + 1}{3(x^2 + x + 1)}\).
Markscheme
Factorize denominator: \((x + 2)(x^2 + x + 1) \quad \mathbf{M1} \)
Form: \(\frac{A}{x + 2} + \frac{Bx + C}{x^2 + x + 1} \quad \mathbf{M1} \)
Equation: \(x^2 + 1 = A(x^2 + x + 1) + (Bx + C)(x + 2) \quad \mathbf{M1} \)
Coefficient equations: \(A + B = 1\), \(A + 2B + C = 0\), \(A + 2C = 1 \quad \mathbf{A1} \)
Solve: \(A = \frac{5}{3} \quad \mathbf{A1} \)
Result: \(B = -\frac{2}{3}\), \(C = -\frac{1}{3}\), final form \(\frac{5}{3(x + 2)} – \frac{2x + 1}{3(x^2 + x + 1)} \quad \mathbf{A1} \)
[6 marks]
Question
Express Following in Partial Fraction
▶️Answer/Explanation
Solution
We can decompose it as such:
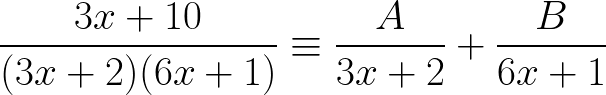
And so we can find A and B, by simplifying the RHS and compare the coefficients.
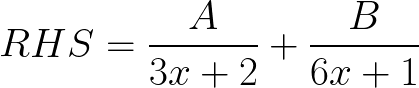
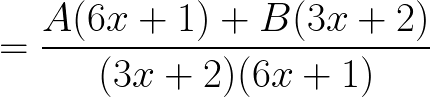
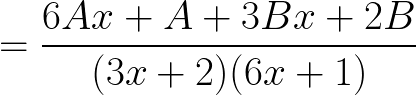
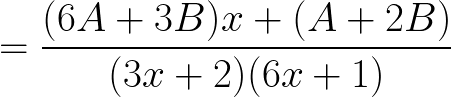
Now we have the case where we can compare the coefficients, since RHS must be equivalent to LHS, the coefficients must be equal. In other words:
We can solve it using our GDC, linSolve() again, and get the answers:
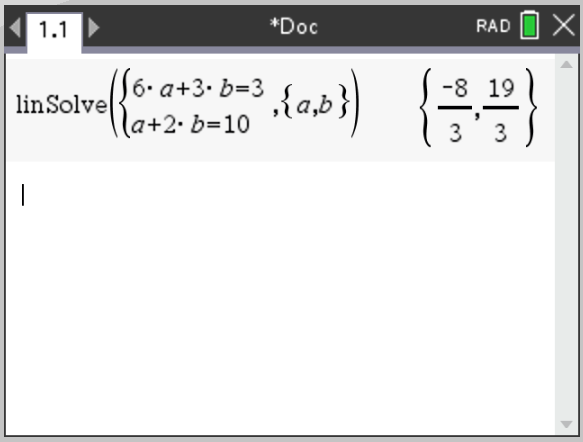
So we can re-write all of these:
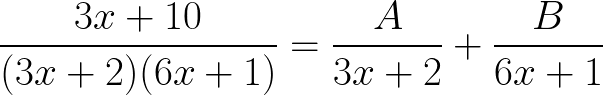
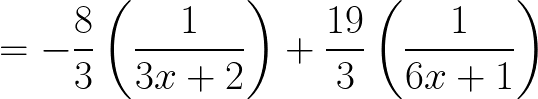
Since the IB only requires us to perform this for at most 2 decomposed fractions, we can stop here. But if there are more, you can just imagine adding a third term with C and it’ll be the same! Do note that it’ll be slightly different when there are non-linear terms in the denominator, but that would be beyond the scope of IB maths.
▶️ Answer/Explanation
Factor the denominator: \(x^2 – 2x – 15 = (x – 5)(x + 3)\).
Write: \(\frac{17x – 53}{(x – 5)(x + 3)} = \frac{A}{x – 5} + \frac{B}{x + 3}\).
Multiply by \((x – 5)(x + 3)\):
\(17x – 53 = A(x + 3) + B(x – 5)\).
Substitute convenient values:
– At \(x = 5\): \(17 \cdot 5 – 53 = 32 = A(5 + 3) \implies 32 = 8A \implies A = 4\).
– At \(x = -3\): \(17 \cdot (-3) – 53 = -51 – 53 = -104 = B(-3 – 5) \implies -104 = -8B \implies B = 13\).
Thus, \(\frac{17x – 53}{x^2 – 2x – 15} = \frac{4}{x – 5} + \frac{13}{x + 3}\).
Markscheme
Factorize denominator: \((x – 5)(x + 3) \quad \mathbf{M1} \)
Form: \(\frac{A}{x – 5} + \frac{B}{x + 3} \quad \mathbf{M1} \)
Solve: \(A = 4 \quad \mathbf{A1} \)
Result: \(B = 13\), final form \(\frac{4}{x – 5} + \frac{13}{x + 3} \quad \mathbf{A1} \)
[4 marks]