Question
The expansion of \((x + h)^8\) , where h > 0, can be written as \(x^8+ax^7 + bx^6 + cx^5 + dx^4 + … + h^8\), where a, c, b, c, d, … \(\epsilon \mathbb{R}\)
(a) Find an expression, in terms of h, for
(i) a;
(ii) b;
(iii) d .
(b) Given that a, b, and d are the first three terms of a geometric sequence, find the value of h.
▶️Answer/Explanation
Answer:
(a) attempt to use the binomial expansion of \((x+h)^8\)![]()
(i) a = 8h (accept \(^8C_1\)h )
(ii) b = 28\(h^2\) (accept \(^8C_2h^2\))
(iii) d = 70\(h^4\) (accept \(^8C_4 h^4\))
(b) recognition that there is a common ratio between their terms
\(8h \times r = 28 h^2\) OR \(28h^2 \times r= 70 h^4\) OR \(8h \times r^2 = 70h^4\)
correct equation in terms of h
\(\frac{28h^2}{8h} = \frac{70h^4}{28h^2}\)(or equivalent)
h = 1.4
Question
Consider the expansion of \((3x^2- \frac{k}{x})^9\)
The coefficient of the term in x6 is 6048. Find the value of k .
Answer/Explanation
Ans
valid approach for expansion (must have correct substitution for parameters, but accept an incorrect value for r).
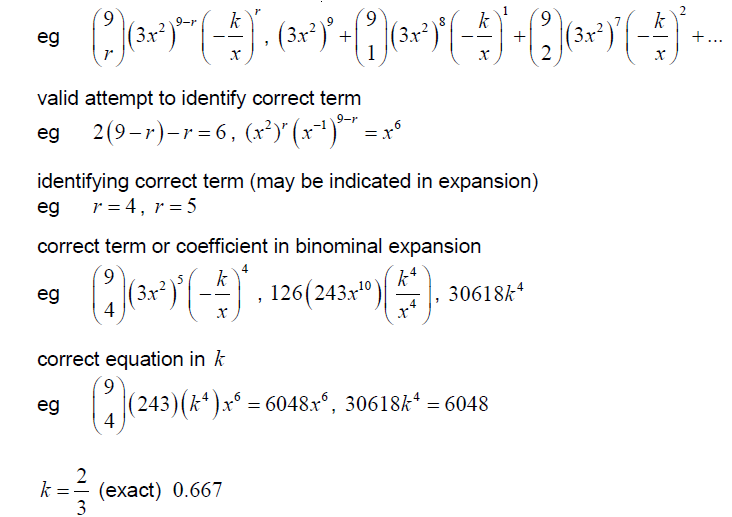
Question
Consider the expansion of \({\left( {2x + \frac{k}{x}} \right)^9}\), where k > 0 . The coefficient of the term in x3 is equal to the coefficient of the term in x5. Find k.
Answer/Explanation
Markscheme
valid approach to find one of the required terms (must have correct substitution for parameters but accept “r” or an incorrect value for r) (M1)
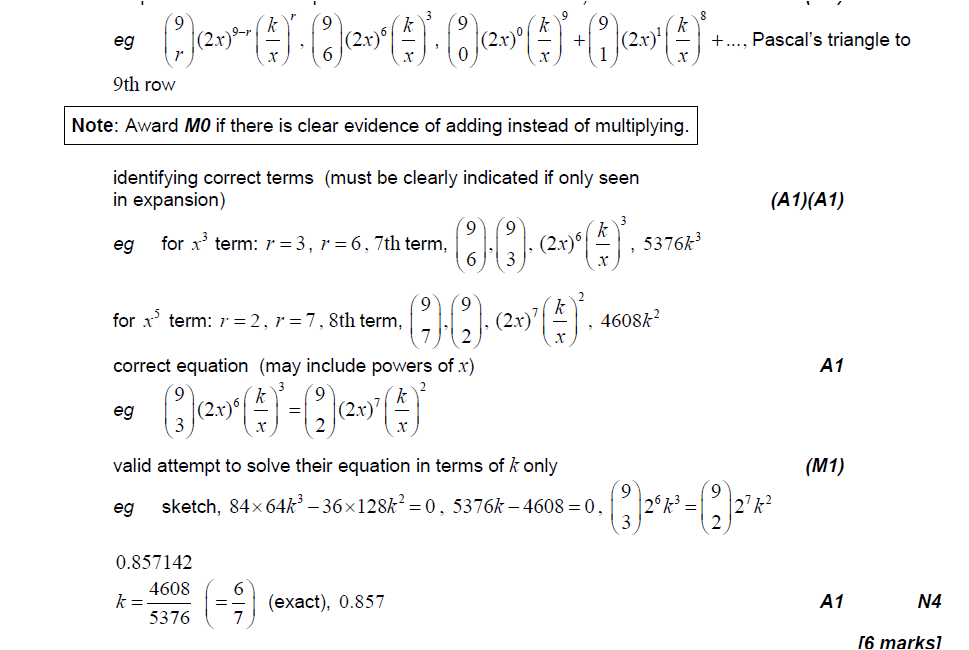
Question
Find the term \({x^3}\) in the expansion of \({\left( {\frac{2}{3}x – 3} \right)^8}\) .
Answer/Explanation
Markscheme
evidence of using binomial expansion (M1)
e.g. selecting correct term, \({a^8}{b^0} + \left( {\begin{array}{*{20}{c}}
8\\
1
\end{array}} \right){a^7}b + \left( {\begin{array}{*{20}{c}}
8\\
2
\end{array}} \right){a^6}{b^2} + \ldots \)
evidence of calculating the factors, in any order A1A1A1
e.g. 56 , \(\frac{{{2^2}}}{{{3^3}}}\) , \( – {3^5}\) , \(\left( {\begin{array}{*{20}{c}}
8\\
5
\end{array}} \right){\left( {\frac{2}{3}x} \right)^3}{( – 3)^5}\)
\( – 4032{x^3}\) (accept = \( – 4030{x^3}\) to 3 s.f.) A1 N2
[5 marks]
Question
Let \(f(x) = {x^3} – 4x + 1\) .
a.Expand \({(x + h)^3}\) .[2]
b.Use the formula \(f'(x) = \mathop {\lim }\limits_{h \to 0} \frac{{f(x + h) – f(x)}}{h}\) to show that the derivative of \(f(x)\) is \(3{x^2} – 4\) .[4]
c.The tangent to the curve of f at the point \({\text{P}}(1{\text{, }} – 2)\) is parallel to the tangent at a point Q. Find the coordinates of Q.[4]
d.The graph of f is decreasing for \(p < x < q\) . Find the value of p and of q.[3]
Answer/Explanation
Markscheme
attempt to expand (M1)
\({(x + h)^3} = {x^3} + 3{x^2}h + 3x{h^2} + {h^3}\) A1 N2
[2 marks]
evidence of substituting \(x + h\) (M1)
correct substitution A1
e.g. \(f'(x) = \mathop {\lim }\limits_{h \to 0} \frac{{{{(x + h)}^3} – 4(x + h) + 1 – ({x^3} – 4x + 1)}}{h}\)
simplifying A1
e.g. \(\frac{{({x^3} + 3{x^2}h + 3x{h^2} + {h^3} – 4x – 4h + 1 – {x^3} + 4x – 1)}}{h}\)
factoring out h A1
e.g. \(\frac{{h(3{x^2} + 3xh + {h^2} – 4)}}{h}\)
\(f'(x) = 3{x^2} – 4\) AG N0
[4 marks]
\(f'(1) = – 1\) (A1)
setting up an appropriate equation M1
e.g. \(3{x^2} – 4 = – 1\)
at Q, \(x = – 1,y = 4\) (Q is \(( – 1{\text{, }}4)\)) A1 A1
[4 marks]
recognizing that f is decreasing when \(f'(x) < 0\) R1
correct values for p and q (but do not accept \(p = 1.15{\text{, }}q = – 1.15\) ) A1A1 N1N1
e.g. \(p = – 1.15{\text{, }}q = 1.15\) ; \( \pm \frac{2}{{\sqrt 3 }}\) ; an interval such as \( – 1.15 \le x \le 1.15\)
[3 marks]
\(f'(x) \ge – 4\) , \(y \ge – 4\) , \(\left[ { – 4,\infty } \right[\) A2 N2
[2 marks]
