Question
The function f is defined by \(f(x)= \frac{4x+1}{x+4},\) where x ∈ R, x ≠ -4 .
(a) For the graph of f
(i) write down the equation of the vertical asymptote;
(ii) find the equation of the horizontal asymptote.
(b) (i) Find f -1(x).
(ii) Using an algebraic approach, show that the graph of f -1 is obtained by a reflection of the graph of f in the y-axis followed by a reflection in the x-axis.
The graphs of f and f -1 intersect at x = p and x = q, where p < q.
(c) (i) Find the value of p and the value of q.
(ii) Hence, find the area enclosed by the graph of f and the graph of f -1.
Answer/Explanation
Ans:
(a) (i) x =−4
(ii) attempt to substitute into \(\frac{a}{c}\) OR table with large values of x OR sketch of f showing asymptotic behaviour
y = 4
(b) (i) \(y=\frac{4x+1}{x+4},\)
attempt to interchange x and y (seen anywhere) 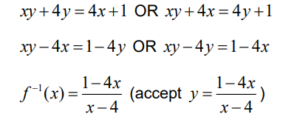
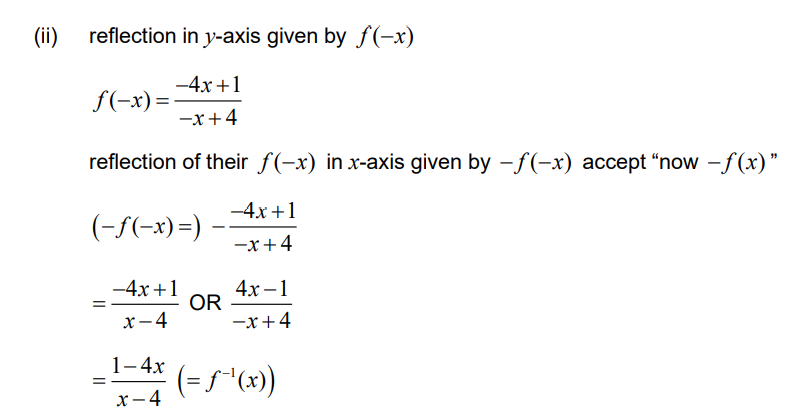
Note: If the candidate attempts to show the result using a particular coordinate on the graph of f rather than a general coordinate on the graph of f, where appropriate, award marks as follows:
M0A0 for eg (2,3) → (- 2,3)
M0A0 for ( -2,3) → ( -2, -3)
(c) (i) attempt to solve f(x) = f-1 (x) using graph or algebraically
p = −1 AND q =1
Note: Award (M1)A0 if only one correct value seen.
(ii) attempt to set up an integral to find area between f and f−1
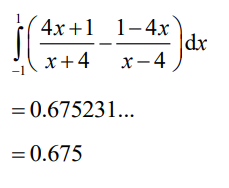
Question
Consider a function \(f\), for \(0 \le x \le 10\). The following diagram shows the graph of \(f’\), the derivative of \(f\).
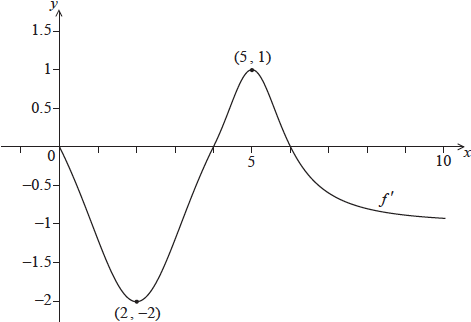
The graph of \(f’\) passes through \((2,{\text{ }} – 2)\) and \((5,{\text{ }}1)\), and has \(x\)-intercepts at \(0\), \(4\) and \(6\).
The graph of \(f\) has a local maximum point when \(x = p\). State the value of \(p\), and justify your answer.
Write down \(f'(2)\).
Let \(g(x) = \ln \left( {f(x)} \right)\) and \(f(2) = 3\).
Find \(g'(2)\).
Verify that \(\ln 3 + \int_2^a {g'(x){\text{d}}x = g(a)} \), where \(0 \le a \le 10\).
The following diagram shows the graph of \(g’\), the derivative of \(g\).
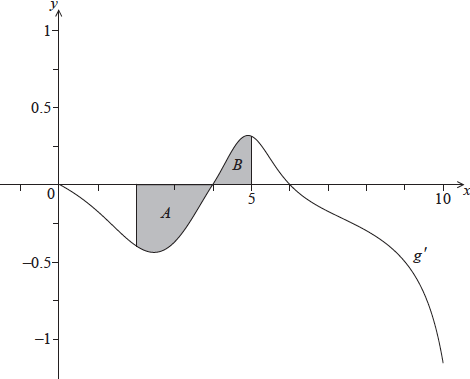
The shaded region \(A\) is enclosed by the curve, the \(x\)-axis and the line \(x = 2\), and has area \({\text{0.66 unit}}{{\text{s}}^{\text{2}}}\).
The shaded region \(B\) is enclosed by the curve, the \(x\)-axis and the line \(x = 5\), and has area \({\text{0.21 unit}}{{\text{s}}^{\text{2}}}\).
Find \(g(5)\).
Answer/Explanation
Markscheme
\(p = 6\) A1 N1
recognizing that turning points occur when \(f'(x) = 0\) R1 N1
eg\(\;\;\;\)correct sign diagram
\(f’\) changes from positive to negative at \(x = 6\) R1 N1
[3 marks]
\(f'(2) = – 2\) A1 N1
[1 mark]
attempt to apply chain rule (M1)
eg\(\;\;\;\ln (x)’ \times f'(x)\)
correct expression for \(g'(x)\) (A1)
eg\(\;\;\;g'(x) = \frac{1}{{f(x)}} \times f'(x)\)
substituting \(x = 2\) into their \(g’\) (M1)
eg\(\;\;\;\frac{{f'(2)}}{{f(2)}}\)
\( – 0.666667\)
\(g'(2) = – \frac{2}{3}{\text{ (exact), }} – 0.667\) A1 N3
[4 marks]
evidence of integrating \(g'(x)\) (M1)
eg\(\;\;\;g(x)|_2^a,{\text{ }}g(x)|_a^2\)
applying the fundamental theorem of calculus (seen anywhere) R1
eg\(\;\;\;\int_2^a {g'(x) = g(a) – g(2)} \)
correct substitution into integral (A1)
eg\(\;\;\;\ln 3 + g(a) – g(2),{\text{ }}\ln 3 + g(a) – \ln \left( {f(2)} \right)\)
\(\ln 3 + g(a) – \ln 3\) A1
\(\ln 3 + \int_2^a {g'(x) = g(a)} \) AG N0
[4 marks]
METHOD 1
substituting \(a = 5\) into the formula for \(g(a)\) (M1)
eg\(\;\;\;\int_2^5 {g'(x){\text{d}}x,{\text{ }}g(5) = \ln 3 + \int_2^5 {g'(x){\text{d}}x\;\;\;} } \left( {{\text{do not accept only }}g(5)} \right)\)
attempt to substitute areas (M1)
eg\(\;\;\;\ln 3 + 0.66 – 0.21,{\text{ }}\ln 3 + 0.66 + 0.21\)
correct working
eg\(\;\;\;g(5) = \ln 3 + ( – 0.66 + 0.21)\) (A1)
\(0.648612\)
\(g(5) = \ln 3 – 0.45{\text{ (exact), }}0.649\) A1 N3
METHOD 2
attempt to set up an equation for one shaded region (M1)
eg\(\;\;\;\int_4^5 {g'(x){\text{d}}x = 0.21,{\text{ }}\int_2^4 {g'(x){\text{d}}x = – 0.66,{\text{ }}\int_2^5 {g'(x){\text{d}}x = – 0.45} } } \)
two correct equations (A1)
eg\(\;\;\;g(5) – g(4) = 0.21,{\text{ }}g(2) – g(4) = 0.66\)
combining equations to eliminate \(g(4)\) (M1)
eg\(\;\;\;g(5) – [\ln 3 – 0.66] = 0.21\)
\(0.648612\)
\(g(5) = \ln 3 – 0.45{\text{ (exact), }}0.649\) A1 N3
METHOD 3
attempt to set up a definite integral (M1)
eg\(\;\;\;\int_2^5 {g'(x){\text{d}}x = – 0.66 + 0.21,{\text{ }}\int_2^5 {g'(x){\text{d}}x = – 0.45} } \)
correct working (A1)
eg\(\;\;\;g(5) – g(2) = – 0.45\)
correct substitution (A1)
eg\(\;\;\;g(5) – \ln 3 = – 0.45\)
\(0.648612\)
\(g(5) = \ln 3 – 0.45{\text{ (exact), }}0.649\) A1 N3
[4 marks]
Total [16 marks]
Question
Note: In this question, distance is in metres and time is in seconds.
A particle moves along a horizontal line starting at a fixed point A. The velocity \(v\) of the particle, at time \(t\), is given by \(v(t) = \frac{{2{t^2} – 4t}}{{{t^2} – 2t + 2}}\), for \(0 \leqslant t \leqslant 5\). The following diagram shows the graph of \(v\)
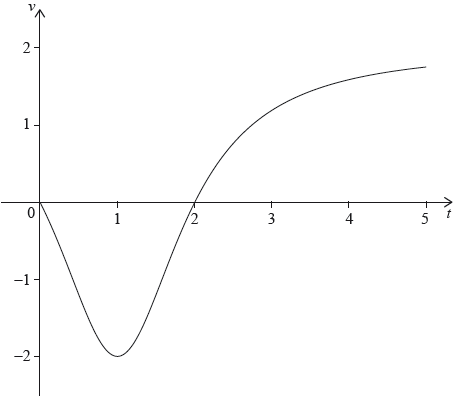
There are \(t\)-intercepts at \((0,{\text{ }}0)\) and \((2,{\text{ }}0)\).
Find the maximum distance of the particle from A during the time \(0 \leqslant t \leqslant 5\) and justify your answer.
Answer/Explanation
Markscheme
METHOD 1 (displacement)
recognizing \(s = \int {v{\text{d}}t} \) (M1)
consideration of displacement at \(t = 2\) and \(t = 5\) (seen anywhere) M1
eg\(\,\,\,\,\,\)\(\int_0^2 v \) and \(\int_0^5 v \)
Note: Must have both for any further marks.
correct displacement at \(t = 2\) and \(t = 5\) (seen anywhere) A1A1
\( – 2.28318\) (accept 2.28318), 1.55513
valid reasoning comparing correct displacements R1
eg\(\,\,\,\,\,\)\(\left| { – 2.28} \right| > \left| {1.56} \right|\), more left than right
2.28 (m) A1 N1
Note: Do not award the final A1 without the R1.
METHOD 2 (distance travelled)
recognizing distance \( = \int {\left| v \right|{\text{d}}t} \) (M1)
consideration of distance travelled from \(t = 0\) to 2 and \(t = 2\) to 5 (seen anywhere) M1
eg\(\,\,\,\,\,\)\(\int_0^2 v \) and \(\int_2^5 v \)
Note: Must have both for any further marks
correct distances travelled (seen anywhere) A1A1
2.28318, (accept \( – 2.28318\)), 3.83832
valid reasoning comparing correct distance values R1
eg\(\,\,\,\,\,\)\(3.84 – 2.28 < 2.28,{\text{ }}3.84 < 2 \times 2.28\)
2.28 (m) A1 N1
Note: Do not award the final A1 without the R1.
[6 marks]
Question
Let \(f(x) = 5 – {x^2}\). Part of the graph of \(f\)is shown in the following diagram.
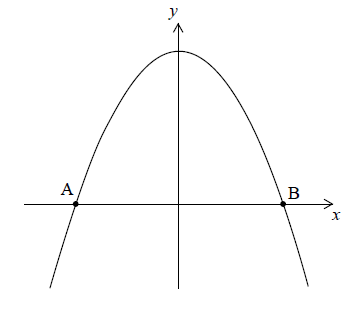
The graph crosses the \(x\)-axis at the points \(\rm{A}\) and \(\rm{B}\).
Find the \(x\)-coordinate of \({\text{A}}\) and of \({\text{B}}\).
The region enclosed by the graph of \(f\) and the \(x\)-axis is revolved \(360^\circ \) about the \(x\)-axis.
Find the volume of the solid formed.
Answer/Explanation
Markscheme
recognizing \(f(x) = 0\) (M1)
eg \(f = 0,{\text{ }}{x^2} = 5\)
\(x = \pm 2.23606\)
\(x = \pm \sqrt 5 {\text{ (exact), }}x = \pm 2.24\) A1A1 N3
[3 marks]
attempt to substitute either limits or the function into formula
involving \({f^2}\) (M1)
eg \(\pi \int {{{\left( {5 – {x^2}} \right)}^2}{\text{d}}x,{\text{ }}\pi \int_{ – 2.24}^{2.24} {\left( {{x^4} – 10{x^2} + 25} \right)} ,{\text{ }}2\pi \int_0^{\sqrt 5 } {{f^2}} } \)
\(187.328\)
volume \(= 187\) A2 N3
[3 marks]
