Question 14. [Maximum mark: 7]
A geometric transformation T : \((_{x}^{y})\rightarrow (_{x}^{y})\)
T: \((_{x}^{y})= (\frac{7}{2}\frac{-10}{-3})(_{x}^{y})+(_{-5}^{4})\)
a. Find the coordinates of the image of the point (6, −2). [2]
b. Given that T : \((_{q}^{p})\rightarrow 2(_{q}^{p})\) find the value of p and the value of q . [3]
c. A triangle L with vertices lying on the xy plane is transformed by T .
Explain why both L and its image will have exactly the same area. [2]
▶️Answer/Explanation
(a)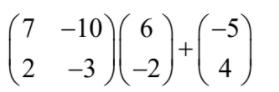 
\( =_{22}^{57}\)OR ((57, 22)\)
(b)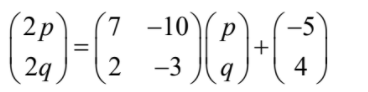 
\(7P -10Q-5=2P\)
\(2P-3Q+4 =Q\) solve simultaneously:
p = 13, q = 6
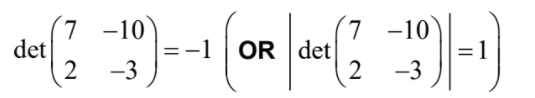scale factor of image area is therefore (| −1|=) 1 (and the translation does not affect the area)
Question
Points in the plane are subjected to a transformation T in which the point (x , y ) is transformed to the point (x’ , y’ ) where
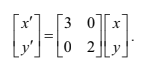
Describe, in words, the effect of the transformation T . [1]
(i) Show that the points A(1 , 4) , B(4 , 8) , C(8 , 5) , D(5 , 1) form a square.(ii) Determine the area of this square.(iii) Find the coordinates of A‘ , B‘ , C‘ , D‘ , the points to which A, B , C , D are transformed under T .
(iv) Show that A‘ B‘ C‘ D‘ is a parallelogram.
(v) Determine the area of this parallelogram. [11]
▶️Answer/Explanation
Ans:
- (a) a stretch of scale factor 3 in the x direction and a stretch of scale factor 2 in the y direction
- (b)
- (i)
- the four sides are equal in length (5)
- Grad AB = \(\frac{4}{3}\) Grad BC = -\(\frac{4}{3}\)
- so product of gradients = – 1, therefore AB is perpendicular to BC
- therefore ABCD is a square AG
- (ii) area of square = 25
- (iii) the transformed points are A’ (3,8) B’ (12,16) C’ (24,10) D’ (15,2)
- (i)
- (iv)
- Grad A’B’ = \(\frac{8}{9}\) Grad C’D’ = \(\frac{8}{9}\)
- therefore A’B’ is parallel to C’D’
- Grad A’D’ = -\(\frac{6}{12}\) Grad B’C’ = -\(\frac{6}{12}\)
- therefore A’D’ is parallel to B’C’
- therefore A’ B ‘C’ D’ is a parallelogram
- (v) area of parallelogram = |determinant | × area of square = 6 × 25 = 150
Question
The transformations T1, T2, T3, T4, in the plane are defined as follows:
T1 : A rotation of 360° about the origin
T2 : An anticlockwise rotation of 270° about the origin
T3 : A rotation of 180° about the origin
T4 : An anticlockwise rotation of 90° about the origin.
The transformation T5 is defined as a reflection in the \(x\)-axis.
The transformation T is defined as the composition of T3 followed by T5 followed by T4.
a.Copy and complete the following Cayley table for the transformations of T1, T2, T3, T4, under the operation of composition of transformations.
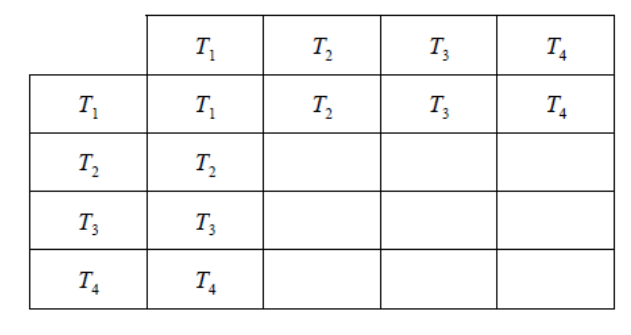[2]
b.i.Show that T1, T2, T3, T4 under the operation of composition of transformations form a group. Associativity may be assumed.[3]
b.ii.Show that this group is cyclic.[1]
c.Write down the 2 × 2 matrices representing T3, T4 and T5.[3]
d.i.Find the 2 × 2 matrix representing T.[2]
d.ii.Give a geometric description of the transformation T.[1]
▶️Answer/Explanation
Markscheme
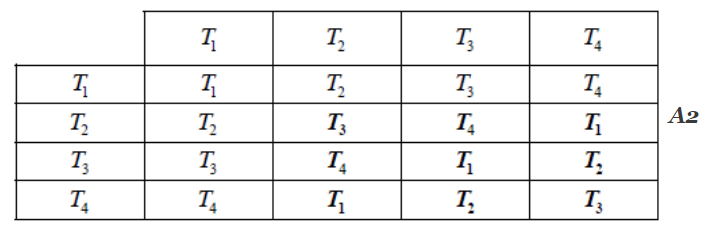
[2 marks]
Note: Award A1 for 6, 7 or 8 correct.
the table is closed – no new elements A1
T1 is the identity A1
T3 (and T1) are self-inverse; T2 and T4 are an inverse pair. Hence every element has an inverse A1
hence it is a group AG
[3 marks]
all elements in the group can be generated by T2 (or T4) R1
hence the group is cyclic AG
[1 mark]
T3 is represented by \(\left( {\begin{array}{*{20}{c}}
{ – 1}&0 \\
0&{ – 1}
\end{array}} \right)\) A1
T4 is represented by \(\left( {\begin{array}{*{20}{c}}
0&{ – 1} \\
1&0
\end{array}} \right)\) A1
T5 is represented by \(\left( {\begin{array}{*{20}{c}}
1&0 \\
0&{ – 1}
\end{array}} \right)\) A1
[3 marks]
\(\left( {\begin{array}{*{20}{c}}
0&{ – 1} \\
1&0
\end{array}} \right)\left( {\begin{array}{*{20}{c}}
1&0 \\
0&{ – 1}
\end{array}} \right)\left( {\begin{array}{*{20}{c}}
{ – 1}&0 \\
0&{ – 1}
\end{array}} \right) = \left( {\begin{array}{*{20}{c}}
0&{ – 1} \\
{ – 1}&0
\end{array}} \right)\) (M1)A1
Note: Award M1A0 for multiplying the matrices in the wrong order.
[2 marks]
a reflection in the line \(y = – x\) A1
[1 mark]
Question
The hyperbola with equation \({x^2} – 4xy – 2{y^2} = 3\) is rotated through an acute anticlockwise angle \(\alpha \) about the origin.
a.The point \((x,{\text{ }}y)\) is rotated through an anticlockwise angle \(\alpha \) about the origin to become the point \((X,{\text{ }}Y)\). Assume that the rotation can be represented by
\[\left[ {\begin{array}{*{20}{c}} X \\ Y \end{array}} \right] = \left[ {\begin{array}{*{20}{c}} a&b \\ c&d \end{array}} \right]\left[ {\begin{array}{*{20}{c}} x \\ y \end{array}} \right].\]
Show, by considering the images of the points \((1,{\text{ }}0)\) and \((0,{\text{ }}1)\) under this rotation that
\[\left[ {\begin{array}{*{20}{c}} a&b \\ c&d \end{array}} \right] = \left[ {\begin{array}{*{20}{l}} {\cos \alpha }&{ – \sin \alpha } \\ {\sin \alpha }&{\cos \alpha } \end{array}} \right].\][3]
b.i.By expressing \((x,{\text{ }}y)\) in terms of \((X,{\text{ }}Y)\), determine the equation of the rotated hyperbola in terms of \(X\) and \(Y\).[3]
b.ii.Verify that the coefficient of \(XY\) in the equation is zero when \(\tan \alpha = \frac{1}{2}\).[3]
b.iii.Determine the equation of the rotated hyperbola in this case, giving your answer in the form \(\frac{{{X^2}}}{{{A^2}}} – \frac{{{Y^2}}}{{{B^2}}} = 1\).[3]
b.iv.Hence find the coordinates of the foci of the hyperbola prior to rotation.[5]
▶️Answer/Explanation
Markscheme
consider \(\left[ {\begin{array}{*{20}{c}} a&b \\ c&d \end{array}} \right]\left[ {\begin{array}{*{20}{c}} 1 \\ 0 \end{array}} \right] = \left[ {\begin{array}{*{20}{c}} a \\ c \end{array}} \right]\) (M1)
the image of \((1,{\text{ }}0)\) is \((\cos \alpha ,{\text{ }}\sin \alpha )\) A1
therefore \(a = \cos \alpha ,{\text{ }}c = \sin \alpha \) AG
consider \(\left[ {\begin{array}{*{20}{c}} a&b \\ c&d \end{array}} \right]\left[ {\begin{array}{*{20}{c}} 0 \\ 1 \end{array}} \right] = \left[ {\begin{array}{*{20}{c}} b \\ d \end{array}} \right]\)
the image of \((0,{\text{ }}1)\) is \(( – \sin \alpha ,{\text{ }}\cos \alpha )\) A1
therefore \(b = – \sin \alpha ,{\text{ }}d = \cos \alpha \) AG
[3 marks]
\(\left[ {\begin{array}{*{20}{c}} X \\ Y \end{array}} \right] = \left[ {\begin{array}{*{20}{l}} {\cos \alpha }&{ – \sin \alpha } \\ {\sin \alpha }&{\cos \alpha } \end{array}} \right]\left[ {\begin{array}{*{20}{c}} x \\ y \end{array}} \right] \Rightarrow \left[ {\begin{array}{*{20}{c}} {\cos \alpha }&{\sin \alpha } \\ { – \sin \alpha }&{\cos \alpha } \end{array}} \right]\left[ {\begin{array}{*{20}{c}} X \\ Y \end{array}} \right]\)
or \(x = X\cos \alpha + Y\sin \alpha ,{\text{ }}y = – X\sin \alpha + Y\cos \alpha \) A1
substituting in the equation of the hyperbola, M1
\({(X\cos \alpha + Y\sin \alpha )^2} – 4(X\cos \alpha + Y\sin \alpha )( – X\sin \alpha + Y\cos \alpha )\)
\( – 2{( – X\sin \alpha + Y\cos \alpha )^2} = 3\) A1
\({X^2}({\cos ^2}\alpha – 2{\sin ^2}\alpha + 4\sin \alpha \cos \alpha ) + \)
\(XY(2\sin \alpha \cos \alpha – 4{\cos ^2}\alpha + 4{\sin ^2}\alpha + 4\sin \alpha \cos \alpha ) + \)
\({Y^2}({\sin ^2}\alpha – 2{\cos ^2}\alpha – 4\sin \alpha \cos \alpha ) = 3\)
[??? marks]
when \(\tan \alpha = \frac{1}{2},{\text{ }}\sin \alpha = \frac{1}{{\sqrt 5 }}\) and \(\cos \alpha = \frac{2}{{\sqrt 5 }}\) A1
the \(XY{\text{ term}} = 6\sin \alpha \cos \alpha – 4{\cos ^2}\alpha + 4{\sin ^2}\alpha \) M1
\( = 6 \times \frac{1}{{\sqrt 5 }} \times \frac{2}{{\sqrt 5 }} – 4 \times \frac{4}{5} + 4 \times \frac{1}{5}\left( {\frac{{12}}{5} – \frac{{16}}{5} + \frac{4}{5}} \right)\) A1
\( = 0\) AG
[??? marks]
the equation of the rotated hyperbola is
\(2{X^2} – 3{Y^2} = 3\) M1A1
\(\frac{{{X^2}}}{{{{\left( {\sqrt {\frac{3}{2}} } \right)}^2}}} – \frac{{{Y^2}}}{{{{(1)}^2}}} = 1\) A1
\(\left( {{\text{accept }}\frac{{{X^2}}}{{\frac{3}{2}}} – \frac{{{Y^2}}}{1} = 1} \right)\)
[??? marks]
the coordinates of the foci of the rotated hyperbola
are \(\left( { \pm \sqrt {\frac{3}{2} + 1} ,{\text{ }}0} \right) = \left( { \pm \sqrt {\frac{5}{2}} ,{\text{ }}0} \right)\) M1A1
the coordinates of the foci prior to rotation were given by
\(\left[ {\begin{array}{*{20}{c}} {\frac{2}{{\sqrt 5 }}}&{\frac{1}{{\sqrt 5 }}} \\ { – \frac{1}{{\sqrt 5 }}}&{\frac{2}{{\sqrt 5 }}} \end{array}} \right]\left[ {\begin{array}{*{20}{c}} { \pm \sqrt {\frac{5}{2}} } \\ 0 \end{array}} \right]\)
M1A1
\(\left[ {\begin{array}{*{20}{c}} { \pm \sqrt 2 } \\ { \mp \frac{1}{{\sqrt 2 }}} \end{array}} \right]\) A1
[??? marks]