Question 11. [Maximum mark: 6]
A newspaper vendor in Singapore is trying to predict how many copies of The Straits Times they will sell. The vendor forms a model to predict the number of copies sold each weekday. According to this model, they expect the same number of copies will be sold each day.
To test the model, they record the number of copies sold each weekday during a particular week. This data is shown in the table.
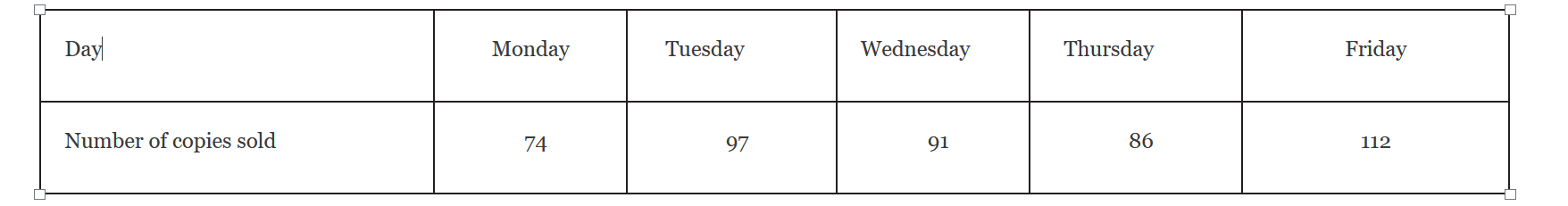
A goodness of fit test at the 5 % significance level is used on this data to determine whether the vendor’s model is suitable.
The critical value for the test is 9.49 and the hypotheses are
H0 : The data satisfies the model.
H1 : The data does not satisfy the model.
Find an estimate for how many copies the vendor expects to sell each day. [1]
(i) Write down the degrees of freedom for this test.
(ii) Write down the conclusion to the test. Give a reason for your answer. [5]
Answer/Explanation
(a) \((\frac{74+97+91+86+112}{5})=92\)
(b) (i) 4 (ii) 2 χ2calc = 8.54 (8.54347…) OR \(p-value = 0.0736 (0.0735802…)\) 8.54 < 9.49 OR 0.0736 > 0.05
therefore there is insufficient evidence to reject \(H_{0}\) (i.e. the data satisfies the model)
Question 8 [Maximum mark: 6]
At Springfield University, the weights, in kg, of 10 chinchilla rabbits and 10 sable rabbits were recorded. The aim was to find out whether chinchilla rabbits are generally heavier than sable rabbits. The results obtained are summarized in the following table.
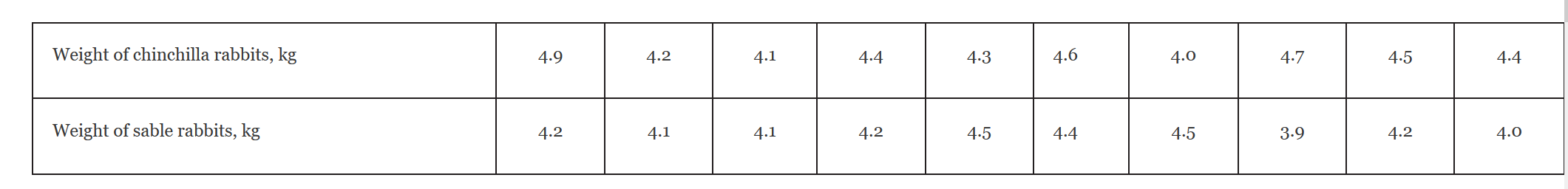
A t-test is to be performed at the 5 % significance level.
a. Write down the null and alternative hypotheses. [2]
b. Find the p-value for this test. [2]
c. Write down the conclusion to the test. Give a reason for your answer. [2]
Answer/Explanation
(a) (let μc = population mean for chinchilla rabbits, μs = population mean for sable rabbits) Ho : μc= μs = \(H_{1}\) : μc > μs
(b) \(p- value = 0.0408 (0.0408065…)\)
(c) \(0.0408 < 0.05\) (there is sufficient evidence to) reject (or not accept) \(H_{0}\) (there is sufficient evidence to suggest that chinchilla rabbits are (generally) heavier than sable rabbits)
Question 6. [Maximum mark: 5]
Arriane has geese on her farm. She claims the mean weight of eggs from her black geese is less than the mean weight of eggs from her white geese.
She recorded the weights of eggs, in grams, from a random selection of geese. The data is shown in the table.
Weights of eggs from black geese | 136 | 134 | 142 | 141 | 128 | 126 |
Weights of eggs from white geese | 135 | 138 | 141 | 140 | 136 | 134 |
In order to test her claim, Arriane performs a t-test at a 10 % level of significance. It is assumed that the weights of eggs are normally distributed and the samples have equal variances.
(a)State, in words, the null hypothesis. [1]
(b)Calculate the p-value for this test. [2]
(c)State whether the result of the test supports Arriane’s claim. Justify your reasoning. [2]
Answer/Explanation
(a) EITHER \(H_{0}\): The population mean weight of eggs from (her/the) black geese is equal to/the same as the population mean weight of eggs from (her/the) white geese. OR
\(H_{0}:\) The population mean weight of eggs from (her/the) black geese is not less than the population mean weight of eggs from (her/the) white geese.
(b) \(p-value = 0.177 (0.176953…)\)
(c) 0.177 > 0.1 (insufficient evidence to reject \(H_{0}\) ) Arriane’s claim is not supported by the evidence
Question
A factory produces bags of sugar with a labelled weight of 500g. The weights of the bags
are normally distributed with a mean of 500g and a standard deviation of 3g.
(a) Write down the percentage of bags that weigh more than 500 g.
A bag that weighs less than 495g is rejected by the factory for being underweight.
(b) Find the probability that a randomly chosen bag is rejected for being underweight.
A bag that weighs more than k grams is rejected by the factory for being overweight.
The factory rejects 2% of bags for being overweight.
(c) Find the value of k .
Answer/Explanation
Ans:
(a) 50%
(b) 0.0478 (0.0477903…, 4.78%)
(c) P(X<k)=0.98 OR P(X>k)=0.02
506g (506.161…)
Question
Leo is investigating whether a six-sided die is fair. He rolls the die 60 times and records the
observed frequencies in the following table:
| Number on die | 1 | 2 | 3 | 4 | 5 | 6 |
| Observed frequency | 8 | 7 | 6 | 15 | 12 | 12 |
Leo carries out a \(X^2\) goodness of fit test at a 5% significance level.
(a) Write down the null and alternative hypotheses.
(b) Write down the degrees of freedom.
(c) Write down the expected frequency of rolling a 1.
(d) Find the p-value for the test.
(e) State the conclusion of the test. Give a reason for your answer.
Answer/Explanation
Ans:
(a) \(H_o\) : The die is fair OR P(any number) = \(\frac{1}{6}\) OR probabilities are equal
\(H_1\) : The die is not fair OR P(any number ) \(\neq = \frac{1}{6}\) OR probabilities are not equal
(b) 5
(c) 10
(d) (p-value=) 0.287 > 0.05
EITHER
Insufficient evidence to reject the null hypothesis
OR
Insufficient evidence to reject that the die is fair.
