Question
(a) Factorise 9y + 12 .
(b) Expand a(a2– 7).
▶️Answer/Explanation
(a) To factorize 9y + 12, we look for the greatest common factor (GCF) of the terms. In this case, both terms are divisible by 3. Thus, we can factor out 3:
\(9y + 12 = 3(3y + 4)\)
So, the factorized form of 9y + 12 is \(3(3y + 4).\)
(b) To expand \(a(a^2 – 7)\), we apply the distributive property. We multiply ‘a’ by each term inside the parentheses:
\(a(a^2 – 7) = a * a^2 – a ^ 7\)
\(a(a^2 – 7) = a^3 – 7a\)
Therefore, the expanded form of \(a(a^2 – 7) \) is \(a^3 – 7a.\)
Question
Simplify the following expression.
3j – 4k – 2 + 5j + k – 6
▶️Answer/Explanation
To simplify the expression 3j – 4k – 2 + 5j + k – 6, we can combine like terms. Like terms have the same variables raised to the same powers.
Grouping the like terms together,
(3j + 5j) + (-4k + k) + (-2 – 6)
Combining the coefficients of the like terms:
8j – 3k – 8
Therefore, the simplified form of the expression 3j – 4k – 2 + 5j + k – 6 is 8j – 3k – 8.
Question
Triangle ABC has sides AB = 40m, BC = 25m and AC = 35m.
Using a scale of 1 cm to represent 5m, construct triangle ABC.
The construction must be completed using a ruler and compasses only.
All construction arcs must be clearly shown.
▶️Answer/Explanation
To construct triangle ABC with the given side lengths AB = 40m, BC = 25m, and AC = 35m using a scale of 1 cm to represent 5m, follow the steps below:
Draw a line segment AB = 8 cm (1 cm represents 5m) in the desired direction.
From point A, draw an arc with radius 7 cm (7 cm = 35m) to intersect the line segment AB.
From point B, draw an arc with radius 5 cm (5 cm = 25m) to intersect the line segment AB.
Label the point of intersection between the arc and the line segment as C.
Draw the line segments AC and BC to complete triangle ABC.
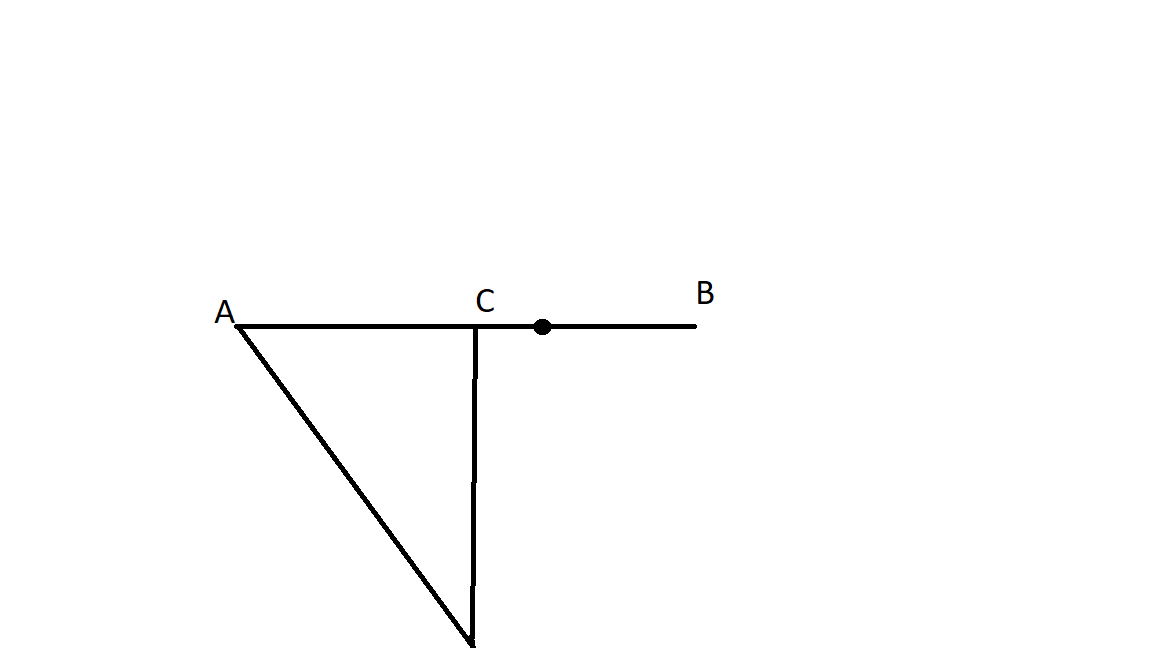
Now, you have constructed triangle ABC with the given side lengths.
Question
(a) Factorise completely.
6ab – 24bc
(b) Rearrange the following formula to make m the subject.
\(j = \frac{m}{n} – k\)
▶️Answer/Explanation
(a) To factorize completely the expression 6ab – 24bc, we can find the greatest common factor (GCF) among the terms and factor it out. In this case, both terms have a common factor of 6b. Thus, we can factor out 6b:
6ab – 24bc = 6b(a – 4c)
So, the completely factorized form of 6ab – 24bc is 6b(a – 4c).
(b)To rearrange the formula\( j = \frac{m}{n} – k\) to make m the subject, we can follow these steps:
Begin with the given formula:
\(j= \frac{m}{n} – k\)
\(j + k = \frac{m}{n}\)
\(n(j + k) = m\)
\(m = n(j + k)\)
So, the rearranged formula with m as the subject is\( m = n(j + k).\)
Question
Simplify the following expression.
5a – 3b – 2a – b
▶️Answer/Explanation
To simplify the expression 5a – 3b – 2a – b, we can combine like terms. Like terms have the same variables raised to the same powers.
(5a – 2a) + (-3b – b)
3a – 4b
Therefore, the simplified form of the expression 5a – 3b – 2a – b is 3a – 4b.
