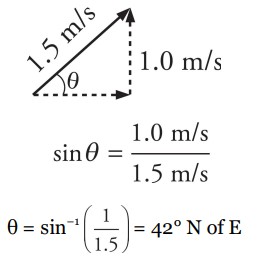Question(a)
Questions (a)–(c) refer to the following scenario:

An explorer travels 30 m east, then 20\(\sqrt{2}\) m in a direction 45° south of east, and then 140 m north.
What is the distance traveled by the explorer?
(A) 167.2 m
(B) 169 m
(C) 170 m
(D) 198.2 m
▶️Answer/Explanation
Ans:D
The total distance traveled is equal to the sum of the individual distances traveled by the explorer:
d = 30 m + 20\(\sqrt{2}\) m + 140 m = 198.2 m.
Question(b)
What is the displacement of the explorer?
(A) 130 m
(B) 169 m
(C) 170 m
(D) 215 m
▶️Answer/Explanation
Ans:A
The displacement is equal to the change in position of the explorer. The horizontal and vertical components of the explorer’s displacement can be calculated as follows:
Δx = 30 m + 20 cos45° = 50 m
Δy = −20 sin45° + 140 m = 120 m
The displacement of the explorer then is the magnitude of the vector (50i + 120j) m:
\(\Delta s=\sqrt{(\Delta x)^{2}+(\Delta y)^{2}}=\sqrt{(50m)^{2}+(120m)^{2}}=130m\)
Question(c)
The explorer took 60 s, 130 s, and 70 s to travel the 30 m, 20\(\sqrt{2}\) m, and 140 m north distances, respectively. What is the average velocity of the explorer over the total distance traveled?
(A) 0.50 m/s
(B) 33.3 m/min
(C) 0.76 m/s
(D) 100 m/min
▶️Answer/Explanation
Ans:A
As the displacement of the explorer was 130 m, the average velocity of the explorer is equal to

Question
A section of a river flows with a velocity of 1 m/s due S. A kayaker who is able to propel her kayak at 1.5 m/s wishes to paddle directly east one bank to the other. In what direction should she direct her kayak?
(A) 37° N of E
(B) 42° N of E
(C) 45° N of E
(D) 48° N of E
▶️Answer/Explanation
Ans:B
The kayaker’s resultant velocity, \(v\), will be the sum of the river’s velocity, \(v_{r}\), and the velocity of her paddling, \(v_{p}\):
\(v\) = \(v_{r}\) + \(v_{p}\)
Since the kayaker wishes to travel due east, the component of vp in the N-S direction must cancel vr, so vp,N-S = 1° m/s due N. Since the magnitude of vp is 1.5 m/s, her heading can be calculated using