Download IITian Academy App for accessing Online Mock Tests and More..
Question
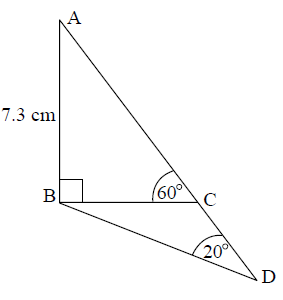
Triangle \({\text{ABC}}\) is drawn such that angle \({\text{ABC}}\) is \({90^ \circ }\), angle \({\text{ACB}}\) is \({60^ \circ }\) and \({\text{AB}}\) is \(7.3{\text{ cm}}\).
(i) Sketch a diagram to illustrate this information. Label the points \({\text{A, B, C}}\). Show the angles \({90^ \circ }\), \({60^ \circ }\) and the length \(7.3{\text{ cm}}\) on your diagram.
(ii) Find the length of \({\text{BC}}\).[3]
Point \({\text{D}}\) is on the straight line \({\text{AC}}\) extended and is such that angle \({\text{CDB}}\) is \({20^ \circ }\).
(i) Show the point \({\text{D}}\) and the angle \({20^ \circ }\) on your diagram.
(ii) Find the size of angle \({\text{CBD}}\).[3]
Answer/Explanation
Markscheme
Unit penalty (UP) is applicable where indicated in the left hand column.
(i)
(A1)
For \({\text{A}}\), \({\text{B}}\), \({\text{C}}\), \(7.3\), \({60^ \circ }\), \({90^ \circ }\), shown in correct places (A1)
Note: The \({90^ \circ }\) should look like \({90^ \circ }\) (allow \( \pm {10^ \circ }\))
(ii) Using \(\tan 60\) or \(\tan 30\) (M1)
(UP) \(4.21{\text{ cm}}\) (A1)(ft)
Note: (ft) on their diagram
Or
Using sine rule with their correct values (M1)
(UP) \( = 4.21{\text{ cm}}\) (A1)(ft)
Or
Using special triangle \(\frac{{7.3}}{{\sqrt 3 }}\) (M1)
(UP) \(4.21{\text{ cm}}\) (A1)(ft)
Or
Any other valid solution
Note: If A and B are swapped then \({\text{BC}} = 8.43{\text{ cm}}\) (C3)[3 marks]
(i) For \({\text{ACD}}\) in a straight line and all joined up to \({\text{B}}\), for \({20^ \circ }\) shown in correct place and \({\text{D}}\) labelled. \({\text{D}}\) must be on \({\text{AC}}\) extended. (A1)
(ii) \({\text{B}}\hat {\text{C}}{\text{D}} = {120^ \circ }\) (A1)
\({\text{C}}\hat {\text{B}}{\text{D}} = {40^ \circ }\) (A1) (C3)[3 marks]
Question
A and B are points on a straight line as shown on the graph below.
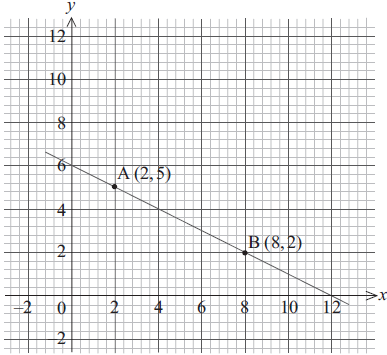
Write down the y-intercept of the line AB.[1]
Calculate the gradient of the line AB.[2]
The acute angle between the line AB and the x-axis is θ.
Show θ on the diagram.[1]
The acute angle between the line AB and the x-axis is θ.
Calculate the size of θ.[2]
Answer/Explanation
Markscheme
6
OR
(0, 6) (A1) (C1)[1 mark]
\(\frac{{(2 – 5)}}{{(8 – 2)}}\) (M1)
Note: Award (M1) for substitution in gradient formula.
\( = – \frac{1}{2}\) (A1) (C2)[2 marks]
Angle clearly identified. (A1) (C1)[1 mark]
\(\tan \theta = \frac{1}{2}\) (or equivalent fraction) (M1)
\(\theta = 26.6^\circ\) (A1)(ft) (C2)
Note: (ft) from (b).
Accept alternative correct trigonometrical methods.[2 marks]
Question
José stands 1.38 kilometres from a vertical cliff.
Express this distance in metres.[1]
José estimates the angle between the horizontal and the top of the cliff as 28.3° and uses it to find the height of the cliff.
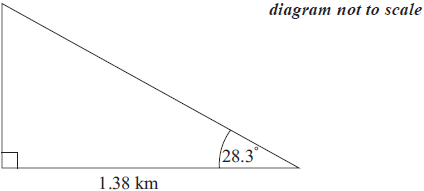
Find the height of the cliff according to José’s calculation. Express your answer in metres, to the nearest whole metre.[3]
José estimates the angle between the horizontal and the top of the cliff as 28.3° and uses it to find the height of the cliff.
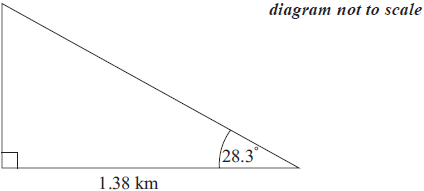
The actual height of the cliff is 718 metres. Calculate the percentage error made by José when calculating the height of the cliff.[2]
Answer/Explanation
Markscheme
1380 (m) (A1) (C1)[1 mark]
\(1380\tan 28.3\) (M1)
\( = 743.05 \ldots \) . (A1)(ft)
\( = 743\) (m) (A1)(ft) (C3)
Notes: Award (M1) for correct substitution in tan formula or equivalent, (A1)(ft) for their 743.05 seen, (A1)(ft) for their answer correct to the nearest m.[3 marks]
\({\text{percentage error}} = \frac{{743.05 \ldots – 718}}{{718}} \times 100\) (M1)
Note: Award (M1) for correct substitution in formula.
= 3.49 % (% symbol not required) (A1)(ft) (C2)
Notes: Accept 3.48 % for use of 743.
Accept negative answer.[2 marks]
Question
In the diagram, \({\text{AD}} = 4{\text{ m}}\), \({\text{AB}} = 9{\text{ m}}\), \({\text{BC}} = 10{\text{ m}}\), \({\text{B}}\hat {\text{D}}{\text{A}} = {90^ \circ }\) and \({\text{D}}\hat {\text{B}}{\text{C}} = {100^ \circ }\) .
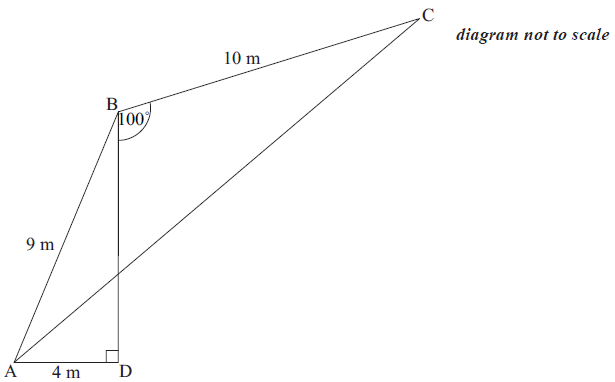
Calculate the size of \({\text{A}}\hat {\text{B}}{\text{C}}\).[3]
Calculate the length of AC.[3]
Answer/Explanation
Markscheme
\(\sin {\text{A}}\hat {\text{B}}{\text{D}} = \frac{4}{9}\) (M1)
\(100 + {\text{their }}({\text{A}}\hat {\text{B}}{\text{D}})\) (M1)
\(126\% \) (A1) (C3)
Notes: Accept an equivalent trigonometrical equation involving angle ABD for the first (M1).
Radians used gives \(100\% \) . Award at most (M1)(M1)(A0) if working shown.
\({\text{BD}} = 8{\text{ m}}\) leading to \(127\% \) . Award at most (M1)(M1)(A0) (premature rounding).[3 marks]
\({\text{A}}{{\text{C}}^2} = {10^2} + {9^2} – 2 \times 10 \times 9 \times \cos (126.38 \ldots )\) (M1)(A1)
Notes: Award (M1) for substituted cosine formula. Award (A1) for correct substitution using their answer to part (a).
\({\text{AC}} = 17.0{\text{ m}}\) (A1)(ft) (C3)
Notes: Accept \(16.9{\text{ m}}\) for using \(126\). Follow through from their answer to part (a). Radians used gives \(5.08\). Award at most (M1)(A1)(A0)(ft) if working shown.[3 marks]
Question
In triangle \({\text{ABC}}\), \({\text{AC}} = 20 {\text{ cm}}\), \({\text{BC}} = 12 {\text { cm}}\) and \({\rm{A\hat BC}} = 90^\circ\).
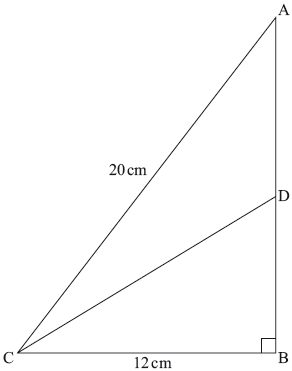diagram not to scale
Find the length of \({\text{AB}}\).[2]
\({\text{D}}\) is the point on \({\text{AB}}\) such that \(\tan ({\rm{D\hat CB}}) = 0.6\).
Find the length of \({\text{DB}}\).[2]
\({\text{D}}\) is the point on \({\text{AB}}\) such that \(\tan ({\rm{D\hat CB}}) = 0.6\).
Find the area of triangle \({\text{ADC}}\).[2]
Answer/Explanation
Markscheme
\(({\text{A}}{{\text{B}}^2}) = {20^2} – {12^2}\) (M1)
Note: Award (M1) for correctly substituted Pythagoras formula.
\({\text{AB}} = 16{\text{ cm}}\) (A1) (C2)[2 marks]
\(\frac{{{\text{DB}}}}{{12}} = 0.6\) (M1)
Note: Award (M1) for correct substitution in tangent ratio or equivalent ie seeing \(12 \times 0.6\).
\({\text{DB}} = 7.2{\text{ cm}}\) (A1) (C2)
Note: Award (M1)(A0) for using \(\tan 31\) to get an answer of \(7.21\).
Award (M1)(A0) for \(\frac{{12}}{{\sin 59}} = \frac{{{\text{DB}}}}{{\sin 31}}\) to get an answer of \(7.2103…\) or any other incorrect answer.[2 marks]
\(\frac{1}{2} \times 12 \times (16 – 7.2)\) (M1)
Note: Award (M1) for their correct substitution in triangle area formula.
OR
\(\frac{1}{2} \times 12 \times 16 – \frac{1}{2} \times 12 \times 7.2\) (M1)
Note: Award (M1) for subtraction of their two correct area formulas.
\( = 52.8{\text{ c}}{{\text{m}}^2}\) (A1)(ft) (C2)
Notes: Follow through from parts (a) and (b).
Accept alternative methods.[2 marks]
Question
A child’s wooden toy consists of a hemisphere, of radius 9 cm , attached to a cone with the same base radius. O is the centre of the base of the cone and V is vertically above O.
Angle OVB is \({27.9^ \circ }\).
Diagram not to scale.
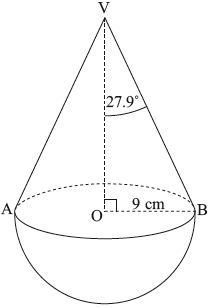
Calculate OV, the height of the cone.[2]
Calculate the volume of wood used to make the toy.[4]
Answer/Explanation
Markscheme
\(\tan 27.9^\circ = \frac{9}{{{\text{OV}}}}\) (M1)
Note: Award (M1) for correct substitution in trig formula.
\({\text{OV}} = 17.0\left( {{\text{cm}}} \right)\left( {16.9980 \ldots } \right)\) (A1) (C2)[2 marks]
\(\frac{{\pi {{(9)}^2}(16.9980 \ldots )}}{3} + \frac{1}{2} \times \frac{{4\pi {{(9)}^3}}}{3}\) (M1)(M1)(M1)
Note: Award (M1) for correctly substituted volume of the cone, (M1) for correctly substituted volume of a sphere divided by two (hemisphere), (M1) for adding the correctly substituted volume of the cone to either a correctly substituted sphere or hemisphere.
\( = 2970{\text{ c}}{{\text{m}}^3}{\text{ (2968.63}} \ldots {\text{)}}\) (A1)(ft) (C4)
Note: The answer is \(2970{\text{ c}}{{\text{m}}^3}\), the units are required.[4 marks]
Question
Günter is at Berlin Tegel Airport watching the planes take off. He observes a plane that is at an angle of elevation of \(20^\circ\) from where he is standing at point \({\text{G}}\). The plane is at a height of 350 metres. This information is shown in the following diagram.
Calculate the horizontal distance, \({\text{GH}}\), of the plane from Günter. Give your answer to the nearest metre.[3]
The plane took off from a point \({\text{T}}\), which is \(250\) metres from where Günter is standing, as shown in the following diagram.
Using your answer from part (a), calculate the angle \({\text{ATH}}\), the takeoff angle of the plane.[3]
Answer/Explanation
Markscheme
\(\frac{{350}}{{\tan 20^\circ }}\) (M1)
\( = {\text{961.617}} \ldots \) (A1)
\( = 962{\text{ (m)}}\) (A1)(ft) (C3)
Notes: Award (M1) for correct substitution into correct formula, (A1) for correct answer, (A1)(ft) for correct rounding to the nearest metre.
Award (M0)(A0)(A0) for \(961\) without working.[3 marks]
\({\text{961.617}} \ldots – 250 = {\text{711.617}} \ldots \) (A1)(ft)
\({\tan ^{ – 1}}\left( {\frac{{350}}{{{\text{711.617}} \ldots }}} \right)\) (M1)
\( = {\text{26.2}}^\circ {\text{ (26.1896}} \ldots {\text{)}}\) (A1)(ft) (C3)
Notes: Accept \(26.1774…\) from use of 3 sf answer \(962\) from part (a). Follow through from their answer to part (a).
Accept alternative methods.[3 marks]
Question
Fabián stands on top of a building, T, which is on a horizontal street.
He observes a car, C, on the street, at an angle of depression of 30°. The base of the building is at B. The height of the building is 80 metres.
The following diagram indicates the positions of T, B and C.
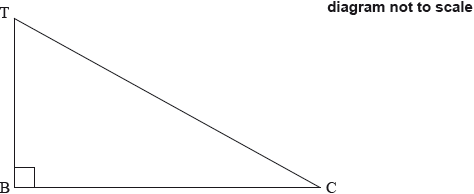
Show, in the appropriate place on the diagram, the values of
(i) the height of the building;
(ii) the angle of depression.[2]
Find the distance, BC, from the base of the building to the car.[2]
Fabián estimates that the distance from the base of the building to the car is 150 metres. Calculate the percentage error of Fabián’s estimate.[2]
Answer/Explanation
Markscheme
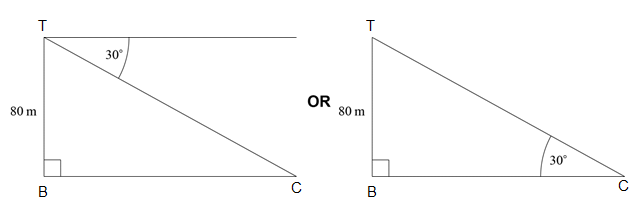(A1)(A1) (C2)
Notes: Award (A1) for 80 m in the correct position on diagram.
Award (A1) for 30° in a correct position on diagram.
\(\tan 30^\circ = \frac{{80}}{{{\text{BC}}}}\;\;\;\)OR\(\;\;\;\tan 60^\circ = \frac{{{\text{BC}}}}{{80}}\;\;\;\)OR\(\;\;\;\frac{{80}}{{\sin 30^\circ }} = \frac{{{\text{BC}}}}{{\sin 60^\circ }}\) (M1)
Note: Award (M1) for a correct trigonometric or Pythagorean equation for BC with correctly substituted values.
\(({\text{BC}} = ){\text{ 139 (m)}}\;\;\;\left( {138.564 \ldots {\text{ (m)}}} \right)\) (A1)(ft) (C2)
Notes: Accept an answer of \(80\sqrt 3 \) which is the exact answer.
Follow through from part (a).
Do not penalize use of radians unless it leads to a negative answer.
\(\left| {\frac{{150 – 138.564 \ldots }}{{138.564 \ldots }}} \right| \times 100\) (M1)
Notes: Award (M1) for their correct substitution into the percentage error formula.
\( = 8.25(\% )\;\;\;(8.25317 \ldots \% )\) (A1)(ft) (C2)
Notes: Accept \(7.91(\%)\) (\(7.91366…\) if \(139\) is used.
Accept \(8.23(\%)\) (\(8.22510…\) if \(138.6\) is used.
Follow through from their answer to part (b).
If answer to part (b) is \(46.2\), answer to part (c) is \(225\%\), award (M1)(A1)(ft) with or without working seen. If answer to part (b) is negative, award at most (M1)(A0).
Question
In the following diagram, ABCD is the square base of a right pyramid with vertex V. The centre of the base is O. The diagonal of the base, AC, is 8 cm long. The sloping edges are 10 cm long.
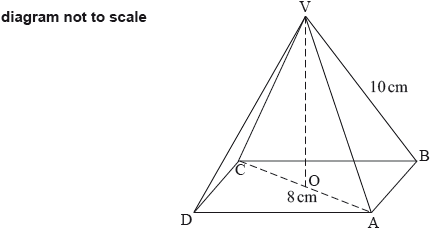
Write down the length of \({\text{AO}}\).[1]
Find the size of the angle that the sloping edge \({\text{VA}}\) makes with the base of the pyramid.[2]
Hence, or otherwise, find the area of the triangle \({\text{CAV}}\).[3]
Answer/Explanation
Markscheme
\({\text{AO}} = 4{\text{ (cm)}}\) (A1) (C1)
\(\cos {\rm{O\hat AV}} = \frac{4}{{10}}\) (M1)
Note: Award (M1) for their correct trigonometric ratio.
OR
\(\cos {\rm{O\hat AV}} = \frac{{{{10}^2} + {8^2} – {{10}^2}}}{{2 \times 10 \times 8}}\;\;\;\)OR\(\;\;\;\frac{{{{10}^2} + {4^2} – {{(9.16515 \ldots )}^2}}}{{2 \times 10 \times 4}}\) (M1)
Note: Award (M1) for correct substitution into the cosine rule formula.
\({\rm{O\hat AV}} = 66.4^\circ \;\;\;(66.4218 \ldots )\) (A1)(ft) (C2)
Notes: Follow through from their answer to part (a).
\({\text{area}} = \frac{{8 \times 10 \times \sin (66.4218 \ldots ^\circ)}}{2}\;\;\;\)OR\(\;\;\;\frac{1}{2} \times 8 \times \sqrt {{{10}^2} – {4^{\text{2}}}} \)
OR\(\;\;\;\frac{1}{2} \times 10 \times 10 \times \sin (47.1563 \ldots ^\circ )\) (M1)(A1)(ft)
Notes: Award (M1) for substitution into the area formula, (A1)(ft) for correct substitutions. Follow through from their answer to part (b) and/or part (a).
\({\text{area}} = 36.7{\text{ c}}{{\text{m}}^2}\;\;\;(36.6606 \ldots {\text{ c}}{{\text{m}}^2})\) (A1)(ft) (C3)
Notes: Accept an answer of \(8\sqrt {21} {\text{ c}}{{\text{m}}^2}\) which is the exact answer.
Question
Temi’s sailing boat has a sail in the shape of a right-angled triangle, \({\text{ABC}}{\text{.}}\,\,\,{\text{BC}} = \,\,5.45{\text{m}}\),
angle \({\text{CAB}} = {76^{\text{o}}}\) and angle \({\text{ABC}} = {90^{\text{o}}}\).
Calculate \({\text{AC}}\), the height of Temi’s sail.[2]
William also has a sailing boat with a sail in the shape of a right-angled triangle, \({\text{TRS}}\).
\({\text{RS}}\,\,{\text{ = }}\,\,{\text{2}}{\text{.80m}}\). The area of William’s sail is \({\text{10}}{\text{.7}}\,{{\text{m}}^2}\).
Calculate \({\text{RT}}\), the height of William’s sail.[2]
Answer/Explanation
Markscheme
Units are required in parts (a) and (b).
\({\text{sin}}\,\,{76^{\text{o}}} = \,\,\frac{{5.45}}{{{\text{AC}}}}\) (M1)
Note: Award (M1) for correct substitution into correct trig formula.
\({\text{AC}}\,\, = \,\,5.62{\text{m}}\,\,\,( = 5.61684…{\text{m}})\) (A1) (C2)
Note: The answer is \(5.62{\text{m}}\), the units are required. [2 marks]
\(\frac{1}{2}\,\, \times \,\,2.80\,\, \times \,\,{\text{RT}}\,\,{\text{ = }}\,\,{\text{10}}{\text{.7}}\) (M1)
Note: Award (M1) for correct substitution into area of a triangle formula or equivalent.
\({\text{RT}}\,\,{\text{ = }}\,\,{\text{7}}{\text{.64}}\,{\text{m (7}}{\text{.64285}}…{\text{m)}}\) (A1) (C2)
Note: The answer is \({\text{7}}{\text{.64}}\,{\text{m}}\), the units are required.[2 marks]
Question
The equation of line \({L_1}\) is \(y = 2.5x + k\). Point \({\text{A}}\) \(\,(3,\, – 2)\) lies on \({L_1}\).
Find the value of \(k\).[2]
The line \({L_2}\) is perpendicular to \({L_1}\) and intersects \({L_1}\) at point \({\text{A}}\).
Write down the gradient of \({L_2}\).[1]
Find the equation of \({L_2}\). Give your answer in the form \(y = mx + c\) .[2]
Write your answer to part (c) in the form \(ax + by + d = 0\) where \(a\), \(b\) and \(d \in \mathbb{Z}\).[1]
Answer/Explanation
Markscheme
\( – 2 = 2.5\, \times 3 + k\) (M1)
Note: Award (M1) for correct substitution of \((3,\, – 2)\) into equation of \({L_1}\).
\((k = ) – 9.5\) (A1) (C2)
\( – 0.4\,\left( { – \frac{2}{5}} \right)\) (A1) (C1)
\(y – ( – 2) = – 0.4\,(x – 3)\) (M1)
OR
\( – 2 = – 0.4\,(3) + c\) (M1)
Note: Award (M1) for their gradient and given point substituted into equation of a straight line. Follow through from part (b).
\(y = – 0.4x – 0.8\) \(\left( {y = – \frac{2}{5}x – \frac{4}{5}} \right)\) (A1)(ft) (C2)
\(2x + 5y + 4 = 0\) (or any integer multiple) (A1)(ft) (C1)
Note: Follow through from part (c).
Question
A ladder is standing on horizontal ground and leaning against a vertical wall. The length of the ladder is \(4.5\) metres. The distance between the bottom of the ladder and the base of the wall is \(2.2\) metres.
Use the above information to sketch a labelled diagram showing the ground, the ladder and the wall.[1]
Calculate the distance between the top of the ladder and the base of the wall.[2]
Calculate the obtuse angle made by the ladder with the ground.[3]
Answer/Explanation
Markscheme
(A1) (C1)
Notes: Award (A1) for drawing an approximately right angled triangle, with correct labelling of the distances \(4.5\,({\text{m}})\) and \(2.2\,({\text{m}})\).
\(\sqrt {\,{{4.5}^2} – {{2.2}^2}} \,\,\,({\text{accept eqivalent }}eg\,\,{d^2} + {2.2^2} = {4.5^2})\) (M1)
\( = 3.93\,({\text{m}})\,\,\,\left( {\sqrt {\,15.41} \,({\text{m}}),\,\,3.92555…\,({\text{m}})} \right)\) (A1) (C2)
Note: Award (M1) for a correct substitution in the Pythagoras formula.
\(180^\circ – {\cos ^{ – 1}}\left( {\frac{{2.2}}{{4.5}}} \right)\) (M1)(M1)
OR
\(180^\circ – {\tan ^{ – 1}}\left( {\frac{{3.92555…}}{{2.2}}} \right)\) (M1)(M1)
OR
\(180^\circ – {\sin ^{ – 1}}\left( {\frac{{3.92555…}}{{4.5}}} \right)\) (M1)(M1)
Note: Award (M1) for a correct substitution in the correct trigonometric ratio.
Award (M1) for subtraction from \(180^\circ \) (this may be implied if the sum of their inverse of the trigonometric ratio and their final answer equals \(180\)).
\( = 119^\circ \,\,\,(119.267…^\circ )\) (A1)(ft) (C3)
Note: Follow through from their part (b) if cosine is not used. Accept \(119.239…\) or \(119.151…\) from use of \(3\) sf values.
Question
AC is a vertical communications tower with its base at C.
The tower has an observation deck, D, three quarters of the way to the top of the tower, A.
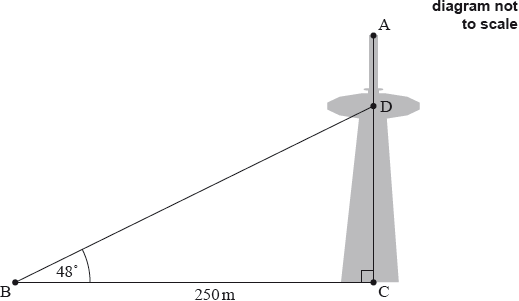
From a point B, on horizontal ground 250 m from C, the angle of elevation of D is 48°.
Calculate CD, the height of the observation deck above the ground.[2]
Calculate the angle of depression from A to B.[4]
Answer/Explanation
Markscheme
\(\tan 48^\circ = \frac{{{\text{CD}}}}{{250}}\) (M1)
Note: Award (M1) for correct substitution into the tangent ratio.
\(({\text{CD}} = ){\text{ }}278{\text{ }}({\text{m}}){\text{ }}(277.653 \ldots )\) (A1) (C2)[2 marks]
\(\tan {\text{ABC (or equivalent)}} = \frac{{\frac{4}{3} \times 277.653 \ldots }}{{250}}\) (M1)(M1)(M1)
Note: Award (M1) for \(\frac{4}{3}\) multiplying their part (a), (M1) for substitution into the tangent ratio, (M1) for correct substitution.
OR
\(90 – {\tan ^{ – 1}}\left( {\frac{{250}}{{\frac{4}{3} \times 277.653 \ldots }}} \right)\) (M1)(M1)(M1)
Note: Award (M1) for \(\frac{4}{3}\) multiplying their part (a), (M1) for substitution into the tangent ratio, (M1) for subtracting from 90 and for correct substitution.
\({\text{(angle of depression}} = {\text{) }}56.0^\circ {\text{ }}(55.9687 \ldots )\) (A1)(ft) (C4)
Note: Follow through from part (a).[4 marks]
Question
A lampshade, in the shape of a cone, has a wireframe consisting of a circular ring and four straight pieces of equal length, attached to the ring at points A, B, C and D.
The ring has its centre at point O and its radius is 20 centimetres. The straight pieces meet at point V, which is vertically above O, and the angle they make with the base of the lampshade is 60°.
This information is shown in the following diagram.
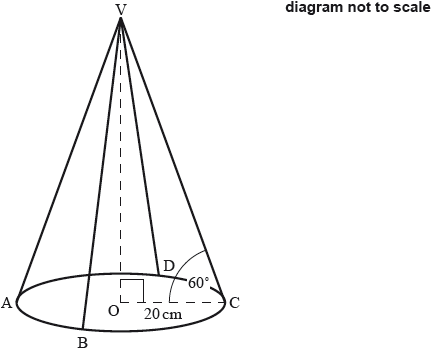
Find the length of one of the straight pieces in the wireframe.[2]
Find the total length of wire needed to construct this wireframe. Give your answer in centimetres correct to the nearest millimetre.[4]
Answer/Explanation
Markscheme
\(\cos 60^\circ = \frac{{20}}{b}\)\(\,\,\,\)OR\(\,\,\,\)\(b = \frac{{20}}{{\cos 60^\circ }}\) (M1)
Note: Award (M1) for correct substitution into a correct trig. ratio.
\((b = ){\text{ 40 (cm)}}\) (A1) (C2)[2 marks]
\(4 \times 40 + 2\pi (20)\) (M1)(M1)
Note: Award (M1) for correct substitution in the circumference of the circle formula, (M1) for adding 4 times their answer to part (a) to their circumference of the circle.
285.6637… (A1)(ft)
Note: Follow through from part (a). This (A1) may be implied by a correct rounded answer.
285.7 (cm) (A1)(ft) (C4)
Notes: Award (A1)(ft) for rounding their answer (consistent with their method) to the nearest millimetre, irrespective of unrounded answer seen.
The final (A1)(ft) is not dependent on any of the previous M marks. It is for rounding their unrounded answer correctly.[4 marks]
Question
Emily’s kite ABCD is hanging in a tree. The plane ABCDE is vertical.
Emily stands at point E at some distance from the tree, such that EAD is a straight line and angle BED = 7°. Emily knows BD = 1.2 metres and angle BDA = 53°, as shown in the diagram
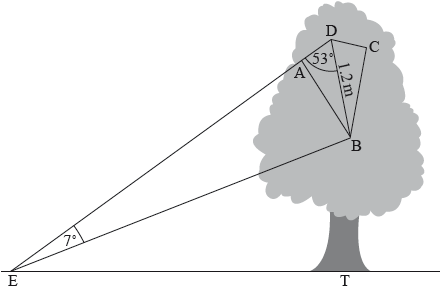
T is a point at the base of the tree. ET is a horizontal line. The angle of elevation of A from E is 41°.
Find the length of EB.[3]
Write down the angle of elevation of B from E.[1]
Find the vertical height of B above the ground.[2]
Answer/Explanation
Markscheme
Units are required in parts (a) and (c).
\(\frac{{{\text{EB}}}}{{\sin 53{\rm{^\circ }}}} = \frac{{1.2}}{{\sin 7{\rm{^\circ }}}}\) (M1)(A1)
Note: Award (M1) for substitution into sine formula, (A1) for correct substitution.
\(({\text{EB}} = ){\text{ }}7.86{\text{ m}}\)\(\,\,\,\)OR\(\,\,\,\)\(786{\text{ cm }}(7.86385 \ldots {\text{ m}}\)\(\,\,\,\)OR\(\,\,\,\)\(786.385 \ldots {\text{ cm}})\) (A1) (C3)[3 marks]
34° (A1) (C1)[1 mark]
Units are required in parts (a) and (c).
\(\sin 34^\circ = \frac{{{\text{height}}}}{{7.86385 \ldots }}\) (M1)
Note: Award (M1) for correct substitution into a trigonometric ratio.
\(({\text{height}} = ){\text{ }}4.40{\text{ m}}\)\(\,\,\,\)OR\(\,\,\,\)\(440{\text{ cm }}(4.39741 \ldots {\text{ m}}\)\(\,\,\,\)OR\(\,\,\,\)\(439.741 \ldots {\text{ cm}})\) (A1)(ft) (C2)
Note: Accept “BT” used for height. Follow through from parts (a) and (b). Use of 7.86 gives an answer of 4.39525….[2 marks]
Question
Tom stands at the top, T , of a vertical cliff \(150{\text{ m}}\) high and sees a fishing boat, F , and a ship, S . B represents a point at the bottom of the cliff directly below T . The angle of depression of the ship is \({40^ \circ }\) and the angle of depression of the fishing boat is \({55^ \circ }\) .
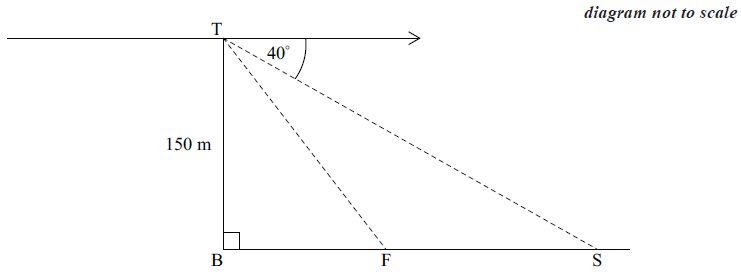
Calculate, SB, the distance between the ship and the bottom of the cliff.[2]
Calculate, SF, the distance between the ship and the fishing boat. Give your answer correct to the nearest metre.[4]
Answer/Explanation
Markscheme
\(150\tan 50\) (M1)
OR
\(\frac{{150}}{{\tan 40}}\) (M1)
\( = 179{\text{ (m)}}\) (\(178.763 \ldots \)) (A1) (C2)
\(150\tan 50 – 150\tan 35\) (M1)(M1)
Note: Award (M1) for \(150\tan 35\), (M1) for subtraction from their part (a).
\( = 73.7{\text{ (m)}}\) (\(73.7319 \ldots \)) (A1)(ft)
\( = 74{\text{ (m)}}\) (A1)(ft) (C4)
Note: The final (A1) is awarded for the correct rounding of their answer to (b).
Note: There will always be one answer with a specified degree of accuracy on each paper.
Question
The diagram shows a rectangular based right pyramid VABCD in which \({\text{AD}} = 20{\text{ cm}}\), \({\text{DC}} = 15{\text{ cm}}\) and the height of the pyramid, \({\text{VN}} = 30{\text{ cm}}\).
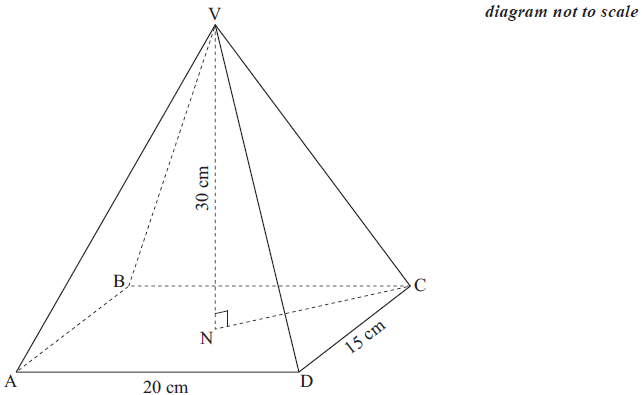
Calculate
(i) the length of AC;
(ii) the length of VC.[4]
Calculate the angle between VC and the base ABCD.[2]
Answer/Explanation
Markscheme
(i) \(\sqrt {{{15}^2} + {{20}^2}} \) (M1)
Note: Award (M1) for correct substitution in Pythagoras Formula.
\({\text{AC}} = 25{\text{ (cm)}}\) (A1) (C2)
(ii) \(\sqrt {{{12.5}^2} + {{30}^2}} \) (M1)
Note: Award (M1) for correct substitution in Pythagoras Formula.
\({\text{VC}} = 32.5{\text{ (cm)}}\) (A1)(ft) (C2)
Note: Follow through from their AC found in part (a).
\(\sin {\text{VCN}} = \frac{{30}}{{32.5}}\) OR \(\tan {\text{VCN}} = \frac{{30}}{{12.5}}\) OR \(\cos {\text{VCN}} = \frac{{12.5}}{{32.5}}\) (M1)
\({ = 67.4^ \circ }\) (\(67.3801 \ldots \)) (A1)(ft) (C2)
Note: Accept alternative methods. Follow through from part (a) and/or part (b).