Download IITian Academy App for accessing Online Mock Tests and More..
Question
The following curves are sketches of the graphs of the functions given below, but in a different order. Using your graphic display calculator, match the equations to the curves, writing your answers in the table below.
(the diagrams are not to scale)
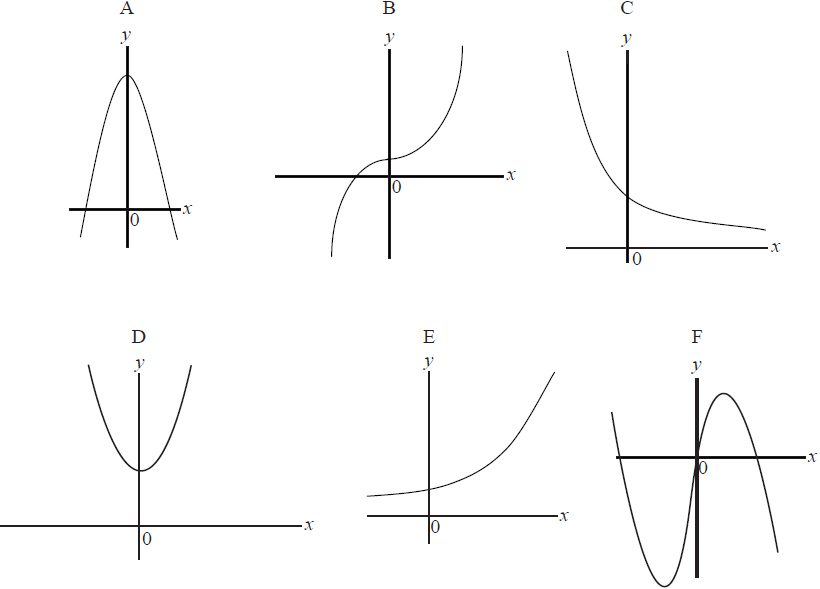
Answer/Explanation
Markscheme
(i) B (A1)
(ii) D (A1)
(iii) A (A1)
(iv) E (A1)
(v) C (A1)
(vi) F (A1) (C6)[6 marks]
Question
In an experiment it is found that a culture of bacteria triples in number every four hours. There are \(200\) bacteria at the start of the experiment.
Find the value of \(a\).[1]
Calculate how many bacteria there will be after one day.[2]
Find how long it will take for there to be two million bacteria.[3]
Answer/Explanation
Markscheme
\(a = 1800\) (A1) (C1)[1 mark]
\(200 \times {3^6}{\text{ (or }}16200 \times 9{\text{) }} = 145800\) (M1)(A1) (C2)[2 marks]
\(200 \times {3^n} = 2 \times {10^6}\) (where \(n\) is each \(4\) hour interval) (M1)
Note: Award (M1) for attempting to set up the equation or writing a list of numbers.
\({3^n} = {10^4}\)
\(n = 8.38{\text{ }}(8.383613097)\) correct answer only (A1)
\({\text{Time}} = 33.5{\text{ hours}}\) (accept \(34\), \(35\) or \(36\) if previous A mark awarded) (A1)(ft) (C3)
Note: (A1)(ft) for correctly multiplying their answer by \(4\). If \(34\), \(35\) or \(36\) seen, or \(32 – 36\) seen, award (M1)(A0)(A0).[3 marks]
Question
The number of cells, C, in a culture is given by the equation \(C = p \times 2^{0.5t} + q\), where t is the time in hours measured from 12:00 on Monday and p and q are constants.
The number of cells in the culture at 12:00 on Monday is 47.
The number of cells in the culture at 16:00 on Monday is 53.
Use the above information to write down two equations in p and q ;[2]
Use the above information to calculate the value of p and of q ;[2]
Use the above information to find the number of cells in the culture at 22:00 on Monday.[2]
Answer/Explanation
Markscheme
p + q = 47 (A1)
4p + q = 53 (A1) (C2)[2 marks]
Reasonable attempt to solve their equations (M1)
p = 2, q = 45 (A1) (C2)
Note: Accept only the answers p = 2, q = 45.[2 marks]
C = 2 × 20.5(10) + 45 (M1)
C = 109 (A1)(ft) (C2)
Note: Award (M1) for substitution of 10 into the formula with their values of p and q.[2 marks]
Question
Given the function \(f (x) = 2 \times 3^x\) for −2 \( \leqslant \) x \( \leqslant \) 5,
find the range of \(f\).[4]
find the value of \(x\) given that \(f (x) =162\).[2]
Answer/Explanation
Markscheme
\(f (-2) = 2 \times 3^{-2}\) (M1)
\(= \frac{{2}}{{9}}(0.222)\) (A1)
\(f (5) = 2 \times 3^5\)
\(= 486\) (A1)
\({\text{Range }}\frac{2}{9} \leqslant f(x) \leqslant 486\) OR \(\left[ {\frac{2}{9},{\text{ }}486} \right]\) (A1) (C4)
Note: Award (M1) for correct substitution of –2 or 5 into \(f (x)\), (A1)(A1) for each correct end point.[4 marks]
\(2 \times 3^x = 162\) (M1)
\(x = 4\) (A1) (C2)[2 marks]
Question
A rumour spreads through a group of teenagers according to the exponential model
\(N = 2 \times {(1.81)^{0.7t}}\)
where N is the number of teenagers who have heard the rumour t hours after it is first started.
Find the number of teenagers who started the rumour.[2]
Write down the number of teenagers who have heard the rumour five hours after it is first started.[1]
Determine the length of time it would take for 150 teenagers to have heard the rumour. Give your answer correct to the nearest minute.[3]
Answer/Explanation
Markscheme
N = 2 × (1.81)0.7×0 (M1)
N = 2 (A1) (C2)
Notes: Award (M1) for correct substitution of t = 0.
Award (A1) for correct answer.[2 marks]
16.0 (3 s.f) (A1) (C1)
Note: Accept 16 and 15.[1 mark]
150 = 2 × (1.81)0.7t (M1)
t = 10.39… h (A1)
t = 624 minutes (A1)(ft) (C3)
Notes: Accept 10 hours 24 minutes. Accept alternative methods.
Award last (A1)(ft) for correct rounding to the nearest minute of their answer.
Unrounded answer must be seen so that the follow through can be awarded.[3 marks]
Question
Consider the function \(f(x) = p{(0.5)^x} + q\) where p and q are constants. The graph of f (x) passes through the points \((0,\,6)\) and \((1,\,4)\) and is shown below.
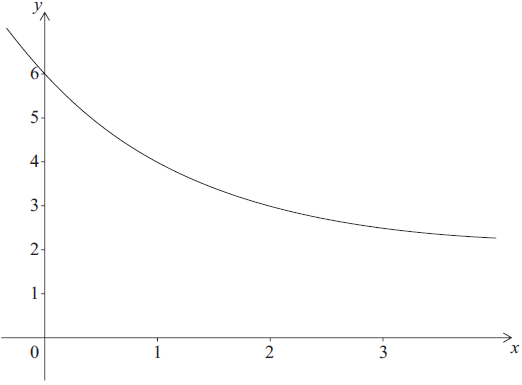
Write down two equations relating p and q.[2]
Find the value of p and of q.[2]
Write down the equation of the horizontal asymptote to the graph of f (x).[2]
Answer/Explanation
Markscheme
p + q = 6 (A1)
0.5p + q = 4 (A1) (C2)
Note: Accept correct equivalent forms of the equations.[2 marks]
p = 4, q = 2 (A1)(A1)(ft) (C2)
Notes: If both answers are incorrect, award (M1) for attempt at solving simultaneous equations.[2 marks]
y = 2 (A1)(A1)(ft) (C2)
Notes: Award (A1) for “y = a constant”, (A1)(ft) for 2. Follow through from their value for q as long as their constant is greater than 2 and less than 6.
An equation must be seen for any marks to be awarded.[2 marks]
Question
Consider the function \(f(x) = 1.25 – {a^{ – x}}\) , where a is a positive constant and \(x \geqslant 0\). The diagram shows a sketch of the graph of \(f\) . The graph intersects the \(y\)-axis at point A and the line \(L\) is its horizontal asymptote.
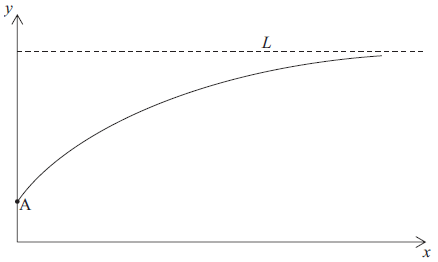
Find the \(y\)-coordinate of A .[2]
The point \((2{\text{, }}1)\) lies on the graph of \(y = f(x)\) . Calculate the value of \(a\) .[2]
The point \((2{\text{, }}1)\) lies on the graph of \(y = f(x)\) . Write down the equation of \(L\) .[2]
Answer/Explanation
Markscheme
\(y = 1.25 – {a^0}\) \(1.25 – 1\) (M1)
\(= 0.25\) (A1) (C2)
Note: Award (M1)(A1) for \((0{\text{, }}0.25)\) .[2 marks]
\(1 = 1.25 – {a^{ – 2}}\) (M1)
\(a = 2\) (A1) (C2)[2 marks]
\(y = 1.25\) (A1)(A1) (C2)
Note: Award (A1) for \(y =\) “a constant”, (A1) for \(1.25\).[2 marks]
Question
Shiyun bought a car in 1999. The value of the car \(V\) , in USD, is depreciating according to the exponential model
\[V = 25000 \times {1.5^{ – 0.2t}}{\text{, }}t \geqslant 0\]
where \(t\) is the time, in years, that Shiyun has owned the car.
Write down the value of the car when Shiyun bought it.[1]
Calculate the value of the car three years after Shiyun bought it. Give your answer correct to two decimal places.[2]
Calculate the time for the car to depreciate to half of its value since Shiyun bought it.[3]
Answer/Explanation
Markscheme
\(25000{\text{ USD}}\) (A1) (C1)[1 mark]
\(25000 \times {1.5^{ – 0.6}}\) (M1)
\(19601.32{\text{ USD}}\) (A1) (C2)[2 marks]
\(12500 = 25000 \times {1.5^{ – 0.2t}}\) (A1)(ft)(M1)
Notes: Award (A1)(ft) for \(12 500\) seen. Follow through from their answer to part (a). Award (M1) for equating their half value to \(25000 \times {1.5^{ – 0.2t}}\) . Allow the use of an inequality.
OR
Graphical method (sketch):
(A1)(ft) for \(y =12 500\) seen on the sketch. Follow through from their answer to part (a). (A1)(ft)
(M1) for the exponent model and an indication of their intersection with their horizontal line. (M1)
\(8.55\) (A1)(ft) (C3)[3 marks]
Question
The function \(g(x)\) is defined as \(g(x) = 16 + k({c^{ – x}})\) where \(c > 0\) .
The graph of the function \(g\) is drawn below on the domain \(x \geqslant 0\) .
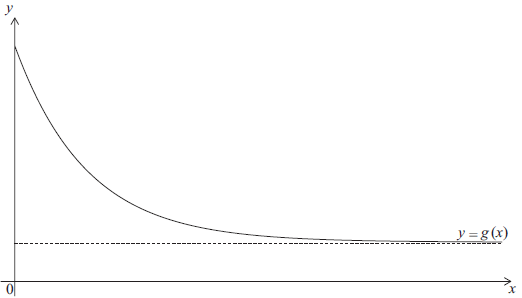
The graph of \(g\) intersects the y-axis at (0, 80) .
Find the value of \(k\) .[2]
The graph passes through the point (2, 48) .
Find the value of \(c\) .[2]
The graph passes through the point (2, 48) .
Write down the equation of the horizontal asymptote to the graph of \(y = g(x)\) .[2]
Answer/Explanation
Markscheme
\(80 = 16 + k({c^0})\) (M1)
\(k = 64\) (A1) (C2)[2 marks]
\(48 = 16 + 64({c^{ – 2}})\) (M1)
Note: Award (M1) for substitution of their \(k\) and (2, 48) into the equation for \(g(x)\).
\(c = \sqrt 2 \) (\(1.41\)) (\(1.41421 \ldots \)) (A1)(ft) (C2)
Notes: Award (M1)(A1)(ft) for \(c = \pm \sqrt 2 \) . Follow through from their answer to part (a).[2 marks]
\(y = 16\) (A1)(A1) (C2)
Note: Award (A1) for \(y = \) a constant, (A1) for \(16\).[2 marks]
Question
A function f (x) = p×2x + q is defined by the mapping diagram below.
Find the value of
(i) p ;
(ii) q .[3]
Write down the value of r .[1]
Find the value of s .[2]
Answer/Explanation
Markscheme
(i) 2p + q = 11 and 4p + q = 17 (M1)
Note: Award (M1) for either two correct equations or a correct equation in one unknown equivalent to 2p = 6 .
p = 3 (A1)
(ii) q = 5 (A1) (C3)
Notes: If only one value of p and q is correct and no working shown, award (M0)(A1)(A0).[3 marks]
r = 8 (A1)(ft) (C1)
Note: Follow through from their answers for p and q irrespective of whether working is seen.[1 mark]
3 × 2s + 5 = 197 (M1)
Note: Award (M1) for setting the correct equation.
s = 6 (A1)(ft) (C2)
Note: Follow through from their values of p and q.[2 marks]
Question
The diagram shows part of the graph of \(y = 2^{-x} + 3 \), and its horizontal asymptote. The graph passes through the points (0, a) and (b, 3.5).
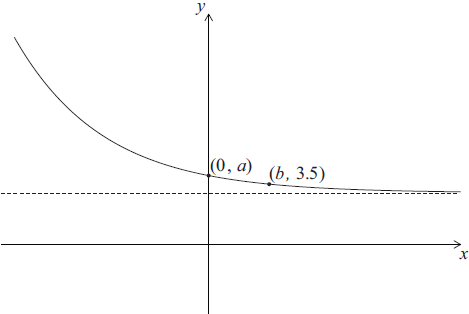
Find the value of
(i) a ;
(ii) b .[4]
Write down the equation of the horizontal asymptote to this graph.[2]
Answer/Explanation
Markscheme
(i) 20 + 3 (M1)
Note: Award (M1) for correct substitution.
= 4 (A1) (C2)
(ii) 3.5 = 2−b + 3 (M1)
Note: Award (M1) for correct substitution.
b = 1 (A1) (C2)[4 marks]
y = 3 (A1)(A1) (C2)
Notes: y = constant (other than 3) award (A1)(A0).[2 marks]
Question
The number of bacteria in a colony is modelled by the function
\(N(t) = 800 \times 3^{0.5t}, {\text{ }} t \geqslant 0\),
where \(N\) is the number of bacteria and \(t\) is the time in hours.
Write down the number of bacteria in the colony at time \(t = 0\).[1]
Calculate the number of bacteria present at 2 hours and 30 minutes. Give your answer correct to the nearest hundred bacteria.[3]
Calculate the time, in hours, for the number of bacteria to reach 5500.[2]
Answer/Explanation
Markscheme
800 (A1) (C1)
\(800 \times {3^{(0.5 \times 2.5)}}\) (M1)
Note: Award (M1) for correctly substituted formula.
\( = 3158.57\)… (A1)
\(= 3200\) (A1) (C3)
Notes: Final (A1) is given for correctly rounding their answer. This may be awarded regardless of a preceding (A0).
\(5500 = 800 \times {3^{(0.5 \times t)}}\) (M1)
Notes: Award (M1) for equating function to 5500. Accept correct alternative methods.
\(= 3.51{\text{ hours}}\) (3.50968…) (A1) (C2)
Question
A computer virus spreads according to the exponential model
\[N = 200 \times {(1.9)^{0.85t}},{\text{ }}t \geqslant 0\]
where \(N\) is the number of computers infected, and \(t\) is the time, in hours, after the initial infection.
Calculate the number of computers infected after \(6\) hours.[2]
Calculate the time for the number of infected computers to be greater than \({\text{1}}\,{\text{000}}\,{\text{000}}\).
Give your answer correct to the nearest hour.[4]
Answer/Explanation
Markscheme
\(200 \times {(1.9)^{0.85 \times 6}}\) (M1)
Note: Award (M1) for correct substitution into given formula.
\( = 5280\) (A1) (C2)
Note: Accept \(5281\) or \(5300\) but no other answer.[2 marks]
\(1\,000\,000 < 200 \times {(1.9)^{0.85t}}\) (M1)(M1)
Note: Award (M1) for setting up the inequality (accept an equation), and (M1) for \({\text{1}}\,{\text{000}}\,{\text{000}}\) seen in the inequality or equation.
\(t = 15.6\) \((15.6113…)\) (A1)
\(16\) hours (A1)(ft) (C4)
Note: The final (A1)(ft) is for rounding up their answer to the nearest hour.
Award (C3) for an answer of \(15.6\) with no working.
Accept \({\text{1}}\,{\text{000}}\,{\text{001}}\) in an equation.[4 marks]
Question
The amount of electrical charge, C, stored in a mobile phone battery is modelled by \(C(t) = 2.5 – {2^{ – t}}\), where t, in hours, is the time for which the battery is being charged.
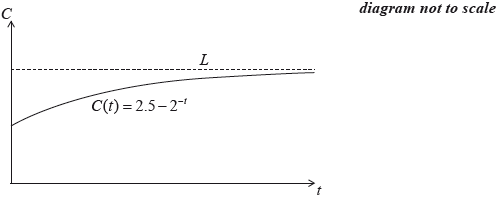
Write down the amount of electrical charge in the battery at \(t = 0\).[1]
The line \(L\) is the horizontal asymptote to the graph.
Write down the equation of \(L\).[2]
To download a game to the mobile phone, an electrical charge of 2.4 units is needed.
Find the time taken to reach this charge. Give your answer correct to the nearest minute.[3]
Answer/Explanation
Markscheme
\(1.5\) (A1) (C1)[1 mark]
\(C = 2.5\) (accept \(y = 2.5\)) (A1)(A1) (C2)
Notes: Award (A1) for \(C{\text{ (or }}y) = \) a positive constant, (A1) for the constant \(= 2.5\).
Answer must be an equation.[2 marks]
\(2.4 = 2.5 – {2^{ – t}}\) (M1)
Note: Award (M1) for setting the equation equal to 2.4 or for a horizontal line drawn at approximately \(C = 2.4\).
Allow \(x\) instead of \(t\).
OR
\( – t\ln (2) = \ln (0.1)\) (M1)
\(t = 3.32192 \ldots \) (A1)
\(t = 3{\text{ hours and 19 minutes (199 minutes)}}\) (A1)(ft) (C3)
Note: Award the final (A1)(ft) for correct conversion of their time in hours to the nearest minute.[3 marks]
Question
In a trial for a new drug, scientists found that the amount of the drug in the bloodstream decreased over time, according to the model
\[D(t) = 1.2 \times {(0.87)^t},{\text{ }}t \geqslant 0\]
where \(D\) is the amount of the drug in the bloodstream in mg per litre \({\text{(mg}}\,{{\text{l}}^{ – 1}}{\text{)}}\) and \(t\) is the time in hours.
Write down the amount of the drug in the bloodstream at \(t = 0\).[1]
Calculate the amount of the drug in the bloodstream after 3 hours.[2]
Use your graphic display calculator to determine the time it takes for the amount of the drug in the bloodstream to decrease to \(0.333{\text{ mg}}{{\text{1}}^{ – 1}}\).[3]
Answer/Explanation
Markscheme
\(1.2{\text{ (mg}}\,{{\text{l}}^{ – 1}}{\text{)}}\) (A1) (C1)[1 mark]
\(1.2 \times {(0.87)^3}\) (M1)
Note: Award (M1) for correct substitution into given formula.
\( = {\text{0.790 (mg}}\,{{\text{l}}^{ – 1}}{\text{) (0.790203}} \ldots {\text{)}}\) (A1) (C2)[2 marks]
\(1.2 \times {0.87^t} = 0.333\) (M1)
Note: Award (M1) for setting up the equation.
(M1)
Notes: Some indication of scale is to be shown, for example the window used on the calculator.
Accept alternative methods.
\(9.21\) (hours) (\(9.20519…\), 9 hours 12 minutes, 9:12) (A1) (C3)[3 marks]
Question
Consider the functions \(f(x) = x + 1\) and \(g(x) = {3^x} – 2\).
Write down
(i) the \(x\)-intercept of the graph of \(y = {\text{ }}f(x)\);
(ii) the \(y\)-intercept of the graph of \(y = {\text{ }}g(x)\).[2]
Solve \(f(x) = g(x)\).[2]
Write down the interval for the values of \(x\) for which \(f(x) > g(x)\).[2]
Answer/Explanation
Markscheme
(i) \(( – 1,{\text{ }}0)\) (A1)
Note: Accept \( – 1\).
(ii) \((0,{\text{ }} – 1)\) (A1) (C2)
Note: Accept \( – 1\).
\((x = ){\text{ }} – 2.96{\text{ }}( – 2.96135 \ldots )\) (A1)
\((x = ){\text{ }}1.34{\text{ }}(1.33508 \ldots )\) (A1) (C2)
\( – 2.96 < x < 1.34\;\;\;{\mathbf{OR}}\;\;\;\left] { – 2.96,{\text{ }}1.34} \right[\;\;\;{\mathbf{OR}}\;\;\;( – 2.96,{\text{ }}1.34)\) (A1)(ft)(A1) (C2)
Notes: Award (A1)(ft) for both correct endpoints of the interval, (A1) for correct strict inequalities (or correct open interval notation).
Follow through from part (b).
Question
A potato is placed in an oven heated to a temperature of 200°C.
The temperature of the potato, in °C, is modelled by the function \(p(t) = 200 – 190{(0.97)^t}\), where \(t\) is the time, in minutes, that the potato has been in the oven.
Write down the temperature of the potato at the moment it is placed in the oven.[2]
Find the temperature of the potato half an hour after it has been placed in the oven.[2]
After the potato has been in the oven for \(k\) minutes, its temperature is 40°C.
Find the value of \(k\).[2]
Answer/Explanation
Markscheme
For parts (a) and (b) only, the first time a correct answer has incorrect or missing units, the final (A1) is not awarded.
\(200 – 190{(0.97)^0}\) (M1)
Note: Award (M1) for correct substitution.
\( = 10\,^\circ {\text{C}}\) (A1) (C2)
Note: Units are required.
For parts (a) and (b) only, the first time a correct answer has incorrect or missing units, the final (A1) is not awarded.
\(200 – 190{(0.97)^{30}}\) (M1)
Note: Award (M1) for correct substitution.
\( = 124^\circ {\text{C }}(123.808 \ldots ^\circ {\text{C}})\) (A1) (C2)
Note: Units are required, unless already omitted in part (a).
\(200 – 190{(0.97)^k} = 40\) (M1)
Note: Award (M1) for correct substitution.
\(k = 5.64{\text{ (minutes) }}(5.64198 \ldots )\) (A1) (C2)
Question
The number of fish, \(N\), in a pond is decreasing according to the model
\[N(t) = a{b^{ – t}} + 40,\;\;\;t \geqslant 0\]
where \(a\) and \(b\) are positive constants, and \(t\) is the time in months since the number of fish in the pond was first counted.
At the beginning \(840\) fish were counted.
Find the value of \(a\).[2]
After \(4\) months \(90\) fish were counted.
Find the value of \(b\).[3]
The number of fish in the pond will not decrease below \(p\).
Write down the value of \(p\).[1]
Answer/Explanation
Markscheme
\(a{b^0} + 40 = 840\) (M1)
Note: Award (M1) for substituting \(t = 0\) and equating to \(840\).
\(a = 800\) (A1)(C2)
\(800{b^{ – 4}} + 40 = 90\) (M1)
Note: Award (M1) for correct substitution of their \(a\) (from part (a)) and \(4\) in the formula of the function and equating to \(90\).
\({b^4} = 16\;\;\;\)OR\(\;\;\;\frac{1}{{{b^4}}} = \frac{1}{{16}}\;\;\;\)OR\(\;\;\;b = \sqrt[4]{{16}}\;\;\;\)OR\(\;\;\;b = {16^{\frac{1}{4}}}\) (M1)
Notes: Award second (M1) for correctly rearranging their equation and eliminating the negative index (see above examples).
Accept \(\frac{{800}}{{50}}\) in place of \(16\).
OR
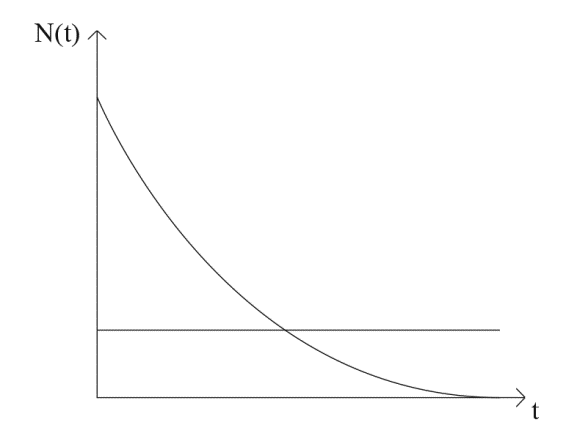(M1)(M1)
Notes: Award (M1) for a decreasing exponential and a horizontal line that are both in the first quadrant, and (M1) for their graphs intersecting.
For graphs drawn in both first and second quadrants award at most (M1)(M0).
\(b = 2\) (A1)(ft) (C3)
Note: Follow through from their answer to part (a) only if \(a\) is positive.
\(40\) (A1) (C1)
Question
A population of \(200\) rabbits was introduced to an island. One week later the number of rabbits was \(210\). The number of rabbits, \(N\) , can be modelled by the function
\[N(t) = 200 \times {b^t},\,\,t \geqslant 0\,,\]
where \(t\) is the time, in weeks, since the rabbits were introduced to the island.
Find the value of \(b\) .[2]
Calculate the number of rabbits on the island after 10 weeks.[2]
An ecologist estimates that the island has enough food to support a maximum population of 1000 rabbits.
Calculate the number of weeks it takes for the rabbit population to reach this maximum.[2]
Answer/Explanation
Markscheme
\(210 = 200 \times {b^1}\) (M1)
Note: Award (M1) for correct substitution into equation.
\((b = )\,\,1.05\) (A1) (C2)
\(200 \times {1.05^{10}}\) (M1)
Note: Award (M1) for correct substitution into formula. Follow through from part (a).
\( = 325\) (A1)(ft) (C2)
Note: The answer must be an integer.
\(200 \times {1.05^t} = 1000\) (M1)
\(t = 33.0\,\,\,(32.9869…)\) (A1)(ft) (C2)
Note: Award (M1) for setting up the equation. Accept alternative methods such as \(t = \frac{{\log \,(5)}}{{\log \,(1.05)}}\) , or a sketch of \(y = 200 \times {1.05^t}\) and \(y = 1000\) with indication of point of intersection. Follow through from (a).
Question
A population of mosquitoes decreases exponentially. The size of the population, \(P\) , after \(t\) days is modelled by
\(P = 3200 \times {2^{ – t}} + 50\) , where \(t \geqslant 0\) .
Write down the exact size of the initial population.[1]
Find the size of the population after \(4\) days.[2]
Calculate the time it will take for the size of the population to decrease to \(60\).[2]
The population will stabilize when it reaches a size of \(k\) .
Write down the value of \(k\) .[1]
Answer/Explanation
Markscheme
\(3250\) (A1) (C1)
\(3200 \times {2^{ – 4}} + 50\) (M1)
Note: Award (M1) for substituting \(t\) into exponential equation.
\( = 250\) (A1) (C2)
\(3200 \times {2^{ – t}} + 50 = 60\) (M1)
Note: Award (M1) for setting up the equation used in part (b).
OR
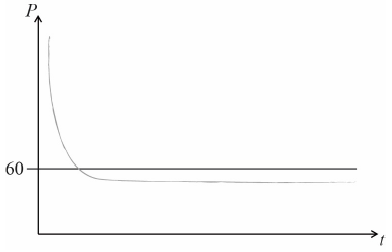
(M1)
Note: Award (M1) for a decreasing exponential graph intersecting a horizontal line.
\((t = )\,\,8.32\,\,(8.32192…)\) (days) (A1) (C2)
Note: Accept a final answer of “\(8\) days, \(7\) hours and \(44\) minutes”, or equivalent. Award (M0)(A0) for an answer of \(8\) days with no working
\(50\) (A1) (C1)
Question
Gabriella purchases a new car.
The car’s value in dollars, \(V\), is modelled by the function
\[V(t) = 12870 – k{(1.1)^t},{\text{ }}t \geqslant 0\]
where \(t\) is the number of years since the car was purchased and \(k\) is a constant.
After two years, the car’s value is $9143.20.
This model is defined for \(0 \leqslant t \leqslant n\). At \(n\) years the car’s value will be zero dollars.
Write down, and simplify, an expression for the car’s value when Gabriella purchased it.[2]
Find the value of \(k\).[2]
Find the value of \(n\).[2]
Answer/Explanation
Markscheme
\(12870 – k{(1.1)^0}\) (M1)
Note: Award (M1) for correct substitution into \(V(t)\).
\( = 12870 – k\) (A1) (C2)
Note: Accept \(12870 – 3080\) OR 9790 for a final answer.[2 marks]
\(9143.20 = 12870 – k{(1.1)^2}\) (M1)
Note: Award (M1) for correct substitution into \(V(t)\).
\((k = ){\text{ }}3080\) (A1) (C2)[2 marks]
\(12870 – 3080{(1.1)^n} = 0\) (M1)
Note: Award (M1) for correct substitution into \(V(t)\).
OR
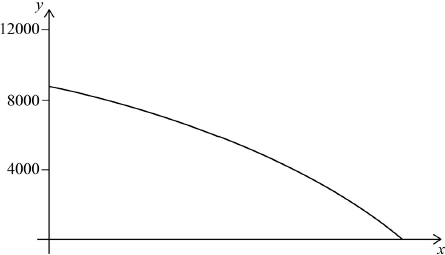(M1)
Note: Award (M1) for a correctly shaped curve with some indication of scale on the vertical axis.
\((n = ){\text{ }}15.0{\text{ }}(15.0033 \ldots )\) (A1)(ft) (C2)
Note: Follow through from part (b).[2 marks]
Question
Jashanti is saving money to buy a car. The price of the car, in US Dollars (USD), can be modelled by the equation
\[P = 8500{\text{ }}{(0.95)^t}.\]
Jashanti’s savings, in USD, can be modelled by the equation
\[S = 400t + 2000.\]
In both equations \(t\) is the time in months since Jashanti started saving for the car.
Jashanti does not want to wait too long and wants to buy the car two months after she started saving. She decides to ask her parents for the extra money that she needs.
Write down the amount of money Jashanti saves per month.[1]
Use your graphic display calculator to find how long it will take for Jashanti to have saved enough money to buy the car.[2]
Calculate how much extra money Jashanti needs.[3]
Answer/Explanation
Markscheme
400 (USD) (A1) (C1)[1 mark]
\(8500{\text{ }}{(0.95)^t} = 400 \times t + 2000\) (M1)
Note: Award (M1) for equating \(8500{(0.95)^t}\) to \(400 \times t + 2000\) or for comparing the difference between the two expressions to zero or for showing a sketch of both functions.
\((t = ){\text{ }}8.64{\text{ (months) }}\left( {8.6414 \ldots {\text{ (months)}}} \right)\) (A1) (C2)
Note: Accept 9 months.[2 marks]
\(8500{(0.95)^2} – (400 \times 2 + 2000)\) (M1)(M1)
Note: Award (M1) for correct substitution of \(t = 2\) into equation for \(P\), (M1) for finding the difference between a value/expression for \(P\) and a value/expression for \(S\). The first (M1) is implied if 7671.25 seen.
4870 (USD) (4871.25) (A1) (C3)
Note: Accept 4871.3.[3 marks]
Question
Sejah placed a baking tin, that contained cake mix, in a preheated oven in order to bake a cake. The temperature in the centre of the cake mix, \(T\), in degrees Celsius (°C) is given by
\[T(t) = 150 – a \times {(1.1)^{ – t}}\]
where \(t\) is the time, in minutes, since the baking tin was placed in the oven. The graph of \(T\) is shown in the following diagram.
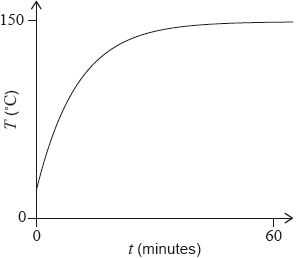
The temperature in the centre of the cake mix was 18 °C when placed in the oven.
The baking tin is removed from the oven 15 minutes after the temperature in the centre of the cake mix has reached 130 °C.
Write down what the value of 150 represents in the context of the question.[1]
Find the value of \(a\).[2]
Find the total time that the baking tin is in the oven.[3]
Answer/Explanation
Markscheme
the temperature in the oven (A1)
OR
the maximum possible temperature of the cake mix (A1) (C1)
Note: Award (A0) for “the maximum temperature”.[1 mark]
\(18 = 150 – a( \times 1.1^\circ )\) (M1)
Note: Award (M1) for correct substitution of 18 and 0. Substitution of 0 can be implied.
\((a) = 132\) (A1) (C2)[2 marks]
\(150-132 \times {1.1^{ – t}} = 130\) (M1)
Note: Award (M1) for substituting their a and equating to 130. Accept an inequality.
Award (M1) for a sketch of the horizontal line on the graph.
\(t = 19.8{\text{ }}(19.7992 \ldots )\) (A1)(ft)
Note: Follow through from part (b).
34.8 (minutes) (34.7992…, 34 minutes 48 seconds) (A1)(ft) (C3)
Note: Award the final (A1) for adding 15 minutes to their \(t\) value.
In part (c), award (C2) for a final answer of 19.8 with no working.[3 marks]
Question
In an experiment, a number of fruit flies are placed in a container. The population of fruit flies, P , increases and can be modelled by the function
\[P\left( t \right) = 12 \times {3^{0.498t}},\,\,t \geqslant 0,\]
where t is the number of days since the fruit flies were placed in the container.
Find the number of fruit flies which were placed in the container.[2]
Find the number of fruit flies that are in the container after 6 days.[2]
The maximum capacity of the container is 8000 fruit flies.
Find the number of days until the container reaches its maximum capacity.[2]
Answer/Explanation
Markscheme
\(12 \times {3^{0.498 \times 0}}\) (M1)
Note: Award (M1) for substituting zero into the equation.
= 12 (A1) (C2)[2 marks]
\(12 \times {3^{0.498 \times 6}}\) (M1)
Note: Award (M1) for substituting 6 into the equation.
320 (A1) (C2)
Note: Accept an answer of 319.756… or 319.[2 marks]
\(8000 = 12 \times {3^{0.498 \times t}}\) (M1)
Note: Award (M1) for equating equation to 8000.
Award (M1) for a sketch of P(t) intersecting with the straight line y = 8000.
= 11.9 (11.8848…) (A1) (C2)
Note: Accept an answer of 11 or 12.[2 marks]
Question
The following function models the growth of a bacteria population in an experiment,
P(t) = A × 2t, t ≥ 0
where A is a constant and t is the time, in hours, since the experiment began.
Four hours after the experiment began, the bacteria population is 6400.
Find the value of A.[2]
Interpret what A represents in this context.[1]
Find the time since the experiment began for the bacteria population to be equal to 40A.[3]
Answer/Explanation
Markscheme
6400 = A × 24 (M1)
Note: Award (M1) for correct substitution of 4 and 6400 in equation.
(A =) 400 (A1) (C2)[2 marks]
the initial population OR the population at the start of experiment (A1) (C1)[1 mark]
40A = A × 2t OR 40 × 400 = 400 × 2t (M1)
Note: Award (M1) for correct substitution into equation. Follow through with their A from part (a).
40 = 2t (M1)
Note: Award (M1) for simplifying.
5.32 (5.32192…) (hours) OR 5 hours 19.3 (19.3156…) minutes (A1) (C3)[3 marks]
Question
On the grid below sketch the graph of the function \(f(x) = 2{(1.6)^x}\) for the domain \(0 \leqslant x \leqslant 3\) .
[2]
Write down the coordinates of the \(y\)-intercept of the graph of \(y = f(x)\) .[1]
On the grid draw the graph of the function \(g(x) = 5 – 2x\) for the domain \(0 \leqslant x \leqslant 3\).[2]
Use your graphic display calculator to solve \(f(x) = g(x)\) .[1]
Answer/Explanation
Markscheme
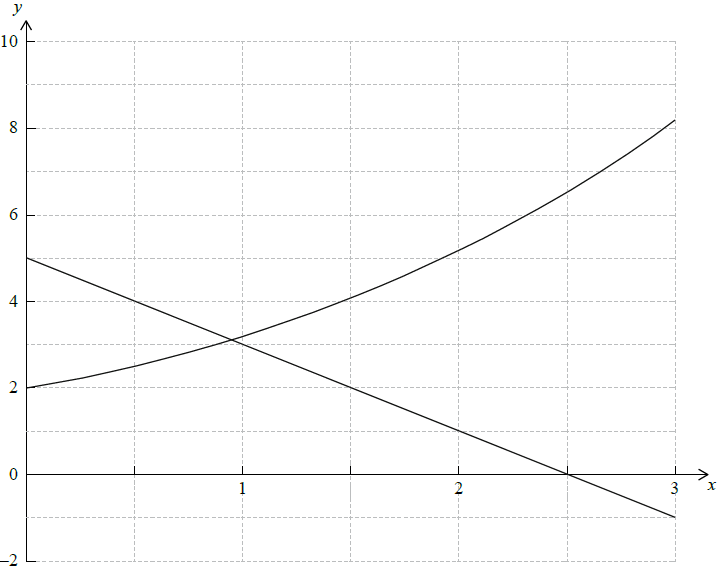
Note: Award (A1) correct endpoints, (A1) for smooth curve. (A1)(A1) (C2)
\((0{\text{, }}2)\) (A1) (C1)
Note: Accept \(x = 0\), \(y = 2\)
Straight line in the given domain (A1)
Axes intercepts in the correct positions (A1) (C2)
\(x = 0.943\) (\(0.94259 \ldots \)) (A1) (C1)
Note: Award (A0) if \(y\)-coordinate given.