Question
Consider the arithmetic sequence \(2{\text{, }}5{\text{, }}8{\text{, }}11{\text{,}} \ldots \) .
Find \({u_{101}}\) .
Consider the arithmetic sequence \(2{\text{, }}5{\text{, }}8{\text{, }}11{\text{,}} \ldots \) .
Find the value of n so that \({u_n} = 152\) .
Answer/Explanation
Markscheme
\(d = 3\) (A1)
evidence of substitution into \({u_n} = a + (n – 1)d\) (M1)
e.g. \({u_{101}} = 2 + 100 \times 3\)
\({u_{101}} = 302\) A1 N3
[3 marks]
correct approach (M1)
e.g. \(152 = 2 + (n – 1) \times 3\)
correct simplification (A1)
e.g. \(150 = (n – 1) \times 3\) , \(50 = n – 1\) , \(152 = – 1 + 3n\)
\(n = 51\) A1 N2
[3 marks]
Examiners report
Candidates probably had the most success with this question with many good solutions which were written with the working clearly shown. Many used the alternate approach of \({u_n} = 3n – 1\) .
Candidates probably had the most success with this question with many good solutions which were written with the working clearly shown. Many used the alternate approach of \({u_n} = 3n – 1\) .
Question
Consider the infinite geometric sequence \(3{\text{, }}3(0.9){\text{, }}3{(0.9)^2}{\text{, }}3{(0.9)^3}{\text{, }} \ldots \) .
Write down the 10th term of the sequence. Do not simplify your answer.
Consider the infinite geometric sequence \(3{\text{, }}3(0.9){\text{, }}3{(0.9)^2}{\text{, }}3{(0.9)^3}{\text{, }} \ldots \) .
Find the sum of the infinite sequence.
Answer/Explanation
Markscheme
\({u_{10}} = 3{(0.9)^9}\) A1 N1
[1 mark]
recognizing \(r = 0.9\) (A1)
correct substitution A1
e.g. \(S = \frac{3}{{1 – 0.9}}\)
\(S = \frac{3}{{0.1}}\) (A1)
\(S = 30\) A1 N3
[4 marks]
Examiners report
This question was well done by most candidates. There were a surprising number of candidates who lost a mark for not simplifying \(\frac{3}{{0.1}}\) to 30 , and there were a few candidates who used the formula for the finite sum unsuccessfully.
This question was well done by most candidates. There were a surprising number of candidates who lost a mark for not simplifying \(\frac{3}{{0.1}}\) to 30, and there were a few candidates who used the formula for the finite sum unsuccessfully.
Question
The first three terms of an infinite geometric sequence are 32, 16 and 8.
Write down the value of r .
Find \({u_6}\) .
Find the sum to infinity of this sequence.
Answer/Explanation
Markscheme
\(r = \frac{{16}}{{32}}\left( { = \frac{1}{2}} \right)\) A1 N1
[1 mark]
correct calculation or listing terms (A1)
e.g. \(32 \times {\left( {\frac{1}{2}} \right)^{6 – 1}}\) , \(8 \times {\left( {\frac{1}{2}} \right)^3}\) , 32, \(\ldots \) 4, 2, 1
\({u_6} = 1\) A1 N2
[2 marks]
evidence of correct substitution in \({S_\infty }\) A1
e.g. \(\frac{{32}}{{1 – \frac{1}{2}}}\) , \(\frac{{32}}{{\frac{1}{2}}}\)
\({S_\infty } = 64\) A1 N1
[2 marks]
Examiners report
This question was very well done by the majority of candidates. There were some who used a value of r greater than one, with the most common error being \(r = 2\) .
This question was very well done by the majority of candidates. There were some who used a value of r greater than one, with the most common error being \(r = 2\) .
A handful of candidates struggled with the basic computation involved in part (c).
Question
In an arithmetic sequence, \({u_1} = 2\) and \({u_3} = 8\) .
Find d .
Find \({u_{20}}\) .
Find \({S_{20}}\) .
Answer/Explanation
Markscheme
attempt to find d (M1)
e.g. \(\frac{{{u_3} – {u_1}}}{2}\) , \(8 = 2 + 2d\)
\(d = 3\) A1 N2
[2 marks]
correct substitution (A1)
e.g. \({u_{20}} = 2 + (20 – 1)3\) , \({u_{20}} = 3 \times 20 – 1\)
\({u_{20}} = 59\) A1 N2
[2 marks]
correct substitution (A1)
e.g. \({S_{20}} = \frac{{20}}{2}(2 + 59)\) , \({S_{20}} = \frac{{20}}{2}(2 \times 2 + 19 \times 3)\)
\({S_{20}} = 610\) A1 N2
[2 marks]
Examiners report
This question was answered correctly by the large majority of candidates. The few mistakes seen were due to either incorrect substitution into the formula or simple arithmetic errors. Even where candidates made mistakes, they were usually able to earn full follow-through marks in the subsequent parts of the question.
This question was answered correctly by the large majority of candidates. The few mistakes seen were due to either incorrect substitution into the formula or simple arithmetic errors. Even where candidates made mistakes, they were usually able to earn full follow-through marks in the subsequent parts of the question.
This question was answered correctly by the large majority of candidates. The few mistakes seen were due to either incorrect substitution into the formula or simple arithmetic errors. Even where candidates made mistakes, they were usually able to earn full follow-through marks in the subsequent parts of the question.
Question
The first three terms of a infinite geometric sequence are \(m – 1,{\text{ 6, }}m + 4\), where \(m \in \mathbb{Z}\).
Write down an expression for the common ratio, \(r\).
Hence, show that \(m\) satisfies the equation \({m^2} + 3m – 40 = 0\).
Find the two possible values of \(m\).
Find the possible values of \(r\).
The sequence has a finite sum.
State which value of \(r\) leads to this sum and justify your answer.
The sequence has a finite sum.
Calculate the sum of the sequence.
Answer/Explanation
Markscheme
correct expression for \(r\) A1 N1
eg \(r = \frac{6}{{m – 1}},{\text{ }}\frac{{m + 4}}{6}\)
[2 marks]
correct equation A1
eg \(\frac{6}{{m – 1}} = \frac{{m + 4}}{6},{\text{ }}\frac{6}{{m + 4}} = \frac{{m – 1}}{6}\)
correct working (A1)
eg \((m + 4)(m – 1) = 36\)
correct working A1
eg \({m^2} – m + 4m – 4 = 36,{\text{ }}{m^2} + 3m – 4 = 36\)
\({m^2} + 3m – 40 = 0\) AG N0
[2 marks]
valid attempt to solve (M1)
eg \((m + 8)(m – 5) = 0,{\text{ }}m = \frac{{ – 3 \pm \sqrt {9 + 4 \times 40} }}{2}\)
\(m = – 8,{\text{ }}m = 5\) A1A1 N3
[3 marks]
attempt to substitute any value of \(m\) to find \(r\) (M1)
eg \(\frac{6}{{ – 8 – 1}},{\text{ }}\frac{{5 + 4}}{6}\)
\(r = \frac{3}{2},{\text{ }}r = – \frac{2}{3}\) A1A1 N3
[3 marks]
\(r = – \frac{2}{3}\) (may be seen in justification) A1
valid reason R1 N0
eg \(\left| r \right| < 1,{\text{ }} – 1 < \frac{{ – 2}}{3} < 1\)
Notes: Award R1 for \(\left| r \right| < 1\) only if A1 awarded.
[2 marks]
finding the first term of the sequence which has \(\left| r \right| < 1\) (A1)
eg \( – 8 – 1,{\text{ }}6 \div \frac{{ – 2}}{3}\)
\({u_1} = – 9\) (may be seen in formula) (A1)
correct substitution of \({u_1}\) and their \(r\) into \(\frac{{{u_1}}}{{1 – r}}\), as long as \(\left| r \right| < 1\) A1
eg \({S_\infty } = \frac{{ – 9}}{{1 – \left( { – \frac{2}{3}} \right)}},{\text{ }}\frac{{ – 9}}{{\frac{5}{3}}}\)
\({S_\infty } = – \frac{{27}}{5}{\text{ }}( = – 5.4)\) A1 N3
[4 marks]
Examiners report
[N/A]
[N/A]
[N/A]
[N/A]
[N/A]
[N/A]
Question
In an arithmetic sequence, the third term is 10 and the fifth term is 16.
Find the common difference.
Find the first term.
Find the sum of the first 20 terms of the sequence.
Answer/Explanation
Markscheme
attempt to find \(d\) (M1)
eg \(\frac{{16 – 10}}{2}{\text{, }}10 – 2d = 16 – 4d{\text{, }}2d = 6{\text{, }}d = 6\)
\(d = 3\) A1 N2
[2 marks]
correct approach (A1)
eg \(10 = {u_1} + 2 \times 3{\text{, }}10 – 3 – 3\)
\({u_1} = 4\) A1 N2
[2 marks]
correct substitution into sum or term formula (A1)
eg \(\frac{{20}}{2}(2 \times 4 + 19 \times 3){\text{, }}{u_{20}} = 4 + 19 \times 3\)
correct simplification (A1)
eg \(8 + 57{\text{, }}4 + 61\)
\({S_{20}} = 650\) A1 N2
[3 marks]
Examiners report
[N/A]
[N/A]
[N/A]
Question
The sides of a square are 16 cm in length. The midpoints of the sides of this square are joined to form a new square and four triangles (diagram 1). The process is repeated twice, as shown in diagrams 2 and 3.

Let \({x_n}\) denote the length of one of the equal sides of each new triangle.
Let \({A_n}\) denote the area of each new triangle.
The following table gives the values of \({x_n}\) and \({A_n}\), for \(1 \leqslant n \leqslant 3\). Copy and complete the table. (Do not write on this page.)
| \(n\) | 1 | 2 | 3 |
| \({x_n}\) | 8 | 4 | |
| \({A_n}\) | 32 | 16 |
The process described above is repeated. Find \({A_6}\).
Consider an initial square of side length \(k {\text{ cm}}\). The process described above is repeated indefinitely. The total area of the shaded regions is \(k {\text{ c}}{{\text{m}}^2}\). Find the value of \(k\).
Answer/Explanation
Markscheme
valid method for finding side length (M1)
eg \({8^2} + {8^2} = {c^2},{\text{ }}45 – 45 – 90{\text{ side ratios, }}8\sqrt 2 ,{\text{ }}\frac{1}{2}{s^2} = 16,{\text{ }}{x^2} + {x^2} = {8^2}\)
correct working for area (A1)
eg \(\frac{1}{2} \times 4 \times 4\)
| \(n\) | 1 | 2 | 3 |
| \({x_n}\) | 8 | \(\sqrt {32}\) | 4 |
| \({A_n}\) | 32 | 16 | 8 |
A1A1 N2N2
[4 marks]
METHOD 1
recognize geometric progression for \({A_n}\) (R1)
eg \({u_n} = {u_1}{r^{n – 1}}\)
\(r = \frac{1}{2}\) (A1)
correct working (A1)
eg \(32{\left( {\frac{1}{2}} \right)^5};{\text{ 4, 2, 1, }}\frac{1}{2},{\text{ }}\frac{1}{4},{\text{ }} \ldots \)
\({A_6} = 1\) A1 N3
METHOD 2
attempt to find \({x_6}\) (M1)
eg \(8{\left( {\frac{1}{{\sqrt 2 }}} \right)^5},{\text{ }}2\sqrt 2 ,{\text{ 2, }}\sqrt 2 ,{\text{ 1, }} \ldots \)
\({x_6} = \sqrt 2 \) (A1)
correct working (A1)
eg \(\frac{1}{2}{\left( {\sqrt 2 } \right)^2}\)
\({A_6} = 1\) A1 N3
[4 marks]
METHOD 1
recognize infinite geometric series (R1)
eg \({S_n} = \frac{a}{{1 – r}},{\text{ }}\left| r \right| < 1\)
area of first triangle in terms of \(k\) (A1)
eg \(\frac{1}{2}{\left( {\frac{k}{2}} \right)^2}\)
attempt to substitute into sum of infinite geometric series (must have \(k\)) (M1)
eg \(\frac{{\frac{1}{2}{{\left( {\frac{k}{2}} \right)}^2}}}{{1 – \frac{1}{2}}},{\text{ }}\frac{k}{{1 – \frac{1}{2}}}\)
correct equation A1
eg \(\frac{{\frac{1}{2}{{\left( {\frac{k}{2}} \right)}^2}}}{{1 – \frac{1}{2}}} = k,{\text{ }}k = \frac{{\frac{{{k^2}}}{8}}}{{\frac{1}{2}}}\)
correct working (A1)
eg \({k^2} = 4k\)
valid attempt to solve their quadratic (M1)
eg \(k(k – 4),{\text{ }}k = 4{\text{ or }}k = 0\)
\(k = 4\) A1 N2
METHOD 2
recognizing that there are four sets of infinitely shaded regions with equal area R1
area of original square is \({k^2}\) (A1)
so total shaded area is \(\frac{{{k^2}}}{4}\) (A1)
correct equation \(\frac{{{k^2}}}{4} = k\) A1
\({k^2} = 4k\) (A1)
valid attempt to solve their quadratic (M1)
eg \(k(k – 4),{\text{ }}k = 4{\text{ or }}k = 0\)
\(k = 4\) A1 N2
[7 marks]
Examiners report
[N/A]
[N/A]
[N/A]
Question
The sums of the terms of a sequence follow the pattern
\({S_1} = 1 + k,{\text{ }}{S_2} = 5 + 3k,{\text{ }}{S_3} = 12 + 7k,{\text{ }}{S_4} = 22 + 15k,{\text{ }} \ldots ,{\text{ where }}k \in \mathbb{Z}.\)
Given that \({u_1} = 1 + k\), find \({u_2},{\text{ }}{u_3}\) and \({u_4}\).
Find a general expression for \({u_n}\).
Answer/Explanation
Markscheme
valid method (M1)
eg \({u_2} = {S_2} – {S_1},{\text{ }}1 + k + {u_2} = 5 + 3k\)
\({u_2} = 4 + 2k,{\text{ }}{u_3} = 7 + 4k,{\text{ }}{u_4} = 10 + 8k\) A1A1A1 N4
[4 marks]
correct AP or GP (A1)
eg finding common difference is \(3\), common ratio is \(2\)
valid approach using arithmetic and geometric formulas (M1)
eg \(1 + 3(n – 1)\) and \({r^{n – 1}}k\)
\({u_n} = 3n – 2 + {2^{n – 1}}k\) A1A1 N4
Note: Award A1 for \(3n – 2\), A1 for \({2^{n – 1}}k\).
[4 marks]
Question
In an arithmetic sequence, the first term is \(2\) and the second term is \(5\).
Find the common difference.
Find the eighth term.
Find the sum of the first eight terms of the sequence.
Answer/Explanation
Markscheme
correct approach (A1)
eg\(\;\;\;d = {u_2} – {u_1},{\text{ }}5 – 2\)
\(d = 3\) A1 N2
[2 marks]
correct approach (A1)
eg\(\;\;\;{u_8} = 2 + 7 \times 3\), listing terms
\({u_8} = 23\) A1 N2
[2 marks]
correct approach (A1)
eg\(\;\;\;{S_8} = \frac{8}{2}(2 + 23)\), listing terms, \(\frac{8}{2}\left( {2(2) + 7(3)} \right)\)
\({S_8} = 100\) A1 N2
[2 marks]
Total [6 marks]
Question
Ann and Bob play a game where they each have an eight-sided die. Ann’s die has three green faces and five red faces; Bob’s die has four green faces and four red faces. They take turns rolling their own die and note what colour faces up. The first player to roll green wins. Ann rolls first. Part of a tree diagram of the game is shown below.

Find the probability that Ann wins on her first roll.
(i) The probability that Ann wins on her third roll is \(\frac{5}{8} \times \frac{4}{8} \times p \times q\ \times \frac{3}{8}\).
Write down the value of \(p\) and of \(q\).
(ii) The probability that Ann wins on her tenth roll is \(\frac{3}{8}{r^k}\) where \(r \in \mathbb{Q},{\text{ }}k \in \mathbb{Z}\).
Find the value of \(r\) and of \(k\).
Find the probability that Ann wins the game.
Answer/Explanation
Markscheme
recognizing Ann rolls green (M1)
eg\(\;\;\;{\text{P(G)}}\)
\(\frac{3}{8}\) A1 N2
[2 marks]
(i) \(p = \frac{4}{8},{\text{ }}q = \frac{5}{8}\) or \(q = \frac{4}{8},{\text{ }}p = \frac{5}{8}\) A1A1 N2
(ii) recognizes Ann and Bob lose \(9\) times (M1)
eg\(\;\;\;\(\overbrace {{A_L}{B_\(\overbrace {{A_L}{B_ \ldots \(\overbrace {{A_L}{B_{\text{ 9 times, }}\underbrace {\left( {\frac{5}{8} \times \frac{4}{8}} \right) \times \ldots \times \left( {\frac{5}{8} \times \frac{4}{8}} \right)}_{{\text{9 times}}}\)
\(k = 9\;\;\;\)(seen anywhere) A1 N2
correct working (A1)
eg\(\;\;\;{\left( {\frac{5}{8} \times \frac{4}{8}} \right)^9} \times \frac{3}{8},{\text{ }}\left( {\frac{5}{8} \times \frac{4}{8}} \right) \times \ldots \times \left( {\frac{5}{8} \times \frac{4}{8}} \right) \times \frac{3}{8}\)
\(r = \frac{{20}}{{64}}\;\;\;\left( { = \frac{5}{{16}}} \right)\) A1 N2
[6 marks]
recognize the probability is an infinite sum (M1)
eg\(\;\;\;\)Ann wins on her \({{\text{1}}^{{\text{st}}}}\) roll or \({{\text{2}}^{{\text{nd}}}}\) roll or \({{\text{3}}^{{\text{rd}}}}\) roll…, \({S_\infty }\)
recognizing GP (M1)
\({u_1} = \frac{3}{8}\;\;\;\)(seen anywhere) A1
\(r = \frac{{20}}{{64}}\;\;\;\)(seen anywhere) A1
correct substitution into infinite sum of GP A1
eg\(\;\;\;\frac{{\frac{3}{8}}}{{1 – \frac{5}{{16}}}},{\text{ }}\frac{3}{8}\left( {\frac{1}{{1 – \left( {\frac{5}{8} \times \frac{4}{8}} \right)}}} \right),{\text{ }}\frac{1}{{1 – \frac{5}{{16}}}}\)
correct working (A1)
eg\(\;\;\;\frac{{\frac{3}{8}}}{{\frac{{11}}{{16}}}},{\text{ }}\frac{3}{8} \times \frac{{16}}{{11}}\)
\({\text{P (Ann wins)}} = \frac{{48}}{{88}}\;\;\;\left( { = \frac{6}{{11}}} \right)\) A1 N1
[7 marks]
Total [15 marks]
Question
An arithmetic sequence has the first term \(\ln a\) and a common difference \(\ln 3\).
The 13th term in the sequence is \(8\ln 9\). Find the value of \(a\).
Answer/Explanation
Markscheme
Note: There are many approaches to this question, and the steps may be done in any order. There are 3 relationships they may need to apply at some stage, for the 3rd, 4th and 5th marks. These are
equating bases eg recognising 9 is \({{\text{3}}^2}\)
log rules: \(\ln b + \ln c = \ln (bc),{\text{ }}\ln b – \ln c = \ln \left( {\frac{b}{c}} \right)\),
exponent rule: \(\ln {b^n} = n\ln b\).
The exception to the FT rule applies here, so that if they demonstrate correct application of the 3 relationships, they may be awarded the A marks, even if they have made a previous error. However all applications of a relationship need to be correct. Once an error has been made, do not award A1FT for their final answer, even if it follows from their working.
Please check working and award marks in line with the markscheme.
correct substitution into \({u_{13}}\) formula (A1)
eg\(\;\;\;\ln a + (13 – 1)\ln 3\)
set up equation for \({u_{13}}\) in any form (seen anywhere) (M1)
eg\(\;\;\;\ln a + 12\ln 3 = 8\ln 9\)
correct application of relationships (A1)(A1)(A1)
\(a = 81\) A1 N3
[6 marks]
Examples of application of relationships
Example 1
correct application of exponent rule for logs (A1)
eg\(\;\;\;\ln a + \ln {3^{12}} = \ln {9^8}\)
correct application of addition rule for logs (A1)
eg\(\;\;\;\ln (a{3^{12}}) = \ln {9^8}\)
substituting for 9 or 3 in ln expression in equation (A1)
eg\(\;\;\;\ln (a{3^{12}}) = \ln {3^{16}},{\text{ }}\ln (a{9^6}) = \ln {9^8}\)
Example 2
recognising \(9 = {3^2}\) (A1)
eg\(\;\;\;\ln a + 12\ln 3 = 8\ln {3^2},{\text{ }}\ln a + 12\ln {9^{\frac{1}{2}}} = 8\ln 9\)
one correct application of exponent rule for logs relating \(\ln 9\) to \(\ln 3\) (A1)
eg\(\;\;\;\ln a + 12\ln 3 = 16\ln 3,{\text{ }}\ln a + 6\ln 9 = 8\ln 9\)
another correct application of exponent rule for logs (A1)
eg\(\;\;\;\ln a = \ln {3^4},{\text{ }}\ln a = \ln {9^2}\)
Question
Consider the following sequence of figures.
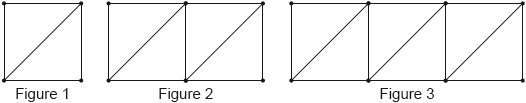
Figure 1 contains 5 line segments.
Given that Figure \(n\) contains 801 line segments, show that \(n = 200\).
Find the total number of line segments in the first 200 figures.
Answer/Explanation
Markscheme
recognizing that it is an arithmetic sequence (M1)
eg\(\,\,\,\,\,\)\(5,{\text{ }}5 + 4,{\text{ }}5 + 4 + 4,{\text{ }} \ldots ,{\text{ }}d = 4,{\text{ }}{u_n} = {u_1} + (n – 1)d,{\text{ }}4n + 1\)
correct equation A1
eg\(\,\,\,\,\,\)\(5 + 4(n – 1) = 801\)
correct working (do not accept substituting \(n = 200\)) A1
eg\(\,\,\,\,\,\)\(4n – 4 = 796,{\text{ }}n – 1 = \frac{{796}}{4}\)
\(n = 200\) AG N0
[3 marks]
recognition of sum (M1)
eg\(\,\,\,\,\,\)\({S_{200}},{\text{ }}{u_1} + {u_2} + \ldots + {u_{200}},{\text{ }}5 + 9 + 13 + \ldots + 801\)
correct working for AP (A1)
eg\(\,\,\,\,\,\)\(\frac{{200}}{2}(5 + 801),{\text{ }}\frac{{200}}{2}{\text{ }}\left( {2(5) + 199(4)} \right)\)
\(80\,600\) A1 N2
[3 marks]
Question
Three consecutive terms of a geometric sequence are \(x – 3\), 6 and \(x + 2\).
Find the possible values of \(x\).
Answer/Explanation
Markscheme
METHOD 1
valid approach (M1)
eg\(\,\,\,\,\,\)\(r = \frac{6}{{x – 3}},{\text{ }}(x – 3) \times r = 6,{\text{ }}(x – 3){r^2} = x + 2\)
correct equation in terms of \(x\) only A1
eg\(\,\,\,\,\,\)\(\frac{6}{{x – 3}} = \frac{{x + 2}}{6},{\text{ }}(x – 3)(x + 2) = {6^2},{\text{ }}36 = {x^2} – x – 6\)
correct working (A1)
eg\(\,\,\,\,\,\)\({x^2} – x – 42,{\text{ }}{x^2} – x = 42\)
valid attempt to solve their quadratic equation (M1)
eg\(\,\,\,\,\,\)factorizing, formula, completing the square
evidence of correct working (A1)
eg\(\,\,\,\,\,\)\((x – 7)(x + 6),{\text{ }}\frac{{1 \pm \sqrt {169} }}{2}\)
\(x = 7,{\text{ }}x = – 6\) A1 N4
METHOD 2 (finding r first)
valid approach (M1)
eg\(\,\,\,\,\,\)\(r = \frac{6}{{x – 3}},{\text{ }}6r = x + 2,{\text{ }}(x – 3){r^2} = x + 2\)
correct equation in terms of \(r\) only A1
eg\(\,\,\,\,\,\)\(\frac{6}{r} + 3 = 6r – 2,{\text{ }}6 + 3r = 6{r^2} – 2r,{\text{ }}6{r^2} – 5r – 6 = 0\)
evidence of correct working (A1)
eg\(\,\,\,\,\,\)\((3r + 2)(2r – 3),{\text{ }}\frac{{5 \pm \sqrt {25 + 144} }}{{12}}\)
\(r = – \frac{2}{3},{\text{ }}r = \frac{3}{2}\) A1
substituting their values of \(r\) to find \(x\) (M1)
eg\(\,\,\,\,\,\)\((x – 3)\left( {\frac{2}{3}} \right) = 6,{\text{ }}x = 6\left( {\frac{3}{2}} \right) – 2\)
\(x = 7,{\text{ }}x = – 6\) A1 N4
[6 marks]
Question
The first two terms of an infinite geometric sequence, in order, are
\(2{\log _2}x,{\text{ }}{\log _2}x\), where \(x > 0\).
The first three terms of an arithmetic sequence, in order, are
\({\log _2}x,{\text{ }}{\log _2}\left( {\frac{x}{2}} \right),{\text{ }}{\log _2}\left( {\frac{x}{4}} \right)\), where \(x > 0\).
Let \({S_{12}}\) be the sum of the first 12 terms of the arithmetic sequence.
Find \(r\).
Show that the sum of the infinite sequence is \(4{\log _2}x\).
Find \(d\), giving your answer as an integer.
Show that \({S_{12}} = 12{\log _2}x – 66\).
Given that \({S_{12}}\) is equal to half the sum of the infinite geometric sequence, find \(x\), giving your answer in the form \({2^p}\), where \(p \in \mathbb{Q}\).
Answer/Explanation
Markscheme
evidence of dividing terms (in any order) (M1)
eg\(\,\,\,\,\,\)\(\frac{{{\mu _2}}}{{{\mu _1}}},{\text{ }}\frac{{2{{\log }_2}x}}{{{{\log }_2}x}}\)
\(r = \frac{1}{2}\) A1 N2
[2 marks]
correct substitution (A1)
eg\(\,\,\,\,\,\)\(\frac{{2{{\log }_2}x}}{{1 – \frac{1}{2}}}\)
correct working A1
eg\(\,\,\,\,\,\)\(\frac{{2{{\log }_2}x}}{{\frac{1}{2}}}\)
\({S_\infty } = 4{\log _2}x\) AG N0
[2 marks]
evidence of subtracting two terms (in any order) (M1)
eg\(\,\,\,\,\,\)\({u_3} – {u_2},{\text{ }}{\log _2}x – {\log _2}\frac{x}{2}\)
correct application of the properties of logs (A1)
eg\(\,\,\,\,\,\)\({\log _2}\left( {\frac{{\frac{x}{2}}}{x}} \right),{\text{ }}{\log _2}\left( {\frac{x}{2} \times \frac{1}{x}} \right),{\text{ }}({\log _2}x – {\log _2}2) – {\log _2}x\)
correct working (A1)
eg\(\,\,\,\,\,\)\({\log _2}\frac{1}{2},{\text{ }} – {\log _2}2\)
\(d = – 1\) A1 N3
[4 marks]
correct substitution into the formula for the sum of an arithmetic sequence (A1)
eg\(\,\,\,\,\,\)\(\frac{{12}}{2}\left( {2{{\log }_2}x + (12 – 1)( – 1)} \right)\)
correct working A1
eg\(\,\,\,\,\,\)\(6(2{\log _2}x – 11),{\text{ }}\frac{{12}}{2}(2{\log _2}x – 11)\)
\(12{\log _2}x – 66\) AG N0
[2 marks]
correct equation (A1)
eg\(\,\,\,\,\,\)\(12{\log _2}x – 66 = 2{\log _2}x\)
correct working (A1)
eg\(\,\,\,\,\,\)\(10{\log _2}x = 66,{\text{ }}{\log _2}x = 6.6,{\text{ }}{2^{66}} = {x^{10}},{\text{ }}{\log _2}\left( {\frac{{{x^{12}}}}{{{x^2}}}} \right) = 66\)
\(x = {2^{6.6}}\) (accept \(p = \frac{{66}}{{10}}\)) A1 N2
[3 marks]
Question
The first three terms of a geometric sequence are \(\ln {x^{16}}\), \(\ln {x^8}\), \(\ln {x^4}\), for \(x > 0\).
Find the common ratio.
Solve \(\sum\limits_{k = 1}^\infty {{2^{5 – k}}\ln x = 64} \).
Answer/Explanation
Markscheme
correct use \(\log {x^n} = n\log x\) A1
eg\(\,\,\,\,\,\)\(16\ln x\)
valid approach to find \(r\) (M1)
eg\(\,\,\,\,\,\)\(\frac{{{u_{n + 1}}}}{{{u_n}}},{\text{ }}\frac{{\ln {x^8}}}{{\ln {x^{16}}}},{\text{ }}\frac{{4\ln x}}{{8\ln x}},{\text{ }}\ln {x^4} = \ln {x^{16}} \times {r^2}\)
\(r = \frac{1}{2}\) A1 N2
[3 marks]
recognizing a sum (finite or infinite) (M1)
eg\(\,\,\,\,\,\)\({2^4}\ln x + {2^3}\ln x,{\text{ }}\frac{a}{{1 – r}},{\text{ }}{S_\infty },{\text{ }}16\ln x + \ldots \)
valid approach (seen anywhere) (M1)
eg\(\,\,\,\,\,\)recognizing GP is the same as part (a), using their \(r\) value from part (a), \(r = \frac{1}{2}\)
correct substitution into infinite sum (only if \(\left| r \right|\) is a constant and less than 1) A1
eg\(\,\,\,\,\,\)\(\frac{{{2^4}\ln x}}{{1 – \frac{1}{2}}},{\text{ }}\frac{{\ln {x^{16}}}}{{\frac{1}{2}}},{\text{ }}32\ln x\)
correct working (A1)
eg\(\,\,\,\,\,\)\(\ln x = 2\)
\(x = {{\text{e}}^2}\) A1 N3
[5 marks]
Question
In an arithmetic sequence, the first term is 3 and the second term is 7.
Find the common difference.
Find the tenth term.
Find the sum of the first ten terms of the sequence.
Answer/Explanation
Markscheme
attempt to subtract terms (M1)
eg\(\,\,\,\,\,\)\(d = {u_2} – {u_1},{\text{ }}7 – 3\)
\(d = 4\) A1 N2
[2 marks]
correct approach (A1)
eg\(\,\,\,\,\,\)\({u_{10}} = 3 + 9(4)\)
\({u_{10}} = 39\) A1 N2
[2 marks]
correct substitution into sum (A1)
eg\(\,\,\,\,\,\)\({S_{10}} = 5(3 + 39),{\text{ }}{S_{10}} = \frac{{10}}{2}(2 \times 3 + 9 \times 4)\)
\({S_{10}} = 210\) A1 N2
[2 marks]
Question
In an arithmetic sequence, the first term is 8 and the second term is 5.
Find the common difference.
Find the tenth term.
Find the sum of the first ten terms.
Answer/Explanation
Markscheme
subtracting terms (M1)
eg\(\,\,\,\,\,\)\(5 – 8,{\text{ }}{u_2} – {u_1}\)
\(d = – 3\) A1 N2
[2 marks]
correct substitution into formula (A1)
eg\(\,\,\,\,\,\)\({u_{10}} = 8 + (10 – 1)( – 3),{\text{ }}8 – 27,{\text{ }} – 3(10) + 11\)
\({u_{10}} = – 19\) A1 N2
[2 marks]
correct substitution into formula for sum (A1)
eg\(\,\,\,\,\,\)\({S_{10}} = \frac{{10}}{2}(8 – 19),{\text{ 5}}\left( {2(8) + (10 – 1)( – 3)} \right)\)
\({S_{10}} = – 55\) A1 N2
[2 marks]
Question
The following diagram shows [AB], with length 2 cm. The line is divided into an infinite number of line segments. The diagram shows the first three segments.
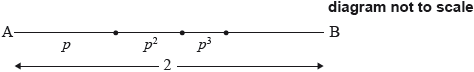
The length of the line segments are \(p{\text{ cm}},{\text{ }}{p^2}{\text{ cm}},{\text{ }}{p^3}{\text{ cm}},{\text{ }} \ldots \), where \(0 < p < 1\).
Show that \(p = \frac{2}{3}\).
The following diagram shows [CD], with length \(b{\text{ cm}}\), where \(b > 1\). Squares with side lengths \(k{\text{ cm}},{\text{ }}{k^2}{\text{ cm}},{\text{ }}{k^3}{\text{ cm}},{\text{ }} \ldots \), where \(0 < k < 1\), are drawn along [CD]. This process is carried on indefinitely. The diagram shows the first three squares.
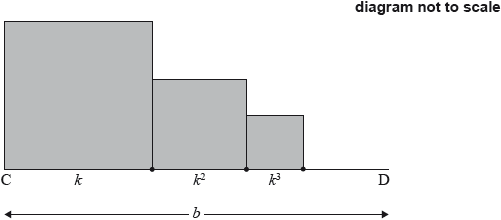
The total sum of the areas of all the squares is \(\frac{9}{{16}}\). Find the value of \(b\).
Answer/Explanation
Markscheme
infinite sum of segments is 2 (seen anywhere) (A1)
eg\(\,\,\,\,\,\)\(p + {p^2} + {p^3} + \ldots = 2,{\text{ }}\frac{{{u_1}}}{{1 – r}} = 2\)
recognizing GP (M1)
eg\(\,\,\,\,\,\)ratio is \(p,{\text{ }}\frac{{{u_1}}}{{1 – r}},{\text{ }}{u_n} = {u_1} \times {r^{n – 1}},{\text{ }}\frac{{{u_1}({r^n} – 1)}}{{r – 1}}\)
correct substitution into \({S_\infty }\) formula (may be seen in equation) A1
eg\(\,\,\,\,\,\)\(\frac{p}{{1 – p}}\)
correct equation (A1)
eg\(\,\,\,\,\,\)\(\frac{p}{{1 – p}} = 2,{\text{ }}p = 2 – 2p\)
correct working leading to answer A1
eg\(\,\,\,\,\,\)\(3p = 2,{\text{ }}2 – 3p = 0\)
\(p = \frac{2}{3}{\text{ (cm)}}\) AG N0
[5 marks]
recognizing infinite geometric series with squares (M1)
eg\(\,\,\,\,\,\)\({k^2} + {k^4} + {k^6} + \ldots ,{\text{ }}\frac{{{k^2}}}{{1 – {k^2}}}\)
correct substitution into \({S_\infty } = \frac{9}{{16}}\) (must substitute into formula) (A2)
eg\(\,\,\,\,\,\)\(\frac{{{k^2}}}{{1 – {k^2}}} = \frac{9}{{16}}\)
correct working (A1)
eg\(\,\,\,\,\,\)\(16{k^2} = 9 – 9{k^2},{\text{ }}25{k^2} = 9,{\text{ }}{k^2} = \frac{9}{{25}}\)
\(k = \frac{3}{5}\) (seen anywhere) A1
valid approach with segments and CD (may be seen earlier) (M1)
eg\(\,\,\,\,\,\)\(r = k,{\text{ }}{S_\infty } = b\)
correct expression for \(b\) in terms of \(k\) (may be seen earlier) (A1)
eg\(\,\,\,\,\,\)\(b = \frac{k}{{1 – k}},{\text{ }}b = \sum\limits_{n = 1}^\infty {{k^n},{\text{ }}b = k + {k^2} + {k^3} + \ldots } \)
substituting their value of \(k\) into their formula for \(b\) (M1)
eg\(\,\,\,\,\,\)\(\frac{{\frac{3}{5}}}{{1 – \frac{3}{5}}},{\text{ }}\frac{{\left( {\frac{3}{5}} \right)}}{{\left( {\frac{2}{5}} \right)}}\)
\(b = \frac{3}{2}\) A1 N3
[9 marks]
Question
The first two terms of an infinite geometric sequence are u1 = 18 and u2 = 12sin2 θ , where 0 < θ < 2\(\pi \) , and θ ≠ \(\pi \).
Find an expression for r in terms of θ.
Find the possible values of r.
Show that the sum of the infinite sequence is \(\frac{{54}}{{2 + {\text{cos}}\,\left( {2\theta } \right)}}\).
Find the values of θ which give the greatest value of the sum.
Answer/Explanation
Markscheme
valid approach (M1)
eg \(\frac{{{u_2}}}{{{u_1}}},\,\,\frac{{{u_1}}}{{{u_2}}}\)
\(r = \frac{{12\,{{\sin }^2}\,\theta }}{{18}}\left( { = \frac{{2\,{{\sin }^2}\,\theta }}{3}} \right)\) A1 N2
[2 marks]
recognizing that sinθ is bounded (M1)
eg 0 ≤ sin2 θ ≤ 1, −1 ≤ sinθ ≤ 1, −1 < sinθ < 1
0 < r ≤ \(\frac{2}{3}\) A2 N3
Note: If working shown, award M1A1 for correct values with incorrect inequality sign(s).
If no working shown, award N1 for correct values with incorrect inequality sign(s).
[3 marks]
correct substitution into formula for infinite sum A1
eg \(\frac{{18}}{{1 – \frac{{2\,{\text{si}}{{\text{n}}^2}\,\theta }}{3}}}\)
evidence of choosing an appropriate rule for cos 2θ (seen anywhere) (M1)
eg cos 2θ = 1 − 2 sin2 θ
correct substitution of identity/working (seen anywhere) (A1)
eg \(\frac{{18}}{{1 – \frac{2}{3}\left( {\frac{{1 – {\text{cos}}\,2\theta }}{2}} \right)}},\,\,\frac{{54}}{{3 – 2\left( {\frac{{1 – {\text{cos}}\,2\theta }}{2}} \right)}},\,\,\frac{{18}}{{\frac{{3 – 2\,{\text{si}}{{\text{n}}^2}\,\theta }}{3}}}\)
correct working that clearly leads to the given answer A1
eg \(\frac{{18 \times 3}}{{2 + \left( {1 – 2\,{\text{si}}{{\text{n}}^2}\,\theta } \right)}},\,\,\frac{{54}}{{3 – \left( {1 – {\text{cos}}\,2\theta } \right)}}\)
\(\frac{{54}}{{2 + {\text{cos}}\left( {2\theta } \right)}}\) AG N0
[4 marks]
METHOD 1 (using differentiation)
recognizing \(\frac{{{\text{d}}{S_\infty }}}{{{\text{d}}\theta }} = 0\) (seen anywhere) (M1)
finding any correct expression for \(\frac{{{\text{d}}{S_\infty }}}{{{\text{d}}\theta }}\) (A1)
eg \(\frac{{0 – 54 \times \left( { – 2\,{\text{sin}}\,2\,\theta } \right)}}{{{{\left( {2 + {\text{cos}}\,2\,\theta } \right)}^2}}},\,\, – 54{\left( {2 + {\text{cos}}\,2\,\theta } \right)^{ – 2}}\,\left( { – 2\,{\text{sin}}\,2\,\theta } \right)\)
correct working (A1)
eg sin 2θ = 0
any correct value for sin−1(0) (seen anywhere) (A1)
eg 0, \(\pi \), … , sketch of sine curve with x-intercept(s) marked both correct values for 2θ (ignore additional values) (A1)
2θ = \(\pi \), 3\(\pi \) (accept values in degrees)
both correct answers \(\theta = \frac{\pi }{2},\,\frac{{3\pi }}{2}\) A1 N4
Note: Award A0 if either or both correct answers are given in degrees.
Award A0 if additional values are given.
METHOD 2 (using denominator)
recognizing when S∞ is greatest (M1)
eg 2 + cos 2θ is a minimum, 1−r is smallest
correct working (A1)
eg minimum value of 2 + cos 2θ is 1, minimum r = \(\frac{2}{3}\)
correct working (A1)
eg \({\text{cos}}\,2\,\theta = – 1,\,\,\frac{2}{3}\,{\text{si}}{{\text{n}}^2}\,\theta = \frac{2}{3},\,\,{\text{si}}{{\text{n}}^2}\theta = 1\)
EITHER (using cos 2θ)
any correct value for cos−1(−1) (seen anywhere) (A1)
eg \(\pi \), 3\(\pi \), … (accept values in degrees), sketch of cosine curve with x-intercept(s) marked
both correct values for 2θ (ignore additional values) (A1)
2θ = \(\pi \), 3\(\pi \) (accept values in degrees)
OR (using sinθ)
sinθ = ±1 (A1)
sin−1(1) = \(\frac{\pi }{2}\) (accept values in degrees) (seen anywhere) A1
THEN
both correct answers \(\theta = \frac{\pi }{2},\,\frac{{3\pi }}{2}\) A1 N4
Note: Award A0 if either or both correct answers are given in degrees.
Award A0 if additional values are given.
[6 marks]
Question
An arithmetic sequence has \({u_1} = {\text{lo}}{{\text{g}}_c}\left( p \right)\) and \({u_2} = {\text{lo}}{{\text{g}}_c}\left( {pq} \right)\), where \(c > 1\) and \(p,\,\,q > 0\).
Show that \(d = {\text{lo}}{{\text{g}}_c}\left( q \right)\).
Let \(p = {c^2}\) and \(q = {c^3}\). Find the value of \(\sum\limits_{n = 1}^{20} {{u_n}} \).
Answer/Explanation
Markscheme
valid approach involving addition or subtraction M1
eg \({u_2} = {\text{lo}}{{\text{g}}_c}\,p + d,\,\,{u_1} – {u_2}\)
correct application of log law A1
eg \({\text{lo}}{{\text{g}}_c}\left( {pq} \right) = {\text{lo}}{{\text{g}}_c}\,p + {\text{lo}}{{\text{g}}_c}\,q,\,\,{\text{lo}}{{\text{g}}_c}\left( {\frac{{pq}}{p}} \right)\)
\(d = {\text{lo}}{{\text{g}}_c}\,q\) AG N0
[2 marks]
METHOD 1 (finding \({u_1}\) and d)
recognizing \(\sum { = {S_{20}}} \) (seen anywhere) (A1)
attempt to find \({u_1}\) or d using \({\text{lo}}{{\text{g}}_c}\,{c^k} = k\) (M1)
eg \({\text{lo}}{{\text{g}}_c}\,c\), \({\text{3}}\,{\text{lo}}{{\text{g}}_c}\,c\), correct value of \({u_1}\) or d
\({u_1}\) = 2, d = 3 (seen anywhere) (A1)(A1)
correct working (A1)
eg \({S_{20}} = \frac{{20}}{2}\left( {2 \times 2 + 19 \times 3} \right),\,\,{S_{20}} = \frac{{20}}{2}\left( {2 + 59} \right),\,\,10\left( {61} \right)\)
\(\sum\limits_{n = 1}^{20} {{u_n}} \) = 610 A1 N2
METHOD 2 (expressing S in terms of c)
recognizing \(\sum { = {S_{20}}} \) (seen anywhere) (A1)
correct expression for S in terms of c (A1)
eg \(10\left( {2\,{\text{lo}}{{\text{g}}_c}\,{c^2} + 19\,{\text{lo}}{{\text{g}}_c}\,{c^3}} \right)\)
\({\text{lo}}{{\text{g}}_c}\,{c^2} = 2,\,\,\,{\text{lo}}{{\text{g}}_c}\,{c^3} = 3\) (seen anywhere) (A1)(A1)
correct working (A1)
eg \({S_{20}} = \frac{{20}}{2}\left( {2 \times 2 + 19 \times 3} \right),\,\,{S_{20}} = \frac{{20}}{2}\left( {2 + 59} \right),\,\,10\left( {61} \right)\)
\(\sum\limits_{n = 1}^{20} {{u_n}} \) = 610 A1 N2
METHOD 3 (expressing S in terms of c)
recognizing \(\sum { = {S_{20}}} \) (seen anywhere) (A1)
correct expression for S in terms of c (A1)
eg \(10\left( {2\,{\text{lo}}{{\text{g}}_c}\,{c^2} + 19\,{\text{lo}}{{\text{g}}_c}\,{c^3}} \right)\)
correct application of log law (A1)
eg \(2\,{\text{lo}}{{\text{g}}_c}\,{c^2} = \,\,{\text{lo}}{{\text{g}}_c}\,{c^4},\,\,19\,{\text{lo}}{{\text{g}}_c}\,{c^3} = \,\,{\text{lo}}{{\text{g}}_c}\,{c^{57}},\,\,10\,\left( {{\text{lo}}{{\text{g}}_c}\,{{\left( {{c^2}} \right)}^2} + \,\,{\text{lo}}{{\text{g}}_c}\,{{\left( {{c^3}} \right)}^{19}}} \right),\,\,10\,\left( {{\text{lo}}{{\text{g}}_c}\,{c^4} + \,{\text{lo}}{{\text{g}}_c}\,{c^{57}}} \right),\,\,10\left( {{\text{lo}}{{\text{g}}_c}\,{c^{61}}} \right)\)
correct application of definition of log (A1)
eg \({\text{lo}}{{\text{g}}_c}\,{c^{61}} = 61,\,\,{\text{lo}}{{\text{g}}_c}\,{c^4} = 4,\,\,{\text{lo}}{{\text{g}}_c}\,{c^{57}} = 57\)
correct working (A1)
eg \({S_{20}} = \frac{{20}}{2}\left( {4 + 57} \right),\,\,10\left( {61} \right)\)
\(\sum\limits_{n = 1}^{20} {{u_n}} \) = 610 A1 N2
[6 marks]

