Question: [Maximum mark: 7]
Rachel and Sophia are competing in a javelin-throwing competition.
The distances, R metres, thrown by Rachel can be modelled by a normal distribution with mean 56.5 and standard deviation 3.
The distances, S metres, thrown by Sophia can be modelled by a normal distribution with mean 57.5 and standard deviation 1.8.
In the first round of competition, each competitor must have five throws. To qualify for the next round of competition, a competitor must record at least one throw of 60 metres or
greater in the first round.
Find the probability that only one of Rachel or Sophia qualifies for the next round of competition.
▶️Answer/Explanation
Ans:
Rachel: R ∼ N (56.5,32)
P(R≥60) = 0.1216….
Sophia: S ∼ N (57.5,82)
P(S≥60) = 0.0824….
recognises binomial distribution with n = 5
let NR represent the number of Rachel’s throws that are longer than 60 metres
NR ~ B (5,0.1216… )
either P(NR≥ 1) = 0.4772… or P(NR =0)= 0.5227…
let NS represent the number of Sophia’s throws that are longer than 60 metres
NS ~ B (5,0.0824…)
either P(NS ≥ 1) = 0.3495… or P(NS= 0) = 0.6504…

Note: M marks are not dependent on the previous A marks.
Question
The flight times, T minutes, between two cities can be modelled by a normal distribution with a mean of 75 minutes and a standard deviation of σ minutes.
(a) Given that 2 % of the flight times are longer than 82 minutes, find the value of σ . [3]
(b) Find the probability that a randomly selected flight will have a flight time of more than 80 minutes. [2]
(c) Given that a flight between the two cities takes longer than 80 minutes, find the probability that it takes less than 82 minutes. [4]
On a particular day, there are 64 flights scheduled between these two cities.
(d) Find the expected number of flights that will have a flight time of more than 80 minutes. [3]
(e) Find the probability that more than 6 of the flights on this particular day will have a flight time of more than 80 minutes. [3]
▶️Answer/Explanation
Ans:
(a) $T\sim \text{N}\left(75,\sigma ^2\right)$.
Since $\text{P}\left(T\gt 82\right)=0.02$, we have $$\begin{eqnarray} \text{P}\left(T\lt 82\right)=0.98 \nonumber \\ \text{P}\left(\frac{T-75}{\sigma}\lt \frac{82-75}{\sigma}\right)=0.98 \nonumber \\ \text{P}\left(Z\lt \frac{82-75}{\sigma}\right)=0.98 \nonumber \\ \frac{82-75}{\sigma} = 2.05375 \nonumber \\ \sigma = 3.41. \end{eqnarray}$$ (b) From the graphing calculator, we have $\text{P}\left(T\gt 80\right)=0.0712$.
(c) $$\begin{eqnarray} \text{P}\left(T\lt 82|T\gt 80\right) &=& \frac{\text{P}\left(T\lt 82\cap T\gt 80\right)}{\text{P}\left(T\gt 80\right)} \nonumber \\ &=& \frac{\text{P}\left(80\lt T\lt 82\right)}{\text{P}\left(T\gt 80\right)} \nonumber \\ &=& 0.719. \end{eqnarray}$$ (d) Let $F$ be the number of flights with a flight time of more than $80$ minutes in $64$ flights, then $F\sim \text{B}\left(64,\text{P}\left(T\gt 80\right)\right)$.
$$\begin{eqnarray} \text{E}\left(F\right) &=& 64\text{P}\left(T\gt 80\right) \nonumber \\ &=& 4.56. \end{eqnarray}$$ (e) $$\begin{eqnarray} \text{P}\left(F\gt 6\right) &=& 1-\text{P}\left(F\leq 6\right) \nonumber \\ &=& 0.169. \end{eqnarray}$$
Question
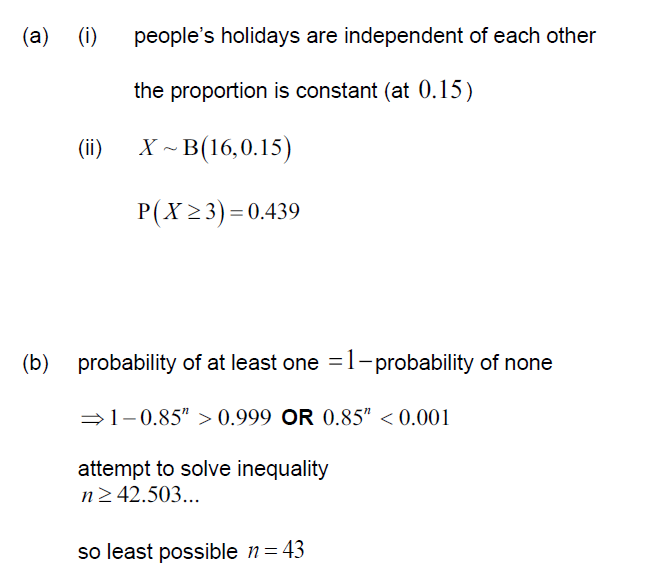
A survey of British holidaymakers found that 15% of those surveyed took a holiday in the Lake District in 2019.
A random sample of 16 British holidaymakers was taken. The number of people in the sample who took a holiday in the Lake District in 2019 can be modelled by a binomial distribution.
State two assumptions made in order for this model to be valid.
Find the probability that at least three people from the sample took a holiday in the Lake District in 2019. [4]
From a random sample of n holidaymakers, the probability that at least one of them took a holiday in the Lake District in 2019 is greater than 0.999.Determine the least possible value of n . [3]
▶️Answer/Explanation
Ans:
Question
Tom, Aayush and Maya decide to observe the colour of each car as it enters their school.
The colours of the cars are assumed to be independent of one another.
The probability that a car entering their school is red is \(\frac{1}{12}\)
Tom observes 20 cars. Find the probability that at least three of these cars are red. [2]
Aayush observes cars until a red one enters. Find the probability that he observes at least six cars. [2]
Maya observes cars until three red cars enter.
Find the probability that Maya observes exactly 20 cars.
Find the expected number of cars that Maya observes. [4]
▶️Answer/Explanation
Ans:
(a)
let X = number of red cars x
\(\sim B(20,\frac{1}{12})\)
\(p(x\geq 3)\)= 0.230
(b)
METHOD 1
let Y = number of cars observed
\(Y \sim Geo(\frac{1}{12})\)
\(p(y\geq 6)\)= 0.647
METHOD 2
P(first five cars not red) =
\((\frac{11}{12}^{5})\)= 0.647
(c)
let Z = number of cars observed Z \(\sim NB (3,\frac{1}{12})\)
(i)
P(Z=20)=\((_{2}^{19})(\frac{11}{12}^{17})(\frac{1}{12}^{3})\)= 0.0225
(ii) 36
Question
The lifts in the office buildings of a small city have occasional breakdowns. The breakdowns at any given time are independent of one another and can be modelled using a Poisson Distribution with mean 0.2 per day.
(a) Determine the probability that there will be exactly four breakdowns during the month of June (June has 30 days).
(b) Determine the probability that there are more than 3 breakdowns during the month of June.
(c) Determine the probability that there are no breakdowns during the first five days of June.
(d) Find the probability that the first breakdown in June occurs on June \({3^{{\text{rd}}}}\).
(e) It costs 1850 Euros to service the lifts when they have breakdowns. Find the expected cost of servicing lifts for the month of June.
(f) Determine the probability that there will be no breakdowns in exactly 4 out of the first 5 days in June.
▶️Answer/Explanation
Markscheme
(a) mean for 30 days: \(30 \times 0.2 = 6\) . (A1)
\({\text{P}}(X = 4) = \frac{{{6^4}}}{{4!}}{{\text{e}}^{ – 6}} = 0.134\) (M1)A1 N3
[3 marks]
(b) \({\text{P}}(X > 3) = 1 – {\text{P}}(X \leqslant 3) = 1 – {{\text{e}}^{ – 6}}(1 + 6 + 18 + 36) = 0.849\) (M1)A1 N2
[2 marks]
(c) EITHER
mean for five days: \(5 \times 0.2 = 1\) (A1)
\({\text{P}}(X = 0) = {{\text{e}}^{ – 1}}\,\,\,\,\,( = 0.368)\) A1 N2
OR
mean for one day: 0.2 (A1)
\({\text{P}}(X = 0) = {({{\text{e}}^{ – 0.2}})^5} = {{\text{e}}^{ – 1}}\,\,\,\,\,( = 0.368)\) A1 N2
[2 marks]
(d) Required probability \( = {{\text{e}}^{ – 0.2}} \times {{\text{e}}^{ – 0.2}} \times (1 – {{\text{e}}^{ – 0.2}})\) M1A1
= 0.122 A1 N3
[3 marks]
(e) Expected cost is \(1850 \times 6 = {\text{11}}\,{\text{100 Euros}}\) A1
[1 mark]
(f) On any one day \({\text{P}}(X = 0) = {{\text{e}}^{ – 0.2}}\)
Therefore, \(\left( {\begin{array}{*{20}{c}}
5 \\
1
\end{array}} \right){({{\text{e}}^{ – 0.2}})^4}(1 – {{\text{e}}^{ – 0.2}}) = 0.407\) M1A1 N2
[2 marks]
Total [13 marks]
Question
Over a one month period, Ava and Sven play a total of n games of tennis.
The probability that Ava wins any game is 0.4. The result of each game played is independent of any other game played.
Let X denote the number of games won by Ava over a one month period.
(a) Find an expression for P(X = 2) in terms of n.
(b) If the probability that Ava wins two games is 0.121 correct to three decimal places, find the value of n.
▶️Answer/Explanation
Markscheme
(a) \(X \sim {\text{B}}(n,{\text{ }}0.4)\) (A1)
Using \({\text{P}}(X = x) = \left( {\begin{array}{*{20}{c}}
n \\
r
\end{array}} \right){(0.4)^x}{(0.6)^{n – x}}\) (M1)
\({\text{P}}(X = 2) = \left( {\begin{array}{*{20}{c}}
n \\
2
\end{array}} \right){(0.4)^2}{(0.6)^{n – 2}}\) \(\left( { = \frac{{n(n – 1)}}{2}{{(0.4)}^2}{{(0.6)}^{n – 2}}} \right)\) A1 N3
(b) P(X = 2) = 0.121 A1
Using an appropriate method (including trial and error) to solve their equation. (M1)
n = 10 A1 N2
Note: Do not award the last A1 if any other solution is given in their final answer.
[6 marks]
Examiners report
Part (a) was generally well done. The most common error was to omit the binomial coefficient i.e. not identifying that the situation is described by a binomial distribution.
Finding the correct value of n in part (b) proved to be more elusive. A significant proportion of candidates attempted algebraic approaches and seemingly did not realise that the equation could only be solved numerically. Candidates who obtained n = 10 often accomplished this by firstly attempting to solve the equation algebraically before ‘resorting’ to a GDC approach. Some candidates did not specify their final answer as an integer while others stated n = 1.76 as their final answer.
Question
(a) A box of biscuits is considered to be underweight if it weighs less than 228 grams. It is known that the weights of these boxes of biscuits are normally distributed with a mean of 231 grams and a standard deviation of 1.5 grams.
What is the probability that a box is underweight?
(b) The manufacturer decides that the probability of a box being underweight should be reduced to 0.002.
(i) Bill’s suggestion is to increase the mean and leave the standard deviation unchanged. Find the value of the new mean.
(ii) Sarah’s suggestion is to reduce the standard deviation and leave the mean unchanged. Find the value of the new standard deviation.
(c) After the probability of a box being underweight has been reduced to 0.002, a group of customers buys 100 boxes of biscuits. Find the probability that at least two of the boxes are underweight.
There are six boys and five girls in a school tennis club. A team of two boys and two girls will be selected to represent the school in a tennis competition.
(a) In how many different ways can the team be selected?
(b) Tim is the youngest boy in the club and Anna is the youngest girl. In how many different ways can the team be selected if it must include both of them?
(c) What is the probability that the team includes both Tim and Anna?
(d) Fred is the oldest boy in the club. Given that Fred is selected for the team, what is the probability that the team includes Tim or Anna, but not both?
▶️Answer/Explanation
Markscheme
(a) \(X \sim {\text{N(231, 1.}}{{\text{5}}^2})\)
\({\text{P}}(X < 228) = 0.0228\) (M1)A1
Note: Accept 0.0227.
[2 marks]
(b) (i) \(X \sim {\text{N(}}\mu {\text{, 1.}}{{\text{5}}^2})\)
\({\text{P}}(X < 228) = 0.002\)
\(\frac{{228 – \mu }}{{1.5}} = – 2.878…\) M1A1
\(\mu = 232{\text{ grams}}\) A1 N3
(ii) \(X \sim {\text{N(231, }}{\sigma ^2})\)
\(\frac{{228 – 231}}{\sigma } = – 2.878…\) M1A1
\(\sigma = 1.04{\text{ grams}}\) A1 N3
[6 marks]
(c) \(X \sim {\text{B(100, 0.002)}}\) (M1)
\({\text{P}}(X \leqslant 1) = 0.982…\) (A1)
\({\text{P}}(X \geqslant 2) = 1 – {\text{P}}(X \leqslant 1) = 0.0174\) A1
[3 marks]
Total [11 marks]
(a) Boys can be chosen in \(\frac{{6 \times 5}}{2} = 15\) ways (A1)
Girls can be chosen in \(\frac{{5 \times 4}}{2} = 10\) ways (A1)
Total \( = 15 \times 10 = 150\) ways A1
[3 marks]
(b) Number of ways \( = 5 \times 4 = 20\) (M1)A1
[2 marks]
(c) \(\frac{{20}}{{150}}{\text{ }}\left( { = \frac{2}{{15}}} \right)\) A1
[1 mark]
(d) METHOD 1
\({\text{P}}(T) = \frac{1}{5};{\text{ P}}(A) = \frac{2}{5}\) A1
P(T or A but not both) \( = {\text{P}}(T) \times {\text{P}}(A’) + {\text{P}}(T’) \times {\text{P}}(A)\) M1A1
\( = \frac{1}{5} \times \frac{3}{5} + \frac{4}{5} \times \frac{2}{5} = \frac{{11}}{{25}}\) A1
METHOD 2
Number of selections including Fred \( = 5 \times \left( {\begin{array}{*{20}{c}}
5 \\
2
\end{array}} \right) = 50\) A1
Number of selections including Tim but not Anna \( = \left( {\begin{array}{*{20}{c}}
4 \\
2
\end{array}} \right) = 6\) A1
Number of selections including Anna but not Tim \( = 4 \times 4 = 16\)
Note: Both statements are needed to award A1.
P(T or A but not both) \( = \frac{{6 + 16}}{{50}} = \frac{{11}}{{25}}\) M1A1
[4 marks]
Total [10 marks]
Examiners report
Part A was well done by many candidates although an arithmetic penalty was often awarded in (b)(i) for giving the new value of the mean to too many significant figures.
Candidates are known, however, to be generally uncomfortable with combinatorial mathematics and Part B caused problems for many candidates. Even some of those candidates who solved (a) and (b) correctly were then unable to deduce the answer to (c), sometimes going off on some long-winded solution which invariably gave the wrong answer. Very few correct solutions were seen to (d).
Question
Casualties arrive at an accident unit with a mean rate of one every 10 minutes. Assume that the number of arrivals can be modelled by a Poisson distribution.
(a) Find the probability that there are no arrivals in a given half hour period.
(b) A nurse works for a two hour period. Find the probability that there are fewer than ten casualties during this period.
(c) Six nurses work consecutive two hour periods between 8am and 8pm. Find the probability that no more than three nurses have to attend to less than ten casualties during their working period.
(d) Calculate the time interval during which there is a 95 % chance of there being at least two casualties.
▶️Answer/Explanation
Markscheme
Note: Accept exact answers in parts (a) to (c).
(a) number of patients in 30 minute period = X (A1)
\(X \sim {\text{Po(3)}}\) (M1)A1
[3 marks]
(b) number of patients in working period = Y (A1)
\(Y \sim {\text{Po(12)}}\) (M1)A1
[3 marks]
(c) number of working period with less than 10 patients = W (M1)(A1)
\(W \sim {\text{B}}(6,{\text{ }}0.2424 \ldots )\) (M1)A1
[4 marks]
(d) number of patients in t minute interval = X
\(X \sim {\text{Po}}(T)\)
\({\text{P}}(X \geqslant 2) = 0.95\)
\({\text{P}}(X = 0) + {\text{P}}(X = 1) = 0.05\) (M1)(A1)
\({{\text{e}}^{ – T}}(1 + T) = 0.05\) (M1)
\(T = 4.74\) (A1)
t = 47.4 minutes A1
[5 marks]
Total [15 marks]
Examiners report
Parts (a) and (b) were well answered, but many students were unable to recognise the Binomial distribution in part (c) and were unable to form the correct equation in part (d). There were many accuracy errors in this question.
