12.2 Nuclear physics
Essential Idea:
The idea of discreteness that we met in the atomic world continues to exist in the nuclear world as well
Understandings:
- Rutherford scattering and nuclear radius
- Nuclear energy levels
- The neutrino
- The law of radioactive decay and the decay constant
Applications and Skills:
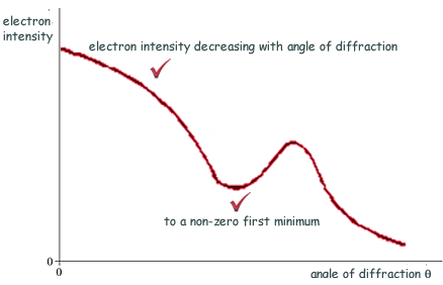
- Describing a scattering experiment including location of minimum intensity for the diffracted particles based on their de Broglie wavelength
- Explaining deviations from Rutherford scattering in high energy experiments
- Describing experimental evidence for nuclear energy levels
- Solving problems involving the radioactive decay law for arbitrary time intervals
- Explaining the methods for measuring short and long half-lives
Data booklet reference:
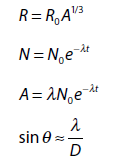
Nuclear Physics
Rutherford Scattering
Simple energy considerations can be used to calculate the distance of closest approach of an alpha-particle.
- If the alpha-particle initially has kinetic energy upon approaching, when it stops close to the nucleus due to the electrostatic repulsion, the electrical potential energy of the alpha particle will be K(2e)(Ze)/d = 2KZe²/d, where Z is the atom’s proton number.
- An alpha-particle approaching with high kinetic energy will get closer to the nuclear (closest = nuclear radius).
- \(\mathbf{R\ = \ }\mathbf{R}_{\mathbf{0}}\mathbf{A}^{\mathbf{1/3}}\) R is the radius, R0 is the Fermi radius ( \(1.2 \times 10^{- 15}\text{\ m}\)) and A is the mass number.
- All nuclei have the same density, and so their volume is given by
\(\mathbf{V\ = \ 4/3\ \pi}\mathbf{R}^{\mathbf{3}}\mathbf{\ = \ 4/3\ \pi A}{\mathbf{R}_{\mathbf{0}}}^{\mathbf{3}}.\)
- All nuclei have the same density, and so their volume is given by

- Derivations from Rutherford scattering: When the alpha-particles have very high kinetic energy, and thus, the distance of closest approach is equal or less than \(10^{- 15}m\), deviations are observed, which is an evidence of the existence of the strong nuclear force, i.e. they are absorbed.
- The derivation of the Rutherford formula is based on a number of assumptions. The most important is that the only force in play during the scattering process is the electric force. As the energy of the alpha particles increases, the alpha particles can get closer to the nucleus. When the distance of closest approach gets to be about 10−15 m or less, deviations from the Rutherford formula are observed . This is due to the fact that the alpha particles are so close to the nucleus that the strong nuclear force begins to act on the alpha particles.
Electron diffraction
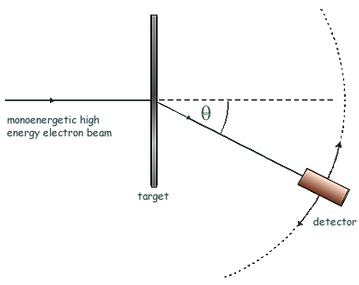
If the de Broglie wavelength λ** **of the electrons is about the same as the nuclear diameter D, then a minimum will be formed at sinθ = λD. **Useful **to measure nuclear radius.
- More accurate than Rutherford scattering, because strong force does not affect electrons.
- When electrons of much higher energy are used, the collisions are no longer elastic and energy is converted into mass, as several mesons are emitted from the nucleus
- At high energies,** **the electrons penetrate into the nucleus and scatter off the quarks within protons and neutrons, something known as deep inelastic scattering, providing evidence for the quark model.
Nuclear energy levels
The emission of alpha and beta particles by radioactive decay often leaves the **daughter nuclei in an excited discrete energy state **(similar to electron energy levels). The state depends on the energy of the alpha or beta particle.
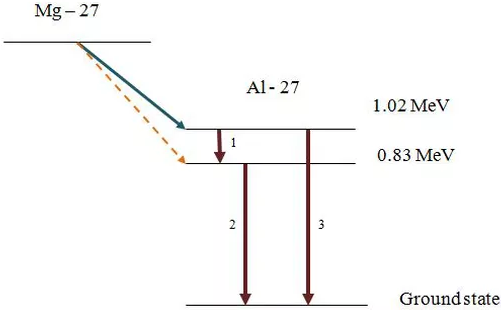
Alpha decay
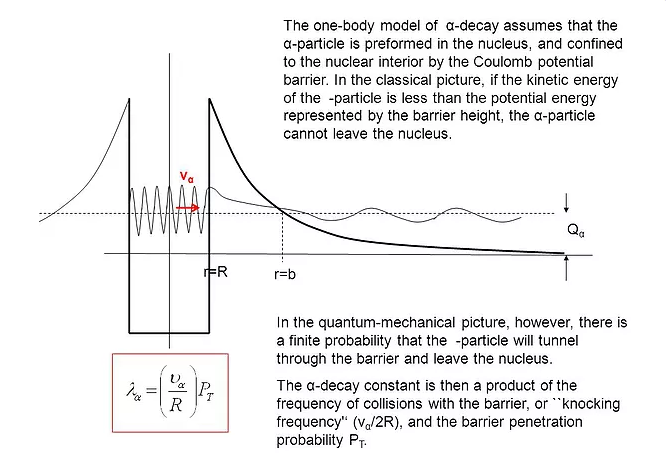
Alpha-particles form as clusters of two protons and two neutros inside the nucleus well before they are emitted as alpha-particles. This is because the nucleons are in random motion within the nucleus but their kinetic energies are much smaller than those needed to escape.
- The wave function │Ψ│ of the alpha-particles is not localized to the nucleus and allows overlaps with the potential energy barrier provided by the strong nuclear force, which means that there is a finite but very small probability of observing the alpha-particles outside the nucleus (thanks to quantum tunneling).
- Higher potential barriers and greater thickness to cross means a longer lifetime (e.g. polonium).
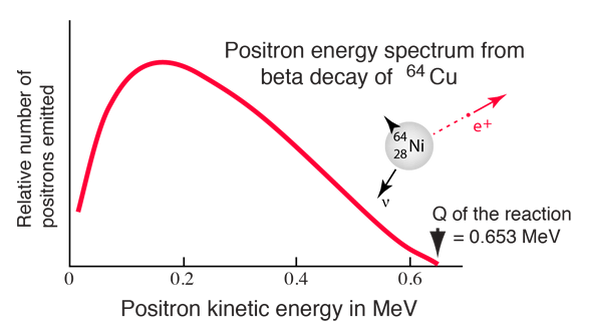
Negative beta decay
Since the beta particles have a continuous energy spectrum, in order to conserve mass, energy, and momentum, the existence of the neutrino was suggested by Pauli (and later anti-neutrino)
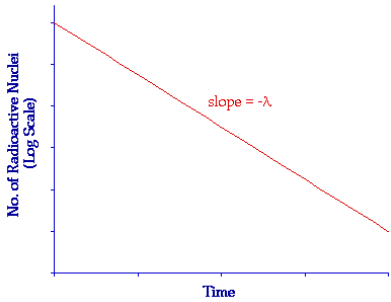
The law of radioactive decay
- Decay constant (λ): The probability that an individual nucleus will decay in a given time interval (e.g.1 s)
- Units:\(s^{- 1},\ min^{- 1},\ hour^{- 1},\ day^{- 1}\text{…\!}\)
- Relationship with half-life:
\(\mathbf{\lambda\ = \ ln(2)/}\mathbf{T}_{\mathbf{half – life}}\) - Activity (A): Number of nuclei decaying in a second in a sample
- Units: becquerel (Bq)
- In a sample of N undecayed nuclei, the activity will be given by n(A) = λN.
- Formulas:
\(\mathbf{N\ = \ }\mathbf{N}_{\mathbf{0}}\mathbf{e}^{\mathbf{- \lambda t}}\mathbf{;\ A\ = \ }\mathbf{A}_{\mathbf{0}}\mathbf{e}^{\mathbf{- \lambda t}}\mathbf{;\ A\ = \ \lambda}\mathbf{N}_{\mathbf{0}}\mathbf{e}^{\mathbf{- \lambda t}}\)where \(\mathbf{A}_{\mathbf{0}}\mathbf{\ = \ \lambda}\mathbf{N}_{\mathbf{0}}\) is the initial activity.
- **Measuring long half-lives: **Not possible to measure using a G-M tube. In these cases, a pure sample of the nuclide in a known chemical form needs to be separated, its mass measured and then a count rate taken. From this reading, the activity can be calculated by multiplying the count rate by the ratio
NUCLEI
SOME IMPORTANT FACTS ABOUT ATOMIC MASS, SIZE AND COMPOSITION OF NUCLEUS
- Proton was discovered by Goldstein
- Atomic mass unit
1.a.m.u = 1/12th of mass of C-12 isotope,
1 a.m.u = 1.660565 × 10–27 kg - Mass of a proton, mp = 1.0073 a.m.u = 1.6726 × 10–27 kg
- Chadwick’s experiment
Neutrons were detected.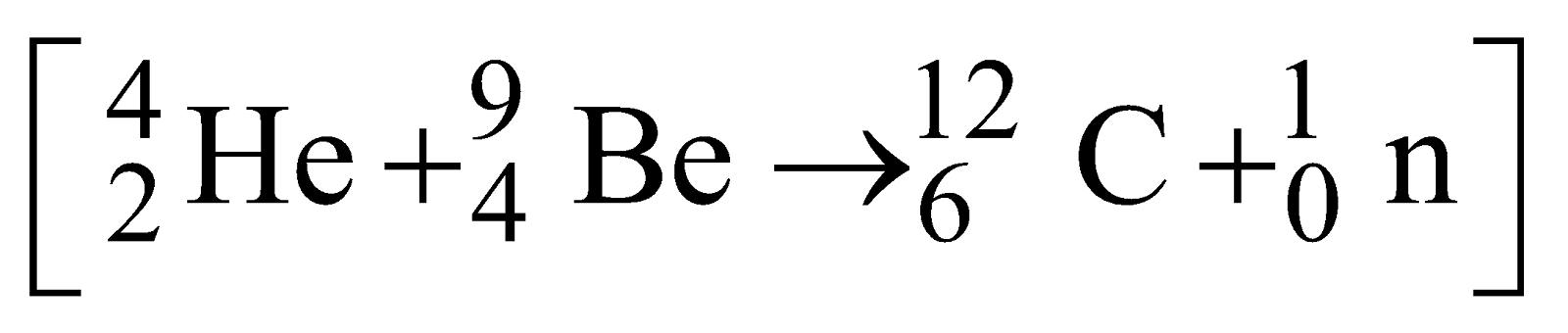
- Mass of neutron, mn = 1.00866 a.m.u = 1.6749 × 10–27 kg
- Mass of electron = 9.1 ×10–31 kg
- Mass number, A = total number of nucleons (neutrons + protons present in the nucleus of an atom)
- Atomic number, Z = number of protons = number of electrons
- Types of nuclei :
- Isotopes : The atoms of the element which have the same atomic number but different atomic mass numbers. e.g., 1H1, 1H2, 1H3 ; 8O16, 8O17, 8O18
- Isobars : The atoms of differents element which have the same atomic mass number but different atomic numbers. e.g., 6C14, 7N14, 18Ar40, 20Ca40 etc.
- Isotones : The nuclides which contain the same number of neutrons e.g., 2H23, 2He24, 2Be59, 5Be510 etc.
- Isomers : having same mass number, same atomic number but different radioactive properties.
- Rest mass of nucleus is less than sum of rest masses of constituent nucleons, the difference is called mass defect.
- Size of the nucleus : Radius of nucleus, R = R0 A1/ 3 where R0 = 1.1 × 10–15 m.
- Nuclear density of all elements ~ 1017 kg m–3.
MASS ENERGY AND NUCLEAR BINDING ENERGY
BINDING ENERGY
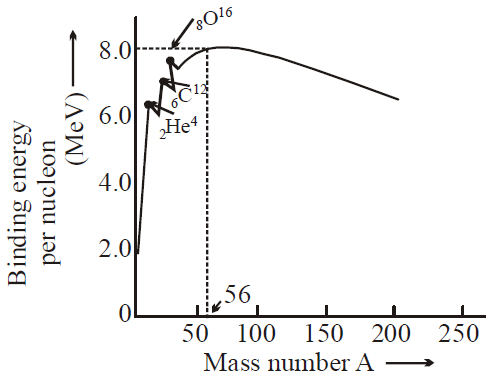
- A nuclide is a specific nucleus of an atom characterised as ZXA where A = mass number and Z = atomic number.
- Binding energy per nucleon is nearly 8.4 MeV for nuclei in the range of mass number 40 to 120.
- Binding energy is highest in Fe56.( 8.8 MeV)
- Binding energy curve predicts :
- Fission : Breaking up of a heavy nucleus (A > 200) into two nuclei of approximately equal size, and release of energy.
- Fusion : Lighter nuclei ( A < 20) combine together to form heavier nucleus and release of energy.
- BE/ A varies by less than 10% above A = 10 suggests that each nucleon interacts with its neighbouring nucleon only.
- For A > 56, BE/A decreases because of the destabilising effect of long-range coulombic force.
NUCLEAR FORCE
CHARACTERISTICS OF NUCLEAR FORCE
- It is a short range force effective only in range 10–15 m
- It is charge independent. It acts between proton-proton, proton-neutron and neutron – neutron.
- It is not a central force.
- It is spin dependent.
- It is 1038 times stronger than gravitational force and 102 times stronger than electric force.
- The main cause of nuclear force is the exchange of π− mesons between nucleus
RADIOACTIVITY
- α-rays (i.e., Helium nuclei or α – particles)
- β-rays (i.e., electron or positron or β – particles)
- γ-rays (photons or gamma radiations)
- chemical combination
- changing physical environment other than nuclear bombardment
FEATURES OF RADIOACTIVITY
- It is a statistical process.
- When a nucleus undergoes alpha or beta decay, its atomic number and mass number changes (in β-decay only atomic number changes) & it transforms into a new element.
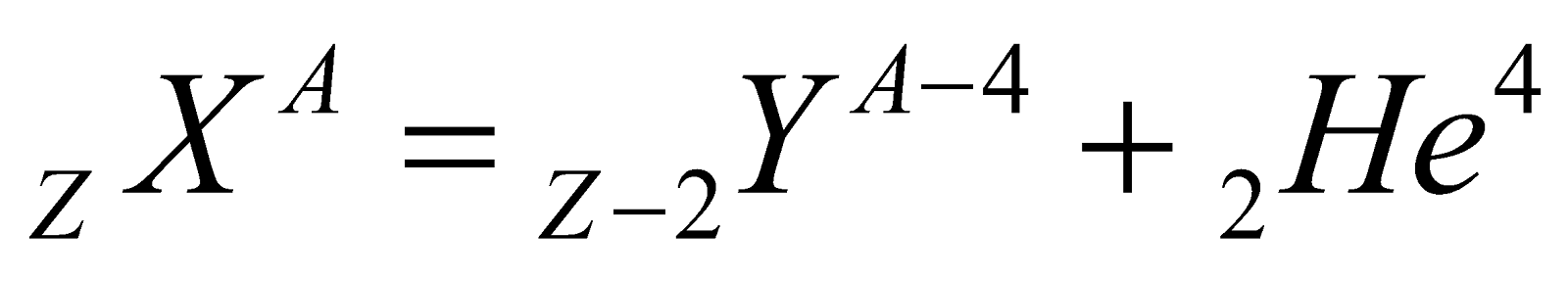 (α-particle), it means that by emission of alpha particle (α-particle), it loses 2 units of charge and 4 units of mass.
(α-particle), it means that by emission of alpha particle (α-particle), it loses 2 units of charge and 4 units of mass.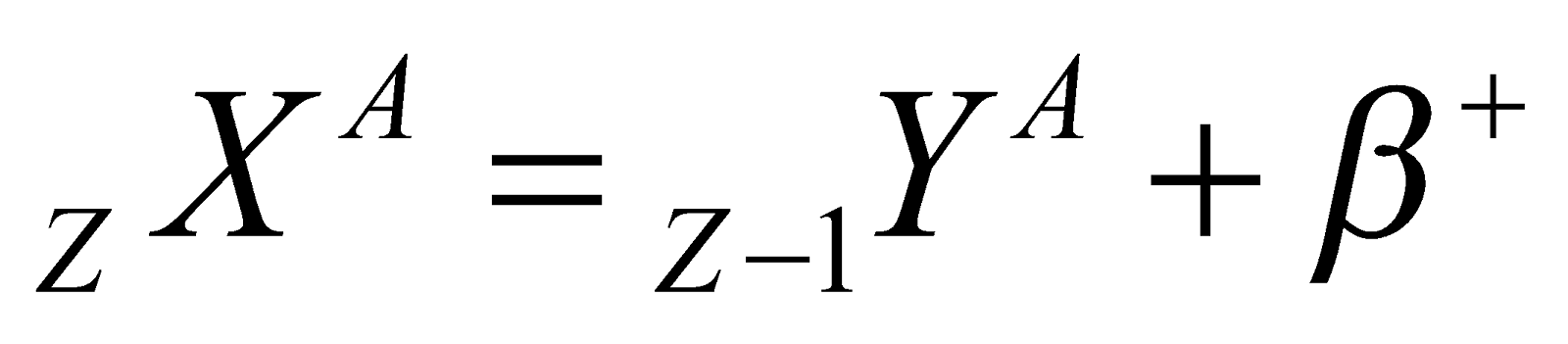 (positron). It means that by emission of beta particle (β+-particle), nucleus loses one unit of charge. It is surprising to note that a nucleus does not contain β+ then how is it emitted. Reason : During a β+ particle(i.e., positron) decay, a protron converts into a neutron
(positron). It means that by emission of beta particle (β+-particle), nucleus loses one unit of charge. It is surprising to note that a nucleus does not contain β+ then how is it emitted. Reason : During a β+ particle(i.e., positron) decay, a protron converts into a neutron
- When a nucleus emits a gamma ray, neither the mass nor the charge of the nucleus changes
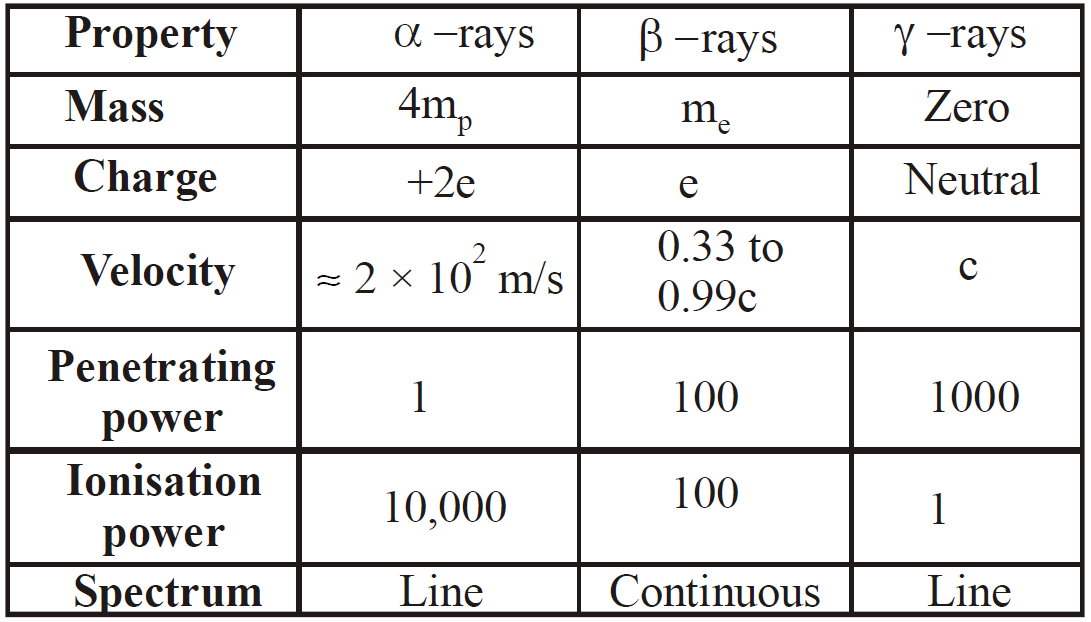
PROPERTIES OF α, β & γ-RAYS
- It is a positively charged particle & contains a charge of 3.2 × 10–19 coulomb (exactly double the charge of electron).
- The mass of α-particles is 6.645 × 10–27kg (It is equal to mass of a helium nucleus). Actually α-particle is nucleus of helium, hence it is called doubly ionised helium.
- They (α-particles) get deflected in both electric & magnetic fields.
- The velocity of α-particle is very less than the velocity of light i.e.,
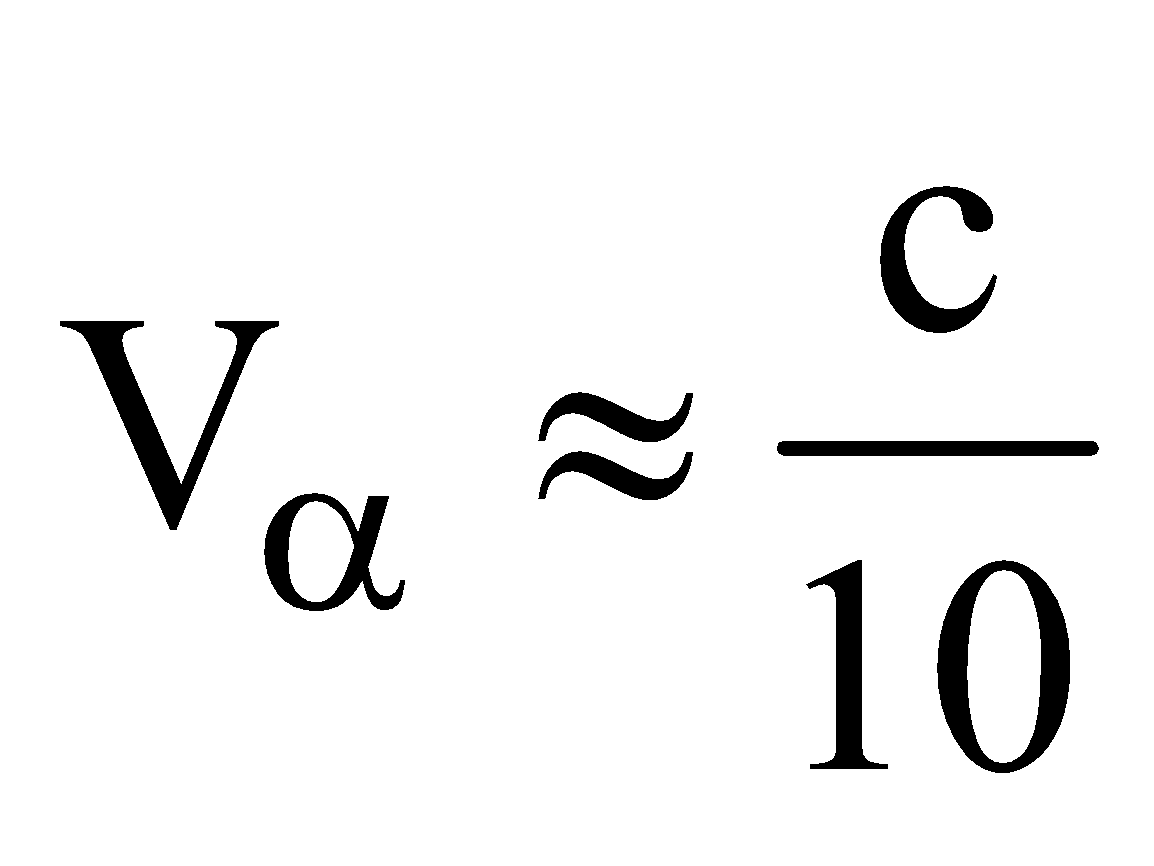 , where c is velocity of light.
, where c is velocity of light. - The range of α-particle in air depends on radioactive substance.
- The ionisation power of α-particle is higher than both β (100 times of β & 10,000 times of γ) and γ particle.
- The penetrating power of α particle is lowest (in comparison to β & γ particles). It is 1/100 times of β-particles & 1/10,000 times of γ-rays.
- The α-particles can produce fluorescence in barium platinocyanide and zinc sulphide.
- They show little effect on photographic plate.
- They show heating effect on stopping.
- The beta particles (i.e., β– or β+) may be positive & negative particle & contain
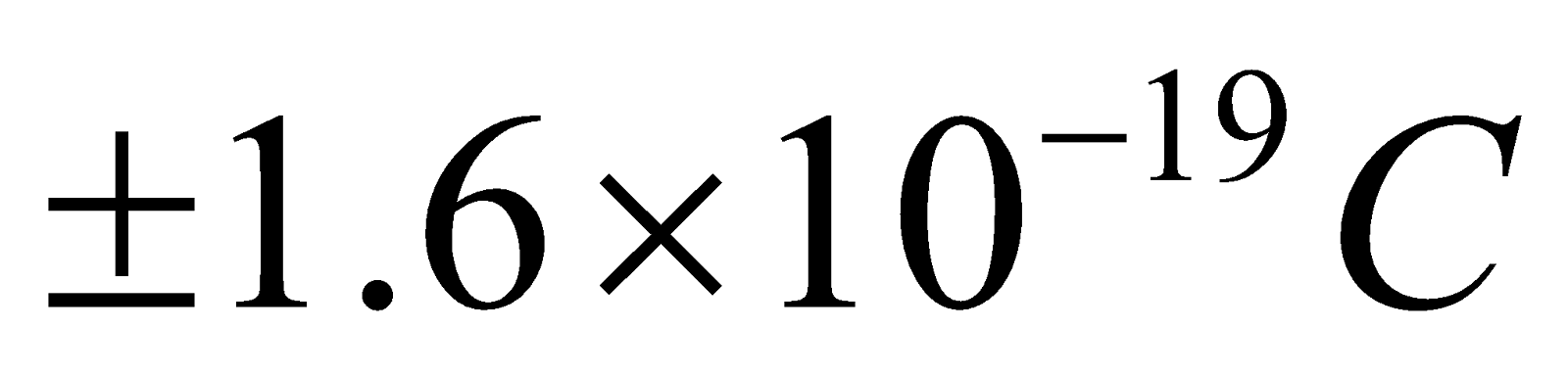 of charge. Actually β– is electron & β+ is positron.
of charge. Actually β– is electron & β+ is positron. - They get deflected in both electric & magnetic field.
- The velocity of β-particle varies between 0.01c to .99c, where c is velocity of light.
- The mass of β particle is relativistic, because its velocity is comparable to velocity of light
- They have both ionisation & penetration power. Ionisation power less than α-particle and penetration power more than α-particle.
- They produce fluorescence on barium platinocyanide & zinc sulphide.
- They are electromagnetic waves as x-rays.
- They are not deflected in electric & magnetic field, it means that they are chargeless.
- The velocity of γ-particle is equal to velocity of light.
- The ionisation power of gamma rays is less than β & α rays but penetration power more than β and α-rays.
- The γ-particles are emitted from the nucleus, while X-rays are obtained, when electron goes from one state to another in an atom.
- When γ-rays photon strikes nucleus in a substance, then it gives rise to a phenomenon of pair production i.e.,
RUTHERFORD AND SODDY LAW FOR RADIOACTIVE DECAY
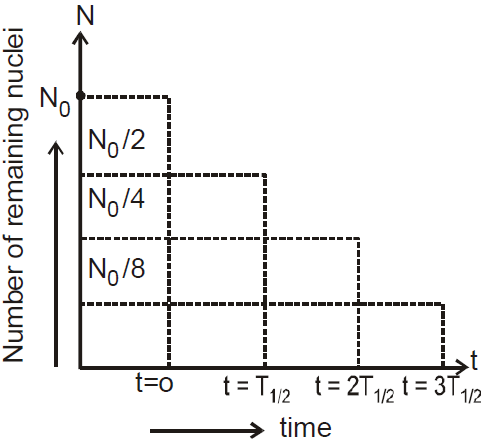
HALF-LIFE OF A RADIOACTIVE SUBSTANCE
MEAN LIFE OF A RADIOACTIVE SUBSTANCE
RADIOACTIVE SERIES
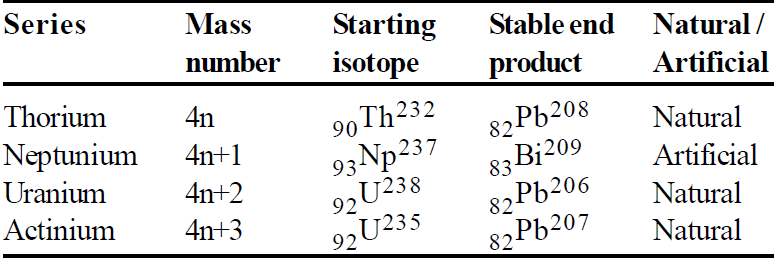
RADIOACTIVE EQUILIBRIUM
CARBON DATING
- Specific activity is the activity of 1 gram of material.
- Geiger Muller Counter is used for detecting α and β particles.
- Cloud chamber is used for detecting radioactive radiations and for determining their paths, range and energy.
- Baryon number
B = 1, for a neutron and a proton. - Lepton number (L)
L = 1 for electron, and neutrino
- Radioactive isotope
- Iodine-131 For detecting the activity of thyroid gland
- Chromium-51 To locate the exact position of haemorrhage
- Phosphorus-32 In agriculture
- C–14 Carbon dating, Photosynthesis in plants
- Co60 Cancer treatment
- Na24 For circulation of blood
NUCLEAR REACTION
- Charge conservation
- Conservation of linear momentum
- Conservation of angular momentum
- Conservation of energy (Rest mass energy + K.E.)
NUCLEAR FISSION (BY OTTO HAHN AND STRASSMANN)
- Nuclear fuel : U233, U235, Pu239 etc.
- Moderator : Graphite, heavy water (D2O). To slow down the neutrons (or slow down the nuclear reaction).
- Control rods : (Cadmium, boron). To absorb excess neutrons. It controls the chain reaction.
- Coolant : (water etc). To remove the heat produced in the core to heat exchanger for production of electricity.
NUCLEAR FUSION
The neutrino
In the 1930s it was thought that beta minus decay was described by
\(_{0}^{1}\textrm{n} \rightarrow _{1}^{1}\textrm{p}+ \;_{{-1}}^{0}\textrm{e}\)
The mass deference for this decay is:
1.008665 u – (1.007276 + 0.0005486) u = 0.00084 u
and corresponds to an energy of:
0.00084 × 931.5 MeV = 0.783 MeV
If only the electron and the proton are produced, then the electron, being the lighter of the two, will carry most of this energy away as kinetic energy.
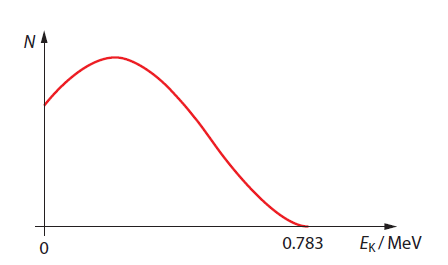
The number of electrons that carry a given energy as a function of energy.
In experiments, however, the electron has a range of energies from zero up to 0.783 MeV, If the electron is not carrying 0.783 MeV of energy, where is the missing energy?
Wolfgang Pauli hypothesised the existence of a third particle in the products of a beta decay in 1933. Since the energy of the electron in beta decay has a range of possible values, it means that a third very light particle must also be produced so that it carries the remainder of the available energy. Enrico Fermi coined the word neutrino for the ‘little neutral one.
Tunneling Through a Potential Barrier
A potential energy barrier is a region where a traveling particle will have an increased potential energy Ub.
The particle can pass through the barrier if its total energy \(E>U_b\).
Classically, it cannot pass through it if \(E<U_b\)., but in quantum physics it can, an effect called tunneling.
For a particle with mass m and a barrier of thickness L, the transmission coefficient is
\(T\approx e^{-2bL}\)
where
\(b=\sqrt{\frac{8\pi ^2 m(U_b-E)}{h^2}}\)
Can the electron pass through the region of negative potential?
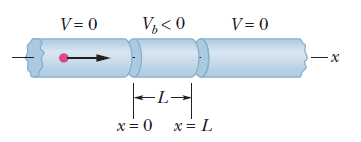
The elements of a narrow tube in which an electron (the dot) approaches a negative electric potential Vb in the region x= 0 to x= L.

An energy diagram containing two plots for the situation of Fig. above:
- The electron’s mechanical energy E is plotted when the electron is at any coordinate x < 0.
- The electron’s electric potential energy U is plotted as a function of the electron’s position x, assuming that the electron can reach any value of x.
- The nonzero part of the plot (the potential barrier) has height Ub and thickness L.

plot of the probability density \(|\Psi|^2\) of the electron matter wave for the situation of first Fig.The value of \(|\Psi|^2\) is nonzero to the right of the potential barrier.
