Question
The following curves are sketches of the graphs of the functions given below, but in a different order. Using your graphic display calculator, match the equations to the curves, writing your answers in the table below.
(the diagrams are not to scale)
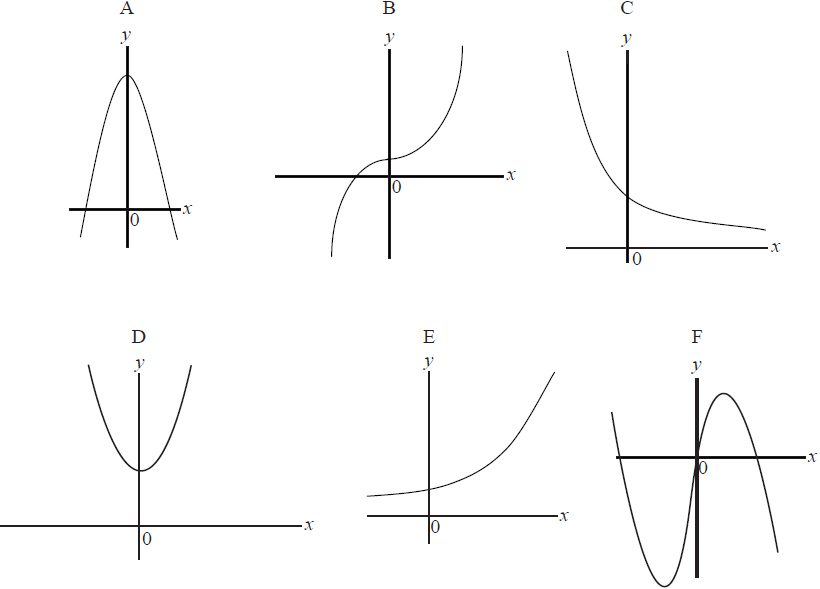
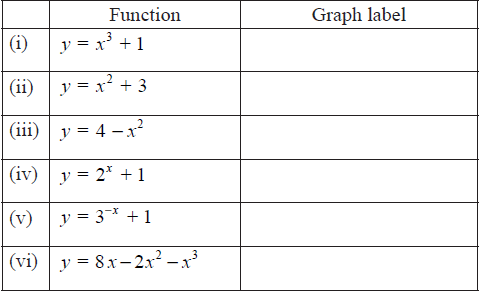
▶️Answer/Explanation
Markscheme
(i) B (A1)
(ii) D (A1)
(iii) A (A1)
(iv) E (A1)
(v) C (A1)
(vi) F (A1) (C6)[6 marks]
Question
The straight line, L, has equation \(2y – 27x – 9 = 0\).
a.Find the gradient of L.[2]
b.Sarah wishes to draw the tangent to \(f (x) = x^4\) parallel to L.
Write down \(f ′(x)\).[1]
c, i.Find the x coordinate of the point at which the tangent must be drawn.[2]
c, ii.Write down the value of \(f (x)\) at this point.[1]
▶️Answer/Explanation
Markscheme
y = 13.5x + 4.5 (M1)
Note: Award (M1) for 13.5x seen.
gradient = 13.5 (A1) (C2)[2 marks]
4x3 (A1) (C1)[1 mark]
4x3 = 13.5 (M1)
Note: Award (M1) for equating their answers to (a) and (b).
x = 1.5 (A1)(ft)[2 marks]
\(\frac{{81}}{{16}}\) (5.0625, 5.06) (A1)(ft) (C3)
Note: Award (A1)(ft) for substitution of their (c)(i) into x4 with working seen.[1 mark]
Question
a.Consider the curve \(y = 1 + \frac{1}{{2x}},\,\,x \ne 0.\)
For this curve, write down
i) the value of the \(x\)-intercept;
ii) the equation of the vertical asymptote.[3]
 [3]
[3]
▶️Answer/Explanation
Markscheme
i) \(\left( {x = } \right) – 0.5\,\,\left( { – \frac{1}{2}} \right)\) (A1)
ii) \(x = 0\) (A1)(A1) (C3)
Note: Award (A1) for “\(x = \)” and (A1) for “\(0\)” seen as part of an equation.
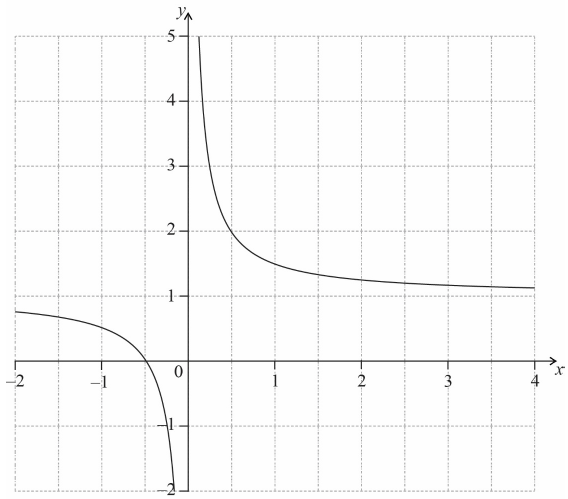
(A1)(ft)(A1)(ft)(A1) (C3)
Note: Award (A1)(ft) for correct \(x\)-intercept, (A1)(ft) for asymptotic behaviour at \(y\)-axis, (A1) for approximately correct shape (cannot intersect the horizontal asymptote of \(y = 1\)). Follow through from part (a).
Question
A function \(f\) is given by \(f(x) = 4{x^3} + \frac{3}{{{x^2}}} – 3,{\text{ }}x \ne 0\).
a.Write down the derivative of \(f\).[3]
b.Find the point on the graph of \(f\) at which the gradient of the tangent is equal to 6.[3]
▶️Answer/Explanation
Markscheme
\(12{x^2} – \frac{6}{{{x^3}}}\) or equivalent (A1)(A1)(A1) (C3)
Note: Award (A1) for \(12{x^2}\), (A1) for \( – 6\) and (A1) for \(\frac{1}{{{x^3}}}\) or \({x^{ – 3}}\). Award at most (A1)(A1)(A0) if additional terms seen.[3 marks]
\(12{x^2} – \frac{6}{{{x^3}}} = 6\) (M1)
Note: Award (M1) for equating their derivative to 6.
\((1,{\text{ }}4)\)\(\,\,\,\)OR\(\,\,\,\)\(x = 1,{\text{ }}y = 4\) (A1)(ft)(A1)(ft) (C3)
Note: A frequent wrong answer seen in scripts is \((1,{\text{ }}6)\) for this answer with correct working award (M1)(A0)(A1) and if there is no working award (C1).[3 marks]
