Question 15. [Maximum mark: 7]
The number of coffees sold per hour at an independent coffee shop is modelled by a Poisson distribution with a mean of 22 coffees per hour.
Sheila, the shop’s owner wants to increase the number of coffees sold in the shop. She decides to offer a discount to customers who buy more than one coffee.
To test how successful this strategy is, Sheila records the number of coffees sold over a single 5-hour period. Sheila decides to use a 5 % level of significance in her test.
a. State the null and alternative hypotheses for the test. [1]
b. Find the probability that Sheila will make a type I error in her test conclusion. [4] Sheila finds 126 coffees were sold during the 5-hour period.
c. State Sheila’s conclusion to the test. Justify your answer. [2]
▶️Answer/Explanation
(a) \(H_{0} : m = 110\) , \(H_{1} : m > 110\)
(b) \(P(X ≥ 128)=0.05024 \)
\(P(X ≥ 129)= 0.04153 \) (probability of making a type I error is) 0.0415
(c) \(X ~ Po (110)\)
\(P(X≥ 126) = 0.072 > 0.05\) OR recognizing 126 < 129 or ≤ 128 so there is insufficient evidence to reject \(H_{0}\) (ie there is insufficient evidence to suggest that the number of coffees being sold has increased)
Question 3 [Maximum mark: 6]
At Springfield University, the weights, in kg, of 10 chinchilla rabbits and 10 sable rabbits were recorded. The aim was to find out whether chinchilla rabbits are generally heavier than sable rabbits. The results obtained are summarized in the following table.
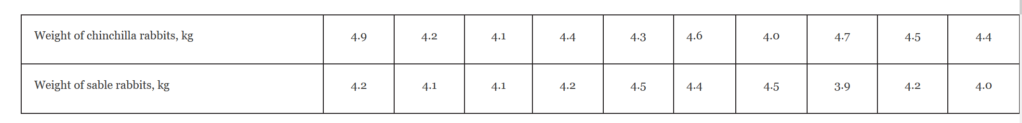
A t-test is to be performed at the 5 % significance level.
a. Write down the null and alternative hypotheses. [2]
b. Find the p-value for this test. [2]
c. Write down the conclusion to the test. Give a reason for your answer. [2]
▶️Answer/Explanation
(a) (let μc = population mean for chinchilla rabbits, μs = population mean for sable rabbits) Ho : μc= μs = H1 : μc > μs
(b) \(p- value = 0.0408 (0.0408065…)\)
(c) \(0.0408 < 0.05\) (there is sufficient evidence to) reject (or not accept) \(H_{0}\) (there is sufficient evidence to suggest that chinchilla rabbits are (generally) heavier than sable rabbits)
11. [Maximum mark: 7]
A factory, producing plastic gifts for a fast food restaurant’s Jolly meals, claims that just 1 % of the toys produced are faulty.
A restaurant manager wants to test this claim. A box of 200 toys is delivered to the restaurant. The manager checks all the toys in this box and four toys are found to be faulty.
(a) Identify the type of sampling used by the restaurant manager. [1] The restaurant manager performs a one-tailed hypothesis test, at the 10 % significance level,
to determine whether the factory’s claim is reasonable. It is known that faults in the toys
occur independently.
(b)Write down the null and alternative hypotheses. [2]
(c)Find the p-value for the test. [2]
(d) State the conclusion of the test. Give a reason for your answer. [2]
▶️Answer/Explanation
(a) Convenience (b) H0 : 1% of the toys produced are faulty H1 : More than 1% are faulty (c) X ∼ B(200, 0.01) P (X ≥ 4) = 0.142 Note: Any attempt using Normal approximation to find p-value is awarded M0A0. (d) 14% > 10% so there is insufficient evidence to reject H0 .
Question
A psychologist records the number of digits ( d ) of π that a sample of IB Mathematics higher
level candidates could recall.
| d | 2 | 3 | 4 | 5 | 6 | 7 |
| Frequency | 2 | 6 | 24 | 21 | 11 | 3 |
(a) Find an unbiased estimate of the population mean of d.
(b) Find an unbiased estimate of the pThe psychologist has read that in the general population people can remember an average
of 4.4 digits of π. The psychologist wants to perform a statistical test to see if IB Mathematics
higher level candidates can remember more digits than the general population.opulation variance of d.
(c) \(H_0 : \mu = 4.4\) is the null hypothesis for this test.
(i) State the alternative hypothesis.
(ii) Given that all assumptions for this test are satisfied, carry out an appropriate hypothesis test. State and justify your conclusion. Use a 5% significance level.
▶️Answer/Explanation
Ans:
(a) \(\overrighthat{x} = 4.63 (4.62686…)\)
(b) \(S_{n-1} = 1.098702\)
\(S_{n-1}=1.21 (1.207146…)\)
(c) (i) \(H_1 : \mu > 4.4\)
(ii) METHOD 1
using a z-test
p = 0.0454992…
p<0.05
reject null hypothesis
(therefore there is significant evidence that the IB HL math students know more digits
of π than the population in general)
METHOD 2
using a t-test
p = 0.0478584…
p<0.05
(therefore there is significant evidence that the IB HL math students know more digits
of π than the population in general)
Question
The number of cars arriving at a junction in a particular town in any given minute between
9:00 am and 10:00 am is historically known to follow a Poisson distribution with a mean of
5.4 cars per minute.
A new road is built near the town. It is claimed that the new road has decreased the number
of cars arriving at the junction.
To test the claim, the number of cars, X, arriving at the junction between 9:00 am and
10:00 am on a particular day will be recorded. The test will have the following hypotheses:
\(H_0\) : the mean number of cars arriving at the junction has not changed,
\(H_1\) : the mean number of cars arriving at the junction has decreased.
The alternative hypothesis will be accepted if X ≤ 300.
(a) Assuming the null hypothesis to be true, state the distribution of X.
(b) Find the probability of a Type I error.
(c) Find the probability of a Type II error, if the number of cars now follows a Poisson
distribution with a mean of 4.5 cars per minute.
▶️Answer/Explanation
Ans:
(a) \(X \sim Po(324)\)
(b) \(P(X \leq 300)\)
\(=0.0946831… \approx 0.0947\)
(c) (mean number of cars =) \(4.5 \times 60 = 270\)
\(P(X > 300| \lambda = 270)\)
\(P(X \geq 301)\) OR \(1-P(X \leq 300)\)
\(=0.0334207… \approx 0.0334\)
