Question
Chloroethene, C2H3Cl, is an important organic compound used to manufacture the polymer poly(chloroethene).
State an equation for the reaction of ethanoic acid with water.[1]
Calculate the pH of \({\text{0.200 mol}}\,{\text{d}}{{\text{m}}^{ – 3}}\) ethanoic acid \(({\text{p}}{K_{\text{a}}} = 4.76)\).[3]
Determine the pH of a solution formed from adding \({\text{50.0 c}}{{\text{m}}^{\text{3}}}\) of \({\text{1.00 mol}}\,{\text{d}}{{\text{m}}^{ – 3}}\) ethanoic acid, \({\text{C}}{{\text{H}}_{\text{3}}}{\text{COOH(aq)}}\), to \({\text{50.0 c}}{{\text{m}}^{\text{3}}}\) of \({\text{0.600 mol}}\,{\text{d}}{{\text{m}}^{ – 3}}\) sodium hydroxide, NaOH(aq).[4]
(if acid added) \({\text{C}}{{\text{H}}_3}{\text{CO}}{{\text{O}}^ – } + {{\text{H}}^ + } \to {\text{C}}{{\text{H}}_3}{\text{COOH}}\);
(if alkali added) \({\text{C}}{{\text{H}}_3}{\text{COOH}} + {\text{O}}{{\text{H}}^ – } \to {\text{C}}{{\text{H}}_3}{\text{CO}}{{\text{O}}^ – } + {{\text{H}}_2}{\text{O}}\);
Explanation marks cannot be awarded without equations.[2]
Answer/Explanation
Markscheme
\({\text{C}}{{\text{H}}_3}{\text{COOH(aq)}} + {{\text{H}}_2}{\text{O(l)}} \rightleftharpoons {\text{C}}{{\text{H}}_3}{\text{CO}}{{\text{O}}^ – }{\text{(aq)}} + {{\text{H}}_3}{{\text{O}}^ + }{\text{(aq)}}\);
OR
\({\text{C}}{{\text{H}}_3}{\text{COOH(l)}} + {{\text{H}}_2}{\text{O(l)}} \rightleftharpoons {\text{C}}{{\text{H}}_3}{\text{CO}}{{\text{O}}^ – }{\text{(aq)}} + {{\text{H}}_3}{{\text{O}}^ + }{\text{(aq)}}\);
OR
\({\text{C}}{{\text{H}}_3}{\text{COOH(aq)}} \rightleftharpoons {\text{C}}{{\text{H}}_3}{\text{CO}}{{\text{O}}^ – }{\text{(aq)}} + {{\text{H}}^ + }{\text{(aq)}}\);
Must include \( \rightleftharpoons \).
Ignore state symbols.
(ii) \({K_{\text{a}}} = {10^{ – 4.76}}/1.74 \times {10^{ – 5}}/{\text{pH}} = {\text{p}}{K_{\text{a}}} + \log \frac{{{\text{[SALT]}}}}{{{\text{[ACID]}}}}\);
\(1.74 \times {10^{ – 5}} = \frac{{{{{\text{[}}{{\text{H}}^ + }{\text{]}}}^2}}}{{{\text{0.200}}}}/{\text{[}}{{\text{H}}^ + }{\text{]}} = 0.00187\);
\({\text{pH}} = 2.73\);
Award [3] for correct final answer, allow mark for correct conversion of [H+] to pH even if [H+] incorrect.
(initial) \({\text{[C}}{{\text{H}}_{\text{3}}}{\text{COOH]}} = 0.500{\text{ mol}}\,{\text{d}}{{\text{m}}^{ – 3}}\) and) eqm \({\text{[C}}{{\text{H}}_{\text{3}}}{\text{COOH]}} = 0.200{\text{ mol}}\,{\text{d}}{{\text{m}}^{ – 3}}\);
(initial) \({\text{[C}}{{\text{H}}_{\text{3}}}{\text{CO}}{{\text{O}}^ – }{\text{]}} = 0.300{\text{ mol}}\,{\text{d}}{{\text{m}}^{ – 3}}\) and) eqm \({\text{[C}}{{\text{H}}_{\text{3}}}{\text{CO}}{{\text{O}}^ – }{\text{]}} = 0.300{\text{ mol}}\,{\text{d}}{{\text{m}}^{ – 3}}\);
Allow 0.02 moles and 0.03 moles instead of 0.200 and 0.300.
\({\text{[}}{{\text{H}}^ + }{\text{]}} = {K_{\text{a}}}\frac{{{\text{[C}}{{\text{H}}_3}{\text{COOH]}}}}{{{\text{[C}}{{\text{H}}_3}{\text{CO}}{{\text{O}}^ – }{\text{]}}}} = 1.16 \times {10^{ – 5}}{\text{ mol}}\,{\text{d}}{{\text{m}}^{ – 3}}\);
\({\text{pH}} = 4.94\);
Award [3 max] for correct final answer if no working shown.
[N/A]
Examiners report
The only issue was that some candidates forgot the reversible arrow in the equation.
A pleasing number were able to complete the pH calculation successfully.
Only the best candidates scored full marks for the buffer calculation; in some cases an incorrect expression was used, but more often there was no attempt to calculate the equilibrium amounts or concentrations.
There were very few who could write appropriate equations for the buffer action, even though it clearly stated that the answer should include equations many explained buffer action without any equations and scored no marks as a result.
Question
Water is an important substance that is abundant on the Earth’s surface.
Buffer solutions resist small changes in pH. A phosphate buffer can be made by dissolving \({\text{Na}}{{\text{H}}_{\text{2}}}{\text{P}}{{\text{O}}_{\text{4}}}\) and \({\text{N}}{{\text{a}}_{\text{2}}}{\text{HP}}{{\text{O}}_{\text{4}}}\) in water, in which \({\text{Na}}{{\text{H}}_{\text{2}}}{\text{P}}{{\text{O}}_{\text{4}}}\) produces the acidic ion and \({\text{N}}{{\text{a}}_{\text{2}}}{\text{HP}}{{\text{O}}_{\text{4}}}\) produces the conjugate base ion.
A \({\text{0.10 mol}}\,{\text{d}}{{\text{m}}^{ – 3}}\) ammonia solution is placed in a flask and titrated with a \({\text{0.10 mol}}\,{\text{d}}{{\text{m}}^{ – 3}}\) hydrochloric acid solution.
(i) State the expression for the ionic product constant of water, \({K_{\text{w}}}\).
(ii) Explain why even a very acidic aqueous solution still has some \({\text{O}}{{\text{H}}^ – }\) ions present in it.
(iii) State and explain the effect of increasing temperature on the value of \({K_{\text{w}}}\) given that the ionization of water is an endothermic process.
(iv) State and explain the effect of increasing temperature on the pH of water.[7]
(i) Deduce the acid and conjugate base ions that make up the phosphate buffer and state the ionic equation that represents the phosphate buffer.
(ii) Describe how the phosphate buffer minimizes the effect of the addition of a
strong base, \({\text{O}}{{\text{H}}^ – }{\text{(aq)}}\), to the buffer. Illustrate your answer with an ionic equation.
(iii) Describe how the phosphate buffer minimizes the effect of the addition of a
strong acid, \({{\text{H}}^ + }{\text{(aq)}}\), to the buffer. Illustrate your answer with an ionic equation.[7]
(i) Explain why the pH of the ammonia solution is less than 13.
(ii) Estimate the pH at the equivalence point for the titration of hydrochloric acid with ammonia and explain your reasoning.
(iii) State the equation for the reaction of ammonia with water and write the \({K_{\text{b}}}\) expression for \({\text{N}}{{\text{H}}_{\text{3}}}{\text{(aq)}}\).
(iv) When half the ammonia has been neutralized (the half-equivalence point), the pH of the solution is 9.25. Deduce the relationship between \({\text{[N}}{{\text{H}}_{\text{3}}}{\text{]}}\) and \({\text{[NH}}_4^ + {\text{]}}\) at the
half-equivalence point.
(v) Determine \({\text{p}}{K_{\text{b}}}\) and \({K_{\text{b}}}\) for ammonia based on the pH at the half-equivalence point.
(vi) Describe the significance of the half-equivalence point in terms of its effectiveness as a buffer.[11]
Answer/Explanation
Markscheme
(i) \({\text{(}}{K_{\text{w}}}{\text{)}} = {\text{[}}{{\text{H}}^ + }{\text{][O}}{{\text{H}}^ – }{\text{] / (}}{K_{\text{w}}}{\text{)}} = {\text{[}}{{\text{H}}_{\text{3}}}{{\text{O}}^ + }{\text{][O}}{{\text{H}}^ – }{\text{]}}\);
Do not award mark if [ ] omitted or other brackets are used.
(ii) \({\text{[}}{{\text{H}}^ + }{\text{]}}\) increases, \({\text{[O}}{{\text{H}}^ – }{\text{]}}\) decreases but still some present (\({K_{\text{w}}}\) constant) / \({\text{[O}}{{\text{H}}^ – }{\text{]}}\) cannot go to zero as equilibrium present / \({\text{[O}}{{\text{H}}^ – }{\text{]}} = \frac{{{K_{\text{w}}}}}{{{\text{[}}{{\text{H}}^ + }{\text{]}}}}\), thus \({\text{[O}}{{\text{H}}^ – }{\text{]}}\) cannot be zero / OWTTE;
Accept equilibrium present.
(iii) (changing T disturbs equilibrium) forward reaction favoured / equilibrium shifts to the right;
to use up (some of the) heat supplied;
\({{K_{\text{w}}}}\) increases (as both \({{\text{[}}{{\text{H}}^ + }{\text{]}}}\) and \({\text{[O}}{{\text{H}}^ – }{\text{]}}\) increase);
(iv) (as \({{\text{[}}{{\text{H}}^ + }{\text{]}}}\) increases) pH decreases / \({\text{pH}} < 7\);
No mark for more acidic.
inverse relationship between pH and \({\text{[}}{{\text{H}}^ + }{\text{] / pH}} = – \log {\text{[}}{{\text{H}}^ + }{\text{] / pH}} = {\log _{10}}\frac{{\text{1}}}{{{\text{[}}{{\text{H}}^ + }{\text{]}}}}\);
Accept [H3O+] in place of [H+].
(i) Acid: \({{\text{H}}_2}{\text{PO}}_4^ – \);
(Conjugate) base: \({\text{HPO}}_4^{2 – }\);
No mark for NaH2PO4 or Na2HPO4.
\({{\text{H}}_2}{\text{PO}}_4^ – {\text{(aq)}} \rightleftharpoons {{\text{H}}^ + }{\text{(aq)}} + {\text{HPO}}_4^{2 – }{\text{(aq)}}\);
Accept reverse equation or reaction with water.
Ignore state symbols, but equilibrium sign is required.
Accept OH– (ions) react with H+ (ions) to form H2O.
(ii) strong base/\({\text{O}}{{\text{H}}^ – }\) replaced by weak base (\({\text{HPO}}_4^{2 – }\), and effect minimized) / strong base reacts with acid of buffer / equilibrium in (i) shifts in forward direction;
Accept OH– added reacts with H+ to form H2O.
\({\text{O}}{{\text{H}}^ – }{\text{(aq)}} + {{\text{H}}_2}{\text{PO}}_4^ – {\text{(aq)}} \to {{\text{H}}_2}{\text{O(l)}} + {\text{HPO}}_4^{2 – }{\text{(aq)}}\);
Ignore state symbols, accept equilibrium sign.
(iii) strong acid/\({{\text{H}}^ + }\) replaced by weak acid (\({{\text{H}}_2}{\text{PO}}_4^ – \), and effect minimized) / strong acid reacts with base of buffer / equilibrium in (i) shifts in reverse direction;
\({{\text{H}}^ + }{\text{(aq)}} + {\text{HPO}}_4^{2 – }{\text{(aq)}} \to {{\text{H}}_2}{\text{PO}}_4^ – {\text{(aq)}}\);
Accept reaction with H3O+.
Ignore state symbols.
(i) \({\text{N}}{{\text{H}}_3}\) weak(er) base/partial dissociation;
\({\text{[O}}{{\text{H}}^ – }{\text{]}} < {\text{0.1(0)}}/{\text{pOH}} > 1{\text{ (thus pH}} < 13/{\text{pH}} + {\text{pOH}} = 14{\text{)}}\);
(ii) around \({\text{pH}} = 5\);
Accept a value between 4 and 6.
strong acid–weak base titration, (thus acidic) / at equivalence point, \({\text{NH}}_4^ + \) present is acidic / \({\text{NH}}_4^ + \rightleftharpoons {\text{N}}{{\text{H}}_3} + {{\text{H}}^ + }\);
(iii) \({\text{N}}{{\text{H}}_3}{\text{(aq)}} + {{\text{H}}_2}{\text{O(l)}} \rightleftharpoons {\text{NH}}_4^ + {\text{(aq)}} + {\text{O}}{{\text{H}}^ – }{\text{(aq)}}\);
Ignore state symbols, but equilibrium sign required.
\({K_{\text{b}}} = \frac{{{\text{[NH}}_4^ + {\text{][O}}{{\text{H}}^ – }{\text{]}}}}{{{\text{[N}}{{\text{H}}_3}{\text{]}}}}\);
(iv) \({\text{[N}}{{\text{H}}_3}{\text{]}} = {\text{[NH}}_4^ + {\text{]}}\);
(v) \({\text{pOH}} = 14.00 – 9.25 = 4.75\);
\({\text{p}}{K_{\text{b}}}{\text{ (}} = {\text{pOH)}} = 4.75\);
\({K_{\text{b}}} = 1.78 \times {10^{ – 5}}\);
Ignore units.
Award [3] for correct final answer.
(vi) optimum/most effective/highest buffer capacity/50%–50% buffer/equally effective as an acidic buffer and a basic buffer / OWTTE;
Examiners report
This was the second least commonly answered question. With the exception of the part on buffer chemistry where very few appreciated what was happening, the question was reasonably well done.
While many candidates gave the correct \({K_{\text{w}}}\) expression, it was not uncommon to either find the value of the constant or \({K_{\text{w}}} = {K_{\text{a}}} \times {K_{\text{b}}}\) given as the answers. A few included \({\text{[}}{{\text{H}}_{\text{2}}}{\text{O]}}\) in the expression. Candidates recognised that increasing the temperature shifts the equilibrium to the right, but most did not explain why, namely to use up some of the heat supplied.
Candidates generally concluded that formation of more \({{\text{H}}^ + }\) and \({\text{O}}{{\text{H}}^ – }\) ions gives a higher value of \({K_{\text{w}}}\). A significant number of candidates were able to state the effect of increasing temperature on the pH of water (it decreases) but failed to explain why. Some simply incorrectly stated that the pH would not change.
Many candidates gave the wrong formulas for the acid and the conjugate base ions of the buffer or offered \({\text{Na}}{{\text{H}}_{\text{2}}}{\text{P}}{{\text{O}}_{\text{4}}}\) and \({\text{N}}{{\text{a}}_{\text{2}}}{\text{HP}}{{\text{O}}_{\text{4}}}\) as the answers. Some candidates gave good answers about the effect of adding a small amount of a strong acid or a strong base, but they could not write correct equations to show these two effects.
Nearly all candidates correctly said that the ammonia solution is a weak base because of partial dissociation and \({\text{[O}}{{\text{H}}^ – }{\text{]}}\) would be less than 0.1 to give a pH less than 13. The majority of candidates correctly identified the pH around 4 – 6 because it is a titration between a strong acid and a weak base. When writing the equation for the reaction of ammonia and water some candidates did not write the equilibrium sign. The \({K_{\text{b}}}\) expression was correct in most cases. However, many did not recognise that at the half-equivalence point both the base and the conjugate acid concentrations are equal. The \({\text{p}}{K_{\text{b}}}\) and \({K_{\text{b}}}\) were correctly calculated from the pH of the solution by many candidates. However, most failed to realize that at the half-equivalence point the capacity of the buffer is optimum.
Question
Buffer solutions are widely used in both chemical and biochemical systems.
Describe the composition of an acidic buffer solution.
Determine the pH of a buffer solution, correct to two decimal places, showing your working, consisting of 10.0 g of \({\text{C}}{{\text{H}}_{\text{3}}}{\text{COOH}}\) and 10.0 g of CH\(_3\)COONa in \({\text{0.250 d}}{{\text{m}}^{\text{3}}}\) of solution. \({K_{\text{a}}}\) for \({\text{C}}{{\text{H}}_{\text{3}}}{\text{COOH}} = 1.8 \times {10^{ – 5}}\) at 298 K.
Answer/Explanation
Markscheme
(solution containing significant/equal amounts of a) weak acid and its salt / (solution containing) strong base to which excess of weak acid has been added / OWTTE;
Accept (solution containing) weak acid and conjugate base.
Do not accept descriptions with specific compounds alone (e.g. CH3COOH and CH3COONa) unless compounds are stated as weak acid and its salt.
Accept answer such as (solution containing) x mol of weak acid and \(\frac{1}{2}x\,mol\) of strong base.
\({M_{\text{r}}}{\text{(C}}{{\text{H}}_{\text{3}}}{\text{COOH)}} = 60.06\) and \({M_{\text{r}}}{\text{ C}}{{\text{H}}_3}{\text{COONa}} = 82.04\);
\({\text{[C}}{{\text{H}}_3}{\text{COOH]}} = 6.66 \times {10^{ – 1}}/0.666{\text{ mol}}\,{\text{d}}{{\text{m}}^{ – 3}}\)
\({\text{[C}}{{\text{H}}_3}{\text{CO}}{{\text{O}}^ – }{\text{]}} = 4.88 \times {10^{ – 1}}/{\text{ }}0.488{\text{ mol}}\,{\text{d}}{{\text{m}}^{ – 3}}\);
\({\text{[}}{{\text{H}}_3}{{\text{O}}^ + }{\text{]}}/{\text{[}}{{\text{H}}^ + }{\text{]}} = (1.8 \times {10^{ – 5}} \times 6.66 \times {10^{ – 1}})/4.88 \times {10^{ – 1}} = 2.46 \times {10^{ – 5}}/0.0000246{\text{ mol}}\,{\text{d}}{{\text{m}}^{ – 3}}\);
\({\text{pH}} = \left( { – {\text{log[}}{{\text{H}}_3}{{\text{O}}^ + }{\text{]}} = – {\text{log(2.46}} \times {\text{1}}{{\text{0}}^{ – 5}}{\text{)}} = } \right){\text{ 4.61 (2dp)}}\);
Award [5] for correct final answer of pH = 4.61 with some working shown.
Award [2 max] for pH = 4.61 without any working at all shown.
Two decimal places are required for M5.
OR
\({M_{\text{r}}}{\text{(C}}{{\text{H}}_{\text{3}}}{\text{COOH)}} = 60.06\) and \({M_{\text{r}}}{\text{ C}}{{\text{H}}_3}{\text{COONa}} = 82.04\);
\({\text{[C}}{{\text{H}}_3}{\text{COOH]}} = 6.66 \times {10^{ – 1}}/0.666{\text{ mol}}\,{\text{d}}{{\text{m}}^{ – 3}}\)
\({\text{[C}}{{\text{H}}_3}{\text{CO}}{{\text{O}}^ – }{\text{]}} = 4.88 \times {10^{ – 1}}/{\text{ }}0.488{\text{ mol}}\,{\text{d}}{{\text{m}}^{ – 3}}\);
\({\text{pH}} = – {\text{log(1.8}} \times {\text{1}}{{\text{0}}^{ – 5}}{\text{)}} + {\text{log}}\frac{{{\text{[salt]}}}}{{{\text{[acid]}}}}\);
\( = \left( {4.74 + \log \frac{{0.488}}{{0.666}} = 4.74 – 0.135 = } \right){\text{ }}4.61{\text{ (2dp)}}\);
M4 can be scored even if not explicitly stated if M5 is correct based on previous values.
Award [5] for correct final answer of pH = 4.61 with some working shown.
Award [2 max] for pH = 4.61 without any working at all shown.
Two decimal places are required for M5.
Examiners report
This question was based on buffer solutions and was found to be quite challenging for candidates. In part (a), some candidates again failed to read the question, which asked for a description of an acidic buffer solution. Many did not state explicitly that a weak acid is involved (acid alone was not sufficient).
In part (b), only the best candidates scored all five marks. In addition to conceptual errors, there were also a number of transcription errors (molar mass and arithmetic errors). Candidates also were required to express their answer to two decimal places. A number of candidates used the Henderson-Hasselbalch equation, but often an incorrect equation was given.
Question
An equilibrium exists between nitrosyl chloride, NOCl, nitrogen oxide, NO, and chlorine, \({\text{C}}{{\text{l}}_{\text{2}}}\).
\[{\text{2NOCl(g)}} \rightleftharpoons {\text{2NO(g)}} + {\text{C}}{{\text{l}}_2}{\text{(g)}}\]
\({\text{20.0 c}}{{\text{m}}^{\text{3}}}\) of hexane, \({{\text{C}}_{\text{6}}}{{\text{H}}_{{\text{14}}}}\), and \({\text{20.0 c}}{{\text{m}}^{\text{3}}}\) of pentan-1-ol, \({{\text{C}}_{\text{5}}}{{\text{H}}_{{\text{11}}}}{\text{OH}}\), were placed separately into two closed containers at 298 K and allowed to reach equilibrium.
Ammonia is a weak base.
(i) Deduce the equilibrium constant expression for this reaction.
(ii) Explain the effect on the position of equilibrium and the value of \({K_{\text{c}}}\) when pressure is decreased and temperature is kept constant.
(iii) 2.00 mol of NOCl was placed in a \({\text{1.00 d}}{{\text{m}}^{\text{3}}}\) container and allowed to reach equilibrium at 298 K. At equilibrium, 0.200 mol of NO was present. Determine the equilibrium concentrations of NOCl and \({\text{C}}{{\text{l}}_{\text{2}}}\), and hence calculate the value of \({K_{\text{c}}}\) at this temperature.
(iv) The value of \({K_{\text{c}}}\) is \(1.60 \times {10^{ – 5}}\) at 318 K. State and explain whether the forward reaction is exothermic or endothermic.
(i) Compare the two liquids in terms of their boiling points, enthalpies of vaporization and vapour pressures.
(ii) Explain your answer given for part (b)(i).
Calculate the pH of a \({\text{1.50 mol}}\,{\text{d}}{{\text{m}}^{ – 3}}\) solution of ammonia at 298 K to two decimal places, using Table 15 of the Data Booklet.
A buffer solution is made using \({\text{25.0 c}}{{\text{m}}^{\text{3}}}\) of \({\text{0.500 mol}}\,{\text{d}}{{\text{m}}^{ – 3}}\) hydrochloric acid, HCl (aq), and \({\text{20.0 c}}{{\text{m}}^{\text{3}}}\) of \({\text{1.50 mol}}\,{\text{d}}{{\text{m}}^{ – 3}}\) ammonia solution, \({\text{N}}{{\text{H}}_{\text{3}}}{\text{(aq)}}\).
Describe the meaning of the term buffer solution.
Determine the pH of the buffer solution at 298 K.
A \({\text{1.50 mol}}\,{\text{d}}{{\text{m}}^{ – 3}}\) solution of ammonia is added to \({\text{25.0 c}}{{\text{m}}^{\text{3}}}\) of a \({\text{0.500 mol}}\,{\text{d}}{{\text{m}}^{ – 3}}\) hydrochloric acid solution in a titration experiment.
Calculate the total volume of the solution at the equivalence point.
Calculate the pH of the solution at the equivalence point, using Table 15 of the Data Booklet.
Identify a suitable indicator for this titration, using Table 16 of the Data Booklet.
Answer/Explanation
Markscheme
(i) \(({K_{\text{c}}} = )\frac{{{\text{[C}}{{\text{l}}_2}{\text{(g)][NO(g)}}{{\text{]}}^2}}}{{{{{\text{[NOCl(g)]}}}^2}}}\);
Ignore state symbols.
(ii) equilibrium shifts to right as there are more moles (of gas) on product side;
no change to \({K_{\text{c}}}\) as it is a constant at fixed temperature / OWTTE;
(iii) \({\text{[NOCl(g)]}} = 1.80{\text{ (mol}}\,{\text{d}}{{\text{m}}^{ – 3}}{\text{)}}\);
\({\text{[C}}{{\text{l}}_2}{\text{(g)]}} = 0.100{\text{ (mol}}\,{\text{d}}{{\text{m}}^{ – 3}}{\text{)}}\);
\({K_{\text{c}}} = \left( {\frac{{0.100 \times {{(0.200)}^2}}}{{{{(1.80)}^2}}}} \right)1.23 \times {10^{ – 3}}{\text{ (mol}}\,{\text{d}}{{\text{m}}^{ – 3}}{\text{)}}\);
Award [3] for correct final answer.
(iv) exothermic as \({K_{\text{c}}}\) is lower at higher temperature;
(i) hexane has lower boiling point and enthalpy of vaporization than pentan-1-ol / OWTTE;
hexane has higher vapour pressure than pentan-1-ol / OWTTE;
(ii) hexane is non-polar / has only van der Waals’/London/dispersion forces / has weaker intermolecular forces than pentan-1-ol;
pentan-1-ol has hydrogen bonding between molecules;
\({\text{[O}}{{\text{H}}^ – }{\text{]}} = \sqrt {1.50 \times 1.78 \times {{10}^{ – 5}}} = 5.17 \times {10^{ – 3}}{\text{ (mol}}\,{\text{d}}{{\text{m}}^{ – 3}}{\text{)}}\);
\({\text{pH}} = (14 – {\text{pOH}} = 14 – 2.29 = ){\text{ }}11.71\);
Award [2] for correct final answer.
Accept correct answer with more than 2 decimal places.
solution which resists change in pH / changes pH slightly / OWTTE;
when small amounts of acid or base are added;
\({\text{[N}}{{\text{H}}_3}{\text{] = }}\left( {\frac{{(1.50 \times 0.0200) – (0.500 \times 0.0250)}}{{0.0450}} = } \right){\text{ }}0.389{\text{ (mol}}\,{\text{d}}{{\text{m}}^{ – 3}}{\text{)}}\);
\({\text{[NH}}_4^ + {\text{]}} = \left( {\frac{{(0.500 \times 0.0250)}}{{0.0450}} = } \right){\text{ }}0.278{\text{ (mol}}\,{\text{d}}{{\text{m}}^{ – 3}}{\text{)}}\);
\({\text{[O}}{{\text{H}}^ – }{\text{]}} = \left( {\frac{{{K_b}{\text{[N}}{{\text{H}}_3}{\text{]}}}}{{{\text{[NH}}_4^ + {\text{]}}}} = } \right){\text{ }}\frac{{1.78 \times {{10}^{ – 5}} \times 0.389}}{{0.278}} = 2.49 \times {10^{ – 5}}{\text{ (mol}}\,{\text{d}}{{\text{m}}^{ – 3}}{\text{)}}\);
\({\text{pH}} = (14.0 – {\text{pOH}} = 14.0 – 4.60 = ){\text{ }}9.40\);
OR
\({\text{pOH}} = {\text{p}}{K_b} + \log \frac{{[{\text{NH}}_4^ + ]}}{{{\text{[N}}{{\text{H}}_3}]}}{\text{ = p}}{K_{\text{b}}} + \log \frac{{(12.5/1000)}}{{(17.5/1000)}}\);
\({\text{pOH}} = 4.75 + \log \left( {\frac{{12.5}}{{17.5}}} \right) = 4.75 – 0.146 = 4.604\);
\({\text{pH}} = 14.0 – 4.604 = 9.40\);
Award [4] for the correct final answer.
\(\left( {{\text{V(N}}{{\text{H}}_{\text{3}}}{\text{)}} = \frac{{25.0 \times 0.500}}{{1.50}} = 8.33{\text{ c}}{{\text{m}}^3}} \right)\)
\({\text{V}} = {\text{V(N}}{{\text{H}}_3}{\text{)}} + {\text{V(HCl)}} = 8.33 + 25.0 = 33.3{\text{ c}}{{\text{m}}^3}/0.0333{\text{ d}}{{\text{m}}^3}\);
(\({\text{NH}}_{\text{4}}^ + \) ions are present at equivalence point \({\text{N}}{{\text{H}}_3} + {\text{HCl}} \to {\text{NH}}_4^ + + {\text{C}}{{\text{l}}^ – }\) at equivalence \({\text{n}}({\text{NH}}_4^ + {\text{ produced}}) = {\text{n}}({\text{N}}{{\text{H}}_3}{\text{ added}}) = {\text{n(HCl)}}\))
\([{\text{NH}}_4^ + ] = \frac{{0.500 \times 0.0250}}{{0.0333}} = 0.375{\text{ (mol}}\,{\text{d}}{{\text{m}}^{ – 3}})\);
\({\text{(NH}}_4^ + {\text{(aq)}} \rightleftharpoons {\text{N}}{{\text{H}}_3}{\text{(aq)}} + {{\text{H}}^ + }{\text{(aq)}}/{\text{NH}}_4^ + {\text{(aq)}} + {{\text{H}}_2}{\text{O(l)}} \rightleftharpoons {\text{N}}{{\text{H}}_3}{\text{(aq)}} + {{\text{H}}_3}{{\text{O}}^ + }{\text{(aq)}}\)
\({\text{p}}{K_{\text{a}}}{\text{(NH}}_4^ + ) = 14 – {\text{p}}{K_{\text{b}}}{\text{(N}}{{\text{H}}_3}) = 14.00 – 4.75 = 9.25)\)
\({K_{\text{a}}} = \frac{{{\text{[N}}{{\text{H}}_3}{\text{(aq)][}}{{\text{H}}^ + }{\text{(aq)]}}}}{{{\text{[NH}}_4^ + {\text{(aq)]}}}} = 5.62 \times {10^{ – 10}}\);
\({\text{[}}{{\text{H}}^ + }{\text{(aq)]}} = \sqrt {5.62 \times {{10}^{ – 10}} \times 0.375} = 1.45 \times {10^{ – 5}}{\text{ (mol}}\,{\text{d}}{{\text{m}}^{ – 3}}{\text{)}}\);
\({\text{pH}} = 4.84\);
Award [4] for the correct final answer.
bromocresol green / methyl red;
ECF for answer in 7(c)(v) if pH given is below 7.
Examiners report
The construction and use of equilibrium expressions for \({K_{\text{c}}}\) showed good understanding. The prediction of the effect of increasing pressure on the position of equilibria by applying Le Chatelier’s principle was good, but the fact that \({K_{\text{c}}}\) remains constant at fixed temperatures was less well known.
pH calculations in c(i), c(ii) and c(v) tended to be very good or completely incorrect.
The construction and use of equilibrium expressions for \({K_{\text{c}}}\) showed good understanding. The prediction of the effect of increasing pressure on the position of equilibria by applying Le Chatelier’s principle was good, but the fact that \({K_{\text{c}}}\) remains constant at fixed temperatures was less well known.
pH calculations in c(i), c(ii) and c(v) tended to be very good or completely incorrect.
The construction and use of equilibrium expressions for \({K_{\text{c}}}\) showed good understanding. The prediction of the effect of increasing pressure on the position of equilibria by applying Le Chatelier’s principle was good, but the fact that \({K_{\text{c}}}\) remains constant at fixed temperatures was less well known.
pH calculations in c(i), c(ii) and c(v) tended to be very good or completely incorrect.
The construction and use of equilibrium expressions for \({K_{\text{c}}}\) showed good understanding. The prediction of the effect of increasing pressure on the position of equilibria by applying Le Chatelier’s principle was good, but the fact that \({K_{\text{c}}}\) remains constant at fixed temperatures was less well known.
pH calculations in c(i), c(ii) and c(v) tended to be very good or completely incorrect.
The construction and use of equilibrium expressions for \({K_{\text{c}}}\) showed good understanding. The prediction of the effect of increasing pressure on the position of equilibria by applying Le Chatelier’s principle was good, but the fact that \({K_{\text{c}}}\) remains constant at fixed temperatures was less well known.
pH calculations in c(i), c(ii) and c(v) tended to be very good or completely incorrect.
The construction and use of equilibrium expressions for \({K_{\text{c}}}\) showed good understanding. The prediction of the effect of increasing pressure on the position of equilibria by applying Le Chatelier’s principle was good, but the fact that \({K_{\text{c}}}\) remains constant at fixed temperatures was less well known.
pH calculations in c(i), c(ii) and c(v) tended to be very good or completely incorrect.
The construction and use of equilibrium expressions for \({K_{\text{c}}}\) showed good understanding. The prediction of the effect of increasing pressure on the position of equilibria by applying Le Chatelier’s principle was good, but the fact that \({K_{\text{c}}}\) remains constant at fixed temperatures was less well known.
pH calculations in c(i), c(ii) and c(v) tended to be very good or completely incorrect.
The construction and use of equilibrium expressions for \({K_{\text{c}}}\) showed good understanding. The prediction of the effect of increasing pressure on the position of equilibria by applying Le Chatelier’s principle was good, but the fact that \({K_{\text{c}}}\) remains constant at fixed temperatures was less well known.
pH calculations in c(i), c(ii) and c(v) tended to be very good or completely incorrect.
Question
Acids can be described as strong or weak.
(i) Outline the difference in dissociation between strong and weak acids of the same concentration.
(ii) Describe three tests that can be carried out in the laboratory, and the expected results, to distinguish between \({\text{0.10 mol}}\,{\text{d}}{{\text{m}}^{ – 3}}{\text{ HCl(aq)}}\) and \({\text{0.10 mol}}\,{\text{d}}{{\text{m}}^{ – 3}}{\text{ C}}{{\text{H}}_{\text{3}}}{\text{COOH(aq)}}\).
Calculate the pH, using table 15 of the data booklet, of a solution of ethanoic acid made by dissolving 1.40 g of the acid in distilled water to make a \({\text{500 c}}{{\text{m}}^{\text{3}}}\) solution.
Determine the pH at the equivalence point of the titration and the \({\text{p}}{K_{\text{a}}}\) of an unknown acid using the acid-base titration curve below.
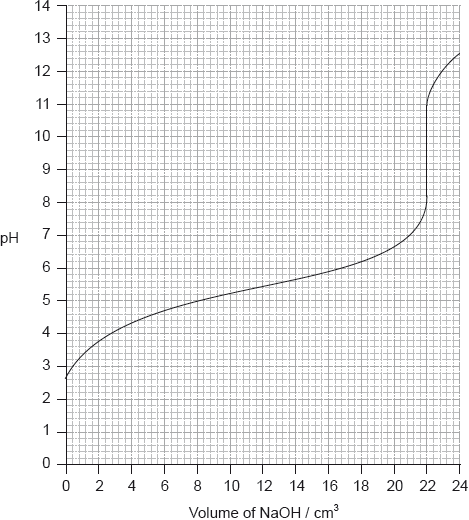
Identify, using table 16 of the data booklet, a suitable indicator to show the end-point of this titration.
Describe how an indicator, that is a weak acid, works. Use Le Chatelier’s principle in your answer.
State the formula of the conjugate base of chloroethanoic acid, \({\text{C}}{{\text{H}}_{\text{2}}}{\text{ClCOOH}}\).
Identify, with a reason, whether chloroethanoic acid is weaker or stronger than ethanoic acid using table 15 of the data booklet.
Determine the pH of the solution resulting when \({\text{100 c}}{{\text{m}}^{\text{3}}}\) of \({\text{0.50 mol}}\,{\text{d}}{{\text{m}}^{ – 3}}\) \({\text{C}}{{\text{H}}_{\text{2}}}{\text{ClCOOH}}\) is mixed with \({\text{200 c}}{{\text{m}}^{\text{3}}}\) of \({\text{0.10 mol}}\,{\text{d}}{{\text{m}}^{ – 3}}\) NaOH.
Describe how chlorine’s position in the periodic table is related to its electron arrangement.
\({\text{SC}}{{\text{l}}_{\text{2}}}\) and \({\text{SCl}}{{\text{F}}_{\text{5}}}\) are two sulfur chloride type compounds with sulfur having different oxidation states. Predict the name of the shape, the bond angle and polarity of these molecules.
Answer/Explanation
Markscheme
(i) weak acids dissociate only partially and strong acids (are assumed to) dissociate fully;
(ii) measuring electrical conductivity and strong acids have greater electrical conductivity/weak acids have lower electrical conductivity;
Do not accept conductivity for electrical conductivity.
Accept explanation in terms of lightbulb in circuit.
measure pH/use universal indicator and pH higher for weak acid/pH lower for strong acid;
conduct titration with a strong base and equivalence point higher for weak acid / buffer region for weak acid;
adding a reactive metal/carbonate/hydrogen carbonate and stronger effervescence/faster reaction with strong acids;
Accept converse argument.
Accept correct example.
adding a strong base and strong acid would increase more in temperature/weak acids increase less in temperature;
Accept correct example.
Award [1 max] for three suitable tests without correct results.
Accept specific examples with given strong acid and weak acid.
Accept “addition of \(AgN{O_3}(aq)\) and white precipitate with HCl (aq)”.
Do not accept “smell”.
\(\frac{{1.40}}{{60.06}} = 0.0233{\text{ (mol)}}\,\,\,\)and\(\,\,\,\frac{{0.0233}}{{0.500}} = 0.0466{\text{ (mol}}\,{\text{d}}{{\text{m}}^{ – 3}})\);
\({\text{(p}}{K_{\text{a}}} = 4.76{\text{)}}{K_{\text{a}}} = 1.7 \times {10^{ – 5}}\);
\({\text{[}}{{\text{H}}^ + }{\text{]}} = \sqrt {{K_{\text{a}}}{\text{[HA]}}} = 8.9 \times {10^{ – 4}}\);
Accept \(9.0 \times 1{0^{ – {\text{4}}}}\).
\({\text{pH}} = 3.05\);
Award [4] for correct final answer.
Accept alternative methods.
Equivalence point: pH of 9.5;
Accept values between 9 and 10.
\({\text{p}}{K_{\text{a}}} = {\text{pH}}\) at half equivalence point;
\({\text{p}}{K_{\text{a}}} = 5.4\);
Accept any value between 5.2 and 5.6.
Award [2] for M2 and M3 if correct \(p{K_a}\) given without explanation.
phenolphthalein;
\({\text{HIn(aq)}} \rightleftharpoons {{\text{H}}^ + }{\text{(aq)}} + {\text{I}}{{\text{n}}^ – }{\text{(aq)}}\) and HIn and \({\text{I}}{{\text{n}}^ – }\) have different colours;
Ignore state symbols.
equilibrium shifts depending on addition of \({{\text{H}}^ + }\) and \({\text{O}}{{\text{H}}^ – }\) / more HIn in acid/low pH / more \({\text{I}}{{\text{n}}^ – }\) in alkali/high pH;
\({\text{C}}{{\text{H}}_{\text{2}}}{\text{ClCO}}{{\text{O}}^ – }\);
stronger because \({\text{p}}{K_{\text{a}}}\) of chloroethanoic acid is \( < {\text{p}}{K_{\text{a}}}\) of ethanoic acid;
Concentration of acid: \(\frac{{0.030}}{{0.300}} = 0.10{\text{ (mol}}\,{\text{d}}{{\text{m}}^{ – 3}}{\text{)}}\);
Concentration of base/salt: \(\frac{{0.020}}{{0.300}} = 0.067{\text{ (mol}}\,{\text{d}}{{\text{m}}^{ – 3}}{\text{)}}\);
\([{{\text{H}}^ + }] = \frac{{{K_{\text{a}}} \times [{\text{HA}}]}}{{[{{\text{A}}^ – }]}}/\frac{{1.3 \times {{10}^{ – 3}} \times 0.10}}{{0.067}}{\text{/}}1.9 \times {10^{ – 3}}{\text{ (mol}}\,{\text{d}}{{\text{m}}^{ – 3}}{\text{)}}\);
\({\text{pH}} = 2.72\);
Award [4] for correct final answer.
Accept 2.69, 2.70 or 2.7.
Alternative for M3 and M4 if Henderson-Hasselbalch equation used:
M3: \(pH = p{K_a} + log\frac{{[base]}}{{[acid]}}{\text{/ }}2.87 + log\left( {\frac{{{\text{0.067}}}}{{0.10}}} \right)\)
M4: \(pH = 2.70\).
Award [1 max] for \({n_{acid}} ( = 100 \times 0.50 \div 1000) = 0.050 mol\) and
\({n_{base}}( = 200 \times 0.10 \div 1000) = 0.020 mol\).
Cl has 7 valence electrons and is in group 7;
Accept “group 17” as suggested by IUPAC.
Cl has 3 occupied (electron) shells/energy levels and so is in period 3;
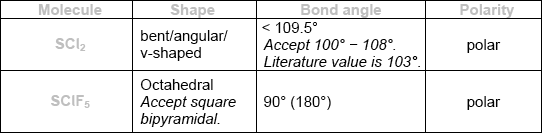
Do not accept ECF for bond angles and polarities from incorrect shapes.
Award [3] for all six correct.
Award [2] for four or five correct.
Award [1] for two or three correct.
Examiners report
There is a difference, which candidates should note, between “not fully dissociated” and “partially dissociated” when describing a weak acid. The latter is correct; the former is not accepted as it could mean anything between 1% and 99%. In (ii), many did not state the difference in behaviour of the two acids. Many gained the first mark in (b) for finding the concentration of ethanoic acid. Thereafter either full marks was obtained – or there was total confusion. The equivalence point in (c) was better known than the \({\text{p}}{K_{\text{a}}}\) where an explanation was expected. The best candidates annotated the graph. Almost all candidates identified phenolphthalein in (ii) correctly but in (iii) any answer that did not begin with an equation was likely to score zero. In questions such as (d) (i), candidates should avoid writing a balanced equation (and there were many) unless the actual answer is clearly indicated. Many were able to identify the stronger acid with the correct reason but in (iii) there were few successful conclusions, many not having recognized that a buffer solution was formed. In (e), most were able to explain why chlorine is in group 7, but the explanation for the period, when it was given, often omitted the idea of occupied shells. In (f), it was disappointing to note that many thought \({\text{SC}}{{\text{l}}_{\text{2}}}\) to be linear and \({\text{SCl}}{{\text{F}}_{\text{5}}}\) trigonal bipyramidal or square pyramidal. Two respondents commented that the column headed “polarity” was confusing; although we could have expressed this more clearly, the candidates did not seem to have a difficulty with this.
Question
A buffer solution with a pH of 3.87 contains \({\text{7.41 g}}\,{\text{d}}{{\text{m}}^{ – 3}}\) of propanoic acid, \({\text{C}}{{\text{H}}_{\text{3}}}{\text{C}}{{\text{H}}_{\text{2}}}{\text{COOH}}\), together with an unknown quantity of sodium propanoate, \({\text{C}}{{\text{H}}_{\text{3}}}{\text{C}}{{\text{H}}_{\text{2}}}{\text{COONa}}\).
Define the term buffer solution.
Explain, using appropriate equations, how this solution acts as a buffer solution.
Calculate the concentration, in \({\text{mol}}\,{\text{d}}{{\text{m}}^{ – 3}}\), of sodium propanoate in this buffer solution.
The \({\text{p}}{K_{\text{a}}}\) of propanoic acid is 4.87 at 298 K.
Answer/Explanation
Markscheme
a solution that resists changes in pH / changes pH slightly / OWTTE;
when small amounts of an acid/\({{\text{H}}^ + }\) or a base/alkali/\({\text{O}}{{\text{H}}^ – }\) are added;
addition of acid:
\({\text{C}}{{\text{H}}_3}{\text{C}}{{\text{H}}_2}{\text{CO}}{{\text{O}}^ – }{\text{(aq)}} + {{\text{H}}^ + }{\text{(aq)}} \to {\text{C}}{{\text{H}}_3}{\text{C}}{{\text{H}}_2}{\text{COOH(aq)}}\) / propanoate ions combine with \({{\text{H}}^ + }\) ions to form undissociated propanoic acid;
addition of base:
\({\text{C}}{{\text{H}}_3}{\text{C}}{{\text{H}}_2}{\text{COOH(aq)}} + {\text{O}}{{\text{H}}^ – }{\text{(aq)}} \to {\text{C}}{{\text{H}}_3}{\text{C}}{{\text{H}}_2}{\text{CO}}{{\text{O}}^ – }{\text{(aq)}} + {{\text{H}}_2}{\text{O(l)}}\) / addition of \({\text{O}}{{\text{H}}^ – }\) removes \({{\text{H}}^ + }\) and more propanoic acid dissociates/ionizes;
Ignore state symbols.
Accept reversible arrows.
Award [1 max] if correct equations are given without reference to addition of acid or alkali.
\({K_{\text{a}}} = \frac{{[{{\text{H}}^ + }{\text{(aq)][C}}{{\text{H}}_3}{\text{C}}{{\text{H}}_2}{\text{CO}}{{\text{O}}^ – }{\text{(aq)]}}}}{{[{\text{C}}{{\text{H}}_3}{\text{C}}{{\text{H}}_2}{\text{COOH(aq)]}}}}/{\text{pH}} = {\text{p}}{K_{\text{a}}} + \log \left( {\frac{{{\text{[base]}}}}{{{\text{[acid]}}}}} \right)\);
\({K_{\text{a}}} = 1.3 \times {10^{ – 5}}/{10^{ – 4.87}}\) and \([{{\text{H}}^ + }] = 1.3 \times {10^{ – 4}}/{10^{ – 3.87}}{\text{ (mol}}\,{\text{d}}{{\text{m}}^{ – 3}}{\text{) }}/\log \frac{{{\text{[C}}{{\text{H}}_3}{\text{C}}{{\text{H}}_2}{\text{CO}}{{\text{O}}^ – }]}}{{[{\text{C}}{{\text{H}}_3}{\text{C}}{{\text{H}}_2}{\text{COOH]}}}} = 3.87 – 4.87 = – 1\);
\(\left( {[{\text{C}}{{\text{H}}_3}{\text{C}}{{\text{H}}_2}{\text{COOH]}} = \frac{{7.41}}{{74.09}} = } \right)0.100/1.00 \times {10^{ – 1}}{\text{ (mol}}\,{\text{d}}{{\text{m}}^{ – 3}}{\text{)}}\);
\({\text{([C}}{{\text{H}}_3}{\text{C}}{{\text{H}}_2}{\text{COONa]}} = )0.010/1.0 \times {10^{ – 2}}{\text{ (mol}}\,{\text{d}}{{\text{m}}^{ – 3}})\);
Award [4] for correct final answer.
Accept corresponding use of \({[{H_3}O]^ + }\) for \([{H^ + }]\), [acid] for \([C{H_3}C{H_2}COOH]\), and [base] or [salt] for \([C{H_3}C{H_2}CO{O^ – }]\) throughout.
Examiners report
Most candidates were able to give a definition of buffer solutions including the detail that pH does not change significantly when small amounts of acid or alkali are added. The explanation of the action of buffers proved to be more challenging with only the stronger candidates giving a complete response in terms of protonation of the conjugate base and increased dissociation of the acid. The calculation of equilibrium concentrations from \({\text{p}}{K_{\text{a}}}\) values was better done than in previous sessions, but still proved too difficult for many. The need to change the units of concentration of propanoic acid made this an additional obstacle in this demanding question.

