Question
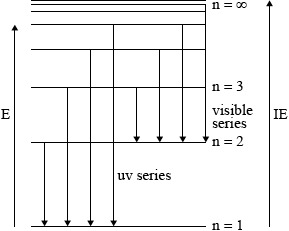
On the above diagram, draw the line that corresponds to the first ionization energy of hydrogen and explain your reasoning.
Answer/Explanation
Markscheme
for showing the energy to remove electron from \({\text{n}} = 1\) to \({\text{n}} = \infty \) on the above diagram;
to ionize an element, electron must be removed from the atom/no longer under influence of nucleus/removed beyond \({\text{n}} = \infty \) / OWTTE;
Examiners report
Candidates in some schools, however, appeared not to have encountered these ideas at all. Common errors were to label the first energy level as\({\text{n}} = 0\) rather than\({\text{n}} = 1\) and to only include one transition for each series. Sometimes the arrows showing the transitions were shown from the bottom up.
While more candidates managed to obtain at least a mark with regard to the first ionization energy of hydrogen, it was less common to find the correct graphical representation of the first IE with diagrams which often were unrelated.
Question
Lithium and boron are elements in period 2 of the periodic table. Lithium occurs in group 1 (the alkali metals) and boron occurs in group 3. Isotopes exist for both elements.
The electron configuration of boron is \({\text{1}}{{\text{s}}^{\text{2}}}{\text{2}}{{\text{s}}^{\text{2}}}{\text{2}}{{\text{p}}^{\text{1}}}\). Draw the shape of an s orbital and a \({{\text{p}}_x}\) orbital on the axes below.
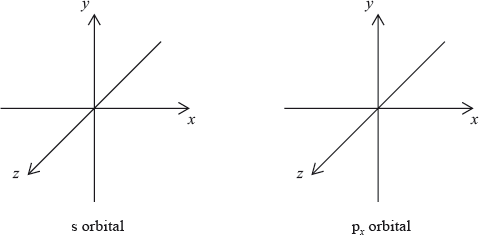 [1]
[1]
(ii) Cobalt is a transition metal. One common ion of cobalt is \({\text{C}}{{\text{o}}^{3 + }}\). Draw the orbital diagram (using the arrow-in-box notation) for the \({\text{C}}{{\text{o}}^{3 + }}\) ion.

(iii) State the other most common ion of cobalt.
(iv) Explain why the complex \({\text{[Co(N}}{{\text{H}}_{\text{3}}}{{\text{)}}_{\text{6}}}{\text{]C}}{{\text{l}}_{\text{3}}}\) is coloured.[5]
Answer/Explanation
Markscheme
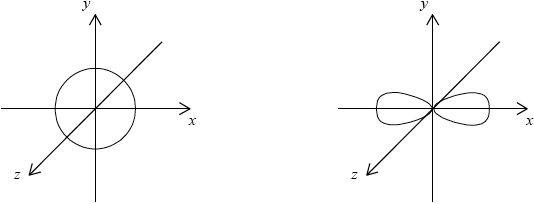
symmetrical shape of s orbital and dumbbell-shaped p orbital with electron density along x-axis;
(ii) 
Allow full arrows instead of half-arrows for example \( \uparrow \downarrow \).
Do not allow arrows with the same spin for example \( \uparrow \uparrow \) or \( \downarrow \downarrow \) in the same orbital.
Do not allow an orbital diagram with a \(4{s^1}3{d^5}\) configuration.
(iii) \({\text{C}}{{\text{o}}^{2 + }}\);
Accept +2, 2+, cobalt(II), II.
(iv) partially filled/incomplete d subshell/sub-level/orbitals;
d orbitals split (into two sets of different energies);
(colour due to) electron transition between (split) d orbitals / d to d transitions / frequencies of visible light absorbed by electrons moving from lower to higher d levels ;
colour due to remaining frequencies / complementary colour seen;
Allow wavelength as well as frequency.
Examiners report
In part (iii), a common mistake involved candidates drawing the lobe of electron density around the y or z axes for the \({{\text{p}}_x}\) orbital. Some candidates drew three dumbells for the s-orbital. Other candidates incorrectly drew hybrid orbitals.
The orbital diagram in (ii) also proved to be quite a good discriminating question. Many candidates failed to realise that the electrons are removed from the 4s level before the 3d for a first-row transition metal ion. In addition, a significant number of candidates showed poor understanding of Hund‟s Rule of Maximum Multiplicity which states that when degenerate orbitals are available, electrons fill the orbitals singly before filling them in pairs. Hence, in many cases incorrect representations were seen for the 3d which involved three pairs of electrons of opposite spin being inserted in three 3d orbitals. Most candidates stated the \({\text{C}}{{\text{o}}^{2 + }}\) ion, though a common incorrect answer was \({\text{C}}{{\text{o}}^{4 + }}\). Part (iv) involved candidates having to explain why the complex \({\text{[Co(N}}{{\text{H}}_{\text{3}}}{{\text{)}}_{\text{6}}}{\text{]C}}{{\text{l}}_{\text{3}}}\) is coloured. This question was asked a number of times in previous examinations and previously was typically really very poorly answered. In N12, the explanations certainly were better though some candidates mixed up the principles of the line emission spectrum of hydrogen with the d to d transitions involved in the explanation of colour pertaining to a transition metal complex.
